迭代函数方程的同胚解

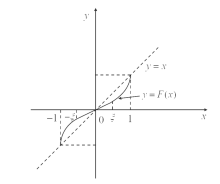
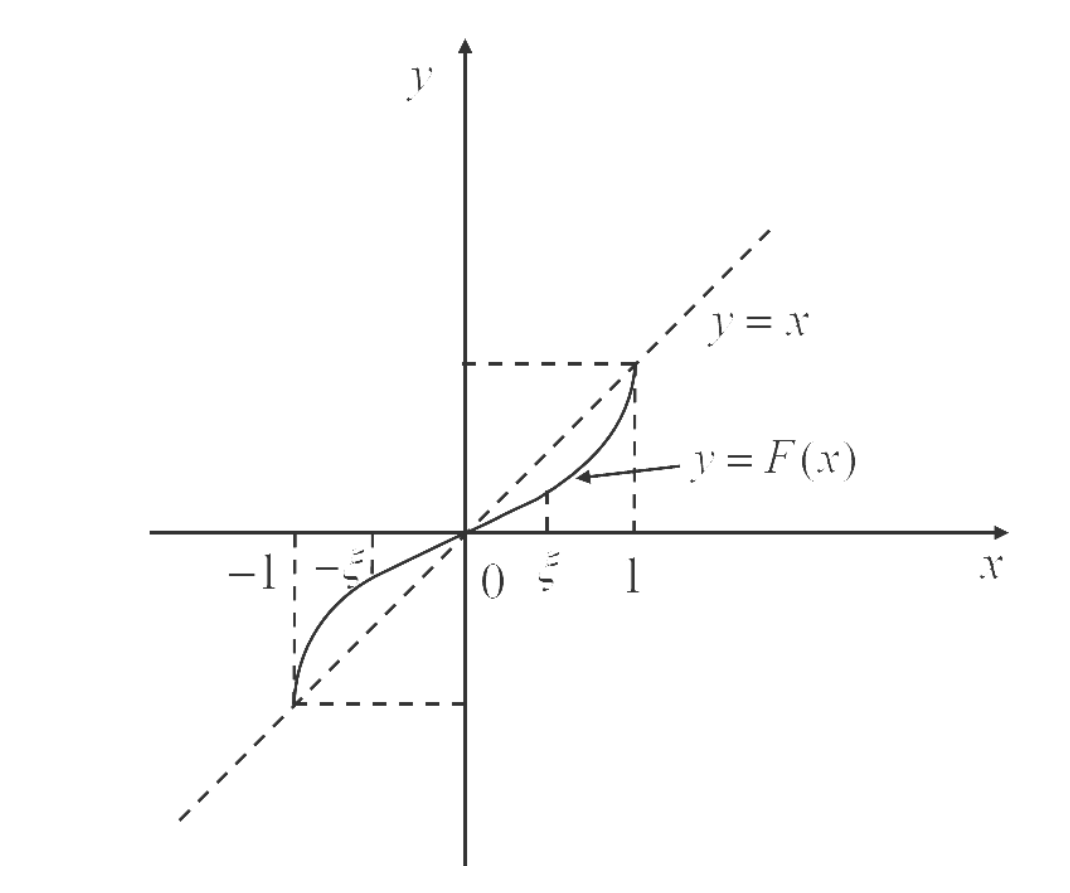
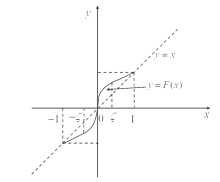
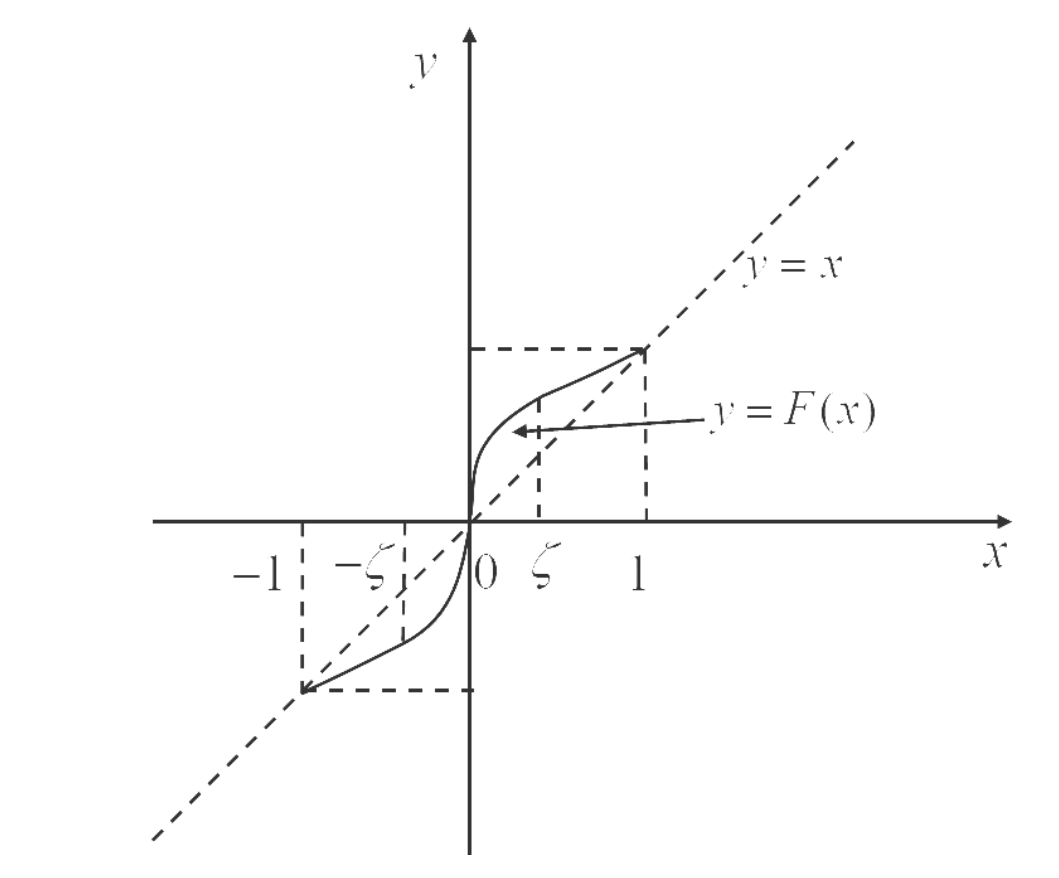
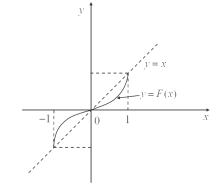
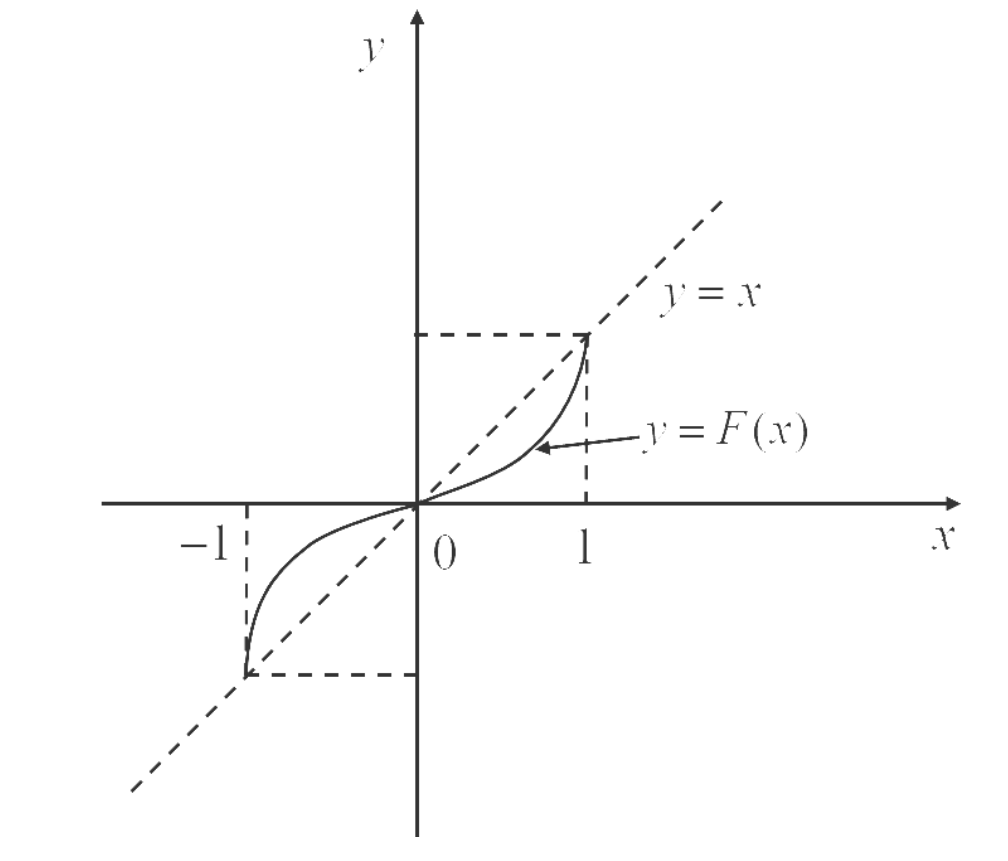
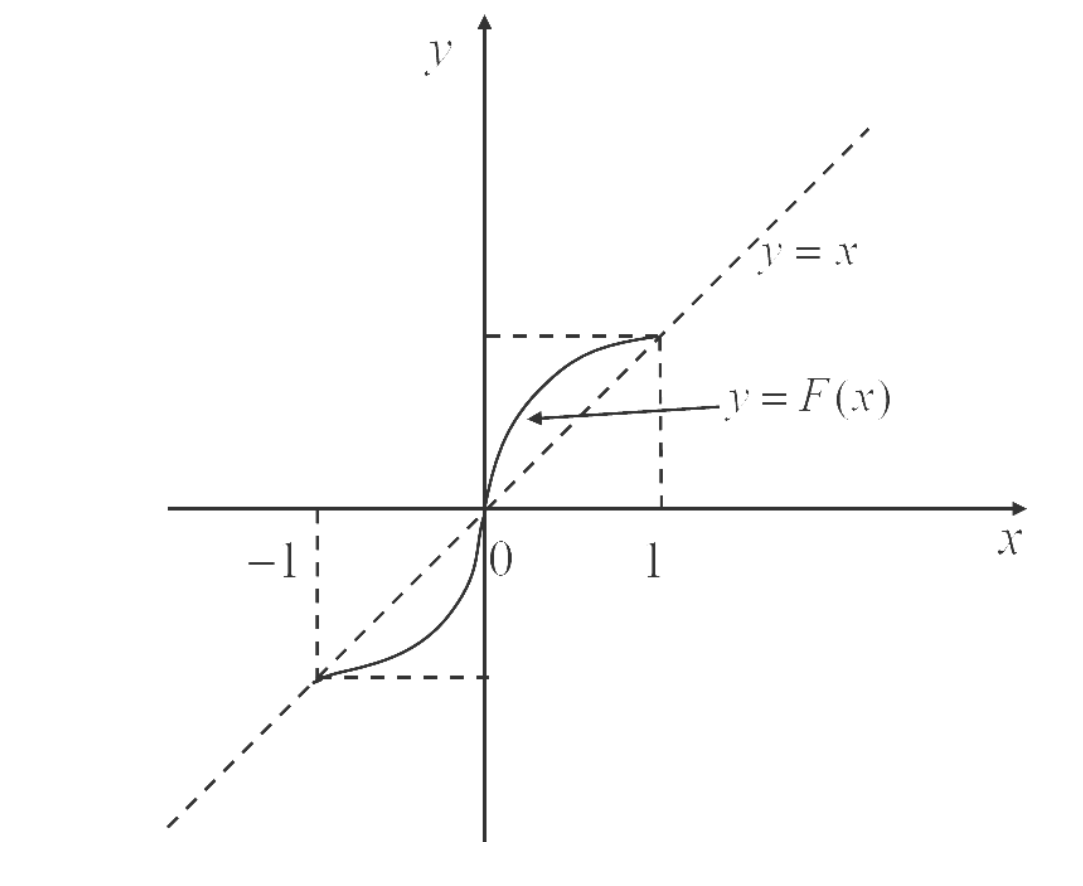
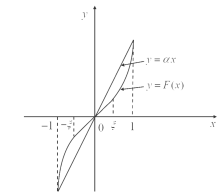
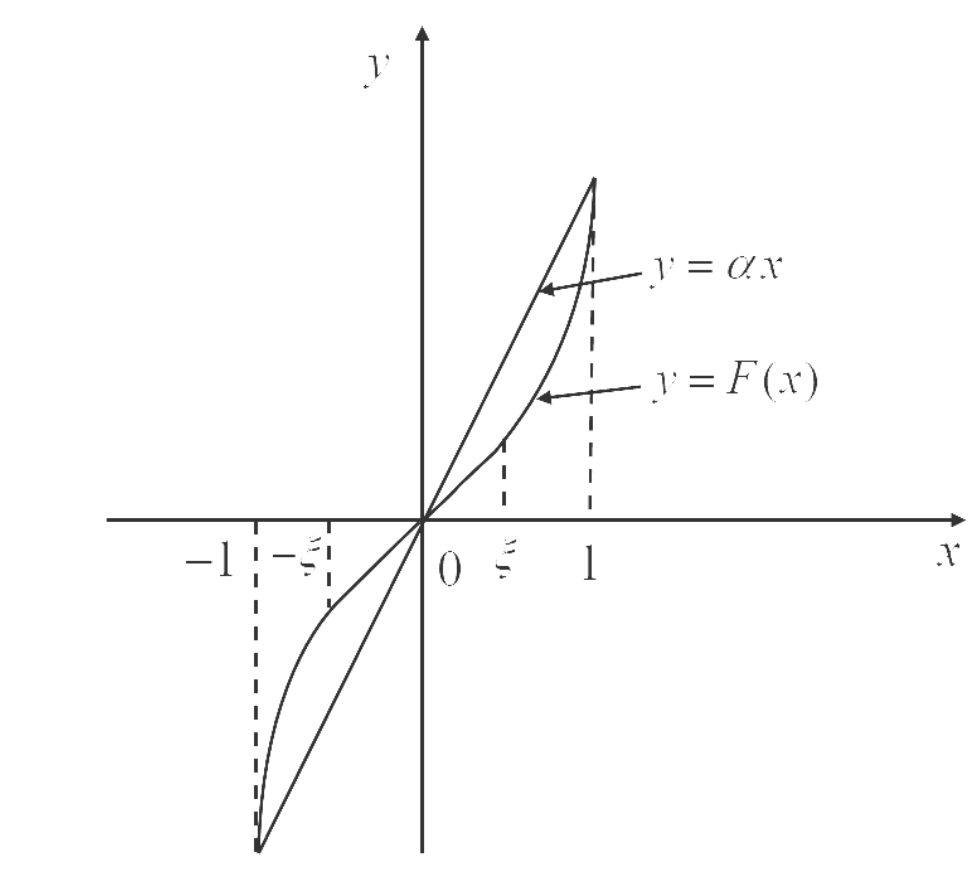
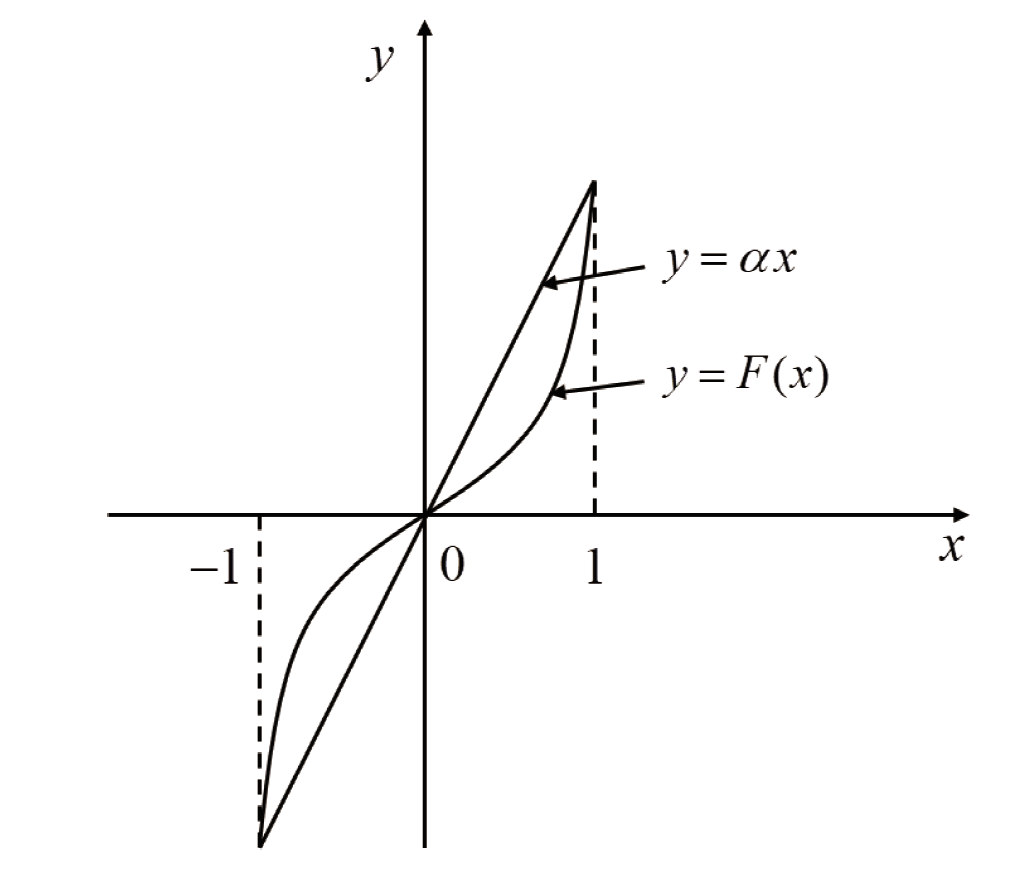
数学物理学报 ›› 2024, Vol. 44 ›› Issue (2): 313-325.

迭代函数方程的同胚解

刘敬华¹,李林^2,^*()

¹韩山师范学院数学与统计学院广东潮州 521041
²嘉兴学院数学系浙江嘉兴 314001

收稿日期:2022-08-11 修回日期:2023-10-14 出版日期:2024-04-26 发布日期:2024-04-07
通讯作者: * 李林,Email:matlinl@zjxu.edu.cn.
基金资助:
浙江省自然科学基金(LY18A010017);国家自然科学基金(12026207);韩山师范学院博士启动项目(QD202210)

Homeomorphic Solutions of Iterative Functional Equations

Liu Jinghua¹,Li Lin^2,^*()

¹School of Mathematics and Statistics, Hanshan Normal University, Guangdong Chaozhou 521041
²Faculty of Mathematics, Jiaxing University, Zhejiang Jiaxing 314001

Received:2022-08-11 Revised:2023-10-14 Online:2024-04-26 Published:2024-04-07
Supported by:
Zhejiang Provincial Natural Science(LY18A010017);National Natural Science Foundatjion of China(12026207);Doctoral Initiation Project of Hanshan Normal University(QD202210)

摘要/Abstract

摘要：

已有的迭代函数方程解结论主要是关于它的连续性和光滑性. 然而在函数方程与动力系统的共轭和线性化理论研究中, 解的同胚性质起到重要作用. 对这一问题虽然已取得部分结论, 但还未有一个完整的描述. 该文首先利用构造和逼近的方法考虑一般类型的迭代函数方程的同胚解, 然后将得到的结论应用到研究多项式型迭代方程.

关键词: 迭代函数方程, 同胚解, 局部线性函数, 一致收敛, 齐次函数

Abstract:

Most known results on the solutions of iterative functional equations are about their continuity and smoothness. However, concerning the study of conjugation and linearization in functional equations and dynamical systems, the property of homeomorphism play an important role in these theories. Although partial results were obtained, a full description is still open. In this paper, we first consider homeomorphic solutions to a general iterative functional equation by the methods of construction and approximation. Then, the obtained results are used to study the solutions of polynomial-like iterative functional equations.

Key words: Iterative equation, Homeomorphic solution, Locally linear function, Uniform convergence, Homogeneous function

中图分类号:

O175.23

刘敬华, 李林. 迭代函数方程的同胚解[J]. 数学物理学报, 2024, 44(2): 313-325.

Liu Jinghua, Li Lin. Homeomorphic Solutions of Iterative Functional Equations[J]. Acta mathematica scientia,Series A, 2024, 44(2): 313-325.

图/表 10

图 1

图 2

图 3

图 4

图 5

图 6

图 7

图 8

图 9

图 10

参考文献 35

[1]	Baron K, Jarczyk W. Recent results on functional equations in a single variable perspectives and open problems. Aequationes Math, 2001, 61: 1-48
[2]	Bessis D, Marmi S, Turchetti G. On the singularities of divergent majorant series arising from normal form theory. Rend Mat Appl, 1989, 9: 645-659
[3]	Block L, Coven M. Topological conjugacy and transitivity for a class of piecewise monotone maps of the interval. Trans Amer Math Soc, 1987, 300: 297-306
[4]	Chen Jingmin, Zhang Weinian. Leading coefficient problem for polynomial-like iterative equations. J Math Anal Appl, 2009, 349: 413-419
[5]	Draga S, Morawiec J. Reducing the polynomial-like iteative equations order and a generalized Zoltán Boros' Problem. Aequationes Math, 2016, 90: 935-950
[6]	Draga S, Morawiec J. Mean of iterates. Aequationes Math, 2019, 93: 21-35
[7]	Fontich E. Transversal homoclinic points of a class of conservative diffeomorphisms. J Differential Equations, 1990, 87: 1-27
[8]	Greenfield S J, Nussbaum R D. Dynamics of a quadratic map in two complex variables. J Differential Equations, 2001, 169: 57-141
[9]	Hu J, Sullivan D P. Topological conjugacy of circle diffeomorphisms. Ergodic Theory Dyn Syst, 1997, 17(1): 173-186 doi: 10.1017/S0143385797061002
[10]	Jarczyk W. On an equation of linear iteration. Aequationes Math, 1996, 51: 303-310
[11]	Kuczma M, Choczewski B, Ger R. Iterative Functional Equations. Encyclopedia Math Appl, Vol 32. Cambridge:Cambridge University Press, 1990
[12]	Lanford O. A computer-assisted proof of the Feigenbaum conjectures. Bull Amer Math Soc (NS), 1982, 6: 427-434
[13]	Li L, Song W. Global solutions for leading coefficient problem of a general iterative equation. Results Math, 2015, 68(1/2): 247-260 doi: 10.1007/s00025-014-0432-0
[14]	Li L, Zhang W M. Continuously decreasing solutions for polynomial-like iterative equations. Sci China Ser A, 2013, 56: 1051-1058
[15]	Li M R, Zhao H Y. Strongly convex solutions of polynomial-like iterative equation. J Math Anal Appl, 2021, 495(2): 124786 doi: 10.1016/j.jmaa.2020.124786
[16]	Liu J H. Analytical invariant curves for a second order difference equation modeled from macroeconomics under the Brjuno condition. J Difference Equ Appl, 2017, 3: 648-656
[17]	Liu J H, Yu Z H, Zhang W N. Invariant curves for a second order difference equation modeled from macroeconomics. J Difference Equ Appl, 2015, 9: 757-773
[18]	Matkowski J, Weinian Z. Method of characteristic for function equations in polynomial form. Acta Math Sinica, 1997, 13: 421-432
[19]	McCarthy P. The general exact bijective continuous solution of Feigenbaum's functional equation. Comm Math Phys, 1983, 91: 431-443
[20]	Nabeya S. On the functional equation $ f(p + qx + rf(x)) = a + bx + cf(x) $. Aequationes Math, 1974, 11: 199-211
[21]	Parry W. Symbolic dynamics and transformations of the unit interval. Trans Amer Math Soc, 1966, 122: 368-378
[22]	Song W, Li L. Continuously decreasing solutions for a general iterative equation. Acta Mathematica Scientia, 2018, 38B: 177-186
[23]	Tabor J, Tabor J. On a linear iterative equation. Results Math, 1995, 27: 412-421
[24]	Xu B, Zhang W N. Construction of continuous solutions and stability for the polynomial-like iterative equation. J Math Anal Appl, 2007, 325: 1160-1170
[25]	Yang D L, Zhang W N. Characteristic solutions of polynomial-like iterative equations. Aequationes Math, 2004, 67: 80-105
[26]	Yang L, Zhang J Z, The second type of Feigenbaum's functional equation. Sci China Ser A, 1986, 29: 1252-1262
[27]	Zhang J Z, Yang L, Zhang W N. Some advances on functional equations. Adv Math (China), 1995, 24: 385-405
[28]	Zhang W N. Discussion on the iterated equation $ \Sigma_{i=1}^{n}\lambda_{i}f^{i}(x)=F(x) $. Chinese Sci Bull, 1987, 32: 1444-1451
[29]	Zhang W N. Discussion on the differentiable solutions of the iterated equation $ \Sigma_{i=1}^{n}\lambda_{i}f^{i}(x)=F(x) $. Nonlinear Anal, 1990, 15: 387-398
[30]	Zhang W N, Baker J. Continuous solutions of a polynomial-like iterative equation with variable coefficients. Ann Polon Math, 2000, 73: 29-36
[31]	Zhang W N, Nikodem K, Xu B. Convex solutions of polynomial-like iterative equations. J Math Anal Appl, 2006, 315: 29-40
[32]	Zhang W M, Xu B, Zhang W N. Global solutions for leading coefficient problem of polynomial-like iterative equations. Results Math, 2013, 63: 79-93
[33]	Zhang W M, Zhang W N. On continuous solutions of $ n $-th order polynomial-like iterative equations. Publ Math Debrecen, 2010, 76: 117-134
[34]	Zhang W M, Zhang W N. $ C^{1} $ linearization for planar contractions. J Funct Anal, 2011, 260: 2043-2063 doi: 10.1016/j.jfa.2010.12.029
[35]	Zhang W M, Zhang W N, Jarczyk W. Sharp regularity of linearization for $ C^{1,1} $ hyperbolic diffeomorphisms. Math Ann, 2014, 358: 69-113