数学物理学报 ›› 2024, Vol. 44 ›› Issue (2): 298-312.
含参数 Nabla 积分比和变限含参数 Nabla 积分比的单调性法则及其应用
- 华北电力大学数理学院 河北省物理学与能源技术重点实验室 河北保定 071003
-
收稿日期:2023-05-22修回日期:2023-09-28出版日期:2024-04-26发布日期:2024-04-07 -
通讯作者:* 孙龙发, Email:sun.longfa@163.com -
作者简介:田景峰, Email:tianjf@ncepu.edu.cn ;毛忠旋, Email:maozhongxuan000@gmail.com -
基金资助:国家自然科学基金(12101234);河北省自然科学基金(A2022502010);中央高校基本科研业务费专项资金(2023MS164);国家留学基金
Monotonicity Rules of the Ratios of Parametric Nabla Integrals and Parametric Nabla Integrals with Variable Limits and Their Applications
Tian Jingfeng( ),Mao Zhongxuan(
),Mao Zhongxuan( ),Sun Longfa*(
),Sun Longfa*( )
)
- Hebei Key Laboratory of Physics and Energy Technology, School of Mathematics and Physics, North China Electric Power University, Hebei Baoding 071003
-
Received:2023-05-22Revised:2023-09-28Online:2024-04-26Published:2024-04-07 -
Supported by:National Natural Science Foundation of China(12101234);Natural Science Foundation of Hebei Province(A2022502010);Fundamental Research Funds for the Central Universities(2023MS164);China Scholarship Council
摘要:
利用时标理论中的 Nabla 积分建立了含参数 Nabla 积分比


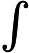


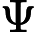







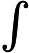


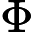






 和
和

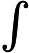

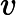

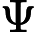







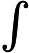

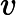

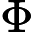







以及变限含参数 Nabla 积分比


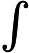














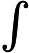













 和
和

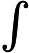

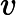













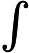

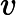













的单调性法则. 在含参数 Nabla 积分比部分中, 还详细研究了一些特殊情形, 包括时标下的多项式之比以及 Nabla 拉普拉斯变换之比. 利用这些单调性法则, 证明了函数 






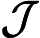
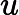





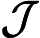
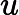











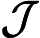
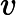






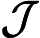
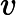












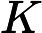
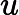





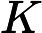
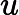











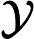
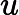





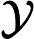
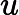











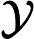
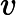






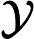
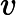




























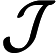

















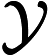

















中图分类号:
- O171
引用本文
田景峰, 毛忠旋, 孙龙发. 含参数 Nabla 积分比和变限含参数 Nabla 积分比的单调性法则及其应用[J]. 数学物理学报, 2024, 44(2): 298-312.
Tian Jingfeng, Mao Zhongxuan, Sun Longfa. Monotonicity Rules of the Ratios of Parametric Nabla Integrals and Parametric Nabla Integrals with Variable Limits and Their Applications[J]. Acta mathematica scientia,Series A, 2024, 44(2): 298-312.
使用本文
| [1] | Biernacki M, Krzyż J. On the monotonicity of certain functionals in the theory of analytic functions. Ann Univ Mariae Curie-Skł lodowska, Sect A, 1955, 9: 135-147 |
| [2] | Cheeger J, Gromov M, Taylor J. Finite propagation speed, kernel estimates for functions of the Laplace operator, and the geometry of complete Riemannian manifolds. J Differ Geom, 1982, 17(1): 15-53 |
| [3] |
Yang Z H, Chu Y M, Wang M K. Monotonicity criterion for the quotient of power series with applications. J Math Anal Appl, 2015, 428(1): 587-604
doi: 10.1016/j.jmaa.2015.03.043 |
| [4] |
Yang Z H, Tian J F. Monotonicity rules for the ratio of two Laplace transforms with applications. J Math Anal Appl, 2019, 470(2): 821-845
doi: 10.1016/j.jmaa.2018.10.034 |
| [5] | Qi F. Decreasing properties of two ratios defined by three and four polygamma functions. C R Math Acad Sci Paris, 2022, 360: 89-101 |
| [6] | Mao Z X, Tian J F. Monotonicity and complete monotonicity of some functions involving the modified Bessel functions of the second kind. C R Math Acad Sci Paris, 2023, 361: 217-235 |
| [7] |
董迎辉, 徐亚娟. 障碍分红策略下的相关双边跳扩散模型. 数学学报, 2014, 57(3): 581-592
doi: 10.12386/A2014sxxb0054 |
|
Dong Y H, Xu Y J, Correlated two-sided jump-diffusion model under the barrier dividend. Acta Math Sin, 2014, 57(3): 581-592
doi: 10.12386/A2014sxxb0054 |
|
| [8] | 邓继勤, 邓子明. 分数阶微分方程非局部柯西问题解的存在和唯一性. 数学物理学报, 2016, 36A(6): 1157-1164 |
| Deng J Q, Deng Z M. Existence and uniquencess of solutions for nonlocal cauchy problem for fractional evolution equations. Acta Math Sci, 2016, 36A(6): 1157-1164 | |
| [9] | 郭庭光, 徐志庭. 带有扩散项和接种的传染病模型的行波解. 数学物理学报, 2017, 37A(6): 1129-1147 |
| Guo T G, Xu Z T. Existence of traveling waves in a spatial infectious disease model with vaccination. Acta Math Sci, 2017, 37A(6): 1129-1147 | |
| [10] | 孙海江, 王延杰, 刘伟宁. 基于自适应平台阈值和拉普拉斯变换的红外图像增强. 中国光学, 2011, 4(5): 474-479 |
| Sun H J, Wang Y J, Liu W N. Enhancement of infrared images based on adaptive platform threshold and Laplace transformation. Chin Optics, 2011, 4(5): 474-479 | |
| [11] | 苗启广, 王宝树. 基于改进的拉普拉斯金字塔变换的图像融合方法. 光学学报, 2007, 27(9): 1605-1610 |
| Miao Q G, Wang B S. Multi-Sensor image fusion based on improved Laplacian pyramid transform. Acta Optical Sin, 2007, 27: 1605-1610 | |
| [12] |
Sahbani J. Quantitative uncertainty principles for the canonical Fourier-Bessel transform. Acta Math Sin (Engl Ser), 2022, 38(2): 331-346
doi: 10.1007/s10114-022-1008-7 |
| [13] |
Jain P, Basu C, Panwar V. On the (   |
| [14] |
Weiss L, McDonough R N. Prony's method,  doi: 10.1137/1005035 |
| [15] | Hilger S. Ein Maßkettenkalkül mit anwendung auf zentrumsmannigfaltigkeiten[D]. Würzburg: Universität Würzburg, 1988 |
| [16] | Bohner M, Peterson A. Dynamic Equations on Time Scales. Boston: Springer, 2001 |
| [17] | Bohner M, Peterson A. Advances in Dynamic Equations on Time Scales. Boston: Springer, 2003 |
| [18] | Bohner M, Georgiev S G. Multivariable Dynamic Calculus on Time Scales. Cham: Springer, 2016 |
| [19] | 欧柳曼, 朱思铭. 时标动力方程的稳定性分析. 数学物理学报, 2008, 28A(2): 308-319 |
| Ou L M, Zhu S M, Stable analysis for dynamic equations on time scales. Acta Math Sci, 2008, 28A(2): 308-319 | |
| [20] | 罗华. 时标线性加权 Sturm-Liouville 特征值问题的谱理论分析. 数学物理学报, 2017, 37A(3): 427-449 |
| Luo H. Spectral theory of linear weighted Sturm-Liouville eigenvalue problems. Acta Math Sci, 2017, 37A(3): 427-449 | |
| [21] |
李继猛, 杨甲山. 时标上二阶拟线性延迟阻尼动态系统的动力学行为分析. 应用数学学报, 2020, 43(5): 853-864
doi: 10.12387/C2020062 |
|
Li J M, Yang J S. Dynamical behavior of second-order quasilinear delay damped dynamic equations on time scales. Acta Math Appl Sin, 2020, 43(5): 853-864
doi: 10.12387/C2020062 |
|
| [22] |
张萍, 杨甲山. 时标上具正负系数的三阶阻尼动力方程的振动性. 应用数学学报, 2021, 44(5): 632-645
doi: 10.12387/C2021045 |
|
Zhang P, Yang J S. Oscillation of third-order damped dynamic equations with positive and negative coefficients on time scales. Acta Math Appl Sin, 2021, 44(5): 632-645
doi: 10.12387/C2021045 |
|
| [23] | Matthews T. Probability theory on time scales and applications to finance and inequalities[D]. Rolla: Missouri University of Science and Technology, 2011 |
| [24] | Georgiev S G, Erhan İ M. The Taylor series method and trapezoidal rule on time scales. Appl Math Comput, 2020, 378: 125200 |
| [25] | Georgiev S G, Erhan İ M. Lagrange interpolation on time scales. J Appl Anal Comput, 2022, 12(4): 1294- 1307 |
| [26] |
Bartosiewicz Z, Pawluszewicz E. Realizations of nonlinear control systems on time scales. IEEE T Automat Contr, 2008, 53(2): 571-575
doi: 10.1109/TAC.9 |
| [27] | 詹再东. 时标型动态微分系统的最优控制问题及其应用[D]. 杭州: 浙江大学, 2012 |
| Zhan Z D. Optimal control problem governed by dynamic differential systems on arbitary time scales and applications[D]. Hangzhou: Zhejiang University, 2012 | |
| [28] |
Xiao Q, Zeng Z. Scale-limited lagrange stability and finite-time synchronization for memristive recurrent neural networks on time scales. IEEE T Cybernetics, 2017, 47(10): 2984-2994
doi: 10.1109/TCYB.2017.2676978 pmid: 28362603 |
| [29] | 肖强. 时标型网络系统的动力学分析与控制[D]. 武汉: 华中科技大学, 2019 |
| Xiao Q. Dynamic analysis and control of timescale-type networked system[D]. Wuhan: Huazhong University of Science and Technology, 2019 | |
| [30] |
Davis J M, Gravagne I A, Marks R J. Convergence of unilateral Laplace transforms on time scales. Circ Syst Signal Pr, 2010, 29(5): 971-997
doi: 10.1007/s00034-010-9182-8 |
| [31] | Benkhettou N, Brito da Cruz A, Torres D. A fractional calculus on arbitrary time scales: Fractional differentiation and fractional integration. Signal Process, 2015, 107: 230-237 |
| [32] | 王竹溪, 郭敦仁. 特殊函数概论. 北京: 北京大学出版社, 2000 |
| Wang Z X, Guo D R. Introduction to Special Functions. Beijing: Peking University Press, 2000 | |
| [33] | Nikiforov A F, Uvarov V B. Special Functions of Mathematical Physics. Basel: Birkhäuser Verlag, 1988 |
| [34] | Rahmat M R S. Integral transform methods for solving fractional dynamic equations on time scales. Abstr Appl Anal, Hindawi, 2014, 2014: 261348 |
| [35] | Anderson D R. Taylor polynomials for nabla dynamic equations on time scales. Panamer Math J, 2002, 12(4): 17-28 |
| [36] |
Yang Z H, Qian W M, Chu Y M, et al. Monotonicity rule for the quotient of two functions and its application. J Inequal Appl, 2017, 2017: 1-13
doi: 10.1186/s13660-017-1388-x |
| [1] | 邱仰聪, 王其如. 一阶非线性时标动态方程的 Hyers-Ulam-Rassias 稳定性[J]. 数学物理学报, 2024, 44(2): 465-475. |
| [2] | 邱仰聪,王其如. 二阶非线性中立型时标动态方程趋向于零的非振动解的存在性[J]. 数学物理学报, 2021, 41(2): 382-387. |
| [3] | 杨晓凤,董华,戴洪帅. 混合观测体系下谱负Lévy过程的Parisian破产问题[J]. 数学物理学报, 2021, 41(2): 548-561. |
| [4] | 张萍,杨甲山. 时标上具非正中立项的二阶动力方程的动力学性质[J]. 数学物理学报, 2021, 41(1): 217-226. |
| [5] | 邓栋,李燕. 一类带治疗项的非局部扩散SIR传染病模型的行波解[J]. 数学物理学报, 2020, 40(1): 72-102. |
| [6] | 张万路, 殷晓龙, 赵翔华. 对偶延迟更新风险模型的占位时[J]. 数学物理学报, 2019, 39(4): 918-931. |
| [7] | 郭庭光, 徐志庭. 带有扩散项和接种的传染病模型的行波解[J]. 数学物理学报, 2017, 37(6): 1129-1147. |
| [8] | 李周红, 张丰硕, 曹进德, Alsaedi Ahmed, Alsaadi Fuad E. 时标上带有反馈控制的非自治两种群竞争系统的概周期解[J]. 数学物理学报, 2017, 37(4): 730-750. |
| [9] | 罗华. 时标线性加权Sturm-Liouville特征值问题的谱理论分析[J]. 数学物理学报, 2017, 37(3): 427-449. |
| [10] | 邓继勤, 邓子明. 分数阶微分方程非局部柯西问题解的存在和唯一性[J]. 数学物理学报, 2016, 36(6): 1157-1164. |
| [11] | 陈大学. 具有振动系数的二阶非线性中立型时滞动力方程的有界振动性[J]. 数学物理学报, 2013, 33(1): 98-113. |
| [12] | 王五生, 周效良. 时标上的推广的Pachpatte型不等式及其在边值问题中的应用[J]. 数学物理学报, 2012, 32(2): 404-413. |
| [13] | 冯由玲. 交换Banach代数中线性时标动态方程的解[J]. 数学物理学报, 2011, 31(2): 439-446. |
| [14] | 李永昆, 张洪涛. 一类时标上具状态依赖时滞的中立型泛函微分方程的周期正解[J]. 数学物理学报, 2010, 30(3): 730-742. |
| [15] | 赵翔华, 尹传存. 一类索赔相关且带干扰的风险模型[J]. 数学物理学报, 2009, 29(6): 1657-1664. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 115
|
|
|||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 88
|
|
|||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||
|
