非线性非一致介质二维Maxwell方程leap-frog Crank-Nicolson多区域Legendre-tau配置谱方法

摘要/Abstract

摘要：

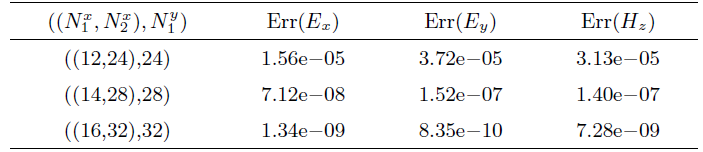
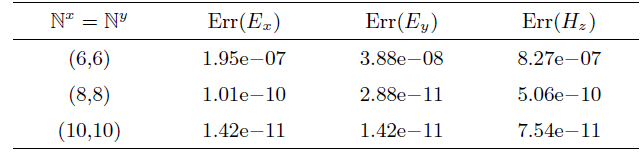
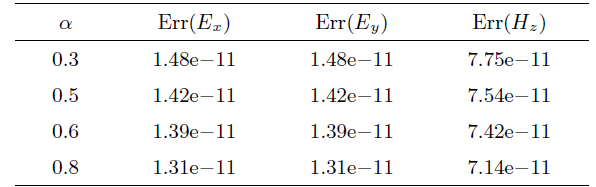
该文研究了具有非线性电导率的非一致介质二维Maxwell方程的数值求解方法, 提出了多区域Legendre-tau配置谱方法. 该数值方法空间上达到谱精度, 时间上是二阶精度. 时间方向采用leap-frog Crank-Nicolson三层格式进行离散. 非线性项放在中间已知层采用谱配置法显式处理, 线性项采用Legendre谱方法隐式处理. 利用显隐数值格式, 既有较好的稳定性又方便算法实施. 基于合理的弱形式,不需要使用额外附加的连接条件, 以自然边界条件的方式处理交界面条件. 定义不同次数多项式逼近空间, 构建一致的数值格式. 详细证明了半离散和全离散数值格式的稳定性和收敛性, 并得到$L^2$ -范数的最优误差估计. 算例中, 利用快速Legendre变换在Chebyshev点上计算非线性项, 提高算法效率. 数值结果证实了该数值方法求解此类非线性问题的有效性, 并且没有因为解的间断而损失谱精度.

关键词: 二维Maxwell方程, 非线性电导率, 非一致介质, 多区域Legendre-tau谱方法, leap-frog Crank-Nicolson 方法, 最优误差估计

Abstract:

In this paper, numerical methods for solving 2D nonlinear Maxwell's equations in inhomogeneous media are discussed. A multidomain Legendre-tau collocation spectral method is proposed. The proposed method is of spectral accuracy in space and second order in time. In time direction, the leap-frog Crank-Nicolson method is applied, which is a three-level scheme with the nonlinear term being treated by some collocation methods explicitly in intermediate level and the linear terms being treated by the Legendre-tau spectral method implicitly. By the implicit-explicit scheme, the numerical method is of better stability and easy implementation. We construct a reasonable weak form which can deal with the interface conditions in a way just like the natural boundary condition without any additional interface conditions. The uniform scheme without any additional interface conditions is constructed by using polynomial spaces of different degrees. For the semi-discrete and fully discrete schemes, the stability and convergence are proved, and the $L^2$-norm optimal error estimates are obtained. In numerical examples, the nonlinear terms are computed at the Chebyshev points by the fast Legendre transform to improve the efficiency of the algorithm. Numerical results show the efficiency of the proposed method for solving this nonlinear problem. Moreover, the results indicate that the spectral accuracy is achieved and not affected by the discontinuity of solutions.

Key words: 2D Maxwell's equations, Nonlinear conductivity, Inhomogeneous media, Multidomain Legendre-tau spectral method, Leap-frog Crank-Nicolson method, Optimal error estimates

中图分类号:

牛翠霞,马和平. 非线性非一致介质二维Maxwell方程leap-frog Crank-Nicolson多区域Legendre-tau配置谱方法[J]. 数学物理学报, 2023, 43(3): 808-828.

Niu Cuixia,Ma Heping. A Leap-Frog Crank-Nicolson Multidomain Legendre-Tau Collocation Spectral Method for 2D Nonlinear Maxwell's Equations in Inhomogeneous Media[J]. Acta mathematica scientia,Series A, 2023, 43(3): 808-828.

图/表 7

表1

表2

表3

表4

表5

表6

表7

参考文献 41

[1]	Taflove A, Hagness S C. Computational Electrodynamics:the Finite-Difference Time-Domain Method. Boston: Artech House, 2000
[2]	Cohen G C. Higher-Order Numerical Methods for Transient Wave Equations, Scientific Computation. Berlin: Springer-Verlag, 2002
[3]	Bondeson A, Rylander T, Ingelström P. Computational Electromagnetics. New York: Springer, 2005
[4]	Yee K S. Numerical solution of initial boundary value problems involving maxwell's equations in isotropic media. IEEE Transactions on Antennas & Propagation, 1966, 14(3): 302-307
[5]	Slodička M. Nonlinear diffusion in type-II superconductors. J Comput Appl Math, 2008, 215(2): 568-576 doi: 10.1016/j.cam.2006.03.055
[6]	Gruner G, Zawadowski A, Chaikin P. Nonlinear conductivity and noise due to charge-density-wave depinning in nbse3. Physical Review Letters, 1981, 46(7): 511-515 doi: 10.1103/PhysRevLett.46.511
[7]	Yin H M. On a singular limit problem for nonlinear Maxwell's equations. J Differential Equations, 1999, 156(2): 355-375 doi: 10.1006/jdeq.1998.3608
[8]	Jochmann F. Asymptotic behavior of the electromagnetic field for a micromagnetism equation without exchange energy. SIAM J Math Anal, 2005, 37(1): 276-290 doi: 10.1137/S0036141004443324
[9]	Yin H M. Existence and regularity of a weak solution to Maxwell's equations with a thermal effect. Math Methods Appl Sci, 2006, 29(10): 1199-1213 doi: 10.1002/(ISSN)1099-1476
[10]	Ferreira M V, Buriol C. Orthogonal decomposition and asymptotic behavior for nonlinear Maxwell's equations. J Math Anal Appl, 2015, 426(1): 392-405 doi: 10.1016/j.jmaa.2014.12.071
[11]	Durand S, Slodička M. Convergence of the mixed finite element method for Maxwell's equations with nonlinear conductivity. Math Methods Appl Sci, 2012, 35(13): 1489-1504 doi: 10.1002/mma.v35.13
[12]	Yao C, Lin Y, Wang C, Kou Y. A third order linearized BDF scheme for Maxwell's equations with nonlinear conductivity using finite element method. Int J Numer Anal Model, 2017, 14(4/5): 511-531
[13]	Bokil V A, Cheng Y, Jiang Y, et al. High spatial order energy stable FDTD methods for Maxwell's equations in nonlinear optical media in one dimension. J Sci Comput, 2018, 77(1): 330-371 doi: 10.1007/s10915-018-0716-8
[14]	Huang Y, Li J, He B. A time-domain finite element scheme and its analysis for nonlinear Maxwell's equations in Kerr media. J Comput Phys, 2021, 435: 110259 doi: 10.1016/j.jcp.2021.110259
[15]	Hesthaven J. High-order accurate methods in time-domain computational electromagnetics: A review. Advances in Imaging and Electron Physics, 2003, 127: 59-123
[16]	Yefet A, Petropoulos P G. A staggered fourth-order accurate explicit finite differences scheme for the time-domain Maxwell's equations. J Comput Phys, 2001, 168(2): 286-315 doi: 10.1006/jcph.2001.6691
[17]	Xie Z, Chan C H, Zhang B. An explicit fourth-order staggered finite-difference time-domain method for Maxwell's equations. J Comput Appl Math, 2002, 147(1): 75-98 doi: 10.1016/S0377-0427(02)00394-1
[18]	Zhao S, Wei G W. High-order FDTD methods via derivative matching for Maxwell's equations with material interfaces. J Comput Phys, 2004, 200(1): 60-103 doi: 10.1016/j.jcp.2004.03.008
[19]	Chen Z, Du Q, Zou J. Finite element methods with matching and nonmatching meshes for Maxwell equations with discontinuous coefficients. SIAM J Numer Anal, 2000, 37(5): 1542-1570 doi: 10.1137/S0036142998349977
[20]	Cai W, Deng S. An upwinding embedded boundary method for Maxwell's equations in media with material interfaces: 2D case. J Comput Phys, 2003, 190(1): 159-183 doi: 10.1016/S0021-9991(03)00269-9
[21]	Deng S. On the immersed interface method for solving time-domain Maxwell's equations in materials with curved dielectric interfaces. Comput Phys Comm, 2008, 179(11): 791-800 doi: 10.1016/j.cpc.2008.07.001
[22]	Nguyen D D, Zhao S. Time-domain matched interface and boundary (MIB) modeling of Debye dispersive media with curved interfaces. J Comput Phys, 2014, 278: 298-325 doi: 10.1016/j.jcp.2014.08.038
[23]	Zhang Y, Nguyen D D, Du K, et al. Time-domain numerical solutions of Maxwell interface problems with discontinuous electromagnetic waves. Adv Appl Math Mech, 2016, 8(3): 353-385 doi: 10.4208/aamm.2014.m811
[24]	Li J, Shi C, Shu C W. Optimal non-dissipative discontinuous Galerkin methods for Maxwell's equations in Drude metamaterials. Comput Math Appl, 2017, 73(8): 1760-1780 doi: 10.1016/j.camwa.2017.02.018
[25]	Yan S, Jin J M. A continuity-preserving and divergence-cleaning algorithm based on purely and damped hyperbolic Maxwell equations in inhomogeneous media. J Comput Phys, 2017, 334: 392-418 doi: 10.1016/j.jcp.2017.01.012
[26]	Camargo L, López-Rodríguez B, Osorio M, Solano M. An HDG method for Maxwell's equations in heterogeneous media. Comput Methods Appl Mech Engrg, 2020, 368: 113178 doi: 10.1016/j.cma.2020.113178
[27]	Bernardi C, Maday Y. Spectral methods. Handbook of Numerical Analysis, 1997, 5: 209-485
[28]	Guo B Y. Spectral Methods and Their Applications. New Jersey: World Scientific Publishing Co, 1998
[29]	Guo B Y, Shen J. Laguerre-Galerkin method for nonlinear partial differential equations on a semi-infinite interval. Numer Math, 2000, 86(4): 635-654 doi: 10.1007/PL00005413
[30]	Guo B Y. Some Developments in Spectral Methods for Nonlinear Partial Differential Equations in Unbounded Domains//Gu C H, Hu H S, Li T. Differential Geometry and Related Topics. Singapore: World Science, 2002: 68-90
[31]	Shen J, Tang T. Spectral and High-order Methods with Applications. Mathematics Monograph Series. Beijing: Science Press, 2006
[32]	Ma H. Chebyshev-Legendre spectral viscosity method for nonlinear conservation laws. SIAM J Numer Anal, 1998, 35(3): 869-892 doi: 10.1137/S0036142995293900
[33]	Ma H. Chebyshev-Legendre super spectral viscosity method for nonlinear conservation laws. SIAM J Numer Anal, 1998, 35(3): 893-908 doi: 10.1137/S0036142995293912
[34]	Ma H, Sun W. Optimal error estimates of the Legendre-Petrov-Galerkin method for the Korteweg-de Vries equation. SIAM J Numer Anal, 2001, 39(4): 1380-1394 doi: 10.1137/S0036142900378327
[35]	Ma H, Qin Y, Ou Q. Multidomain Legendre-Galerkin Chebyshev-collocation method for one-dimensional evolution equations with discontinuity. Appl Numer Math, 2017, 111: 246-259 doi: 10.1016/j.apnum.2016.09.010
[36]	Chan T F, Kerkhoven T. Fourier methods with extended stability intervals for the Korteweg-de Vries equation. SIAM J Numer Anal, 1985, 22(3): 441-454 doi: 10.1137/0722026
[37]	Wu H, Ma H, Li H. Chebyshev-Legendre spectral method for solving the two-dimensional vorticity equations with homogeneous Dirichlet conditions. Numer Methods Partial Differential Equations, 2009, 25(3): 740-755 doi: 10.1002/num.v25:3
[38]	Shen J, Tang T, Wang L L. Spectral Methods: Algorithms, Analysis and Applications. Heidelberg: Springer, 2011
[39]	Canuto C, Hussaini M Y, Quarteroni A, Zang T A. Spectral Methods: Fundamentals in Single Domains. Berlin: Springer-Verlag, 2006
[40]	Canuto C, Hussaini M Y, Quarteroni A, Zang T A. Spectral Methods: Evolution to Complex Geometries and Applications to Fluid Dynamics. Berlin: Springer, 2007
[41]	Niu C, Ma H, Liang D. Energy-conserved splitting multidomain Legendre-Tau spectral method for two dimensional Maxwell's equations. J Sci Comput, 2022, 90(2): 77 doi: 10.1007/s10915-021-01744-0