非定常 Stokes/Darcy 模型一种新的time filter 算法的分析

数学物理学报 ›› 2023, Vol. 43 ›› Issue (3): 829-854.

非定常 Stokes/Darcy 模型一种新的time filter 算法的分析

王阳¹(),李剑¹(),李祎²(),秦毅^1,^*()

¹陕西科技大学数学与数据科学学院西安710021
²西北大学数学学院西安710127

收稿日期:2022-07-25 修回日期:2022-10-10 出版日期:2023-06-26 发布日期:2023-06-01
通讯作者: 秦毅 E-mail:210911017@sust.edu.cn;jianli@sust.edu.cn;liyizz@nwu.edu.cn;4545@sust.edu.cn
作者简介:王阳,E-mail: 210911017@sust.edu.cn;|李剑,E-mail: jianli@sust.edu.cn;|李祎,E-mail: liyizz@nwu.edu.cn
基金资助:
国家自然科学基金(12001347);国家自然科学基金(11771259);国家自然科学基金(12101494);陕西省教育厅科研项目(21JK0935);陕西省教育厅创新团队项目(21JP013);陕西省教育厅创新团队项目(21JP019);陕西省自然科学基金(2021-426);陕西省人工智能联合实验室(2022JC-SYS-05);陕西省自然科学基础研究计划重点项目(2023-JC-ZD-02)

Analysis of a New Time Filter Algorithm for the Unsteady Stokes/Darcy Model

Wang Yang¹(),Li Jian¹(),Li Yi²(),Qin Yi^1,^*()

¹School of Mathematics and Data Science, Shaanxi University of Science and Technology, Xi'an 710021
²School of Mathematics, Northwest University, Xi'an 710127

Received:2022-07-25 Revised:2022-10-10 Online:2023-06-26 Published:2023-06-01
Contact: Yi Qin E-mail:210911017@sust.edu.cn;jianli@sust.edu.cn;liyizz@nwu.edu.cn;4545@sust.edu.cn
Supported by:
NSFC(12001347);NSFC(11771259);NSFC(12101494);Scientific Research Program Funded by Shaanxi Provincial Education Department(21JK0935);Innovative Team Project of Shaanxi Provincial Department of Education(21JP013);Innovative Team Project of Shaanxi Provincial Department of Education(21JP019);Natural Science Foundation of Shaanxi Province(2021-426);Shaanxi Provincial Joint Laboratory of Artificial Intelligence(2022JC-SYS-05);Shaanxi Province Natural Science Basic Research Program Key Project(2023-JC-ZD-02)

摘要/Abstract

摘要：

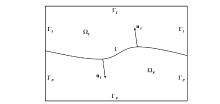
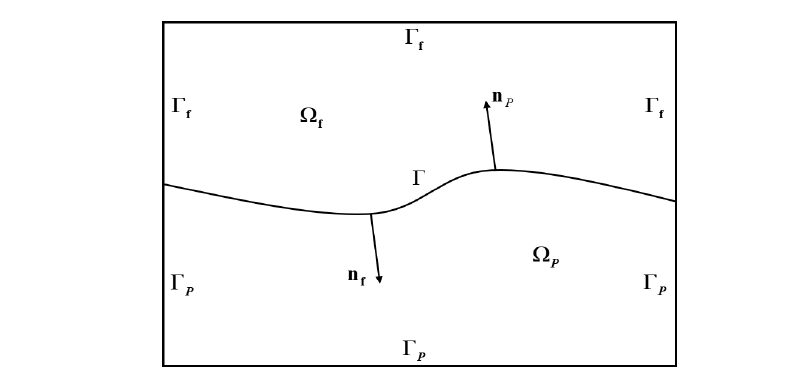
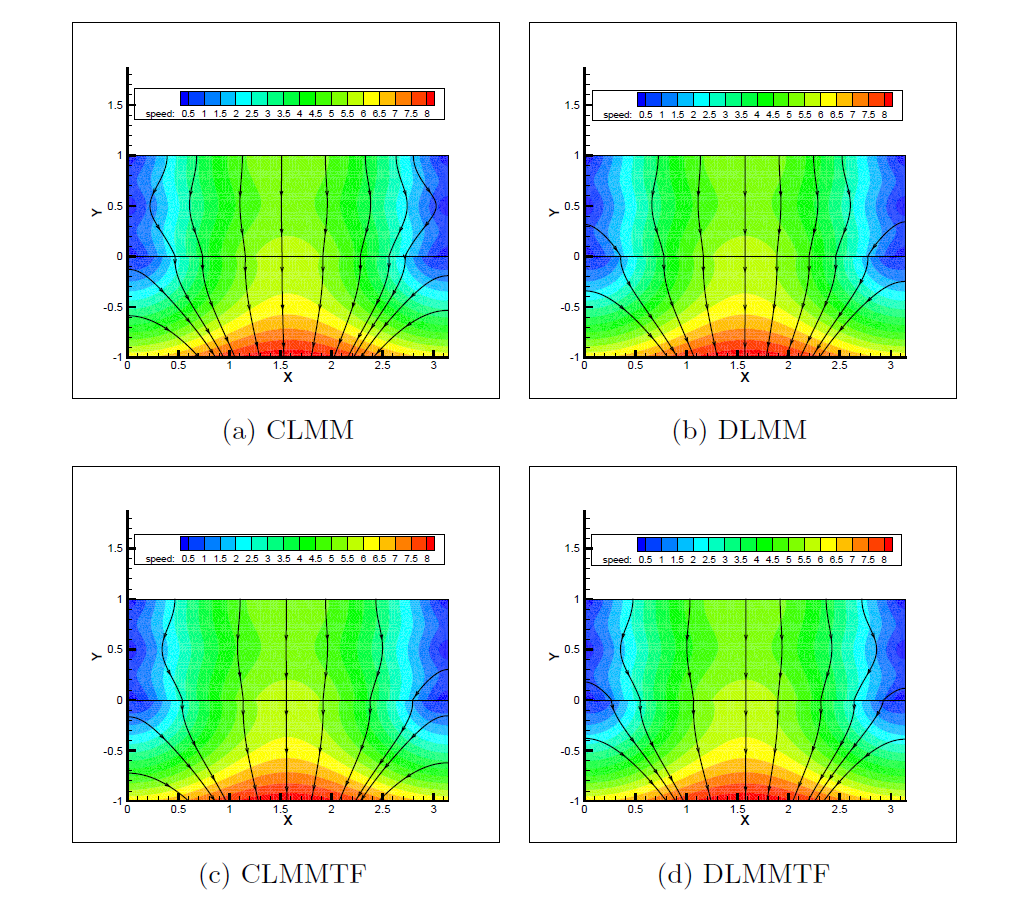
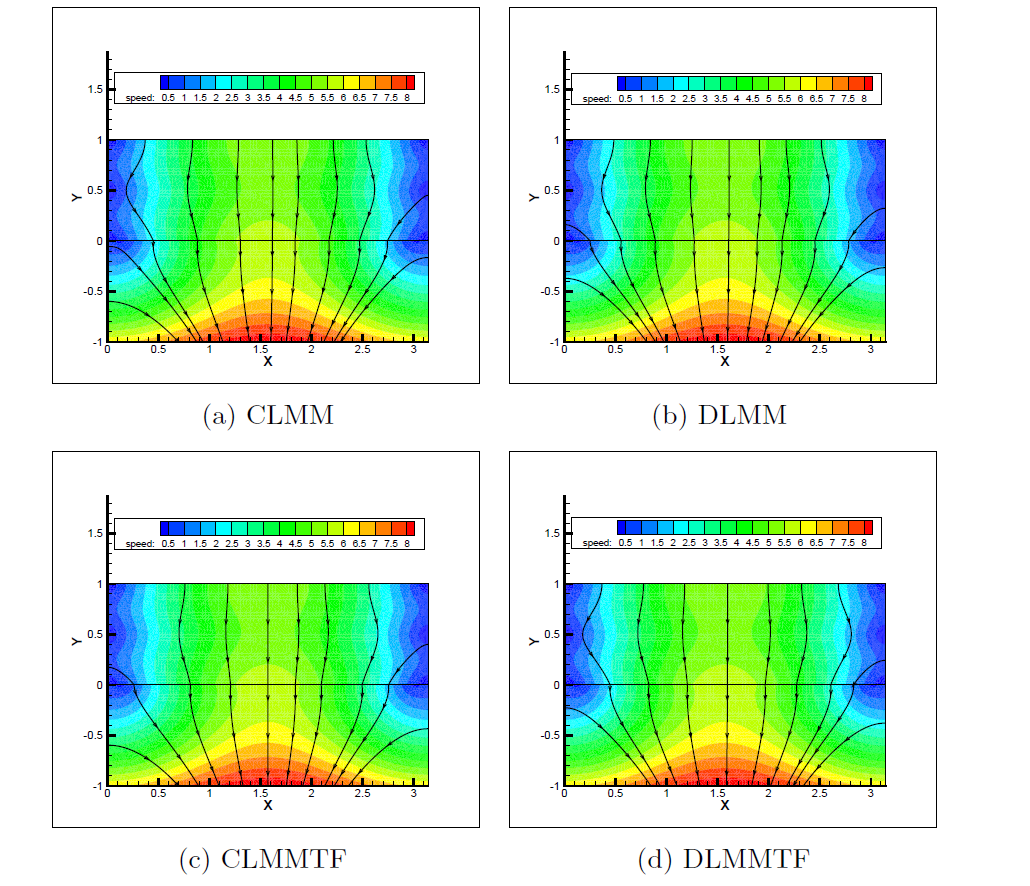
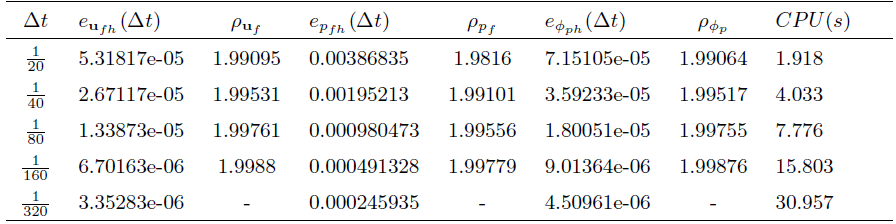
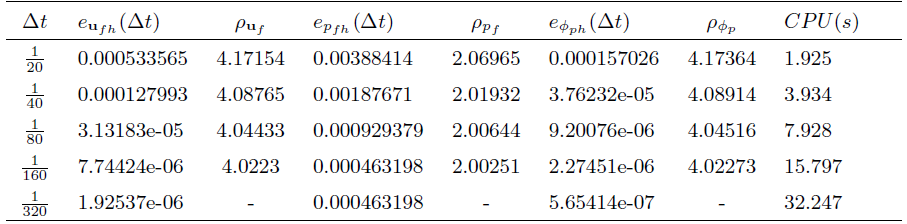
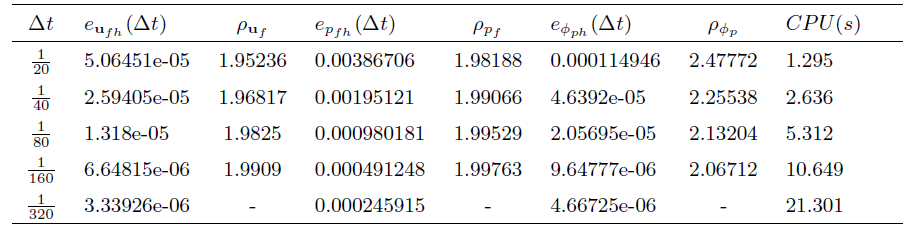
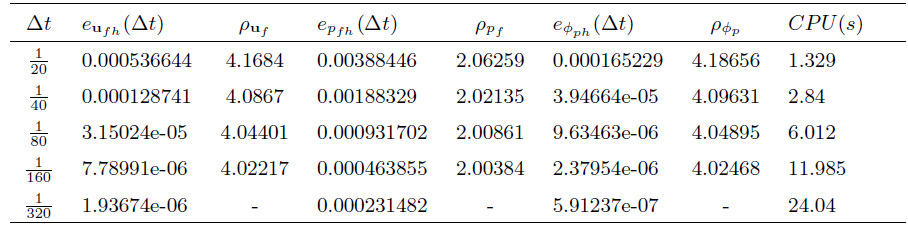
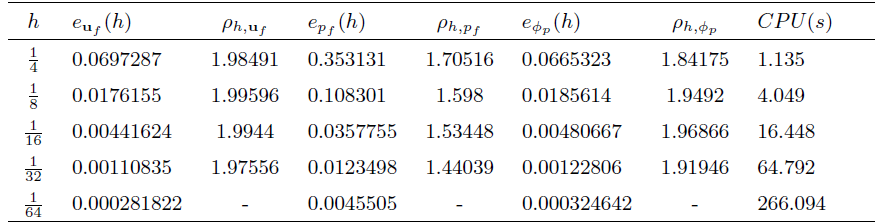
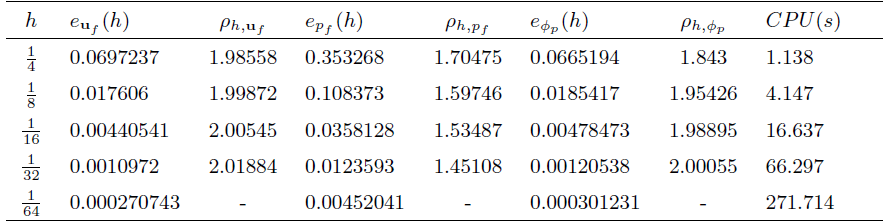
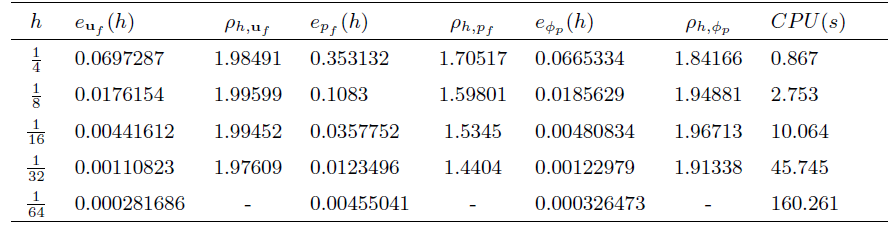
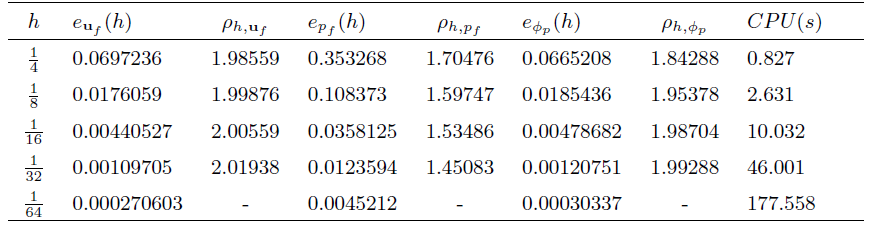
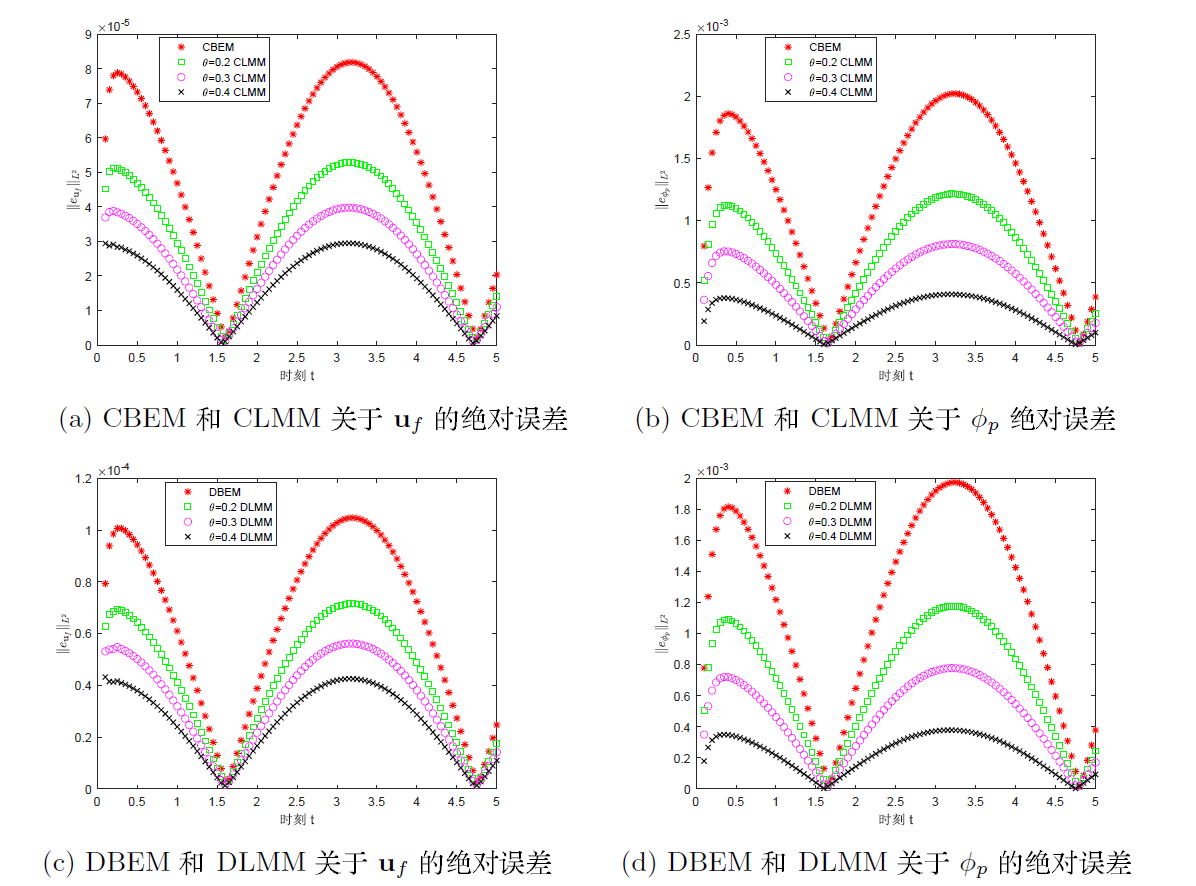
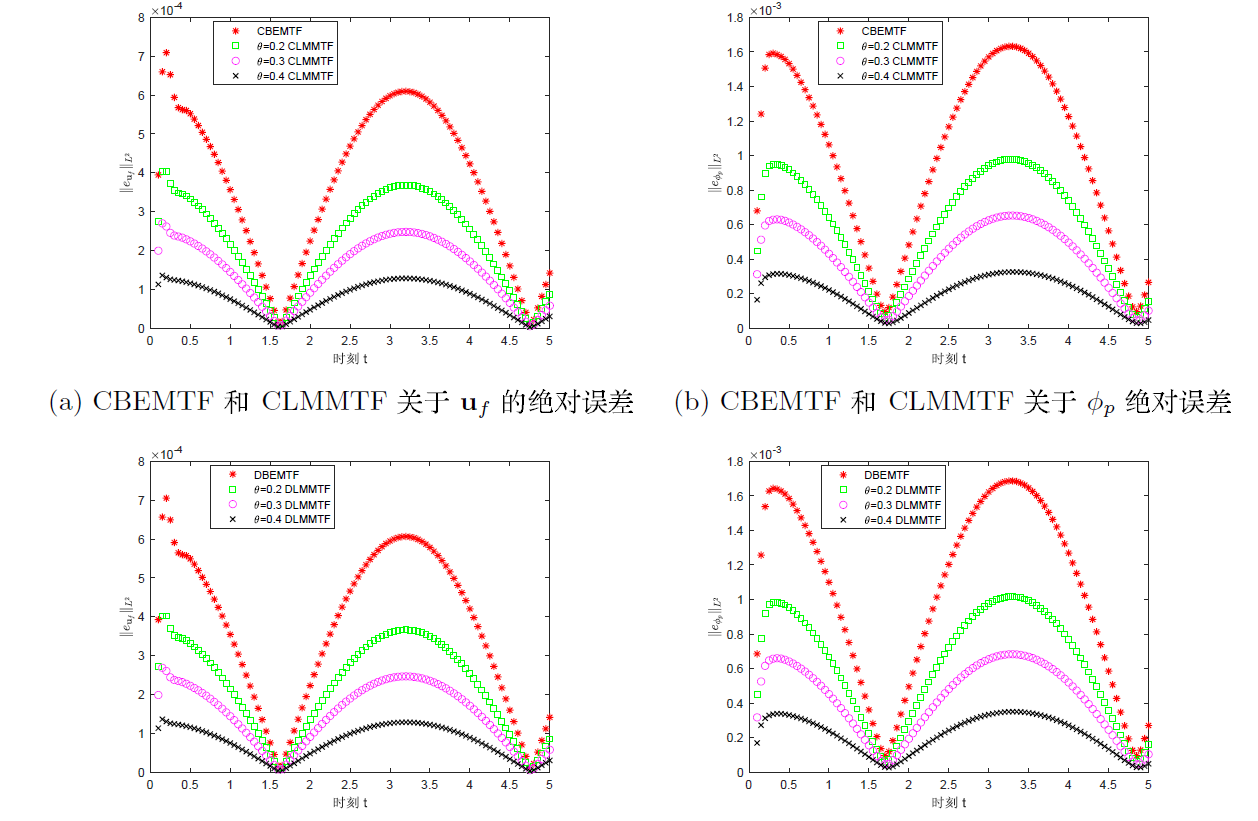
首先, 在非定常 Stokes/Darcy 模型的线性多步法的一阶 $\theta$ -格式的基础上, 该文结合time filter 算法在几乎不增加计算量的情况下有效地将线性多步法的收敛阶由一阶提高到二阶, 从而提出一种新的高效数值算法. 其次, 该文分别对耦合和解耦的线性多步法加 time filter 算法的稳定性和误差估计进行了理论分析. 最后, 数值实验进一步展示了耦合和解耦算法的有效性, 收敛性和高效性.

关键词: Stokes/Darcy 模型, 线性多步法, Time filter 算法, 二阶收敛

Abstract:

Firstly, based on the first-order $\theta$-scheme of the Linear Multistep method for the unsteady Stokes/Darcy model, this paper combines the time filter algorithm to effectively improve the convergence order of the Linear Multistep method from first order to second order almost without increasing the amount of calculation, and a new efficient numerical algorithm is proposed. Secondly, the stability and error estimation of the coupled and decoupled Linear Multistep method plus time filter algorithm are analyzed theoretically. Finally, numerical experiments further demonstrate the effectiveness, convergence and efficiency of the coupled and decoupled algorithms.

Key words: Stokes/Darcy model, Linear Multistep method, Time filter algorithm, Second order convergence

中图分类号:

O241.82

王阳,李剑,李祎,秦毅. 非定常 Stokes/Darcy 模型一种新的time filter 算法的分析[J]. 数学物理学报, 2023, 43(3): 829-854.

Wang Yang,Li Jian,Li Yi,Qin Yi. Analysis of a New Time Filter Algorithm for the Unsteady Stokes/Darcy Model[J]. Acta mathematica scientia,Series A, 2023, 43(3): 829-854.

图/表 14

图 1

图 2

图 3

图 4

表1

表2

表3

表4

表5

表6

表7

表8

图 5

图 6

参考文献 39

[1]	Ervin V J. Approximation of coupled Stokes-Darcy flow in an axisymmetric domain. Computer Methods in Applied Mechanics and Engineering, 2013, 258: 96-108 doi: 10.1016/j.cma.2013.02.004
[2]	Hou Y R, Qin Y. On the solution of coupled Stokes/Darcy model with Beavers-Joseph interface condition. Computers & Mathematics with Applications, 2019, 77(1): 50-65 doi: 10.1016/j.camwa.2018.09.011
[3]	Cao Y Z, Gunzburger M, Hu X L, et al. Finite element approximations for Stokes-Darcy flow with Beavers-Joseph interface conditions. SIAM Journal on Numerical Analysis, 2010, 47(6): 4239-4256 doi: 10.1137/080731542
[4]	Kanschat G, Riviére B. A strongly conservative finite element method for the coupling of Stokes and Darcy flow. Journal of Computational Physics, 2010, 229(17): 5933-5943 doi: 10.1016/j.jcp.2010.04.021
[5]	Glowinshi R. Finite element methods for incompressible viscous flow. Handbook of Numerical Analysis, 2003, 9: 3-1176
[6]	Lipnikov K, Vassilev D, Yotov I. Discontinuous Galerkin and mimetic finite difference methods for coupled Stokes-Darcy flows on polygonal and polyhedral grids. Numerische Mathematik, 2014, 126(2): 321-360 doi: 10.1007/s00211-013-0563-3
[7]	Chen W B, Wang F, Wang Y Q. Weak Galerkin method for the coupled Darcy-Stokes flow. IMA Journal of Numerical Analysis, 2016, 36(2): 897-921 doi: 10.1093/imanum/drv012
[8]	Girault V, Riviére B. DG approximation of coupled Navier-Stokes and Darcy equations by Beaver-Joseph-Saffman interface condition. SIAM Journal on Numerical Analysis, 2009, 47(3): 2052-2089 doi: 10.1137/070686081
[9]	Markus S, Houstis E N, Catlin A C, et al. An agent-based netcentric framework for multidisciplinary problem solving environments (MPSE). Inter J Comput Engineering Sci, 2000, 1(1): 33-60 doi: 10.33545/26633582
[10]	Mu M. Solving composite problems with interface relaxation. SIAM Journal on Scientific Computing, 1999, 20(4): 1394-1416 doi: 10.1137/S1064827597321180
[11]	Gatica G N, Oyarzúa R, Sayas F J. A residual-based a posteriori error estimator for a fully-mixed formulation of the Stokes-Darcy coupled problem. Computer Methods in Applied Mechanics and Engineering, 2011, 200(21/22): 1877-1891 doi: 10.1016/j.cma.2011.02.009
[12]	Layton W J, Schieweck F, Yotov I. Coupling fluid flow with porous media flow. SIAM Journal on Numerical Analysis, 2002, 40(6): 2195-2218 doi: 10.1137/S0036142901392766
[13]	He X M, Li J, Lin Y P, et al. A domain decomposition method for the steady-state Navier-Stokes-Darcy model with Beavers-Joseph interface condition. SIAM J Sci Comput, 2015, 37(5): S264-S290 doi: 10.1137/140965776
[14]	Cao Y Z, Gunzburger M, He X M, et al. Robin-Robin domain decomposition methods for the steady Stokes-Darcy model with Beaver-Joseph interface condition. Numer Math, 2011, 117(4): 601-629 doi: 10.1007/s00211-011-0361-8
[15]	Chen W B, Gunzburger M, Hua F, et al. A parallel Robin-Robin domain decomposition method for the Stokes-Darcy system. SIAM Journal on Numerical Analysis, 2011, 49(3): 1064-1084 doi: 10.1137/080740556
[16]	Hou Y R. Optimal error estimates of a decoupled scheme based on two-grid finite element for mixed Stokes-Darcy model. Applied Mathematics Letters, 2016, 57: 90-96 doi: 10.1016/j.aml.2016.01.007
[17]	Qin Y, Hou Y R. Optimal error estimates of a decoupled scheme based on two-grid finite element for mixed Navier-Stokes/Darcy model. Acta Mathematica Scientia, 2018, 38(4): 1361-1369 doi: 10.1016/S0252-9602(18)30819-1
[18]	Zuo L Y, Du G Z. A multi-grid technique for coupling fluid flow with porous media flow. Computers & Mathematics with Applications, 2018, 75(11): 4012-4021 doi: 10.1016/j.camwa.2018.03.010
[19]	Zuo L Y, Hou Y R. A two-grid decoupling method for the mixed Stokes-Darcy model. Journal of Computational and Applied Mathematics, 2015, 275: 139-147 doi: 10.1016/j.cam.2014.08.008
[20]	Cao Y Z, Gunzburger M, Hua F, et al. Coupled Stokes-Darcy model with Beavers-Joseph interface boundary condition. Communications in Mathematical Sciences, 2010, 8(1): 1-25 doi: 10.4310/CMS.2010.v8.n1.a2
[21]	Cao Y Z, Gunzburger M, He X M, et al. Parallel, non-iterative, multi-physics domain decomposition methods for time-dependent Stokes-Darcy systems. Math Comput, 2014, 83(288): 1617-1644 doi: 10.1090/mcom/2014-83-288
[22]	Cao Y Z, Gunzburger M, Hu X L, et al. Finite element approximation for time-dependent Stokes-Darcy flow with Beavers-Joseph interface boundary condition. SIAM J Numer Anal, 2010, 47(6): 4239-4256 doi: 10.1137/080731542
[23]	Li Y, Hou Y R. A second-order partitioned method with different subdomain time steps for the evolutionary Stokes-Darcy system. Mathematical Methods in the Applied Sciences, 2018, 41(5): 2178-2208 doi: 10.1002/mma.v41.5
[24]	Layton W J, Tran H, Xiong X. Long time stability of four methods for splitting the evolutionary Stokes-Darcy problem into Stokes and Darcy subproblems. Journal of Computational and Applied Mathematics, 2012, 236(13): 3198-3217 doi: 10.1016/j.cam.2012.02.019
[25]	Layton W J, Tran H, Trenchea C. Analysis of long time stability and errors of two partitioned methods for uncoupling evolutionary groundwater-surface water flows. SIAM J Numer Anal, 2013, 51(1): 248-272 doi: 10.1137/110834494
[26]	Shan L, Zheng H B, Layton W J. A decoupling method with different subdomain time steps for the nonstationary Stokes-Darcy model. Numer Meth Partial Differ Equ, 2013, 29(2): 549-583 doi: 10.1002/num.v29.2
[27]	Guzel A, Layton W J. Time filters increase accuracy of the fully implicit method. BIT Numerical Mathematic, 2018, 58(2): 301-315
[28]	Li Y, Hou Y R, Layton W J. Adaptive partitioned methods for the time-accurate approximation of the evolutionary Stokes-Darcy system. Computer Meth Appl Mech Engineering, 2020, 364: 112923 doi: 10.1016/j.cma.2020.112923
[29]	Qin Y, Hou Y R. The time filter for the non-stationary coupled Stokes/Darcy model. Applied Numerical Mathematics, 2019, 146: 260-275 doi: 10.1016/j.apnum.2019.07.015
[30]	Girault V, Raviart P A. Finite Element Approximation of the Navier-Stokes Equations. Berlin: Springer-Verlag, 1981
[31]	Mu M, Zhu X H. Decoupled schemes for a non-stationary mixed Stokes-Darcy model. Mathematics of Computation, 2010, 79(270): 707-731 doi: 10.1090/S0025-5718-09-02302-3
[32]	Li J, Lin X L, Chen Z X. Finite Volume Methods for the Incompressible Navier-Stokes Equations. Berlin: Springer-Verlag, 2021
[33]	李剑. 不可压缩 Navier-Stokes 方程数值方法. 北京: 科学出版社, 2019
	Li J. Numerical Methods for the Incompressible Navier-Stokes Equations. Beijing: Science Press, 2019
[34]	李剑, 白云霄, 赵昕. 数学物理方程的现代数值方法. 北京: 科学出版社, 2022
	Li J, Bai Y X, Zhao X. Modern Numerical Methods for Mathematical Physics Equations. Beijing: Science Press, 2022
[35]	Qin Y, Wang Y S, Li J. A variable time step time filter algorithm for the geothermal system. SIAM Journal on Numerical Analysis, 2022, 60(5): 2781-2806 doi: 10.1137/21M1464828
[36]	Cao L L, He Y N, Li J. A parallel Robin-Robin domain decomposition method based on modified characteristic FEMs for the time-dependent dual-porosity-Navier-Stokes model with the Beavers-Joseph interface condition. Journal of Scientific Computing, 2022, 90(1): 1-34 doi: 10.1007/s10915-021-01681-y
[37]	Jiang N, Qiu C. An efficient ensemble algorithm for numerical approximation of stochastic Stokes-Darcy equations. Computer Methods in Applied Mechanics and Engineering, 2019, 343: 249-275 doi: 10.1016/j.cma.2018.08.020
[38]	Li Y, Hou Y R, Rong Y. A second-order artificial compression method for the evolutionary Stokes-Darcy system. Numerical Algorithms, 2020, 84(3): 1019-1048 doi: 10.1007/s11075-019-00791-x
[39]	Li Y, Hou Y R. Error estimates of second-order decoupled scheme for the evolutionary Stokes-Darcy system. Applied Numerical Mathematics, 2020, 154: 129-148