具有恐惧效应和 Holling III 功能性反应的随机捕食-食饵系统

数学物理学报 ›› 2024, Vol. 44 ›› Issue (5): 1380-1391.

具有恐惧效应和 Holling III 功能性反应的随机捕食-食饵系统

汪秋分¹(),张树文^1,^2,^*()

¹厦门工学院数据科学与计算机学院福建厦门 361021
²集美大学理学院福建厦门 361021

收稿日期:2023-07-24 修回日期:2024-03-06 出版日期:2024-10-26 发布日期:2024-10-16
通讯作者: *张树文, E-mail: zhangsw_123@126.com
作者简介:汪秋分, E-mail: 356672150@qq.com
基金资助:
厦门工学院基于大数据的模糊系统理论及其应用科研创新团队(KYTD202005);厦门工学院校级课程建设项目“数学建模”(AL2019002)

Stochastic Predator-Prey System with Fear Effect and Holling-Type III Functional Response

Wang Qiufen¹(),Zhang Shuwen^1,^2,^*()

¹School of data science and computer science, Xiamen Institute of Technology, Fujian Xiamen 361021
²School of Science, Jimei University, Fujian Xiamen 361021

Received:2023-07-24 Revised:2024-03-06 Online:2024-10-26 Published:2024-10-16
Supported by:
Fuzzy System Theory and its Application Research and Innovation Team Based on Big Data, Xiamen Institute of Technology(KYTD202005);Xiamen Institute of Technology School-Level Curriculum Construction Project "Mathematical Modeling"(AL2019002)

摘要/Abstract

摘要：

该文研究了一类具有恐惧效应和 Holling III 功能反应的随机捕食-食饵系统. 首先, 证明了在任意给定正初始值下, 系统全局正解的存在唯一性与均值有界性及随机最终有界性; 其次, 研究了食饵种群和捕食者种群灭绝和平均持续生存的充分条件, 并构造 Lyapunov 函数证明了系统存在平稳分布及遍历性; 最后, 通过数值模拟验证了文章的结果.

关键词: 恐惧效应, 捕食-食饵系统, Holling III 功能反应, 灭绝性与持久性, 平稳分布

Abstract:

A stochastic predator-prey system with fear effect and Holling-type III functional response is studied. Firstly, the existence and uniqueness of global positive solutions, mean boundedness and stochastic ultimate boundedness of the system for any given positive initial value are proved. Secondly, the sufficient conditions for extinction and persistence in mean of the prey and predator populations are obtained, and Lyapunov function is constructed to prove the existence of stationary distribution and ergodicity. Finally, the results are verified by numerical simulations.

Key words: Fear effect, Predator-prey system, Holling-type III functional response, Extinction and persistence, Stationary distribution

中图分类号:

O211.63

汪秋分, 张树文. 具有恐惧效应和 Holling III 功能性反应的随机捕食-食饵系统[J]. 数学物理学报, 2024, 44(5): 1380-1391.

Wang Qiufen, Zhang Shuwen. Stochastic Predator-Prey System with Fear Effect and Holling-Type III Functional Response[J]. Acta mathematica scientia,Series A, 2024, 44(5): 1380-1391.

图/表 5

图1

图2

图3

图4

图5

参考文献 17

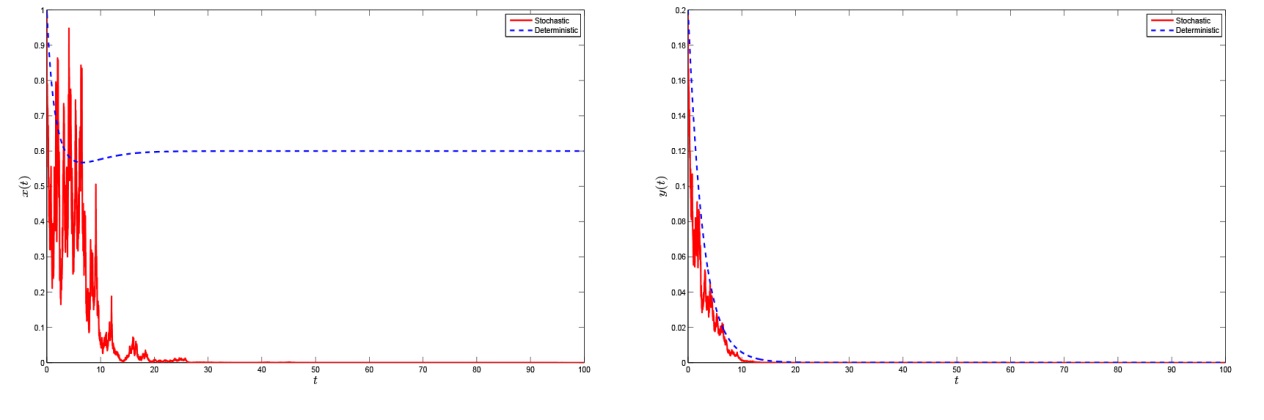
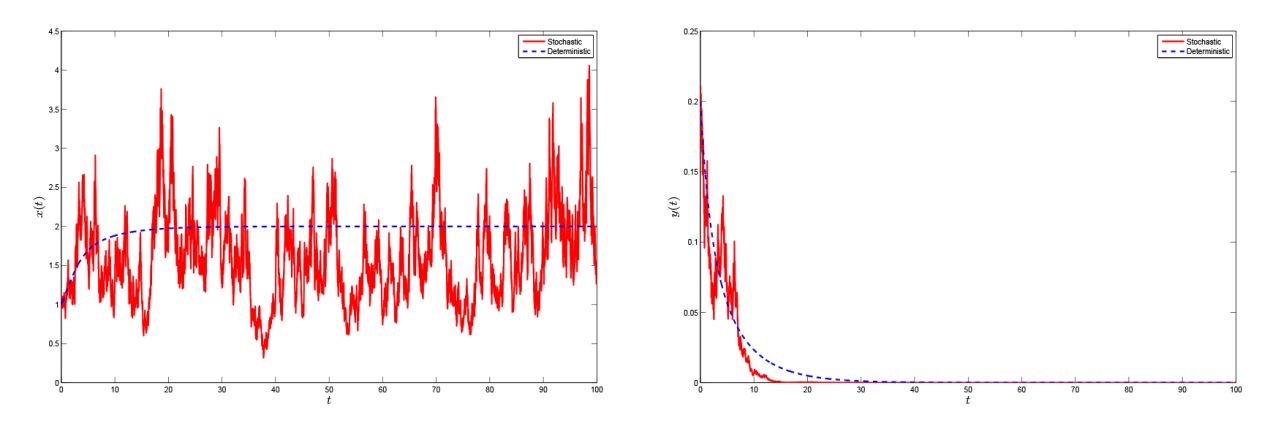
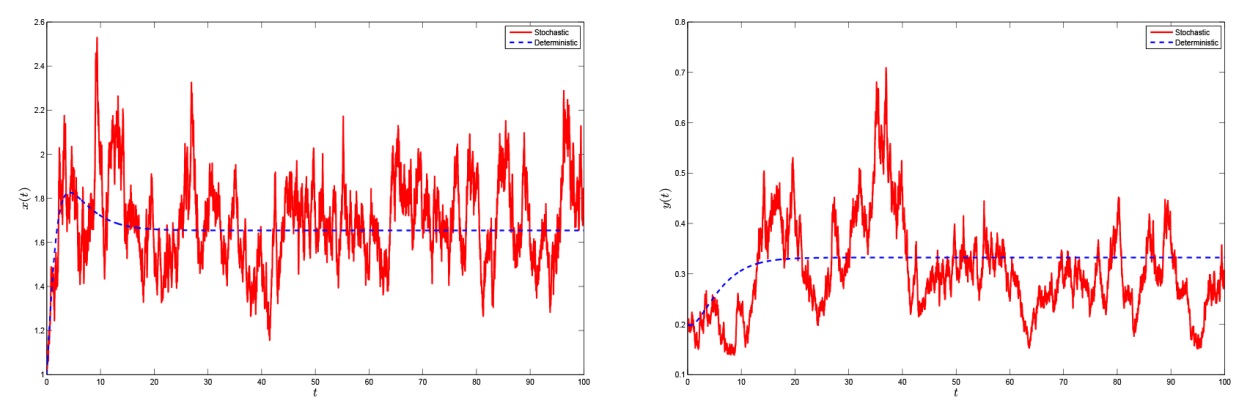
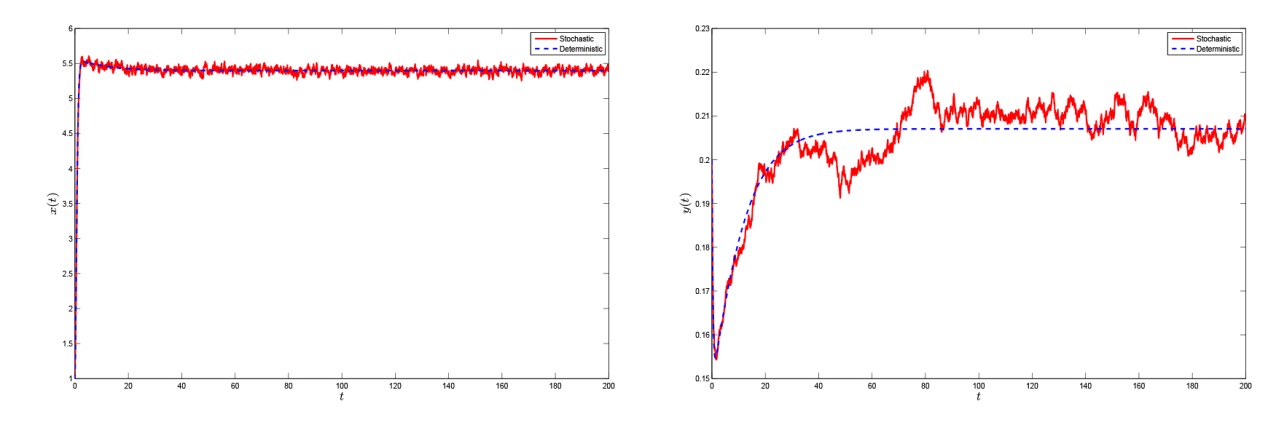
[1]	Qi H K, Meng X Z. Dynamics of a stochastic predator-prey model with fear effect and hunting cooperation. J Appl Math Comput, 2023, 69(4): 2077-2103 doi: 10.1007/s12190-022-01746-7
[2]	Gokila C, Sambath M, Balachandran K, Ma Y K. Stationary distribution and global stability of stochastic predator-prey model with disease in prey population. J Biol Dyn, 2023, 17(1): 1-30
[3]	Gao J, Zhang J, Lian W. Dynamical complexity of a predator-prey model with a prey refuge and Allee effect. Turk J Math, 2023, 47(7): 2061-2085
[4]	Liu M, Mandal P S. Dynamical behavior of a one-prey two-predator model with random perturbations. Commun Nonl Sci Numer Simulat, 2015, 28(1/3): 123-137
[5]	Kumar C P, Reddy K S, Srinivas M. Dynamics of prey predator with Holling interactions and stochastic influences. Alex Eng J, 2018, 57(2): 1079-1086
[6]	Holling C S. The functional response of predators to prey density and its role in mimicry and population regulation. Mem Ent Soc Can, 1965, 97(45): 1-60
[7]	陈兰荪, 宋新宇, 陆征一. 数学生态学模型与研究方法. 成都: 四川科学技术出版社, 2003
	Chen L S, Song X Y, Lu Z Y. Mathematical Ecology Models and Research Methods. Chengdu: Sichuan Sci Technol Press, 2003
[8]	Beroual N, Bendjeddou A. On a predator-prey system with Holling functional response: $x^p/(a + x^p)$. Natl Acad Sci Lett, 2016, 39(1): 43-46
[9]	Wang X Y, Zanette L, Zou X F. Modelling the fear effect in predator-prey interactions. J Math Biol, 2016, 73(5): 1179-1204 pmid: 27002514
[10]	Mondal S, Maiti A, Samanta G P. Effects of fear and additional food in a delayed predator-prey model. Biophys Rev Lett, 2018, 13(4): 157-177
[11]	Pal S, Majhi S, Mandal S, Pal N. Role of fear in a predator-prey model with Beddington-DeAngelis functional response. Z Naturforsch A, 2019, 74(7): 581-595
[12]	Wang Q F, Zhang S W. Dynamics of a stochastic delay predator-prey model with fear effect and diffusion for prey. J Math Anal Appl, 2024, 537(2): 128267
[13]	蓝桂杰, 付盈洁, 魏春金, 张树文. 具有 Holling III 功能性反应的随机捕食食饵模型的平稳分布和周期解. 数学物理学报, 2018, 38A(5): 984-1000
	Lan G J, Fu Y J, Wei C J, Zhang S W. Stationary distribution and periodic solution for stochastic predator-prey model with Holling-type III functional response. Acta Math Sci, 2018, 38A(5): 984-1000
[14]	张树文. 具有时滞和扩散的随机捕食-食饵系统. 数学物理学报, 2015, 35A(3): 592-603
	Zhang S W. A stochastic predator-prey system with time delay and prey dispersal. Acta Math Sci, 2015, 35A(3): 592-603
[15]	千美华, 崔莹, 李晓月. 随机环境下 Holling III 型捕食-食饵系统动力学行为. 东北师大学报 (自然科学版), 2023, 55(1): 40-44
	Qian M H, Cui Y, Li X Y. Dynamics behavior of the Holling III type predator-prey model in a random environment. J Northeast Norm Univ (Nat Sci Ed), 2023, 55(1): 40-44
[16]	王克. 随机生物数学模型. 北京: 科学出版社, 2010
	Wang K. Random Biological Mathematical Model. Beijing: Science Press, 2010
[17]	Mandal P S, Banerjee M. Stochastic persistence and stationary distribution in a Holling-Tanner type prey-predator model. Physica A, 2012, 391(4): 1216-1233