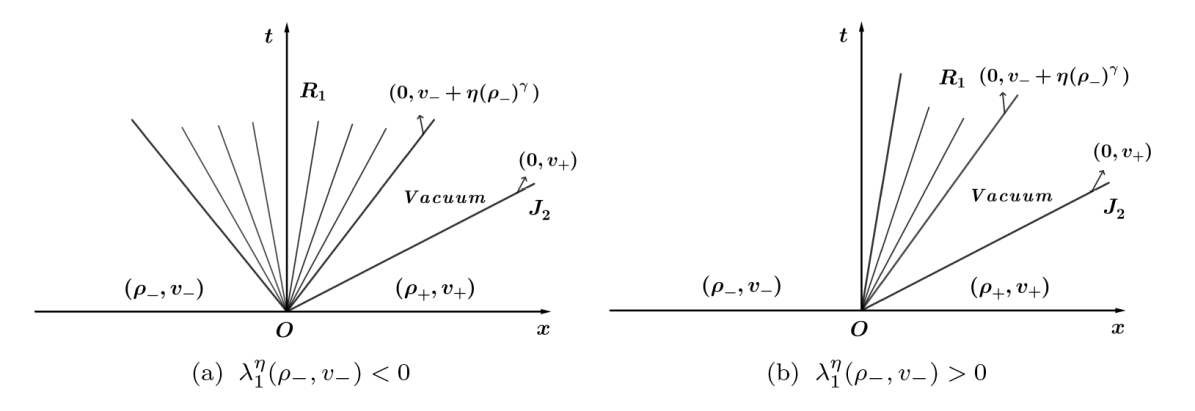
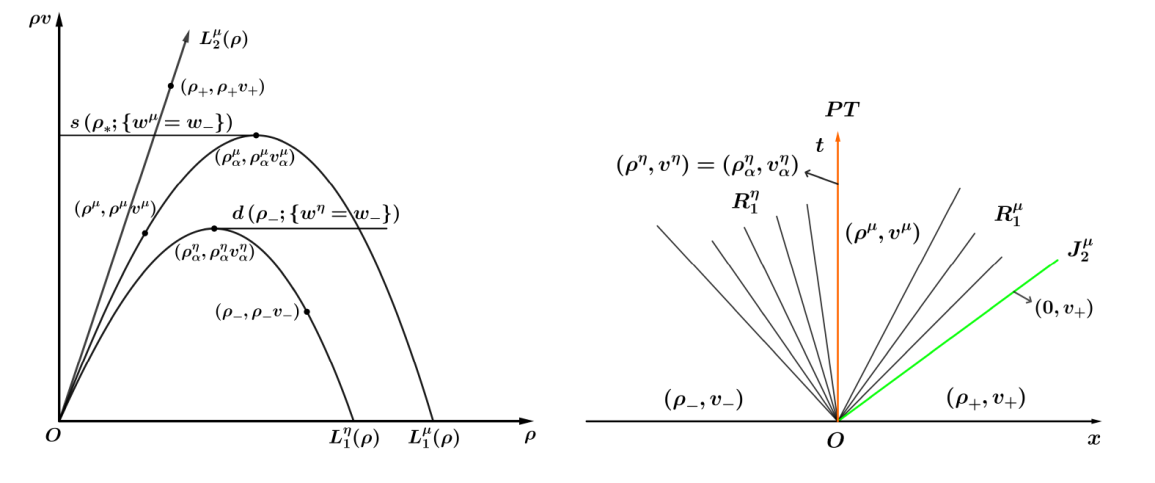
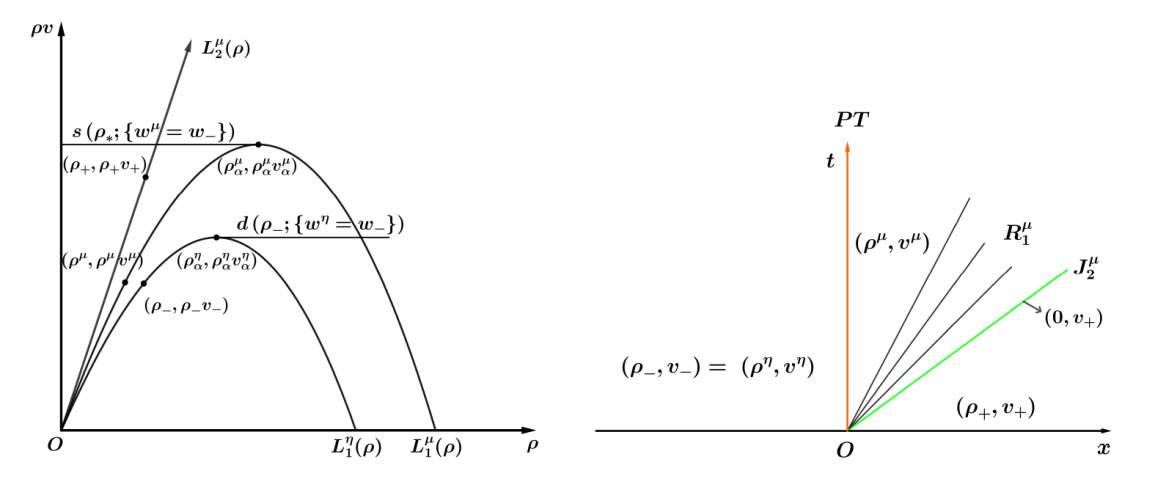
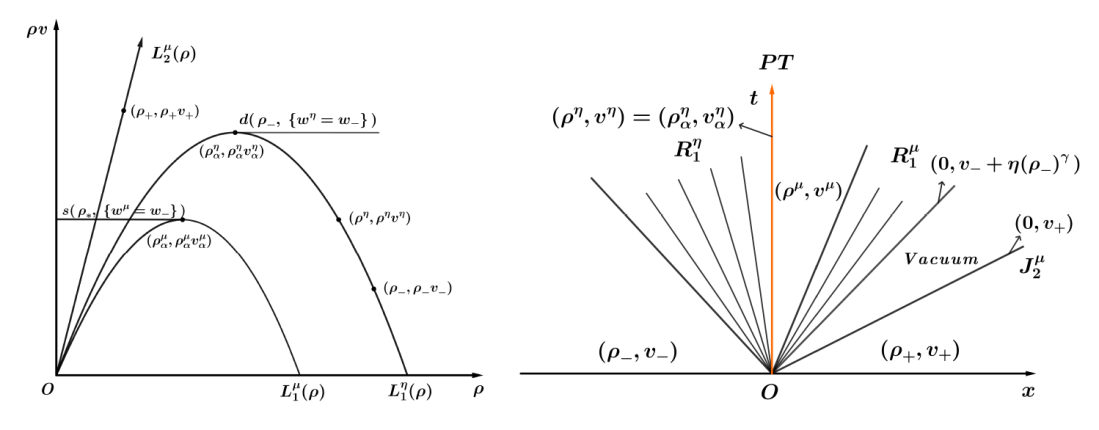
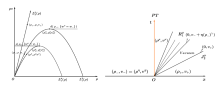
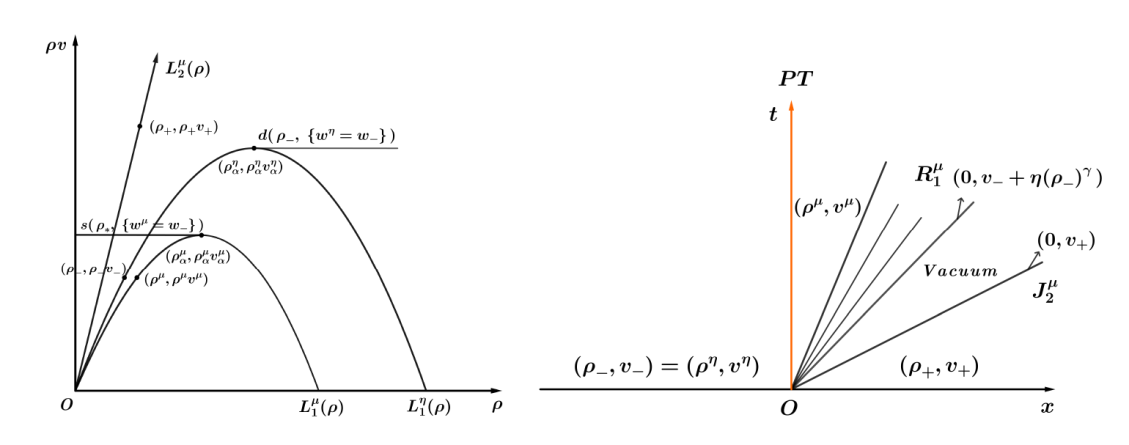
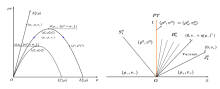
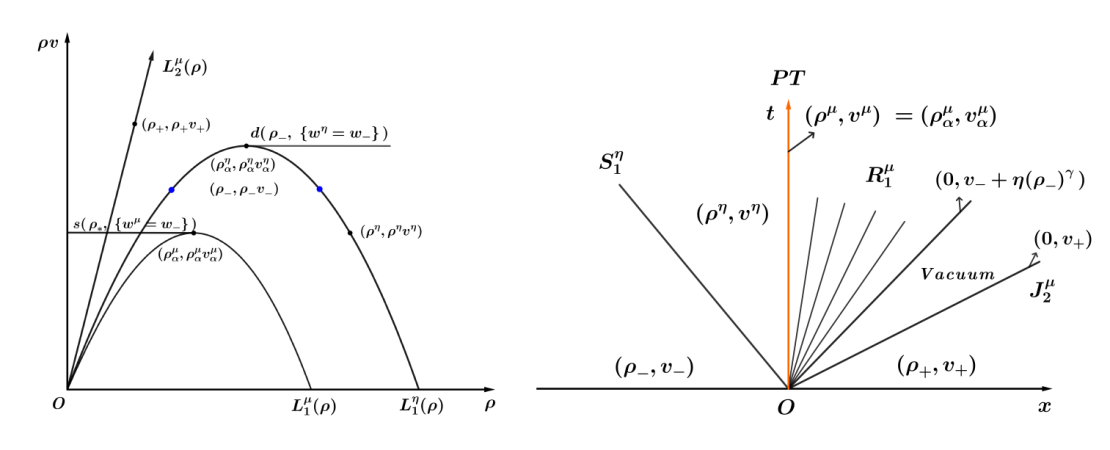
耦合Aw-Rascle-Zhang模型的Riemann解及其稳定性

数学物理学报 ›› 2024, Vol. 44 ›› Issue (4): 885-895.

耦合Aw-Rascle-Zhang模型的Riemann解及其稳定性

潘丽君^*(),吕顺(),翁莎莎()

南京航空航天大学数学学院南京 211106；航空飞行器数学建模与高性能计算重点实验室南京 211106

收稿日期:2023-05-30 修回日期:2024-01-25 出版日期:2024-08-26 发布日期:2024-07-26
通讯作者: *潘丽君, E-mail:98010149@163.com
作者简介:吕顺, E-mail:shun0419@nuaa.edu.cn;|翁莎莎, E-mail:wengshasha@nuaa.edu.cn
基金资助:
中国留学基金(201506835005)

Riemann Solution and Stability of Coupled Aw-Rascle-Zhang Model

Pan Lijun^*(),Lv Shun(),Weng Shasha()

School of Mathematics, Nanjing University of Aeronautics and Astronautics, Nanjing 211106; Key Laboratory of Mathematical Modelling and High Performance Computing of Air Vehicles (NUAA), MIIT, Nanjing 211106

Received:2023-05-30 Revised:2024-01-25 Online:2024-08-26 Published:2024-07-26
Supported by:
China Scholarship Fund(201506835005)

摘要/Abstract

摘要：

该文主要研究在单连通道路上具有不同压力项的耦合 Aw-Rascle-Zhang(CARZ) 交通流模型的黎曼问题, 利用特征分析法和相变的相关理论, 构造了文献 [5]缺失的情形 $v_- + \eta (\rho_-)^{\gamma} = v_+$ 的稳定显式解, 并修正了情形 $v_+ + \eta(\rho_-)^{\gamma} < v_+$ 的黎曼解, 完善了 Herty[5] 等人的工作. 当 CARZ 模型中压力项前面的参数 $\mu \to \eta$ 时, 证明了该模型黎曼解的唯一性和稳定性.

关键词: 耦合 Aw-Rascle-Zhang 交通流模型, 黎曼问题, 唯一性, 稳定性

Abstract:

This paper studies the Riemann problem of the coupled Aw-Rascle-Zhang traffic model with different pressure laws on the connected roads. Using the method of characteristic analysis and theories of phase transition, we construct the Riemann solution to the coupled Aw-Rascle-Zhang model for the ommitted case $v_- + \eta (\rho_-)^{\gamma} = v_+$ in reference [5], and correct Riemann solution for the case $v_+ + \eta(\rho_-)^{\gamma} < v_+$ , which complete the work of Herty, et al. Furthermore, when the parameter of the pressure term $\mu \to \eta$ , the uniqueness and stability of the Riemann solution of the coupled Aw-Rascle model are proved.

Key words: Coupled Aw-Rascle-Zhang model, Riemann problem, Uniqueness, Stability

中图分类号:

O175.27

潘丽君, 吕顺, 翁莎莎. 耦合Aw-Rascle-Zhang模型的Riemann解及其稳定性[J]. 数学物理学报, 2024, 44(4): 885-895.

Pan Lijun, Lv Shun, Weng Shasha. Riemann Solution and Stability of Coupled Aw-Rascle-Zhang Model[J]. Acta mathematica scientia,Series A, 2024, 44(4): 885-895.

图/表 6

图1

ARZ 模型 $v_- + \eta (\rho_-)^{\gamma} < v_+$ 情形下的黎曼解"

图1

图2

CARZ 模型 $v_- + \eta(\rho_-)^{\gamma} = v_+$ 情形下的黎曼解 ( $\rho_- \geqslant \rho_{\alpha}^{\eta}$ )"

图2

图3

CARZ 模型 $v_- + \eta(\rho_-)^{\gamma} = v_+$ 情形下的黎曼解 $(\rho_- < \rho_{\alpha}^{\eta})$ "

图3

图4

CARZ 模型 $v_- + \eta (\rho_-)^{\gamma} < v_+$ 且 $\rho_- v_- < \rho_{\alpha}^{\mu} v_{\alpha}^{\mu}$ 情形下的黎曼解 ( $\rho_- \geqslant \rho_{\alpha}^{\eta}$ )"

图4

图5

CARZ 模型 $v_- + \eta (\rho_-)^{\gamma} < v_+$ 且 $\rho_- v_- < \rho_{\alpha}^{\mu} v_{\alpha}^{\mu}$ 情形下的黎曼解 ( $\rho_- < \rho_{\alpha}^{\eta}$ )"

图5

图6

CARZ 模型 $v_- + \eta (\rho_-)^{\gamma} < v_+$ 且 $\rho_- v_- > \rho_{\alpha}^{\mu} v_{\alpha}^{\mu}$ 情形下的黎曼解"

图6

参考文献 10

[1]	Aw A, Rascle M. Resurrection of second order models of traffic flow. SIAM Journal on Applied Mathematics, 2000, 60(3): 916-938
[2]	Colombo R M, Goatin P, Piccoli B. Road networks with phase transitions. Journal of Hyperbolic Differential Equations, 2010, 7(1): 85-106
[3]	Goatin P. The Aw-Rascle vehicular traffic flow model with phase transitions. Mathematical and Computer Modelling, 2006, 44(3/4): 287-303
[4]	Gttlich S, Herty M, Moutari S, et al. Second-Order traffic flow models on networks. SIAM Journal on Applied Mathematics, 2021, 81(1): 258-281
[5]	Herty M, Schleper V. Traffic flow with unobservant drivers. ZAMM Journal of Applied Mathematics and Mechanics: Ztschrift Fur Angewandte Mathematik und Mechanik, 2011, 91(10): 763-776
[6]	Holden H, Risebro N H. A Mathematical model of traffic flow on a network of unidirectional roads. Siam Journal on Mathematical Analysis, 1995, 26(4): 999-1017
[7]	Jiang W F, Wang Z. Developing an Aw-Rascle model of traffic flow. Journal of Engineering Mathematics, 2016, 97(1): 135-146
[8]	Moutari S, Herty M, Klein A, et al. Modelling road traffic accidents using macroscopic second-order models of traffic flow. Ima Journal of Applied Mathematics, 2013, 78(5): 1087-1108
[9]	王文雷. 针对耦合的 AR 交通模型 Riemann 问题的研究. 江苏: 南京航空航天大学, 2021
	Wang W L. Study on the Riemann of Coupling AR model. Jiangsu: Nanjing University of Aeronautics and Astronautics, 2021
[10]	Zhang H M. A non-equilibrium traffic model devoid of gas-like behavior. Transportation Research Part B: Methodological, 2002, 36(3): 275-290

相关文章 15

[1]	郭燕, 徐小川. 基于混合谱数据的不连续Sturm-Liouville逆谱问题局部可解性和稳定性[J]. 数学物理学报, 2024, 44(4): 859-870.
[2]	章春国, 孙宝楠, 付煜之, 于欣. 具有局部阻尼的二维 Mindlin-Timoshenko 板系统的镇定[J]. 数学物理学报, 2024, 44(4): 946-959.
[3]	高彩霞, 赵东霞. 带干扰项的 ARZ 交通流模型的时滞控制与 ISS 稳定性[J]. 数学物理学报, 2024, 44(4): 960-977.
[4]	徐瑞, 周凯娟, 白宁. 一类基于游离病毒感染和细胞-细胞传播的宿主体内 HIV-1 感染动力学模型[J]. 数学物理学报, 2024, 44(3): 771-782.
[5]	何芬, 王振. 一类双曲椭圆混合型趋化模型的黎曼问题[J]. 数学物理学报, 2024, 44(2): 354-360.
[6]	邱仰聪, 王其如. 一阶非线性时标动态方程的 Hyers-Ulam-Rassias 稳定性[J]. 数学物理学报, 2024, 44(2): 465-475.
[7]	刘鑫艳, 李晓光. 一类非齐次非线性 Schrödinger 方程驻波的轨道稳定性[J]. 数学物理学报, 2024, 44(2): 476-483.
[8]	李远飞, 李丹丹, 石金诚. 温度相关双扩散模型在半无穷柱体上的结构稳定性[J]. 数学物理学报, 2024, 44(1): 120-132.
[9]	韩梦洁, 刘俊利, 张太雷. 基于两类媒介的炭疽传播模型的全局动力学分析[J]. 数学物理学报, 2024, 44(1): 227-245.
[10]	徐斐, 张勇. 分数阶 Burgers 方程时间周期弱解的唯一性与渐近稳定性[J]. 数学物理学报, 2023, 43(6): 1710-1722.
[11]	马亚妮, 袁海龙. 一类具有时滞的 Gierer-Meinhardt 活化抑制模型的分支分析[J]. 数学物理学报, 2023, 43(6): 1774-1788.
[12]	庞玉婷, 赵东霞. 星形明渠网络系统的 PDP 反馈控制和指数镇定[J]. 数学物理学报, 2023, 43(6): 1803-1813.
[13]	范示示, 李海侠, 路银豆. 具有捕获项的 Beddington-DeAnglis 型捕食-食饵扩散模型的动力学分析[J]. 数学物理学报, 2023, 43(6): 1929-1942.
[14]	庞玉婷,赵东霞,赵鑫,高彩霞. 一类 2 $\times$ 2 双曲偏微分系统的 PDP 边界控制[J]. 数学物理学报, 2023, 43(5): 1471-1482.
[15]	廖远康. 粘性依赖于密度的一维等熵可压缩 Navier-Stokes 方程组粘性激波的非线性稳定性[J]. 数学物理学报, 2023, 43(4): 1149-1169.