数学物理学报(英文版) ›› 2024, Vol. 44 ›› Issue (6): 2443-2464.doi: 10.1007/s10473-024-0621-1
THE STABILITY OF AF-RELATIONS
Jiajie HUA
- College of Data Science, Jiaxing University, Jiaxing 314001, China
-
收稿日期:2023-06-16修回日期:2024-07-13发布日期:2024-12-06 -
作者简介:Jiajie HUA, E-mail: jiajiehua@zjxu.edu.cn -
基金资助:Scientific Research Fund of Zhejiang Provincial Education Department (Y202249575), the National Natural Science Foundation of China (11401256) and the Zhejiang Provincial Natural Science Foundation of China (LQ13A010016).
THE STABILITY OF AF-RELATIONS
Jiajie HUA
- College of Data Science, Jiaxing University, Jiaxing 314001, China
-
Received:2023-06-16Revised:2024-07-13Published:2024-12-06 -
About author:Jiajie HUA, E-mail: jiajiehua@zjxu.edu.cn -
Supported by:Scientific Research Fund of Zhejiang Provincial Education Department (Y202249575), the National Natural Science Foundation of China (11401256) and the Zhejiang Provincial Natural Science Foundation of China (LQ13A010016).
摘要: For given 











































































































































































































































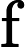

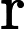























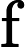

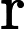










中图分类号:
- 46L05
引用本文
Jiajie HUA. THE STABILITY OF AF-RELATIONS[J]. 数学物理学报(英文版), 2024, 44(6): 2443-2464.
Jiajie HUA. THE STABILITY OF AF-RELATIONS[J]. Acta mathematica scientia,Series B, 2024, 44(6): 2443-2464.
使用本文
| [1] Berg I D, Davidson K R.Almost commuting matrices and the Brown-Douglas-Fillmore theorem. Bull Amer Math Soc (NS), 1987, 16(1): 97-100 [2] Boca F P.Projections in rotation algebras and theta functions. Commun Math Phys, 1999, 202(2): 325-357 [3] Bratteli O, Elliott G A, Evans D E, et al.Noncommutative spheres Ⅰ. Internat J Math, 1991, 2(2): 139-166 [4] Bratteli O, Elliott G A, Evans D E, et al.Noncommutative spheres Ⅱ: Rational rotations. J Operator Theory, 1992, 27(1): 53-85 [5] Bratteli O, Kishimoto A.Noncommutative spheresⅢ, Irrational rotations. Commun Math Phys, 1992, 147(3): 605-624 [6] Brenken B A.Representations and automorphisms of the irrational rotation algebra. Pacific J Math, 1984, 111(2): 257-282 [7] Chakraborty S.Symmetrized non-commutative tori revisited. To appear in Journal of Noncommutative Geometry, arXiv:2204.11546 [8] Chakraborty S, Hua J J.Higher dimensional bott classes and the stability of rotation relations. Indiana Univ Math J, 2023, 72(6): 2285-2339 [9] Davidson K R.Almost commuting Hermitian matrices. Math Scand, 1985, 56(2): 222-240 [10] Echterhoff S, Lück W, Phillips N C, et al.The structure of crossed products of irrational rotation algebras by finite subgroups of       [11] Eilers S, Loring T A.Computing contingencies for stable relations. Internat J Math, 1999, 10(3): 301-326 [12] Eilers S, Loring T A, Pedersen G K.Morphisms of extensions of   [13] Eilers S, Loring T A, Pedersen G K.Stability of anticommutation relations: an application of noncommutative CW complexes. J Reine Angew Math, 1998, 499: 101-143 [14] Elliott G A.On the K-theory of the   [15] Exel R, Loring T A.Almost commuting unitary matrices. Proc Amer Math Soc, 1989, 106(4): 913-915 [16] Exel R, Loring T A.Invariants of almost commuting unitaries. J Funct Anal, 1991, 95(2): 364-376 [17] Fan Q, Fang X.Crossed products by finite group actions with certain tracial Rokhlin property. Acta Math Sci, 2018, 38B(3): 829-842 [18] Friis P, Rørdam M.Almost commuting self-adjoint matrices-a short proof of Huaxin Lin's theorem. J Reine Angew Math, 1996, 479: 121-131 [19] Farsi C, Watling N.   [20] Farsi C, Watling N.Symmetrized non-commutative tori. Math Ann, 1993, 296(4): 739-741 [21] Gong G H, Lin H X.Almost multiplicative morphisms and  [22] Halmos P R. Some unsolved problems of unknown depth about operators on Hilbert space. Proc Roy Soc Edinburgh Sect A, 1976/77, 76(1): 67-76 [23] Hua J J. Stability of certain relations of three unitaries in   [24] Hua J J. Stability of rotation relation of two unitaries with the flip action in   [25] Hua J J.Stability of rotation relation of two unitaries with          [26] Hua J J, Fang X C, Xu X M.Continuity of functors with respect to generalized inductive limits. Front Math China, 2019, 14(3): 551-566 [27] Hua J J, Lin H X.Rotation algebras and the Exel trace formula. Canad J Math, 2015, 67(2): 404-423 [28] Hua J J, Wang Q Y.Stability of rotation relations in   [29] Hua J J, Wang Z J.Stability of rotation relation of two unitaries with the flip action in   [30] Kumjian A.On the  [31] Lin H X.Almost commuting selfadjoint matrices and applications// Operator Algebras and Their Applications (Waterloo, ON, 1994/1995), Fields Inst Commun, vol 13. Providence, RI: Amer Math Soc, 1997: 193-233 [32] Lin H X.An Introduction to the Classification of Amenable   [33] Newman M. Integral Matrices.New York: Academic Press, 1972 [34] Phillips N C.Every simple higher dimensional noncommutative torus is an   [35] Rieffel M A.Dimension and stable rank in the    [36] Rieffel M A.Non-commutative tori-a case study of non-commutative differentiable manifolds. Contemporary Mathematics, 1990, 105: 191-211 [37] Rieffel M A.Projective modules over higher dimensioanl noncommutative tori. Canad J Math, 1988, 40(2): 257-338 [38] R      [39] Rosenthal P.Research Problems: Are almost commuting matrices near commuting matrices?. Amer Math Monthly, 1969, 76(8): 925-926 [40] Voiculescu D.Asymptotically commuting finite rank unitary operators without commuting approximants. Acta Sci Math (Szeged), 1983, 45: 429-431 [41] Voiculescu D.Remarks on the singular extension in the   [42] Walters S.Projective modules over the non-commutative sphere. J London Math Soc, 1995, 51(2): 589-602 [43] Walters S.Toroidal orbifolds of     [44] Wang Z J, Hu J Y, Hua J J.Stability of rotation relations of three unitaries with the flip action in   [45] Watatani Y.Toral automorphisms on irrational rotation algebras. Math Japon, 1981, 26(4): 479-484 |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 4
|
|
|||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 30
|
|
|||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||
|
