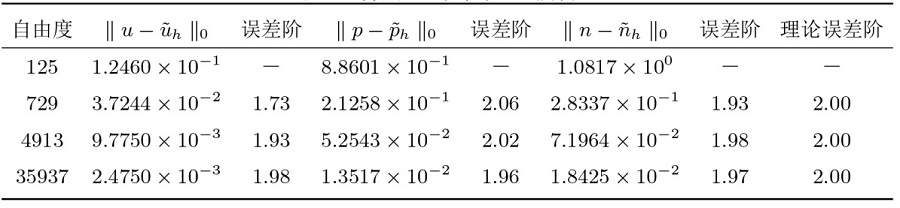
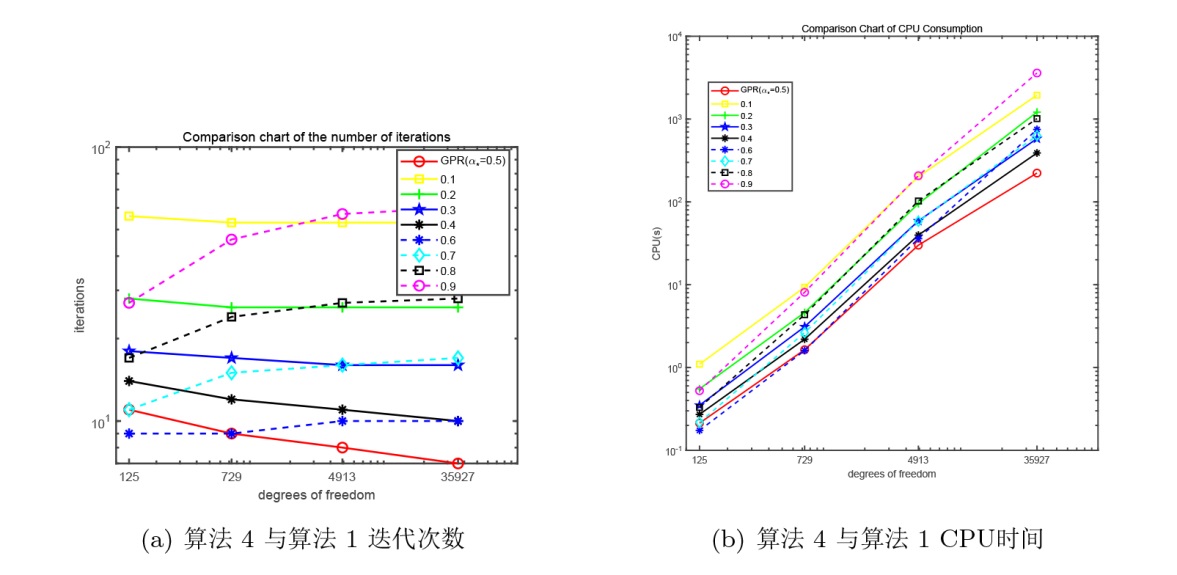
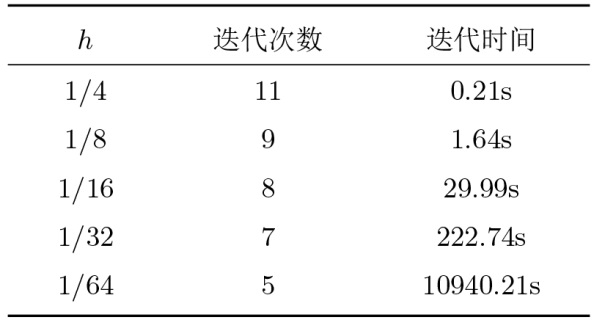
| [1] |
Lu B Z, Holst M J, McCammon J A, et al. Poisson-Nernst-Planck equations for simulating biomolecular diffusion-reaction processes I: Finite element solutions. Journal of Computational Physics, 2010, 229(19): 6979-6994
pmid: 21709855
|
| [2] |
Silva V L S, Salinas P, Jackson M D, et al. Machine learning acceleration for nonlinear solvers applied to multiphase porous media flow. Computer Methods in Applied Mechanics and Engineering, 2021, 384: 113989
doi: 10.1016/j.cma.2021.113989
|
| [3] |
Jiang K, Su X H, Zhang J. A General alternating-direction implicit framework with Gaussian process regression parameter prediction for large sparse linear systems. SIAM Journal on Scientific Computing, 2022, 44(4): A1960-A1988
|
| [4] |
Uyan O G, Akbas A, Gungor V C. Machine Learning Approaches for Underwater Sensor Network Parameter Prediction. Ad Hoc Networks, 2023, 144: 103139
|
| [5] |
Singh S K, Das A K, Singh S R, et al. Prediction of rail-wheel contact parameters for a metro coach using machine learning. Expert Systems with Applications, 2023, 215: 119343
|
| [6] |
Wu S d, Lu B Z. INN: Interfaced neural networks as an accessible meshless approach for solving interface PDE problems. Journal of Computational Physics, 2022, 470: 111588
|
| [7] |
Wang Q, Li H L, Zhang L B, et al. A stabilized finite element method for the Poisson-Nernst-Planck equations in three-dimensional ion channel simulations. Applied Mathematics Letters, 2020, 111: 106652
|
| [8] |
Yang Y, Tang M, Zhong L Q, et al. Superconvergent gradient recovery for nonlinear Poisson-Nernst-Planck equations with applications to the ion channel problem. Advances in Computational Mathematics, 2020, 46(6): 1-35
|
| [9] |
Seeger M. Gaussian processes for machine learning. International Journal of Neural Systems, 2004, 14(2): 69-106
pmid: 15112367
|
| [10] |
Williams C K I, Rasmussen C E. Gaussian Processes for Machine Learning. Cambridge: MIT Press, 2006
|
| [11] |
Box G E P, Cox D R. An analysis of transformations. Journal of the Royal Statistical Society: Series B (M ethodological), 1964, 26(2): 211-252
|
| [12] |
Yang Y, Lu B Z. An error analysis for the finite element approximation to the steady-state Poisson-Nernst-Planck equations. Advances in Applied Mathematics and Mechanics, 2013, 5(1): 113-130
|