具有异质空间扩散的梅毒模型的阈值动力学分析与仿真

数学物理学报 ›› 2024, Vol. 44 ›› Issue (5): 1352-1367.

具有异质空间扩散的梅毒模型的阈值动力学分析与仿真

吴鹏¹(),方诚^2,^*()

¹杭州电子科技大学理学院杭州 310018
²浙江财经大学数据科学学院杭州 310018

收稿日期:2023-07-11 修回日期:2024-04-16 出版日期:2024-10-26 发布日期:2024-10-16
通讯作者: *方诚, E-mail: fangcheng12@zufe.edu.cn
作者简介:吴鹏, E-mail: hzpengwu@163.com
基金资助:
国家自然科学基金(12201557);国家自然科学基金(12001483);浙江省教育厅一般项目(Y202249921);安徽省自然科学基金(2108085MA10)

Dynamical Analysis and Numerical Simulation of a Syphilis Epidemic Model with Heterogeneous Spatial Diffusion

Wu Peng¹(),Fang Cheng^2,^*()

¹School of Sciences, Hangzhou Dianzi University, Hangzhou 310018
²School of Data Sciences, Zhejiang University of Finance and Economics, Hangzhou 310018

Received:2023-07-11 Revised:2024-04-16 Online:2024-10-26 Published:2024-10-16
Supported by:
NSFC(12201557);NSFC(12001483);Foundation of Zhejiang Provincial Education Department(Y202249921);Anhui Provincial Natural Science Foundation(2108085MA10)

摘要/Abstract

摘要：

为了研究个体扩散和空间异质环境对梅毒传播的影响, 该文构建了一类具异质空间反应扩散梅毒动力学模型. 首先, 研究了模型的适定性问题, 包括解的全局存在性、系统的耗散性和解半流吸引子存在性; 其次利用下一代再生算子定义推导出模型的基本再生数 $R_0$ 的泛函表达式; 再次, 讨论了系统解关于阈值-$R_0$ 的动力学行为, 具体地, 当 $R_0>1$ 时, 无病平衡态是全局稳定的, 当 $R_0>1$ 时, 系统是一致持久的. 在特殊情形下, 还证明了系统常数正平衡点的存在唯一性和全局稳定性. 最后, 通过数值模拟验证了理论结果并分析了空间因素对梅毒传播的影响. 数值结果表明: (1) 加强对隐性梅毒感染者的治疗可以有效地降低梅毒在人群中传播的风险; (2) 忽略空间异质性将会低估梅毒的流行趋势. 另外, 个体扩散率对梅毒传播的影响同样也是不容忽视的.

关键词: 梅毒, 反应扩散模型, 空间异质, 基本再生数, 阈值动力学, 数值模拟

Abstract:

To study the effects of individual diffusion and spatial heterogeneity on the transmission of syphilis, we construct a heterogeneous spatial reaction diffusion model of syphilis. Firstly, the well posed problem of the model is studied, including the global existence of the solution, the dissipativity of the system and the existence of the attractor for the semiflow; Secondly, based on the definition of the next generation regeneration operator, we derive the functional expression of the basic regeneration number $R_0$; Thirdly, we discussed the dynamical behaviors of the solution regarding the threshold-$R_0 $, specifically, when $R_0>1$, the disease-free steady state is globally stable, when $R_0>1 $, the system is uniformly persistent. In special cases, we also prove the existence, uniqueness, and global stability of the positive equilibrium of the system. Finally, the theoretical results were validated and the influence of spatial factors on the transmission of syphilis was analyzed through numerical simulation. Our numerical results indicate that: (1) strengthening the treatment of early latent syphilis carriers can effectively reduce the risk of syphilis transmission among population; (2) Ignoring spatial heterogeneity will underestimate the epidemic trend of syphilis. In addition, the impact of individual diffusion rate on the transmission of syphilis cannot be ignored.

Key words: Syphilis, Reaction-diffusion model, Spatial heterogeneity, Basic reproduction number, Threshold dynamics, Numerical simulation

中图分类号:

O175.23

吴鹏, 方诚. 具有异质空间扩散的梅毒模型的阈值动力学分析与仿真[J]. 数学物理学报, 2024, 44(5): 1352-1367.

Wu Peng, Fang Cheng. Dynamical Analysis and Numerical Simulation of a Syphilis Epidemic Model with Heterogeneous Spatial Diffusion[J]. Acta mathematica scientia,Series A, 2024, 44(5): 1352-1367.

图/表 8

图1

图2

图3

图4

图5

图6

图7

图8

参考文献 21

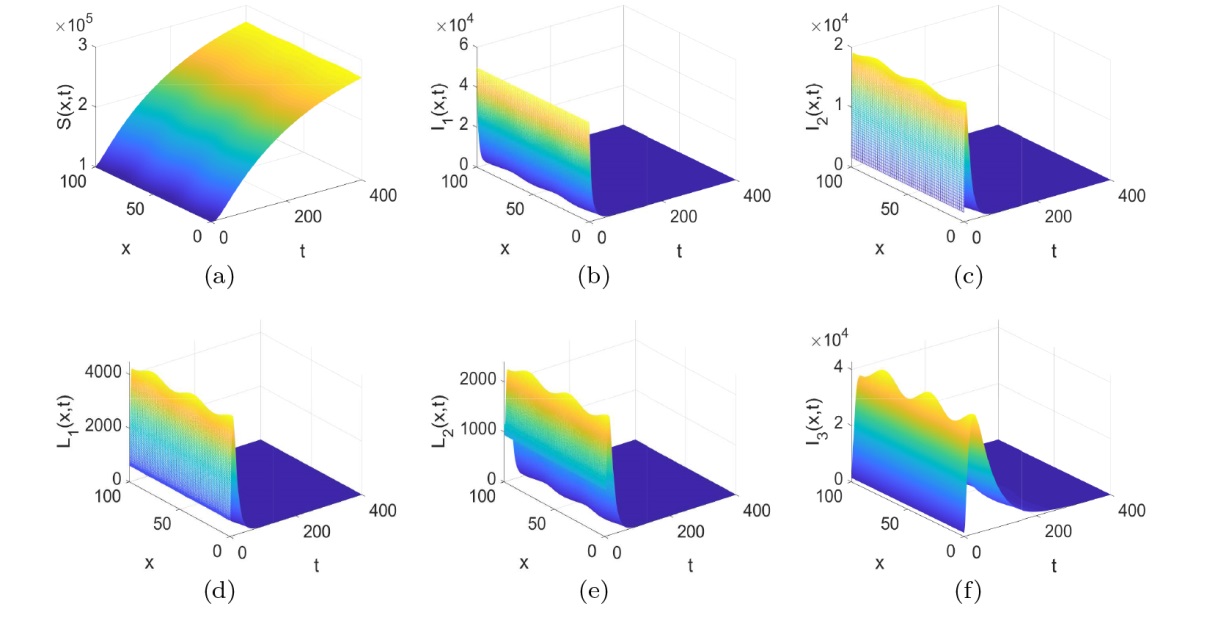
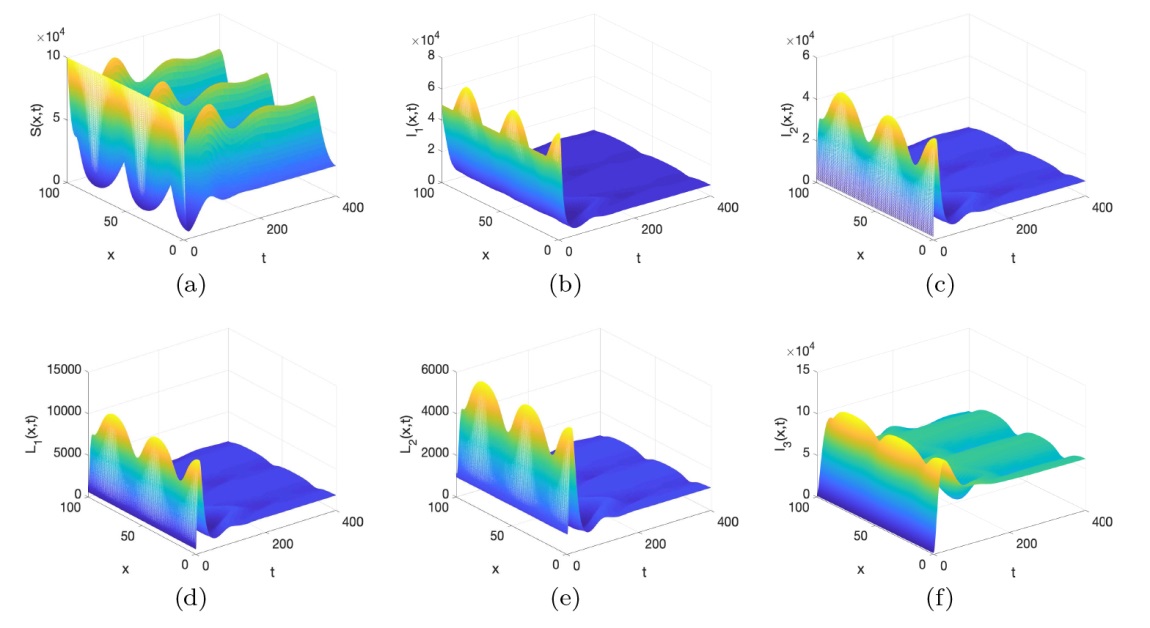
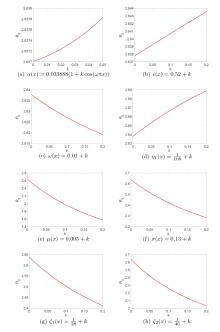
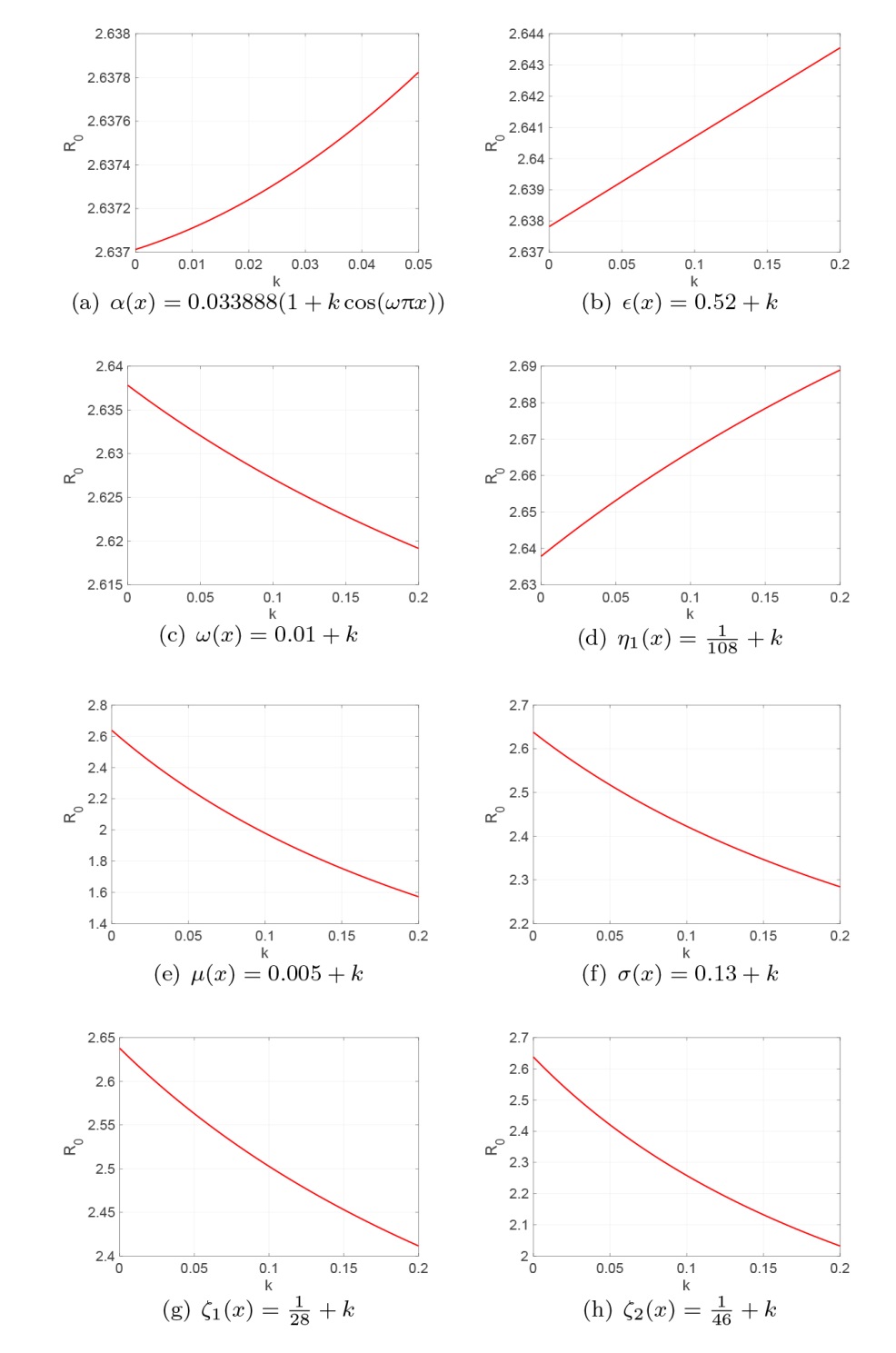
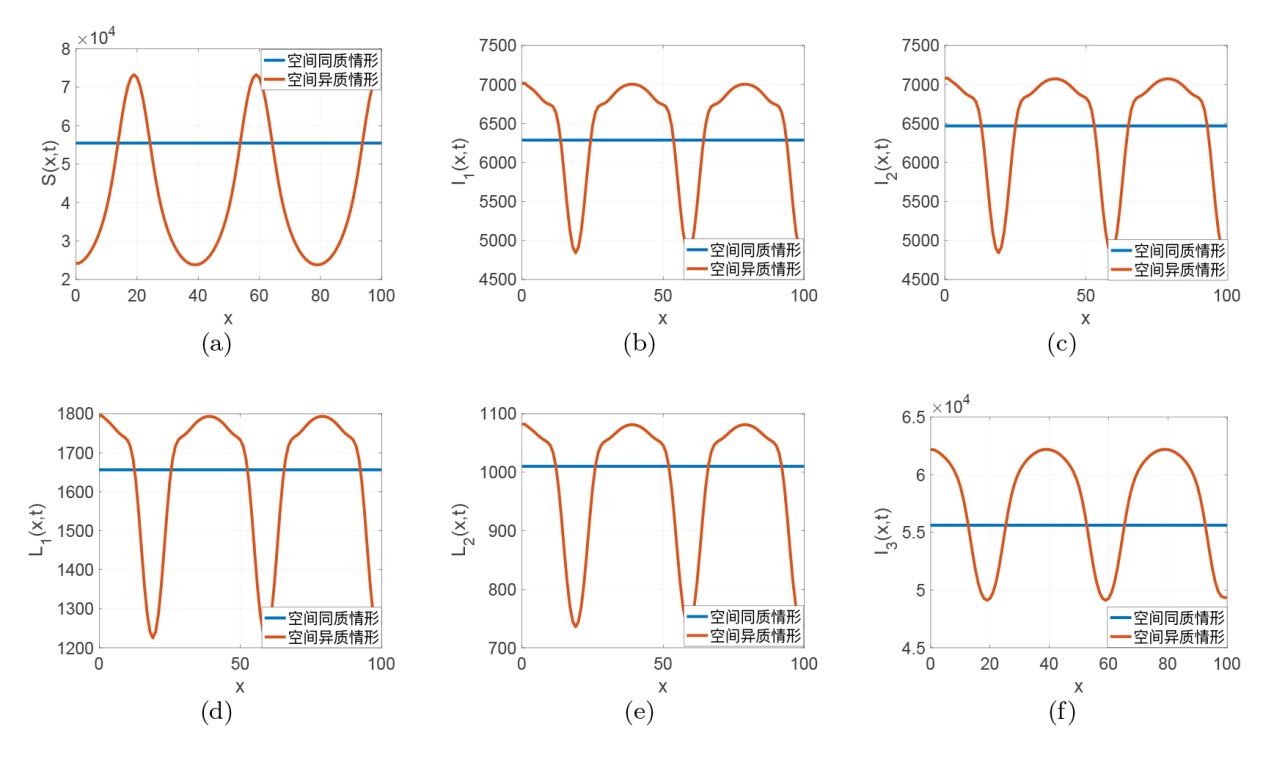
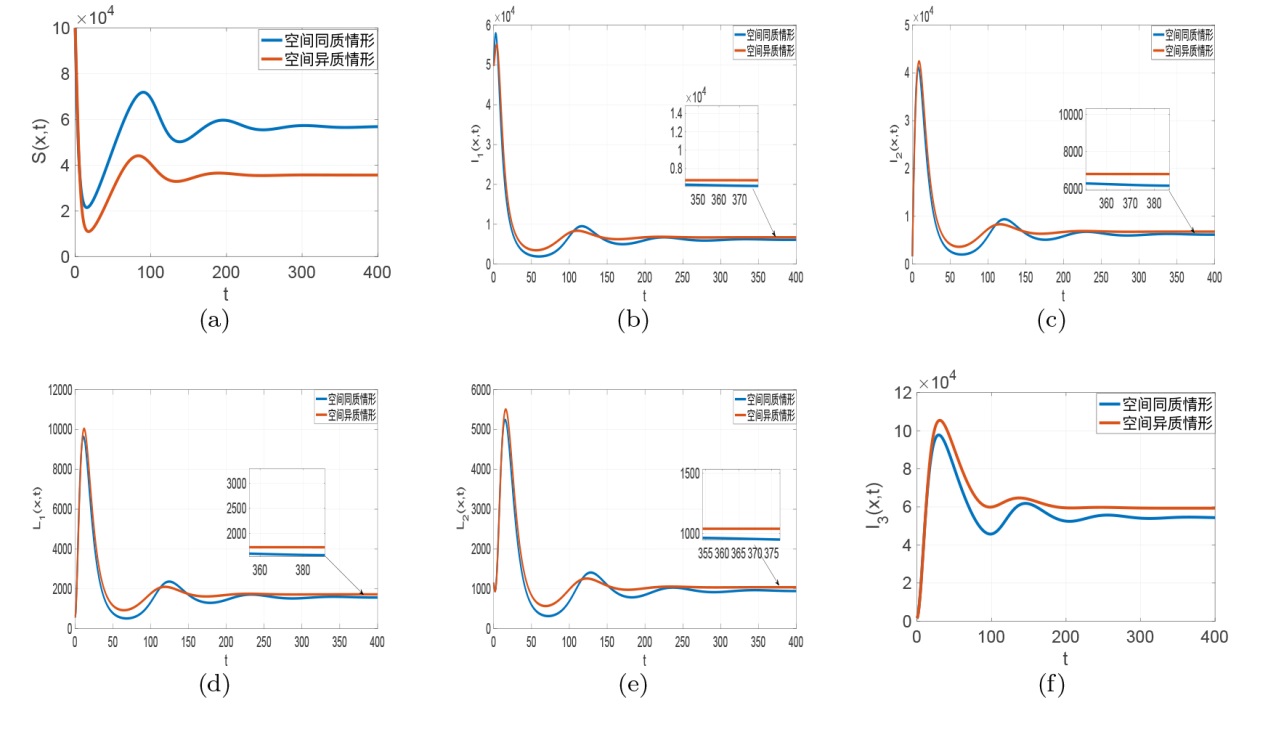
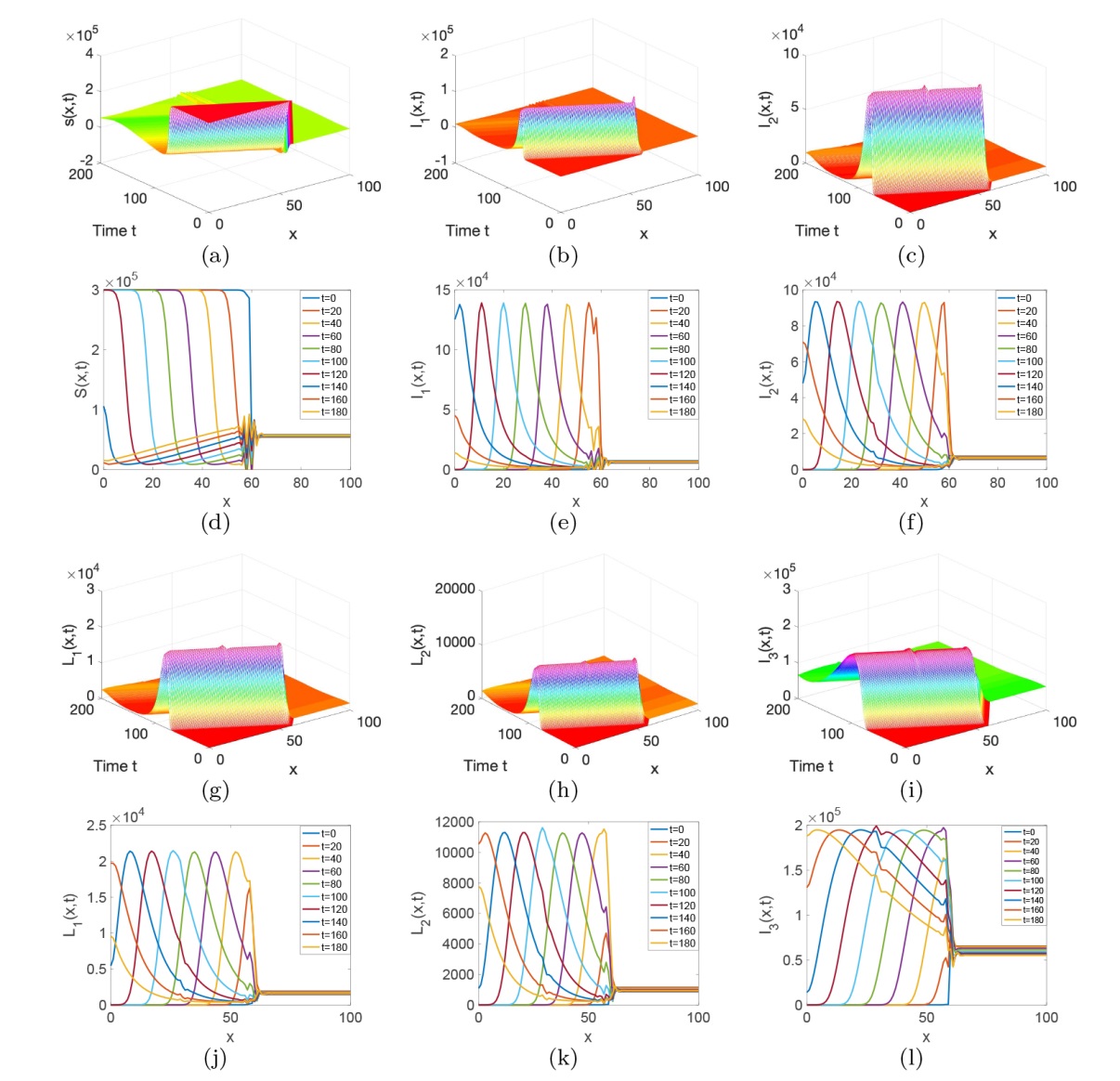
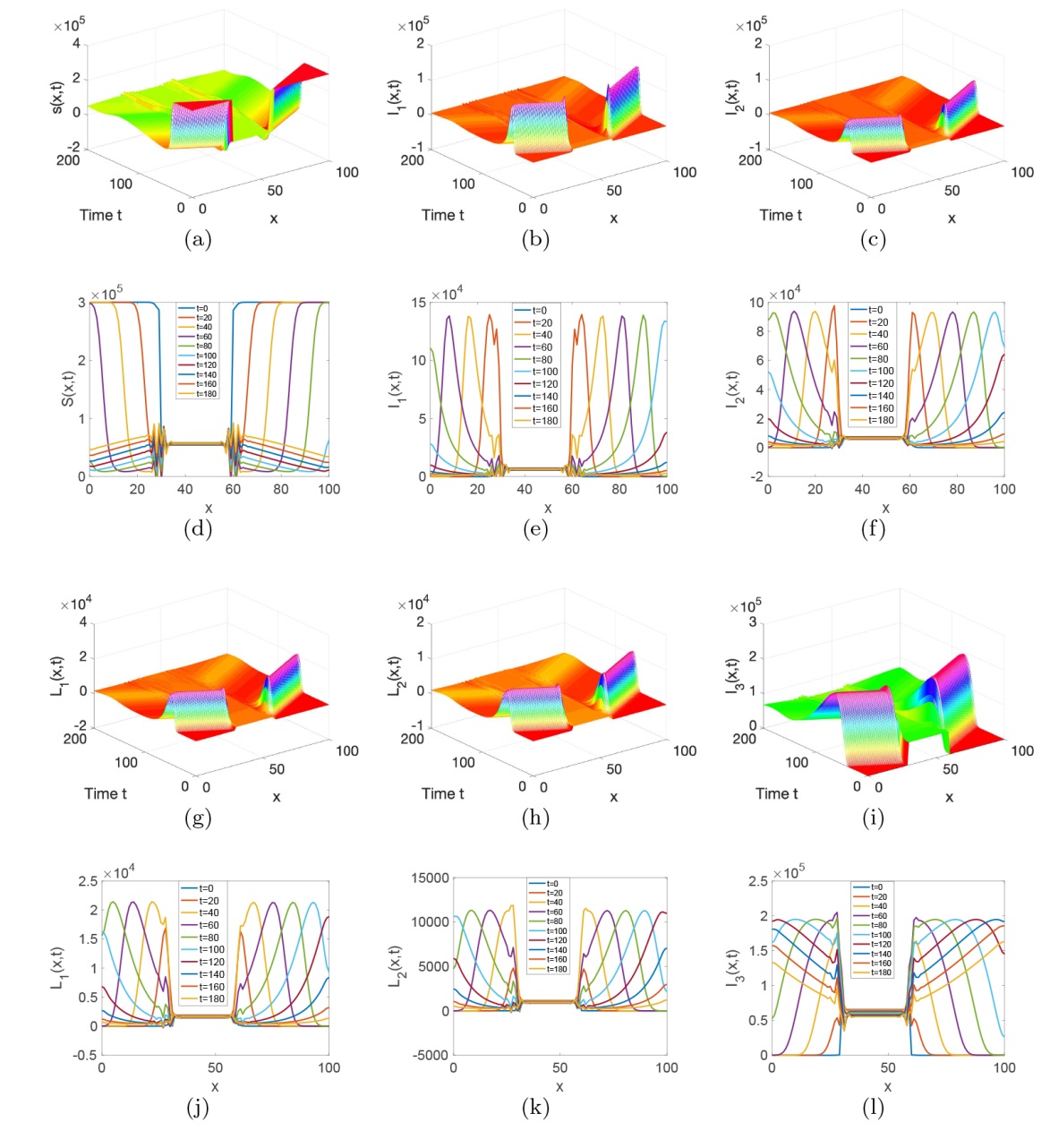
[1]	Sparling T F. Natural history of syphilis// Helmes KK, et al. Sexually Transmitted Diseases, New York: McGrans-Hill Book co. 1981: 298-299
[2]	Mathew A B, Michael M, et al. Global phylogeny of Treponema pallidum lineages reveals recent expansion and spread of contemporary syphilis. Nature Microbiology, 2021, 6(12): 1549-1560 doi: 10.1038/s41564-021-01000-z pmid: 34819643
[3]	National Institute of Infectious Diseases, Infectious agents surveillance report, 2020, https://www.niid.go.jp/niid/en/iasr-e.html [2023-6-11]
[4]	Garnett G P, Aral S O, Hoyle D V, et al. The natural history of syphilis: Implications for the transmission dynamics and control of infection. Sexually Transmitted Dis, 1997, 24(4): 185-200
[5]	Fenton K A, Breban R, Vardavas R, et al. Infectious syphilis in high-income settings in the 21st century. Lancet Infect Dis, 2008, 8(4): 244-253 doi: 10.1016/S1473-3099(08)70065-3 pmid: 18353265
[6]	Grassly C, Fraser C, Garnett G P. Host immunity and synchronized epidemics of syphilis across the United States. Nature, 2005, 433(7024): 417-421
[7]	Iboi E, Okuonghaea D. Population dynamics of a mathematical model for syphilis. Appl Math Model, 2016, 40: 3573-3590
[8]	Saad-Roy C M, Shuai Z, van den Driessche P. A mathematical model of syphilis transmission in an MSM population. Math Biosci, 2016, 277: 59-70 doi: 10.1016/j.mbs.2016.03.017 pmid: 27071977
[9]	Gumel A B, Lubuma J M-S, Sharomi O, Terefe Y A. Mathematics of a sex-structured model for syphilis transmission dynamics. Math Meth Appl Sci, 2017, 23: 26-47
[10]	Nwankwo A, Okuonghae D. Mathematical analysis of the transmission dynamics of HIV syphilis co-infection in the presence of treatment for syphilis. Bull Math Biol, 2018, 80: 437-492
[11]	Omame A, Okuonghae D, Nwafor U E, et al. A co-infection model for HPV and syphilis with optimal control and cost-effectiveness analysis. Int J Biomath, 2021, 14: 2150050
[12]	吴鹏, 赵洪涌. 基于空间异质反应扩散 HIV 感染模型的最优治疗策略. 应用数学学报, 2022, 45(5): 752-766
	Wu P, Zhao H. Optimal treatment strategies for a reaction-dffusion HIV infection model with spatial heterogeneity. Acta Math Appl Sinica, 2022, 45(5): 752-766
[13]	Wang X, Wang H, Michael Y L. Modeling rabies transmission in spatially heterogeneous environments via $\theta$-diffusion. Bull Math Biol, 2021, 83: Article 16
[14]	Gao Y, Wang J. Threshold dynamics of a delayed nonlocal reaction-diffusion HIV infection model with both cell-free and cell-to-cell transmissions. J Math Anal Appl, 2020, 488: 1-21
[15]	Smith H L. Monotone Dynamical Systems: An Introduction to the Theory of Competitive and Cooperative Systems. Providence, RI: American Mathematical Society, 1995
[16]	叶其孝, 李元正, 王明新, 吴雅萍. 反应扩散方程引论. 北京: 科学出版社, 2016
	Ye Q X, Li Z Y, Wang M X, Wu Y P. Introduction to Reaction Diffusion Equations. Beijing: Science Press, 2016
[17]	Martin R H, Smith H L. Abstract functional differential equations and reaction-diffusion systems. Trans Am Math Soc, 1990, 321: 1-44
[18]	Wu J. Theory and Applications of Partial Functional-Differential Equations. Vol 119. New York: Springer, 1996
[19]	Hale J. Asymptotic Behavior of Dissipative Systems. Province, RI: American Mathematical Society, 1988
[20]	Pazy A. Semigroups of Linear Operators and Applications to Partial Differential Equations. Applied Mathematical Science. New York: Springer-Verlag, 1983
[21]	Ren X, Tian Y, Liu L, Liu X. A reaction-diffusion within-host HIV model with cell-to-cell transmission. J Math Biol, 2018, 76(7): 1831-1872 doi: 10.1007/s00285-017-1202-x pmid: 29305736