基于添加粘度抵消项的 MRT-LBM 对高雷诺数流态的研究

数学物理学报 ›› 2024, Vol. 44 ›› Issue (5): 1216-1229.

基于添加粘度抵消项的 MRT-LBM 对高雷诺数流态的研究

张宗宁¹,张巧玲^2,^*(),景何仿³,沈启霞¹

¹郑州科技学院郑州 450064
²广东理工学院广东肇庆 526100
³北方民族大学银川 750021

收稿日期:2023-12-29 修回日期:2024-04-28 出版日期:2024-10-26 发布日期:2024-10-16
通讯作者: *张巧玲, E-mail: zhangqiaoling@gdlgxy.edu.cn
基金资助:
国家自然科学基金(11861003);国家自然科学基金(11761005);宁夏自然科学基金(2023AAC02049);宁夏自然科学基金(2022AAC02004);河南省高校重点科研项目(24B110020)

Research on High Reynolds Number Flow Using MRT-LBM with Viscosity Counteracting

Zhang Zongning¹,Zhang Qiaoling^2,^*(),Jing Hefang³,Shen Qixia¹

¹Department of Basic Courses, Zhengzhou University of Science and Technology, Zhengzhou 450064
²Department of Teaching and Research of Basic Courses, Guangdong Technology College, Guangdong Zhaoqing 526100
³School of Civil Engineering, North Minzu University, Yinchuan 750021

Received:2023-12-29 Revised:2024-04-28 Online:2024-10-26 Published:2024-10-16
Supported by:
NSFC(11861003);NSFC(11761005);Ningxia Natural Science Foundation(2023AAC02049);Ningxia Natural Science Foundation(2022AAC02004);Key scientific research project of Henan(24B110020)

摘要/Abstract

摘要：

该文探究了添加粘度抵消方法 (viscosity counteracting, VC) 的多松弛格子玻尔兹曼方法 (multiple-relaxation-time lattice Boltzmann method, MRT-LBM), 即 MRT-VC 方法可以模拟的最大雷诺数. 首先, 模拟了经典二维顶盖驱动方腔流来验证模型的准确性, 重点分析了雷诺数为 5430 和 7000 的流场, 并分析了方腔流流场、涡心坐标、轴向速度和速度空间图谱. 其次, 随着模拟雷诺数的增大, 流场内旋涡的数目逐渐增加, 且流动依次呈现出稳定流、周期流、不完全混沌流、混沌流等状态. 从稳定流到周期流的临界跃迁雷诺数在 10000-12500 之间, 周期流到不完全混沌态流的临界跃迁雷诺数在 45000-50000 之间, 不完全混沌态流到混沌流的临界跃迁雷诺数在 95000-100000 之间.

关键词: 多松弛时间格子玻尔兹曼方法, 粘度抵消方法, 高雷诺数流, 临界跃迁雷诺数

Abstract:

This paper explores the maximum Reynolds number that can be simulated by the multiple-relaxation-time lattice Boltzmann method with viscosity counteracting (MRT-VC). Firstly, the accuracy of the model is validated by simulating the classic 2D lid-driven cavity flow. The focus is on the flow fields at Reynolds numbers of 5430 and 7000, analyzing the flow fields, vortex core coordinates, axial velocity, and velocity spectra. Secondly, as the simulated Reynolds number increases, the number of swirling vortices in the flow field gradually increases. The flow exhibits a sequence of stable flow, periodic flow, incomplete chaotic flow, and chaotic flow. The critical transition Reynolds number from stable flow to periodic flow is between 10000 and 12500, from periodic flow to incomplete chaotic flow is between 45000 and 50000, and from incomplete chaotic flow to chaotic flow is between 95000 and 100000.

Key words: Multiple-relaxation-time lattice Boltzmann method, Viscosity counteracting approach, High reynolds number flow, Critical transition reynolds number

中图分类号:

O357.5

张宗宁, 张巧玲, 景何仿, 沈启霞. 基于添加粘度抵消项的 MRT-LBM 对高雷诺数流态的研究[J]. 数学物理学报, 2024, 44(5): 1216-1229.

Zhang Zongning, Zhang Qiaoling, Jing Hefang, Shen Qixia. Research on High Reynolds Number Flow Using MRT-LBM with Viscosity Counteracting[J]. Acta mathematica scientia,Series A, 2024, 44(5): 1216-1229.

图/表 17

图1

表1

图2

表2

图3

图4

图5

图6

图7

图8

图9

图10

图11

图12

图13

图14

图15

参考文献 27

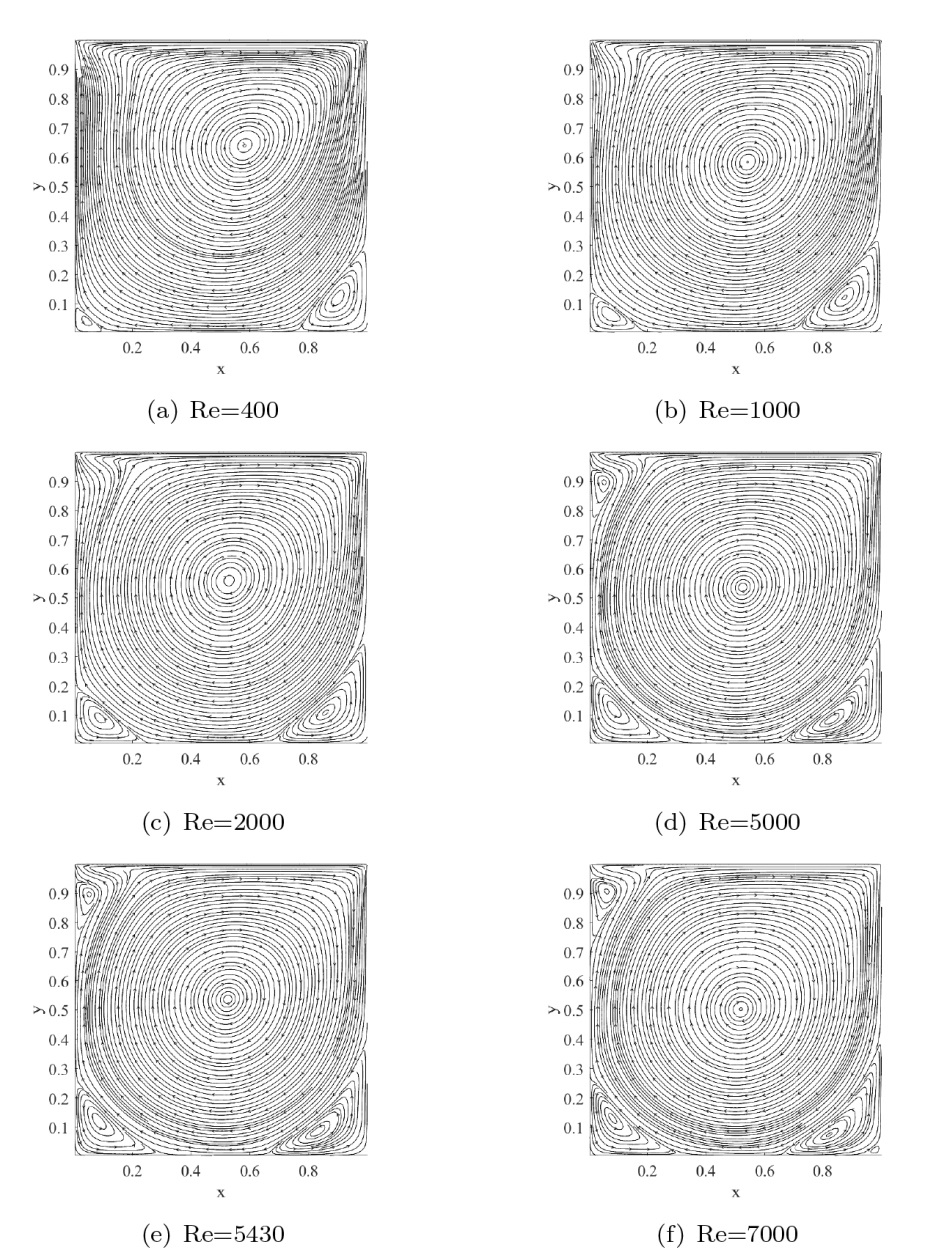
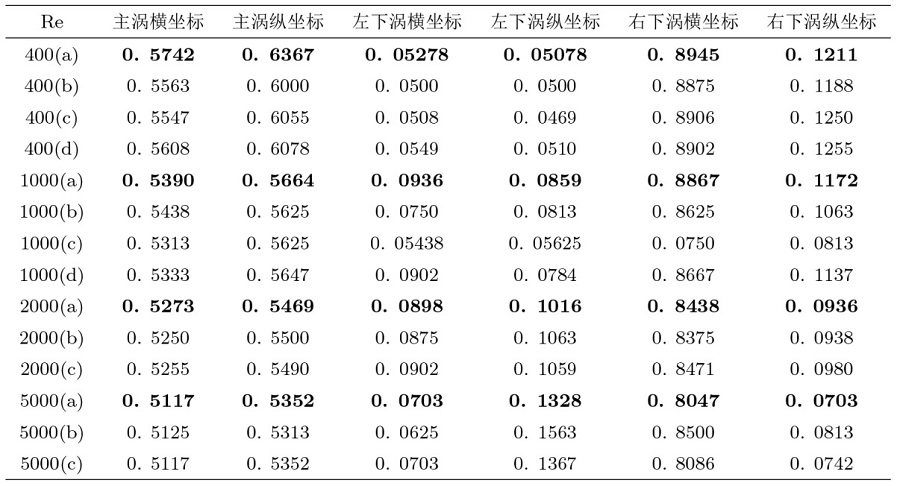
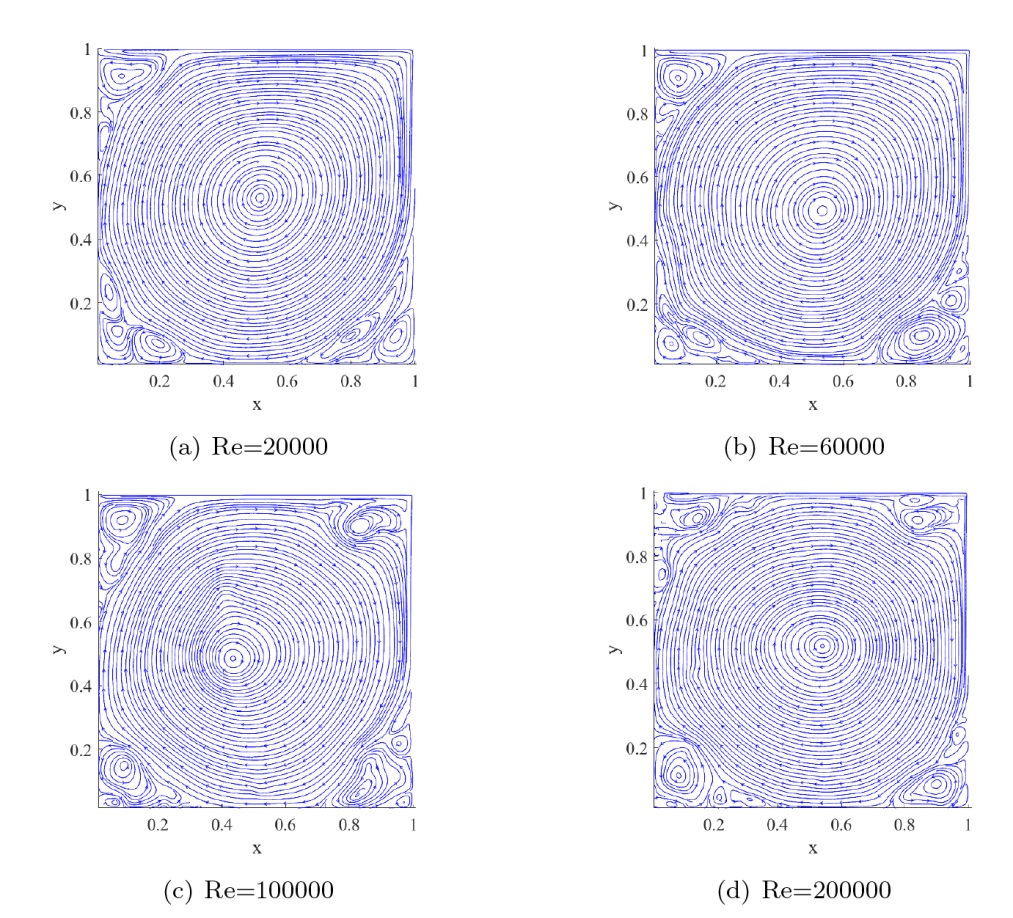
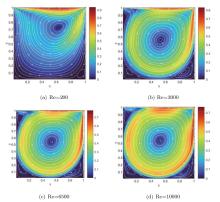
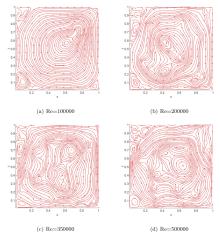
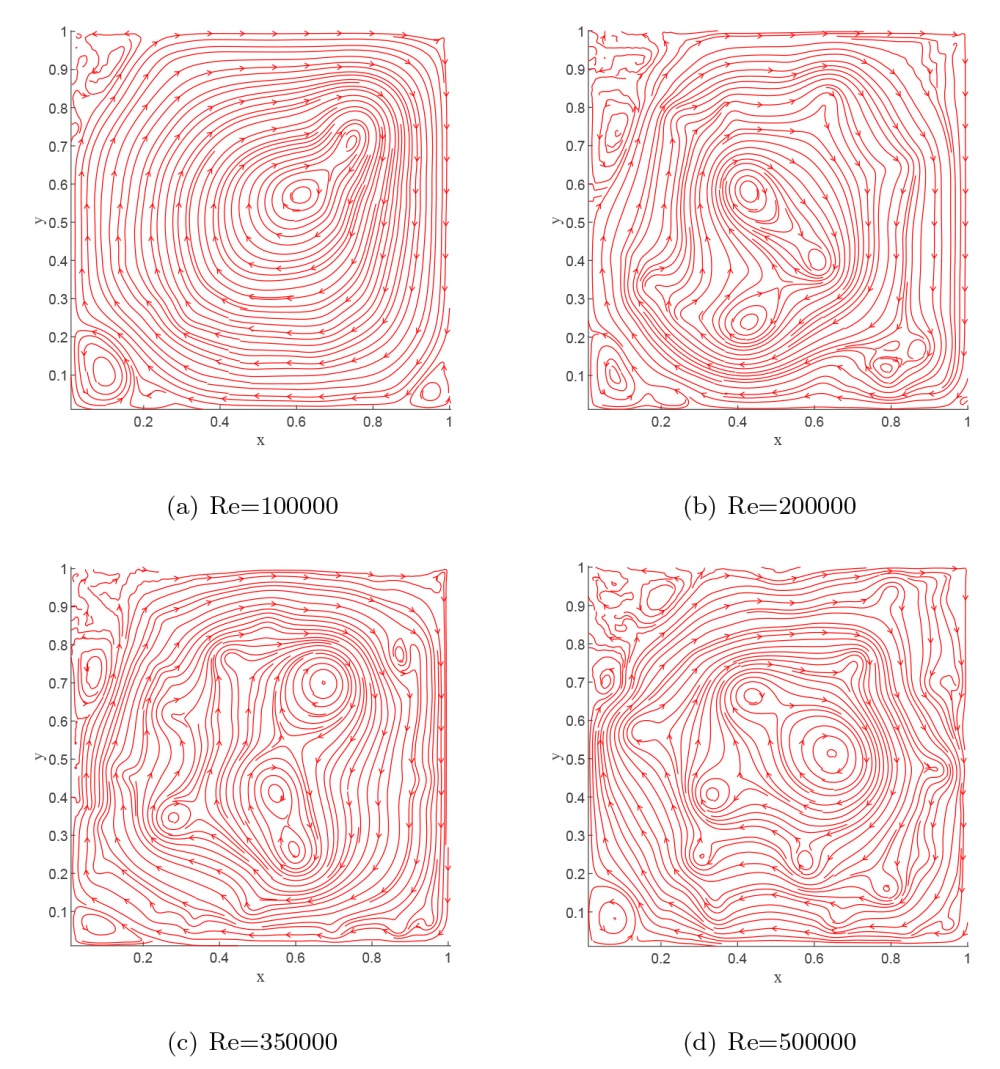
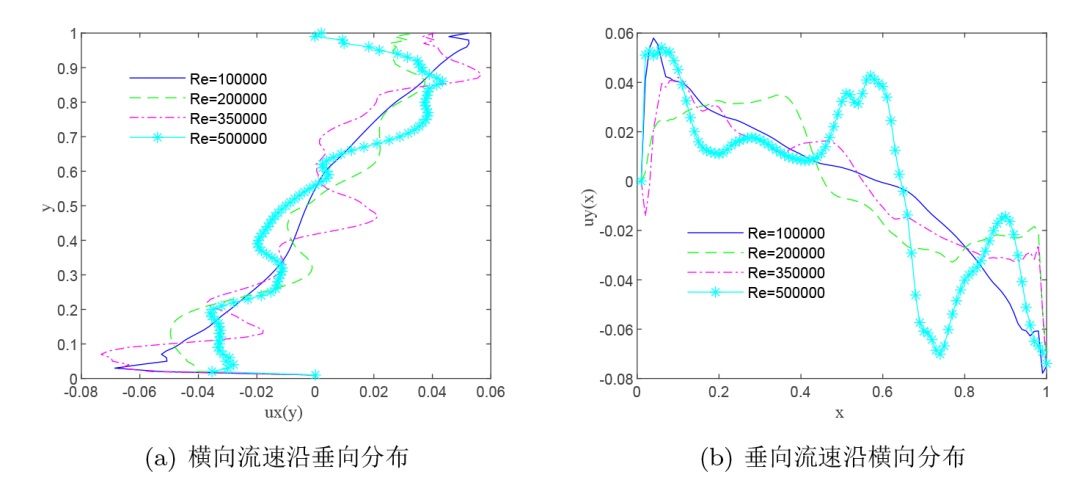
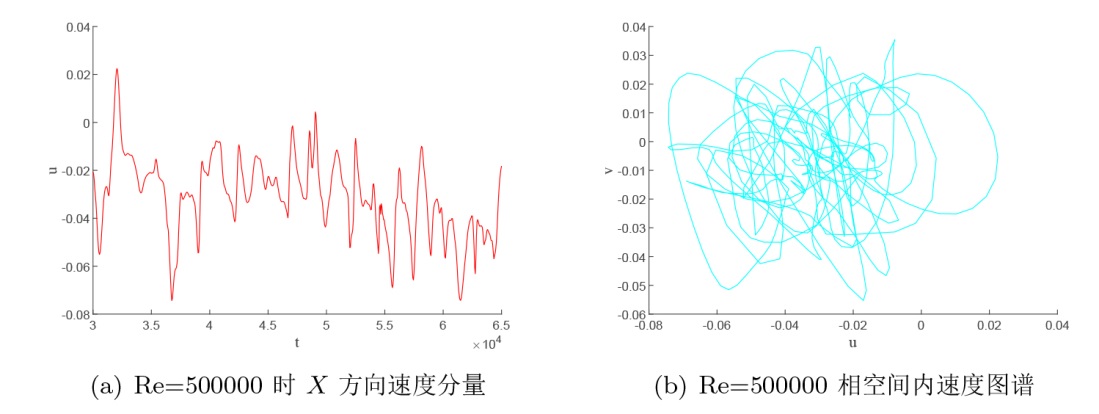
[1]	Hou S, Zou Q, Chen S, et al. Simulation of cavity flow by the lattice Boltzmann method. Journal of Computational Physics, 1994, 118(2): 329-347 doi: 10.1006/jcph.1995.1103
[2]	Feldman Y, Gelfgat A Y. Oscillatory instability of a three-dimensional lid-driven flow in a cube. Physics of Fluids, 2010, 22(9): 093602
[3]	Shan X W. The mathematical structure of the lattices of the lattice Boltzmann method. Journal of Computational Science, 2016, 17(2): 475-481
[4]	Somers J A. Direct simulation of fluid flow with cellular automata and the lattice-Boltzmann equation. Applied Scientific Research, 1993, 51(1/2): 127-133
[5]	张宗宁, 李春光, 董建强. 变系数广义 KdV-Burgers 方程的格子 Boltzmann 模型. 数学物理学报, 2021, 41A(5): 1283-1295
	Zhang Z Z, Li C G, Dong J Q. General Propagation Lattice Boltzmann Model for a Variable-Coefficient Compound KdV-Burgers Equation. Acta Math Sci, 2021, 41A(5): 1283-1295
[6]	Chen S Y, Doolen G D. Lattice Boltzmann method for fluid flows. Annu Rev Fluid Mech, 1998, 30(1): 329-364
[7]	Boghosian B M, Boon J P. Lattice Boltzmann and nonextensive diffusion. Europhysics News, 2005, 36(6): 192-194
[8]	Qian Y H, D'Humieres D, Lallemand P. Lattice BGK Models for the Navier-Stokes Equations. Europhysics Letters, 1992, 17(6): 479-484
[9]	He X, Doolen G. Lattice Boltzmann method on curvilinear coordinates system: Flow around a circular cylinder. Journal of Computational Physics, 1997, 134(2): 306-315
[10]	Sterling J D, Chen S. Stability analysis of lattice Boltzmann methods. Journal of Computational Physics, 1996, 123(1): 196-206
[11]	D'Humieres D. Generalized lattice Boltzmann equations. Rarefied Gas Dynamics-Theory and Simulations, 1994: 450-458
[12]	Luo L S, Liao W, Chen X, et al. Numerics of the lattice Boltzmann method: Effects of collision models on the lattice Boltzmann simulations. Physical Review E, 2011, 83(5): 056710
[13]	Chai Z H, Shi B C. Multiple-relaxation-time lattice Boltzmann method for the Navier-Stokes and nonlinear conveection-diffusion equations: Modeling, analysis and elements. Physical Review E, 2020, 102(2): 023306
[14]	Luo L S, Krafczyk M, Shyy W. Lattice Boltzmann Method for Computational Fluid Dynamics. John Wiley & Sons, Ltd, 2010
[15]	Yu H D, Luo L S, Girimaji S S. LES of turbulent square jet flow using an MRT lattice Boltzmann model. Comput Fluids, 2006, 35: 957-965
[16]	Jing H F, Cai Y J, Wang W H, et al. Investigation of open channel flow with unsubmerged rigid vegetation by the lattice Boltzmann method. Journal of Hydrodynamics, 2020, 4: 771-783
[17]	张巧玲, 景何仿. 三维曲面腔顶盖驱动流的 MRT-LBM 研究. 计算物理, 2022, 39(4): 427-439 doi: 10.19596/j.cnki.1001-246x.8447
	Zhang Q L, Jing H F. Flow patterns in three-dimensional lid-driven cavities with curved boundary : MRT-LBM study. Chinese Journal of Computional Physics, 2022, 39(4): 427-439 doi: 10.19596/j.cnki.1001-246x.8447
[18]	Zhang K, Feng X F, Jing H F, Jiang Y L. An improved MRT-LBM and investigation to the transition and periodicity of 2D lid-driven cavity flow with high Reynolds numbers. Chinese Journal of Physics, 2023, 84: 51-65
[19]	Das S S, Das P K. Fluid flow in a cavity driven by an oscillating lid-A simulation by lattice Boltzmann method. European Journal of Mechanics-B/Fluids, 2013, 39(3): 59-70
[20]	Premnath K N, Pattison M J, Banerjee S. Generalized lattice Boltzmann equation with forcing term for computation of wall-bounded turbulent flows. Physical Review E, 2009, 79(2): 026703
[21]	Cheng Y G, Zhang H. A viscosity counteracting approach in the lattice Boltzmann BGK model for low viscosity flow: Preliminary verification. Computers & Mathematics with Applications, 2011, 61(12): 3690-3702
[22]	Zhang C Z, Cheng Y G, Huang S, et al. Improving the stability of the multiple-relaxation-time lattice Boltzmann method by a viscosity counteracting approach. Advances in Applied Mathematics & Mechanics, 2016, 8(1): 37-51
[23]	Lallemand P, Luo L S. Theory of the lattice Boltzmann method: dispersion, dissipation, isotropy, galilean invariance, and stability. Physical review E, 2000, 61(6): 6546-6562
[24]	Cheng Y G, Li J P. Introducing unsteady non-uniform source terms into the lattice Boltzmann model. International Journal for Numerical Methods in Fluids, 2008, 56(6): 629-641
[25]	Ghia U, Ghia K N, Shin C T. High-Re solutions for incompressible flow using the Navier-Stokes equations and a multigrid method. Journal of Computational Physics, 1982, 48(3): 387-411
[26]	Erturk E, Corke T C, Gokcol C. Numerical solutions of 2-D steady incompressible driven cavity flow at high Reynolds numbers. Int J Numer Meth Fluid, 2005, 48(7): 747-774
[27]	Guo Z L, Zheng C G, Shi B C. Non-equilibrium extrapolation method for velocity and pressure boundary conditions in the lattice Boltzmann method. Chinese Physics, 11(4): 366-374