基于4/2随机波动率模型考虑错误定价和保费退还条款的DC型养老金计划的均衡投资策略

数学物理学报 ›› 2023, Vol. 43 ›› Issue (3): 939-956.

基于4/2随机波动率模型考虑错误定价和保费退还条款的DC型养老金计划的均衡投资策略

卢嘉鑫(),董华^*()

曲阜师范大学统计与数据科学学院山东曲阜 273165

收稿日期:2022-05-12 修回日期:2023-02-12 出版日期:2023-06-26 发布日期:2023-06-01
通讯作者: 董华 E-mail:lujiaxin0928@163.com;sddh1978@126.com
作者简介:卢嘉鑫,E-mail: lujiaxin0928@163.com
基金资助:
国家自然科学基金(12071251);山东省自然科学基金(ZR2020MA035)

Equilibrium Investment Strategy of DC Pension Plan with Mispricing and Return of Premiums Clauses Under the 4/2 Stochastic Volatility Model

Lu Jiaxin(),Dong Hua^*()

School of Statistics and Data Science, Qufu Normal University, Shandong Qufu 273165

Received:2022-05-12 Revised:2023-02-12 Online:2023-06-26 Published:2023-06-01
Contact: Hua Dong E-mail:lujiaxin0928@163.com;sddh1978@126.com
Supported by:
NSFC(12071251);NSF of Shandong Province(ZR2020MA035)

摘要/Abstract

摘要：

该文在均值-方差准则下,引入保费退还条款, 研究了存在错误定价的DC型养老金的时间一致性投资组合问题. 假设养老金计划管理者可以将养老金账户中的财富投资到由无风险资产, 遵循$4/2$随机波动率模型的市场指数和一对错误定价的股票组成的金融市场中. 在博弈论的框架下, 利用随机控制方法, 通过求解广义HJB方程系统分别得到了时间一致的均衡投资策略和均衡有效前沿的显性表达式. 最后, 通过一些数值模拟分析了风险厌恶系数, 错误定价和保费退还条款对均衡策略和有效前沿的影响.

关键词: DC型养老金, 均值-方差准则, $4/2$随机波动率模型, 保费退还, 错误定价, 均衡投资策略

Abstract:

In this paper, we consider a time-consistent investment strategy for DC pension plan with a return of premiums clause and mispricing under the mean-variance criterion. We assume that the pension plan manager is allowed to invest the wealth in the pension account in a financial market consisting of a risk-free asset, a pair of mispriced stocks, and a market index following a $4/2$ stochastic volatility model. Under the framework of game theory, the explicit expressions of the time-consistent equilibrium investment strategy and the equilibrium efficient frontier are obtained by using stochastic control methods and solving the extended HJB system. Finally, the effects of risk aversion coefficient, mispricing and return of premiums clauses on equilibrium strategy and efficient frontier are illustrated by numerical simulations.

Key words: Defined contribution pension plan, Mean-variance criterion, $4/2$ stochastic volatility model, Return of premiums clauses, Mispricing, Equilibrium strategy

中图分类号:

O211.67

卢嘉鑫,董华. 基于4/2随机波动率模型考虑错误定价和保费退还条款的DC型养老金计划的均衡投资策略[J]. 数学物理学报, 2023, 43(3): 939-956.

Lu Jiaxin,Dong Hua. Equilibrium Investment Strategy of DC Pension Plan with Mispricing and Return of Premiums Clauses Under the 4/2 Stochastic Volatility Model[J]. Acta mathematica scientia,Series A, 2023, 43(3): 939-956.

图/表 7

图 1

图 2

图 3

图 4

图 5

图 6

图 7

参考文献 27

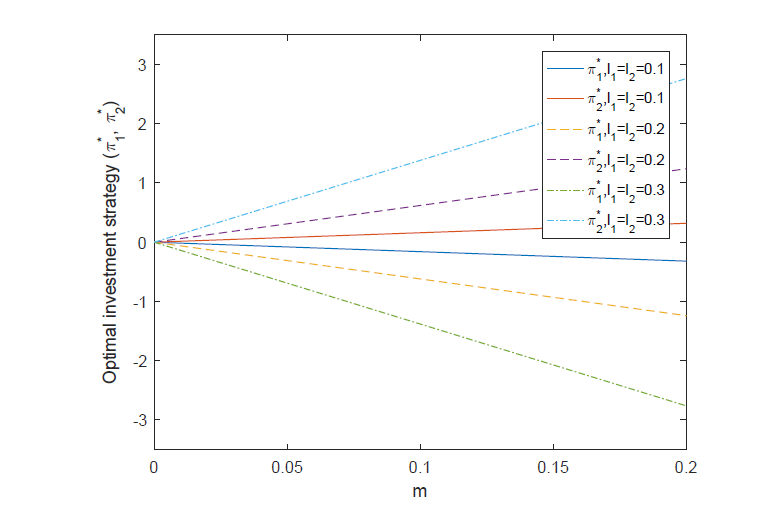
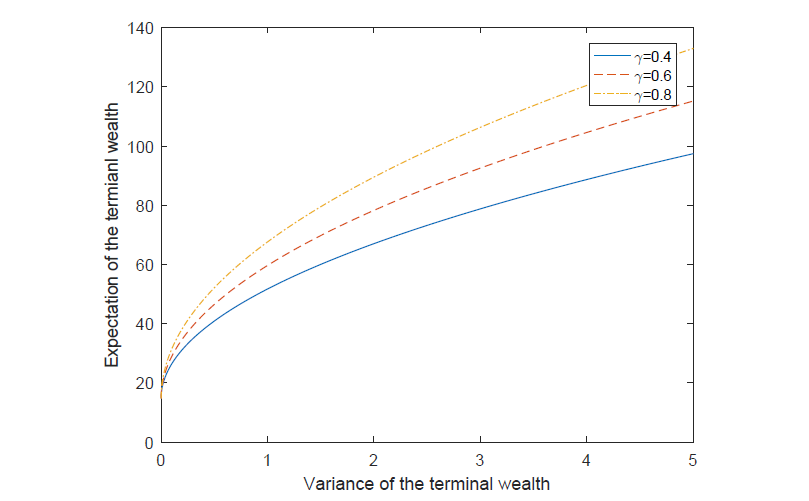
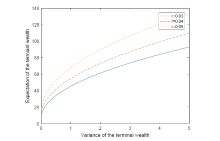
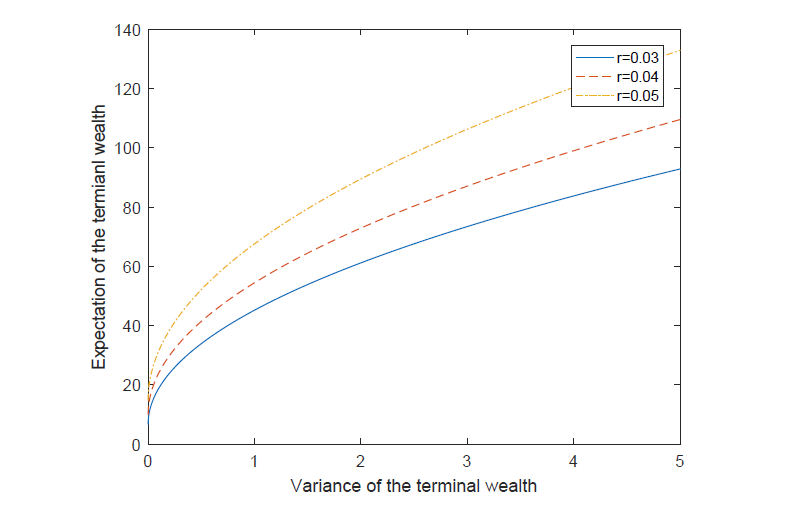
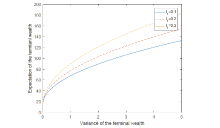
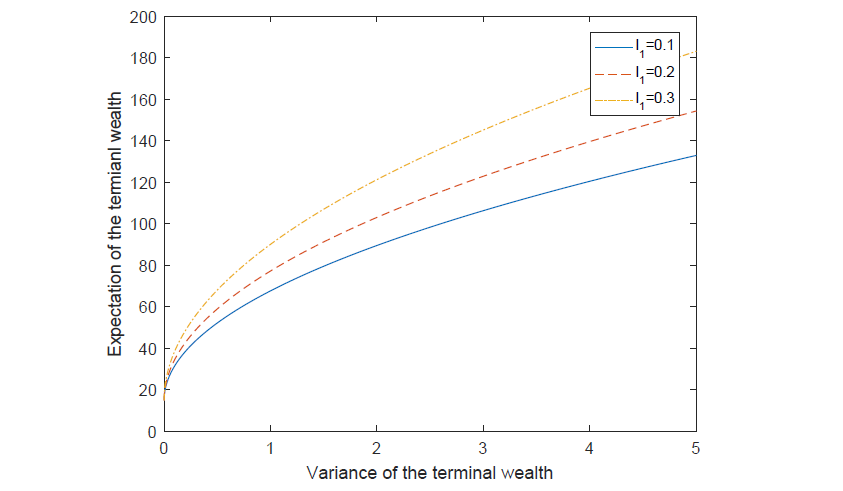
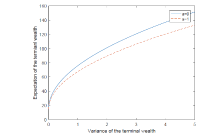
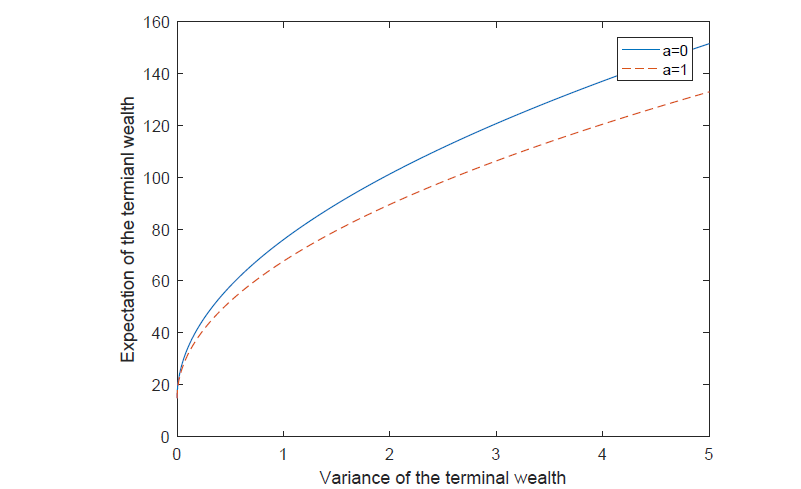
[1]	Bian L, Li Z, Yao H. Pre-commitment and equilibrium investment strategies for the DC pension plan with regime switching and a return of premiums clause. Insurance: Mathematics and Economics, 2018, 81: 78-94 doi: 10.1016/j.insmatheco.2018.05.005
[2]	Björk T, Murgoci A. A general theory of Markovian time inconsistent stochastic control problems. Ssrn Electronic Journal, 2010, 18(3): 545-592
[3]	Chang H, Li J. Robust equilibrium strategy for DC pension plan with the return of premiums clauses in a jump-diffusion model. Optimization, 2021: 1-30
[4]	Cheng Y, Escobar-Anel M. Optimal investment strategy in the family of $4/2$ stochastic volatility models. Quantitative Finance, 2021, 21: 1-29 doi: 10.1080/14697688.2020.1825781
[5]	Grasselli M. The $4/2$ stochastic volatility model: A unified approach for the Heston and the $3/2$ model. Mathematical Finance, 2017, 27(4): 1013-1034 doi: 10.1111/mafi.2017.27.issue-4
[6]	Gu A, Viens F G, Yao H. Optimal robust reinsurance-investment strategies for insurers with mean reversion and mispricing. Insurance: Mathematics and Economics, 2018, 80: 93-109 doi: 10.1016/j.insmatheco.2018.03.004
[7]	Gu A, Viens F G, Yi B. Optimal reinsurance and investment strategies for insurers with mispricing and model ambiguity. Insurance: Mathematics and Economics, 2017, 72: 235-249 doi: 10.1016/j.insmatheco.2016.11.007
[8]	Guan G, Liang Z. Optimal management of DC pension plan in a stochastic interest rate and stochastic volatility framework. Insurance: Mathematics and Economics, 2014, 57: 58-66 doi: 10.1016/j.insmatheco.2014.05.004
[9]	Guan G, Liang Z. Mean-variance efficiency of DC pension plan under stochastic interest rate and mean-reverting returns. Insurance: Mathematics and Economics, 2015, 61: 99-109 doi: 10.1016/j.insmatheco.2014.12.006
[10]	He L, Liang Z X. Optimal investment strategy for the DC plan with the return of premiums clauses in a mean-variance framework. Insurance: Mathematics and Economics, 2013, 53(3): 643-649 doi: 10.1016/j.insmatheco.2013.09.002
[11]	Heston S L. A closed-form solution for options with stochastic volatility with applications to bond and currency options. The Review of Financial Studies, 1993, 6(2): 327-343 doi: 10.1093/rfs/6.2.327
[12]	Heston S L. A simple new formula for options with stochastic volatility. Social Science Electronic Publishing, 1997, 15(4): 23-44
[13]	Kohler P H, Kohler I. Frailty modeling for adult and old age mortality: the application of a modified De Moivre Hazard function to sex differentials in mortality. Demographic Research, 2000, 3(8): 1-32
[14]	Li D, Ng W L. Optimal dynamic portfolio selection: Multi-period mean-variance formulation. Mathematical Finance, 2000, 10(3): 387-406 doi: 10.1111/mafi.2000.10.issue-3
[15]	Li D P, Rong X M, Zhao H, Yi B. Equilibrium investment strategy for DC pension plan with default risk and return of premiums clauses under CEV model. Insurance: Mathematics and Economics, 2017, 72: 6-20 doi: 10.1016/j.insmatheco.2016.10.007
[16]	Liu J, Timmermann A. Optimal convergence trade strategies. The Review of Financial Studies, 2013, 26: 1048-1086 doi: 10.1093/rfs/hhs130
[17]	Liu Z L, Wang Y J, Huang Y, Zhou J M. Optimal portfolios for the DC pension fund with mispricing under the HARA utility framework. Journal of Industrial and Management Optimization, 2023, 19(2): 1262-1281 doi: 10.3934/jimo.2021228
[18]	Ma J, Zhao H, Rong X. Optimal investment strategy for a DC pension plan with mispricing under the Heston model. Communications in Statistics-Theory and Methods, 2020, 49(13): 3168-3183 doi: 10.1080/03610926.2019.1586938
[19]	Markowit H. Portfolio selection. The Journal of Finance, 1952, 7(1): 77-91
[20]	Mudzimbabwe W. A simple numerical solution for an optimal investment strategy for a DC pension plan in a jump diffusion model. Journal of Computational and Applied Mathematics, 2019, 360: 55-61 doi: 10.1016/j.cam.2019.03.043
[21]	Wang Y J, Deng Y C, Huang Y, Zhou J M. Optimal reinsurance-investment policies for insurers with mispricing under mean-variance criterion. Communications in Statistics-Theory and Methods, 2020, 49: 1-28 doi: 10.1080/03610926.2018.1532006
[22]	Wang W Y, Muravey D, Shen Y, Zeng Y. Optimal investment and reinsurance strategies under $4/2$ stochastic volatility model. Scandinavian Actuarial Journal, 2022: 1-37
[23]	Wang P Q, Rong X M, Zhao H, Wang Y J. Robust optimal insurance and investment strategies for the government and the insurance company under mispricing phenomenon. Communications in Statistics-Theory and Methods, 2021, 50: 993-1017 doi: 10.1080/03610926.2019.1646765
[24]	Wang P, Shen Y, Zhang L, Kang Y X. Equilibrium investment strategy for a DC pension plan with learning about stock return predictability. Insurance: Mathematics and Economics, 2021, 100: 384-407 doi: 10.1016/j.insmatheco.2021.07.001
[25]	Yao H X, Lai Y Z, Ma Q H, Jian M J. Asset allocation for a DC pension fund with stochastic income and mortality risk: a multi-period mean-variance framework. Insurance: Mathematics and Economics, 2014, 54(1): 84-92 doi: 10.1016/j.insmatheco.2013.10.016
[26]	Zhang Y. Dynamic optimal mean-variance investment with mispricing in the family of $4/2$ stochastic volatility models. Mathematics, 2021, 9(18): 2293 doi: 10.3390/math9182293
[27]	Zhou X Y, Li D. Continuous-time mean-variance portfolio selection: A stochastic LQ framework. Applied Mathematics and Optimization, 2000, 42(1): 19-33 doi: 10.1007/s002450010003