数学物理学报 ›› 2022, Vol. 42 ›› Issue (1): 139-156.
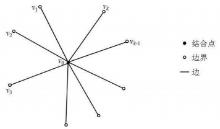
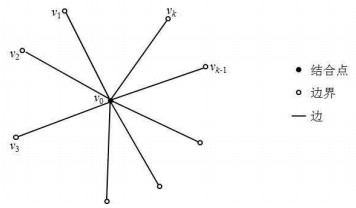
星图上非线性分数阶微分方程边值问题解的存在唯一性
- 1 西北师范大学数学与统计学院 兰州 730070
2 兰州财经大学长青学院 兰州 730022
Existence and Uniqueness of Solutions for the Boundary Value Problems of Nonlinear Fractional Differential Equations on Star Graph
Xiaoling Han1,*( ),Huize Cai2,Hujun Yang1
),Huize Cai2,Hujun Yang1
- 1 College of Mathematics and Statistics, Northwest Normal University, Lanzhou 730070
2 Changqing College, Lanzhou University of Finance and Economics, Lanzhou 730022