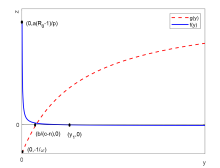
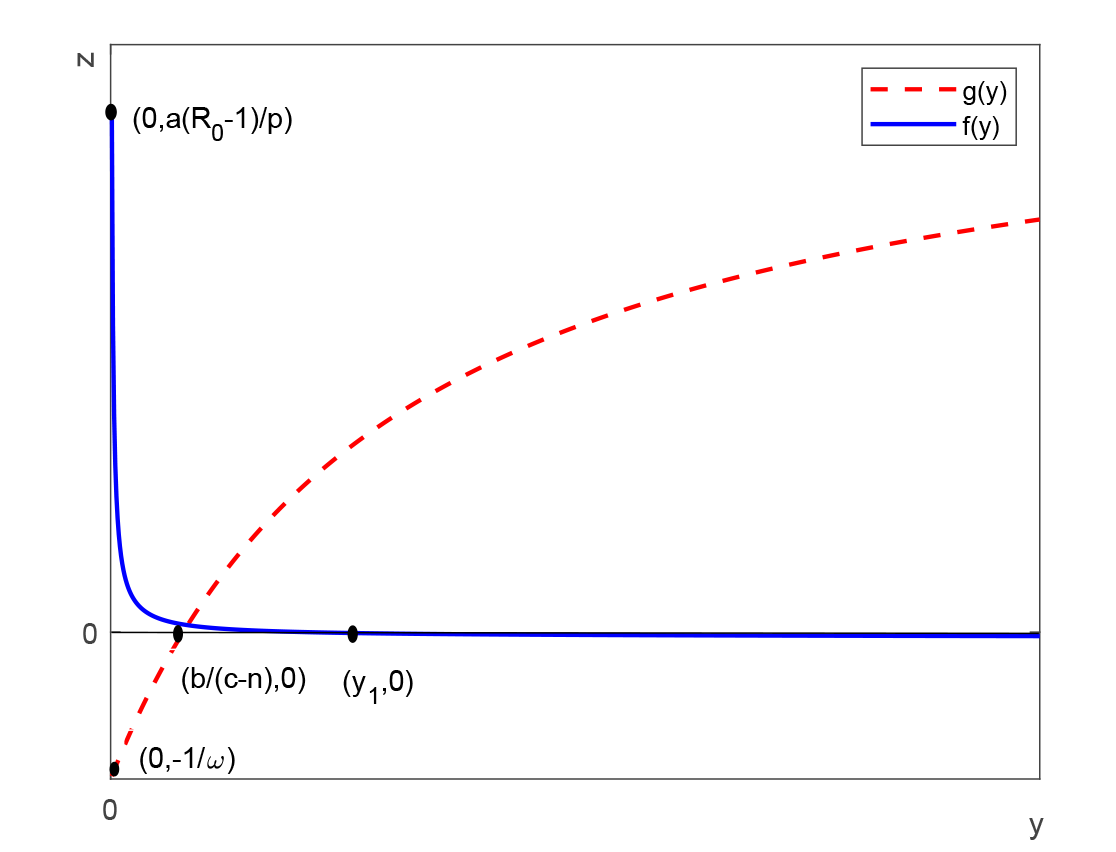
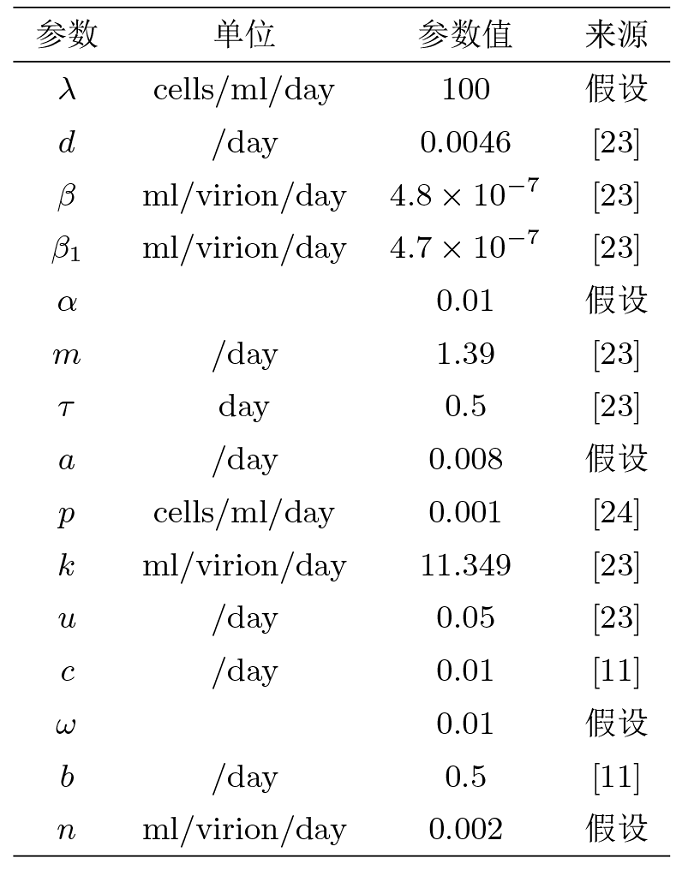
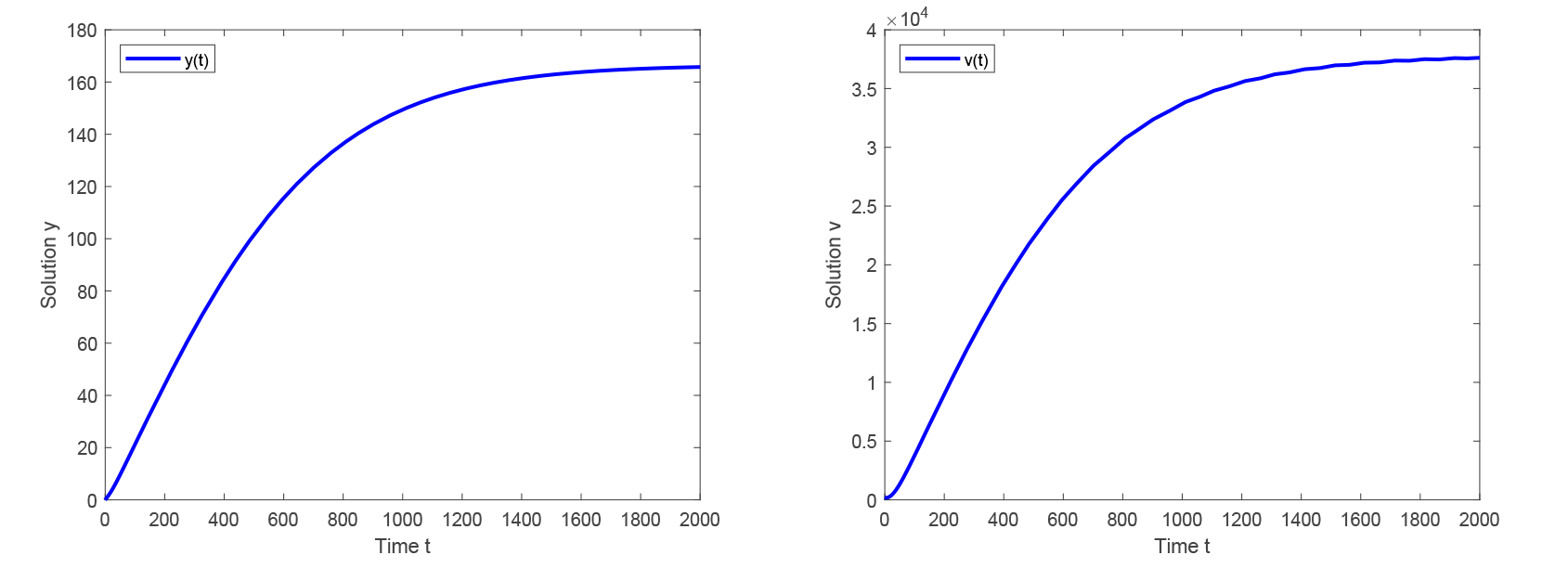
| [1] |
Perelson A S, Neumann A U, Markowitz M, et al. HIV-1 dynamics in vivo: Virion clearance rate, infected cell life-span, and viral generation time. Science, 1996, 271(5255): 1582-1586
pmid: 8599114
|
| [2] |
Egger M, Hirschel B, Francioli P, et al. Impact of new antiretroviral combination therapies in HIV infected patients in Switzerland: Prospective multicentre study. The British Medical Journal, 1997, 315(7117): 1194-1199
|
| [3] |
Perelson A S, Nelson P W. Mathematical analysis of HIV-1: Dynamics in vivo. SIAM Review, 1999, 41(1): 3-44
|
| [4] |
Wasserstein-Robbins F. A mathematical model of HIV infection: Simulating T4, T8, macrophages, antibody, and virus via specific anti-HIV response in the presence of adaptation and tropism. Bulletin of Mathematical Biology, 2010, 72(5): 1208-1253
doi: 10.1007/s11538-009-9488-5
pmid: 20151219
|
| [5] |
Weusten J, Drimmelen H, Lelie P N. Mathematic modeling of the risk of HBV, HCV, and HIV transmission by window phase donations not detected by NAT. Transfusion, 2010, 42(5): 537-548
|
| [6] |
Yan Y, Wang W. Global stability of a five-dimensional model with immune responses and delay. Discrete and Continuous Dynamical Systems-B, 2011, 17(1): 401-416
|
| [7] |
Jiang C, Wang W. Complete classification of global dynamics of a virus model with immune response. Discrete and Continuous Dynamical Systems-B, 2014, 19(4): 1087-1103
|
| [8] |
Willie R, Zheng P, Parumasur N, et al. Asymptotic and stability dynamics of an HIV-1-Cytotoxic T Lymphocytes (CTL) chemotaxis model. Journal of Nonlinear Science, 2020, 30(3): 1055-1080
|
| [9] |
Wang Y, Zhou Y C, Brauer F, et al. Viral dynamics model with CTL immune response incorporating antiretroviral therapy. Journal of Mathematical Biology, 2013, 67(4): 901-934
doi: 10.1007/s00285-012-0580-3
pmid: 22930342
|
| [10] |
Kang C J, Miao H, Chen X, et al. Global stability of a diffusive and delayed virus dynamics model with Crowley-Martin incidence function and CTL immune response. Advances in Difference Equations, 2017, 2017(1): 1-16
|
| [11] |
Ren J, Xu R, Li L C. Global stability of an HIV infection model with saturated CTL immune response and intracellular delay. Mathematical Biosciences and Engineering, 2021, 18(1): 57-68
|
| [12] |
Sattentau Q. Avoiding the void: Cell-to-cell spread of human viruses. Nature Reviews Microbiology, 2008, 6(11): 815-826
doi: 10.1038/nrmicro1972
pmid: 18923409
|
| [13] |
Sattentau Q. The direct passage of animal viruses between cells. Current Opinion in Virology, 2011, 1(5): 396-402
doi: 10.1016/j.coviro.2011.09.004
pmid: 22440841
|
| [14] |
Gummuluru S, Kinsey C M, Emerman M. An in vitro rapid-turnover assay for human immunodeficiency virus type 1 replication selects for cell-to-cell spread of virus. Journal of Virology, 2000, 74(23): 10882-10891
pmid: 11069982
|
| [15] |
Lai X L, Zou X F. Modeling cell-to-cell spread of HIV-1 with logistic target cell growth. Journal of Mathematical Analysis and Applications, 2015, 426(1): 563-584
|
| [16] |
Pourbashash H, Pilyugin S S, Leenheer P D, et al. Global analysis of within host virus models with cell-to-cell viral transmission. Discrete and Continuous Dynamical Systems, 2014, 19(10): 3341-3357
|
| [17] |
Akbari N, Asheghi R. Optimal control of an HIV infection model with logistic growth, celluar and homural immune response, cure rate and cell-to-cell spread. Boundary Value Problems, 2022, Article number 5
|
| [18] |
Iwami S, Nakaoka S, Takeuchi Y, et al. Immune impairment thresholds in HIV infection. Immunology Letters, 2009, 123(2): 149-154
doi: 10.1016/j.imlet.2009.03.007
pmid: 19428563
|
| [19] |
Elaiw A M, Raezah A A, Azoz S A. Stability of delayed HIV dynamics models with two latent reservoirs and immune impairment. Advances in Difference Equations, 2018, Atricle number 414
|
| [20] |
Krishnapriya P, Pitchaimani M. Modeling and bifurcation analysis of a viral infection with time delay and immune impairment. Japan Journal of Industrial and Applied Mathematics, 2017, 34(1): 99-139
|
| [21] |
Hale J, Lunel S V. An Introduction to Functional Differential Equations. Hoboken: Mathematical Methods in the Applied Sciences, 1993
|
| [22] |
Zhao X Q. Basic reproduction ratios for periodic compartmental models with time delay. Journal of Dynamics and Differential Equations, 2017, 29(1): 67-82
|
| [23] |
Wang J L, Guo M, Liu X N, et al. Threshold dynamics of HIV-1 virus model with cell-to-cell transmission, cell-mediated immune responses and distributed delay. Applied Mathematics and Computation, 2016, 291: 149-161
|
| [24] |
Wang J L, Pang J M, Kuniya T, et al. Global threshold dynamics in a five-dimensional virus model with cell-mediated, humoral immune responses and distributed delays. Applied Mathematics and Computation, 2014, 241: 298-316
|
 ),Zhou Kaijuan1,2,3,Bai Ning1,2,3
),Zhou Kaijuan1,2,3,Bai Ning1,2,3