带有Caputo-Fabrizio导数的分数阶微分方程的快速高阶算法的研究

数学物理学报 ›› 2023, Vol. 43 ›› Issue (3): 896-912.

带有Caputo-Fabrizio导数的分数阶微分方程的快速高阶算法的研究

傅博¹,王世宇¹,高婷婷¹,吕学琴^1,^2,^*()

¹哈尔滨师范大学数学科学学院哈尔滨 150025
²天津中德应用技术大学天津 300350

收稿日期:2021-11-09 修回日期:2022-10-08 出版日期:2023-06-26 发布日期:2023-06-01
通讯作者: 吕学琴 E-mail:xueqinhsd@163.com
基金资助:
天津市技术计划项目(21YDTPJC00120);哈尔滨师范大学高等教育教学改革研究重点项目(XJGZ2022010)

A Fast High Order Method for Fractional Differential Equations with the Caputo-Fabrizio Derivative

Fu Bo¹,Wang Shiyu¹,Gao Tingting¹,Lv Xueqin^1,^2,^*()

¹School of Mathematical Sciences, Harbin Normal University, Harbin 150025
²Tianjin Sino-German University of Applied Sciences, Tianjin 300350

Received:2021-11-09 Revised:2022-10-08 Online:2023-06-26 Published:2023-06-01
Contact: Xueqin Lv E-mail:xueqinhsd@163.com
Supported by:
Tianjin Technical Expert Project(21YDTPJC00120);Key Research Project of Higher Education Teaching Reform in Harbin Normal University(XJGZ2022010)

摘要/Abstract

摘要：

对于数值求解常微分方程的传统方法而言, 其计算量和存储量一般都是比较大的, 为了解决这个问题, 该文基于L2方案和一个简单的递归关系提出了一个快速高阶数值方法求解带有Caputo-Fabrizio 导的分数阶微分方程, 该算法在很大程度上减少了存储量和计算量,进一步的, 该文分析了快速算法的可行性、误差估计和稳定性分析, 并通过数值算例论证的所提方法的正确性.

关键词: Caputo-Fabrizio导数, 快速高阶数值算法, L2方案

Abstract:

The computational work and storage of numerically solving the ODEs are generally huge for the traditional direct methods. To overcome this difficulty, we presents a fast high order numerical method for fractional ordinary differential equations with the Caputo-Fabrizio derivative based on a L2 scheme and a simply recurrence relation. The algorithm greatly reduces the storage capacity and the total calculation cost. Furthermore, we also analyzes the feasibility of algorithm, error estimation and stability analysis of the fast scheme. Illustrative examples are included to demonstrate the performance of our technique.

Key words: Caputo-Fabrizio derivative, Fast high order numerical method, L2 scheme

中图分类号:

O241.5

傅博,王世宇,高婷婷,吕学琴. 带有Caputo-Fabrizio导数的分数阶微分方程的快速高阶算法的研究[J]. 数学物理学报, 2023, 43(3): 896-912.

Fu Bo,Wang Shiyu,Gao Tingting,Lv Xueqin. A Fast High Order Method for Fractional Differential Equations with the Caputo-Fabrizio Derivative[J]. Acta mathematica scientia,Series A, 2023, 43(3): 896-912.

图/表 8

表1

表2

表3

图1

图2

图3

表4

表5

参考文献 21

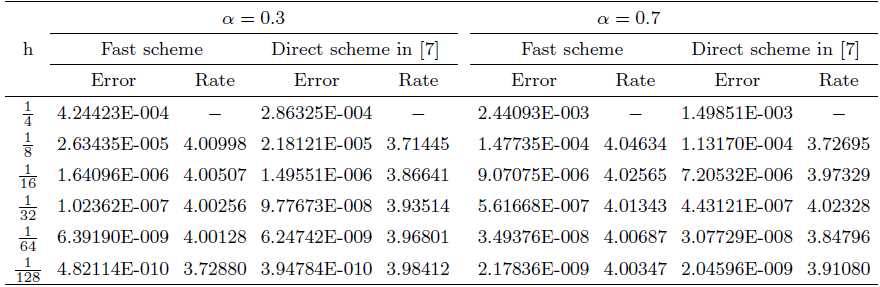
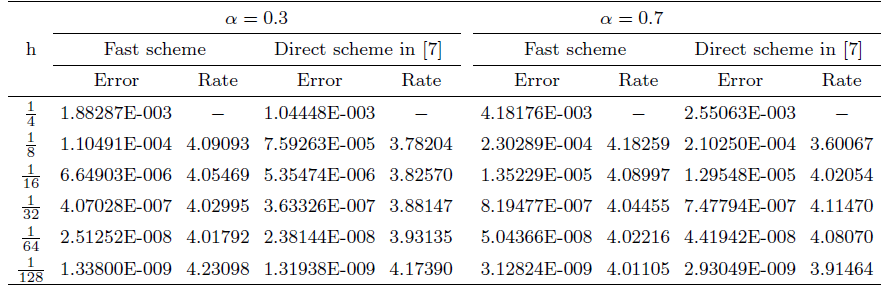
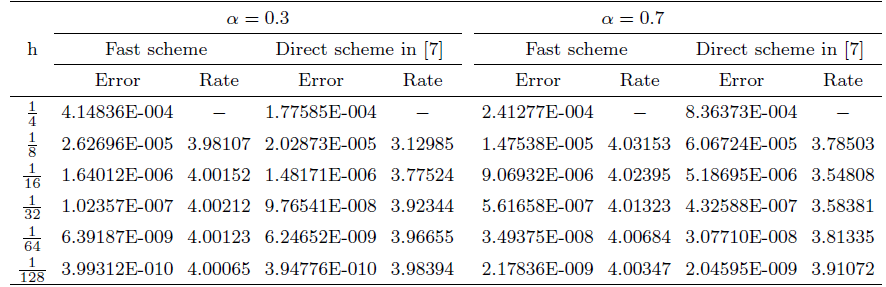
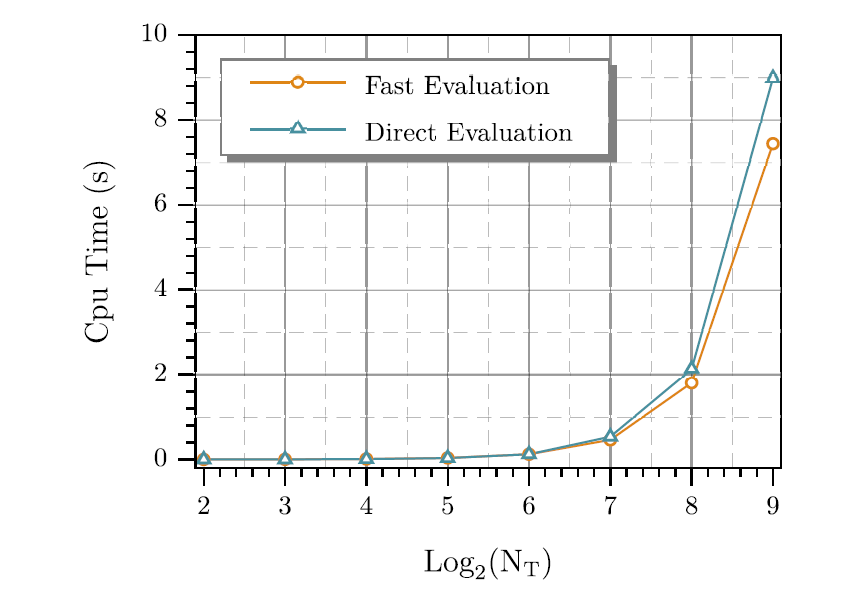
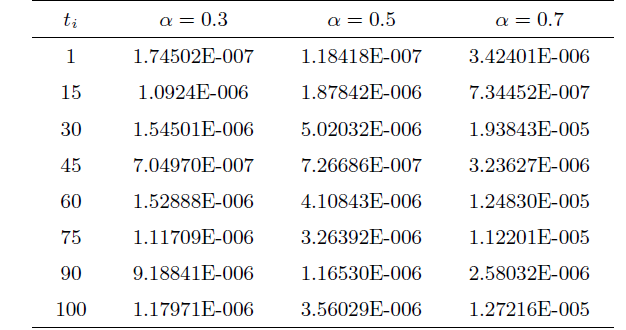
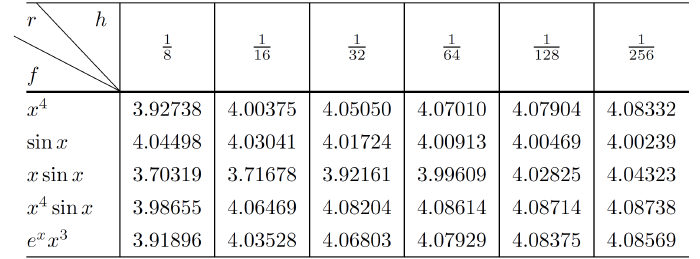
[1]	Oldham K B, Spanier J. The Fractional Calculus. New York: Academic Press, 1974
[2]	Samko S, Kilbas A A, Marichev O. Fractional Integrals and Derivatives: Theory and Applications. Oxfordshire: Taylor & Francis, 1993
[3]	Du M, Wang Z, Hu H. Measuring memory with the order of fractional derivative. Sci Rep, 2013, 3: Article number 3431
[4]	Michele C, Mauro F. A new definition of fractional derivative without singular kernel. Progr Fract Differ Appl, 2015, 1(2): 73-85
[5]	Atangana A, Baleanu D. New fractional derivatives with nonlocal and non-singular kernel: theory and application to heat transfer model. arXiv preprint arXiv:1602.03408, 2016
[6]	Cao J, Xu C. A high order schema for the numerical solution of the fractional ordinary differential equations. J Comput Phys, 2013, 38: 154-168
[7]	Cao J, Wang Z, Xu C. A high-order scheme for fractional ordinary differential equations with the Caputo Fabrizio derivative. Communications on Applied Mathematics and Computation, 2019, 1: 1-21 doi: 10.1007/s42967-019-0010-2
[8]	Yang J, Huang J, Liang D, Tang Y. Numerical solution of fractional diffusion-wave equation based on fractional multistep method. Appl Math Model, 2014, 38(14): 3652-3661 doi: 10.1016/j.apm.2013.11.069
[9]	Sun Z, Wu X. A fully discrete difference scheme for a diffusion-wave system. Appl Numer Math, 2006, 56(2): 193-209 doi: 10.1016/j.apnum.2005.03.003
[10]	Lin Y, Xu C. Finite difference/spectral approximations for the time-fractional diffusion equation. J Comput Phys, 2007, 225(2): 1533-1552 doi: 10.1016/j.jcp.2007.02.001
[11]	Jiang S, Zhang J, Zhang Q, Zhang Z. Fast evaluation of the Caputo fractional derivative and its applications to fractional diffusion equations. Commun Comput Phys, 2017, 21(3): 650-678 doi: 10.4208/cicp.OA-2016-0136
[12]	Huang J, Tang Y, vazquez L. Convergence analysis of a block-by-block method for fractional differential equations. Numer Math Theor Methods Appl, 2012, 5(2): 229-241 doi: 10.4208/nmtma
[13]	Alikhanov A A. A new difference scheme for the time fractional diffusion equation. J Comput Phys, 2015, 280: 424-438 doi: 10.1016/j.jcp.2014.09.031
[14]	Baffet D, Hesthaven J. A kernel compression scheme for fractional differential equations. SIAM J Numer Anal, 2017, 55(2): 496-520 doi: 10.1137/15M1043960
[15]	Baffet D, Hesthaven J. High-order accurate adaptive kernel compression time-stepping schemes for fractional differential equaitons. J Sci Comput, 2017, 72(3): 1169-1195 doi: 10.1007/s10915-017-0393-z
[16]	Deng W H. Finite element method for the space and time fractional Fokker-Planck equation. SIAM J Numer Anal, 2008, 47(1): 204-226 doi: 10.1137/080714130
[17]	Ke R H, Ng M K, Sun H W. A fast direct method for block triangular Toeplitz-like with tri-diagonal block systems from time-fractional partial differential equations. J Comput Phys, 2015, 303: 203-211 doi: 10.1016/j.jcp.2015.09.042
[18]	Liu F, Shen S, Anh V, Turner I. Analysis of a discrete non-Markovian random walk approximation for the time fractional diffusion equation. ANZIAM J, 2005, 46: C488-C504
[19]	McLean W. Fast summation by interval clustering for an evolution equation with memory. J Sci Comput, 2012, 34(6): A3039-A3056
[20]	Hou D, Xu C. A fractional spectral method with applications to some singular problems Adv Comput Math, 2017, 343(5): 911-944
[21]	Stynes M, O'Riordan E, Gracia J. Error analysis of a finite difference method of graded meses for a time-fractional Caputo-Fabrizio derivatives. Eur Phys J C, 2016, 76: 362 doi: 10.1140/epjc/s10052-016-4209-3