西藏自治区包虫病传播的数学建模及动力学分析

摘要/Abstract

摘要：

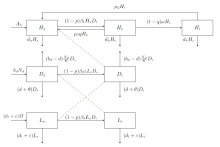
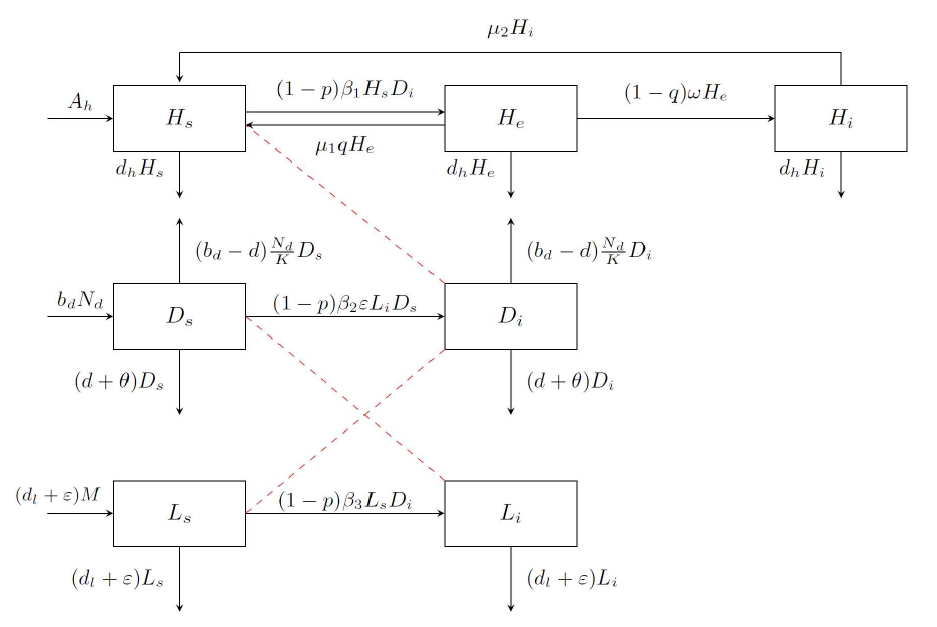
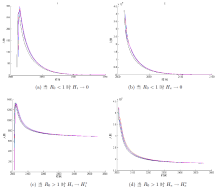
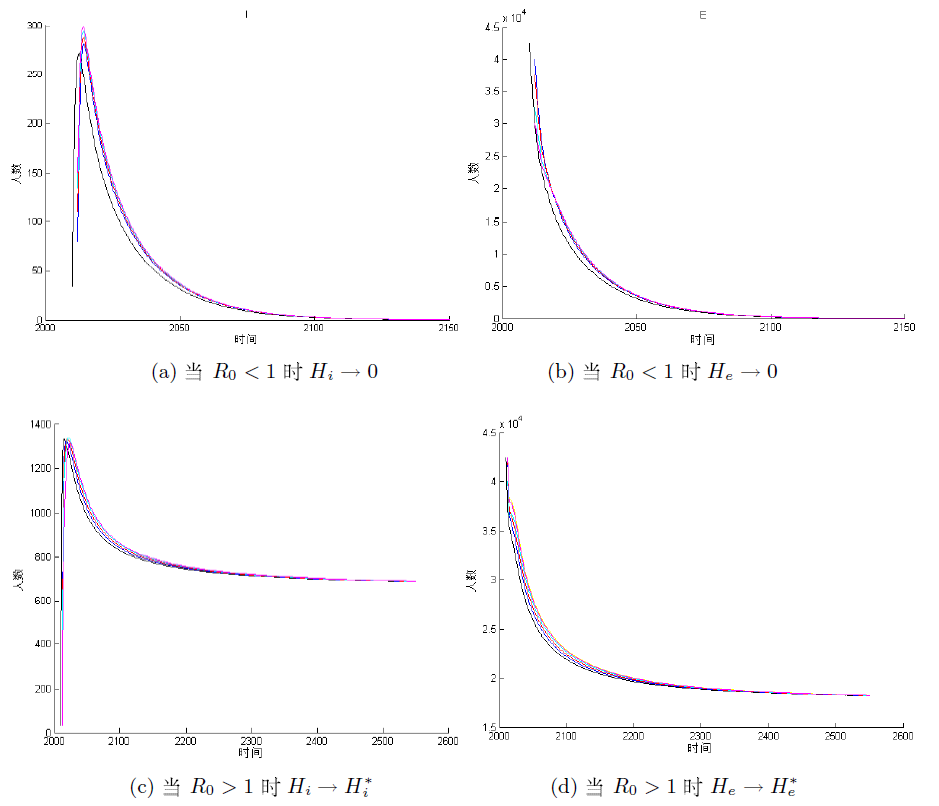
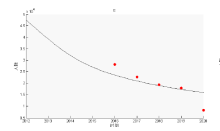
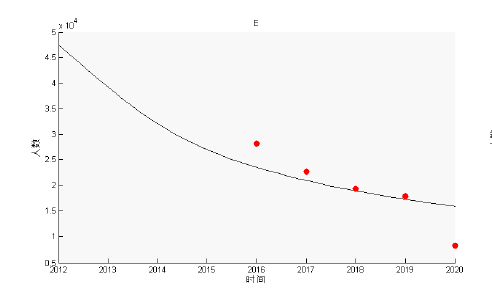
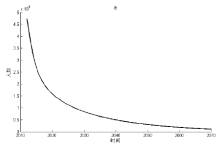
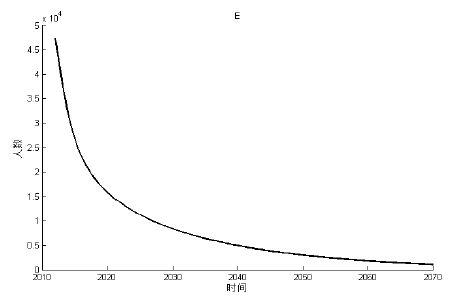
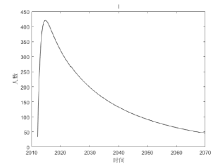
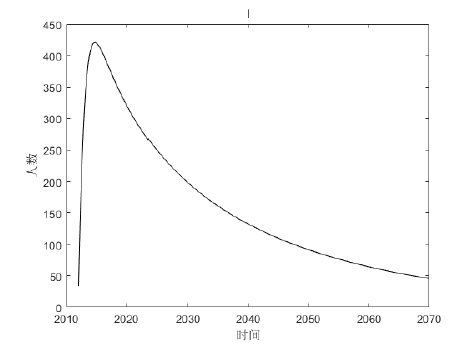
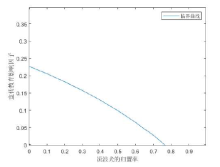
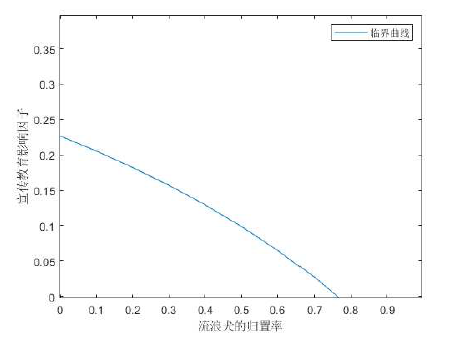
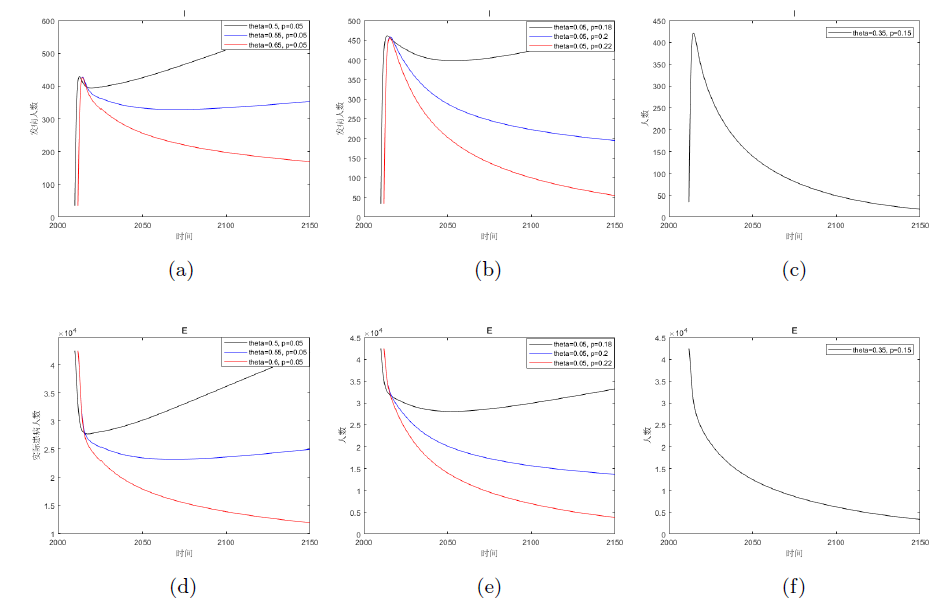
该文通过对包虫病传播机理以及西藏地区包虫病流行现状的研究, 构建了一类符合西藏地区实际情况的包虫病动力学模型. 利用 Lyapunov 函数对模型平衡点进行了稳定性分析, 证明了无病平衡点和地方病平衡点的全局稳定性. 并用收集到的数据, 依据模型对基本再生数 $R_{0}$ 和包虫病流行情况进行了估计和模拟, 结果表明构建的模型符合当地实际传播情况, 具有一定的合理性. 最后针对归置流浪犬和宣传教育两种防治措施给出了合理的建议.

关键词: 包虫病, 动力学模型, 平衡点, 基本再生数

Abstract:

In this paper, by studying the transmission mechanism of echinococcosis and the epidemic status of echinococcosis in Tibet, we constructed a dynamic model of echinococcosis in line with the actual situation in Tibet. The stability of the equilibrium point of the model is analyzed by Lyapunov function, and the global stability of disease-free equilibrium point and endemic equilibrium point is proved. Using the collected data, according to the model, the basic regeneration number $R_{0}$ and the prevalence of echinococcosis were estimated and simulated. The results show that the model is in line with the local actual communication situation and has certain rationality. Finally, reasonable suggestions are given for the prevention and treatment of domesticated stray dogs and publicity and education.

Key words: Echinococcosis, Dynamic model, Equilibrium point, Basic regeneration number

中图分类号:

O175.1

许越, 韩晓玲. 西藏自治区包虫病传播的数学建模及动力学分析[J]. 数学物理学报, 2023, 43(2): 646-656.

Xu Yue, Han Xiaoling. Mathematical Modeling and Dynamic Analysis of Echinococcosis Transmission in Tibet Autonomous Region[J]. Acta mathematica scientia,Series A, 2023, 43(2): 646-656.

图/表 9

图1

图2

图3

西藏地区 2016-2020 年实际患病人数与 $H_{e}$ 的比较"

图3

图4

西藏地区未来 $50$ 年 $H_{e}$ 的变化趋势"

图4

图5

西藏地区未来 $50$ 年 $H_{i}$ 的变化趋势"

图5

表1

表2

图6

$R_{0}=1$ 其中 $p, \theta$ 为参数的临界曲线"

图6

图7

犬的归置率 $\theta$ 与宣传教育因素 $p$ 对疾病流行的影响"

图7

参考文献 18

[1]	Eckert J, Deplazes P. Biological, epidemiological, and clinical aspects of echinococ-cosis, a zoonosis of increasing concern. Clin Microbiol Rev, 2004, 17(1): 107-135 doi: 10.1128/CMR.17.1.107-135.2004
[2]	Davidson R K, Romig T, Jenkins E, et al. The impact of globalisation on the distribution of Echinococcus multilocularis. Trends Parasitol, 2012, 28(6): 239-247 doi: 10.1016/j.pt.2012.03.004 pmid: 22542923
[3]	陈伟奇, 张雅兰, 贡桑曲珍, 等. 西藏自治区棘球蚴病病例分析. 中国寄生虫学与寄生虫病杂志, 2018, 36(1): 43-46
	Chen W Q, Zhang Y L, GongSang Q Z, et al. Analysis of hydatid disease cases in Tibet Autonomous Region. Chinese Journal of Parasitology and Parasitic Diseases, 2018, 36(1): 43-46
[4]	韩帅, 蒉嫣, 薛垂召, 等. 2004-2020年全国棘球蚴病疫情分析. 中国寄生虫学与寄生虫病杂志, 2021, 39(5): 1-6
	Han S, Kuai Y, Xue C Z, et al. The endemic status of echinococcosis in China from 2004 to 2020. Chinese Journal of Parasitology and Parasitic Diseases, 2021, 39(5): 1-6
[5]	蒉嫣, 伍卫平, 韩帅, 等. 2018-2019年全国棘球蚴病监测分析. 中国病原生物学杂志, 2021, 16(9): 1025-1029
	Kuai Y, Wu W P, Han S, et al. Analysis of the results of echinococcosis surveillance in China from 2018 to 2019. Journal of Pathogen Biology, 2021, 16(9): 1025-1029
[6]	Gemmell M A, Lawson J R, Roberts M G. Population dynamics in echinococcosis and cysticercosis: biological parameters of Echinococcus granulosus in dogs and sheep. Parasitology, 1986, 92(3): 599-620 doi: 10.1017/S0031182000065483
[7]	Roberts M G, Lawson J R, Gemmell M A. Population dynamics in echinococcosis and cysticercosis: mathematical model of the life-cycle of Echinococcus granulosus. Parasitology, 1986, 92(3): 621-641 doi: 10.1017/S0031182000065495
[8]	杜守洪, 王蕾, 张学良, 等. 一类具有饱和发生率的包虫病传播模型研究. 数学的实践与认识, 2013, 43(20): 269-273
	Du S H, Wang L, Zhang X L, et al. A Echinococcosis model with saturation incidence. Journal of Mathematics in Practice and Theory, 2013, 43(20): 269-273
[9]	Liu J, Liu L, Feng X, et al. Global dynamics of a time-delayed echinococcosis transmission model. Advances in Difference Equations, 2015, 2015(1): 1-16 doi: 10.1186/s13662-014-0331-4
[10]	Xu Z, Ai C. A spatial echinococcosis transmission model with time delays: stability and traveling waves. International Journal of Biomathematics, 2017, 10(6): 1-32
[11]	赵瑜, 杨诗杰. 一类随机包虫病传播模型及CDC数据拟合. 生物数学学报, 2017, 32(1): 41-48
	Zhao Y, Yang S J. Modeling a stochastic Echinococcosis transmission and fitting the statistical data of CDC. Journal of Biomathematics, 2017, 32(1): 41-48
[12]	Rong X M, Fan M, Sun X D, et al. Impact of disposing stray dogs on risk assessment and control of Echinococcosis in Inner Mongolia. Mathematical Biosciences, 2018, 299: 85-96 doi: S0025-5564(17)30436-4 pmid: 29526551
[13]	Wang K, Zhang X, Jin Z, et al. Modeling and analysis of the transmission of Echinococcosis with application to Xinjiang Uygur Autonomous Region of China. Journal of Theoretical Biology, 2013, 333: 78-90 doi: 10.1016/j.jtbi.2013.04.020 pmid: 23669505
[14]	唐丹丹. 新疆地区包虫病模型的建立与仿真. 乌鲁木齐: 新疆医科大学, 2016
	Tang D D. The Establishment and Simulation of Echinococcosis Model in Xinjiang. Urumqi: Xinjiang Medical University, 2016
[15]	Zhang Y, Xiao Y. Modeling and analyzing the effects of fixed-time intervention on transmission dynamics of echinococcosis in Qinghai province. Mathematical Methods in the Applied Sciences, 2018, 76: 1249-1267
[16]	Dreessche P, Watmough J. Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Mathematical Biosciences, 2002, 180: 29-48 pmid: 12387915
[17]	刘平, 吴海荣, 张伟超, 等. 我国西部地区农牧民包虫病防控认知情况及其影响因素. 中国动物检疫, 2021, 38(5): 1-4
	Liu P, Wu H R, Zhang W C, et al. Awareness of herdsmen for the prevention and control of echinococcosis and potential risk factors in western china. China Animal Health Inspection, 2021, 38(5): 1-4
[18]	马知恩, 周义仓, 王稳地, 靳帧. 传染病动力学的数学建模与研究. 北京: 科学出版社, 2004
	Ma Z E, Zhou Y C, Wang W D, Jin Z. Mathematical Modeling and Research on the Dynamics of Infectious Diseases. Beijing: Science Press, 2004

Metrics

Viewed

Full text

128

HTML			PDF

Just accepted	Online first	Issue	Just accepted	Online first	Issue
0	0	2	0	0	126

From	Others	local

Times	1	127
Rate	1%	99%

Abstract

Just accepted	Online first	Issue

0	0	82

From	Others	local

Times	80	2
Rate	98%	2%

Cited

Web of Science	Crossref	ScienceDirect	Search for Citations in Google Scholar >>


This page requires you have already subscribed to WoS.

Shared

Discussed