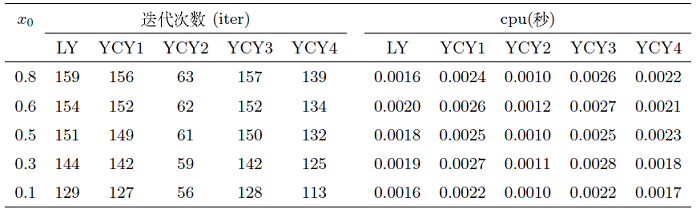
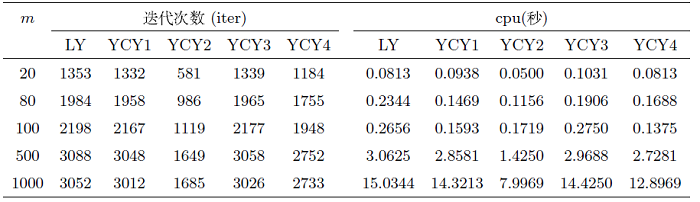
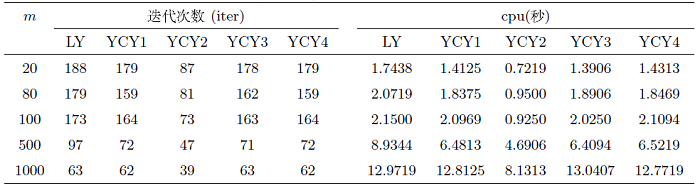
| [1] |
Cottle R W, Yao J C. Pseudo-monotone complementarity problems in Hilbert space. J Optim Theory Appl, 1992, 75: 281-295
doi: 10.1007/BF00941468
|
| [2] |
Goldstein A A. Convex programming in Hilbert space. Bull Amer Math Soc, 1964, 70(5): 709-710
doi: 10.1090/bull/1964-70-05
|
| [3] |
Korpelevich G M. An extragradient method for finding saddle points and other problems. Ekonomika i Mat Metody, 1976, 12: 747-756
|
| [4] |
Antipin A S. On a method for convex programs using asymmetrical modification of the Lagrange function. Ekonomika i Mat Metody, 1976, 12(6): 1164-1173
|
| [5] |
Tseng P. A modified forward-backward splitting method for maximal monotone mappings. SIAM J Control Optim, 2000, 38: 431-446
doi: 10.1137/S0363012998338806
|
| [6] |
Censor Y, Gibali A, Reich S. The subgradient extragradient method for solving variational inequalities in Hilbert Space. J Optim Theory Appl, 2011, 148: 318-335
doi: 10.1007/s10957-010-9757-3
|
| [7] |
Vuong P T. On the weak convergence of the extragradient method for solving pseudo-monotone variational Inequalities. J Optim Theory Appl, 2018, 176: 399-409
doi: 10.1007/s10957-017-1214-0
|
| [8] |
陈艺, 叶明露. 求解伪单调变分不等式的修正投影收缩算法. 西华师范大学学报(自然科学版), 2021, 42(3): 246-253
|
|
Chen Y, Ye M L. Modified projection and contraction algorithm for solving pseudomonotone variational inequality problems. Journal of China West Normal University(Natural Sciences), 2021, 42(3): 246-253
|
| [9] |
杨静, 龙宪军. 关于伪单调变分不等式与不动点问题的新投影算法. 数学物理学报, 2022, 42A(3): 904-919
|
|
Yang J, Long X J. A new projection algorithm for solving pseudo-monotone variational inequality and fixed point problems. Acta Mathematica Scientia, 2022, 42A(3): 904-919
|
| [10] |
万升联. 解变分不等式的一种二次投影算法. 数学物理学报, 2021, 41A(1): 237-244
|
|
Wan S L. A double projection algorithm for solving variational inequalities. Acta Mathematica Scientia, 2021, 41A(1): 237-244
|
| [11] |
胡雨贤. 求解变分不等式的一种双投影算法. 数学物理学报, 2019, 39A(6): 1492-1498
|
|
Hu Y X. A double projection method for solving variational inequalities. Acta Mathematica Scientia, 2019, 39A(6): 1492-1498
|
| [12] |
Ye M L, He Y R. A double projection method for solving variational inequalities without monotonicity. Comput Optim and Appl, 2015, 60(1): 141-150
doi: 10.1007/s10589-014-9659-7
|
| [13] |
He Y R. Solvability of the minty variational inequality. J Optim Theory Appl, 2017, 174(3): 686-692
doi: 10.1007/s10957-017-1124-1
|
| [14] |
Liu H W, Yang J. Weak convergence of iterative methods for solving quasimonotone variational inequalities. Comput Optim and Appl, 2020, 77(2): 491-508
doi: 10.1007/s10589-020-00217-8
|
| [15] |
Ye M L. An infeasible projection type algorithm for nonmonotone variational inequalities. Numer Algor, 2022: 89(4), 1723-1742
doi: 10.1007/s11075-021-01170-1
|
| [16] |
Polyak B T. Some methods of speeding up the convergence of iteration methods. USSR Comput Math Math Phys, 1964: 4(5): 1-17
|
| [17] |
Wang Z B, Chen X, Yi J, et al. Inertial projection and contraction algorithms with larger step sizes for solving quasimonotone variational inequalities. J Global Optim, 2022, 82(3): 499-522
doi: 10.1007/s10898-021-01083-2
|
| [18] |
Thong D V, Vinh N T, Cho Y J. New strong convergence theorem of the inertial projection and contraction method for variational inequality problems. Comput Optim and Appl, 2020, 84(1): 285-305
|
| [19] |
杨蓝翔, 叶明露. 一类伪单调变分不等式与不动点问题的自适应惯性投影算法. 西华师范大学学报(自然科学版), 1-13[2022-11-16]. http://kns.cnki.net/kcms/detail/51.1699.N.20220927.1812.007.html
|
|
Yang L X, Ye M L. A Self-adaptive inertial projection algorithm for a class of pseudomonotone variational inequalities and fixed-point problems. Journal of China West Normal University(Natural Sciences), 1-13[2022-11-16]. http://kns.cnki.net/kcms/detail/51.1699.N.20220927.1812.007.html
|
| [20] |
Chen J X, Ye M L. A new inertial two-subgradient extragradient algorithm for variational inequality problems. Adv Math (China), 2022, 51(1): 165-182
|
| [21] |
Shehu Y, Iyiola O S. Projection methods with alternating inertial steps for variational inequalities: Weak and linear convergence. Appl Numer Math, 2020, 157: 315-337
doi: 10.1016/j.apnum.2020.06.009
|
| [22] |
Goebel K, Rech S. Uniform Convexity, Hyperbolic Geometry, and Nonexpansive Mappings. New York: Marcel Dekker, 1984
|
| [23] |
Bauschke H H, Combettes P L. Convex Analysis and Monotone Operator Theory in Hilbert Spaces. Berlin: Springer, 2017
|
| [24] |
Alvarez F. Weak convergence of a relaxed and inertial hybrid projection-proximal point algorithm for maximal monotone operators in Hilbert Space. SIAM J Optimiz, 2004, 14(3): 773-782
doi: 10.1137/S1052623403427859
|
| [25] |
Dang H, Pham K A, Le D M. Modified hybrid projection methods for finding common solutions to variational inequality problems. Comput Optim and Appl, 2017, 66(1): 75-96
doi: 10.1007/s10589-016-9857-6
|
 ),Chen Yi(
),Chen Yi( ),Ye Minglu(
),Ye Minglu( )
)