数学物理学报 ›› 2023, Vol. 43 ›› Issue (1): 249-260.
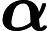
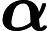
- 1安徽工程大学数理与金融学院 安徽芜湖241000
2东华大学理学院 上海201620
-
收稿日期:2021-04-22修回日期:2022-07-05出版日期:2023-02-26发布日期:2023-03-07 -
通讯作者:*张雪康, E-mail:xkzhang@ahpu.edu.cn -
作者简介:万山林, E-mail:2221020107@stu.ahpu.edu.cn |舒慧生, E-mail:hsshu@dhu.edu.cn -
基金资助:国家自然科学基金(12101004);国家自然科学基金(62073071);国家自然科学基金(12271003);安徽工程大学引进人才科研启动基金(2020YQQ064);安徽省高端装备智能控制国际联合研究中心开放基金(IRICHE-05)
Parameter Estimation for Nonlinear Stochastic Differential Equations Driven by 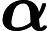
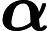
Zhang Xuekang1,*( ),Wan Shanlin1(
),Wan Shanlin1( ),Shu Huisheng2(
),Shu Huisheng2( )
)
- 1School of Mathematics-Physics and Finance, Anhui Polytechnic University, Anhui Wuhu 241000
2College of Science, Donghua University, Shanghai 201620
-
Received:2021-04-22Revised:2022-07-05Online:2023-02-26Published:2023-03-07 -
Supported by:The NSFC(12101004);The NSFC(62073071);The NSFC(12271003);Startup Foundation for Introducing Talent of Anhui Polytechnic University(2020YQQ064);Open Project of Anhui Province Center for International Reasearch of Intelligent Control of High-end Equipment(IRICHE-05)
摘要:
该文研究了基于连续时间状态观测的 
中图分类号:
- O211
引用本文
张雪康, 万山林, 舒慧生. 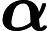
Zhang Xuekang, Wan Shanlin, Shu Huisheng. Parameter Estimation for Nonlinear Stochastic Differential Equations Driven by 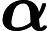
使用本文
| [1] | Applebaum D. Lévy Processes and Stochastic Calculus. United Kingdom: Cambridge University Press, 2009 |
| [2] | Basawa I, Scott D. Asymptotic Optimal inference for Non-ergodic Models. New York: Springer, 1983 |
| [3] |
Bercu B, ProÏa F, Savy N. On Ornstein-Uhlenbeck driven by Ornstein-Uhlenbeck processes. Statist Probab Lett, 2014, 85: 36-44
doi: 10.1016/j.spl.2013.11.002 |
| [4] |
Bo L, Wang Y, Yang X, Zhang G. Maximum likelihood estimation for reflected Ornstein-Uhlenbeck processes. J Statist Plann Inference, 2011, 141: 588-596
doi: 10.1016/j.jspi.2010.07.001 |
| [5] |
Dietz H. Asymptotic behavior of trajectory fitting estimators for certain non-ergodic SDE. Stat Inference Stoch Process, 2001, 4: 249-258
doi: 10.1023/A:1012254332474 |
| [6] | Dietz H, Kutoyants Y. A class of minimum-distance estimators for diffusion processes with ergodic properties. Stat Decis, 1997, 15: 211-227 |
| [7] | Dietz H, Kutoyants Y. Parameter estimation for some non-recurrent solutions of SDE. Stat Decis, 2003, 21: 29-45 |
| [8] |
Fama E. Mandelbrot and the stable Paretian hypothesis. J Business, 1963, 36: 420-429
doi: 10.1086/294633 |
| [9] |
Hu Y, Long H. Parameter estimation for Ornstein-Uhlenbeck processes driven by  |
| [10] |
Hu Y, Long H. Least squares estimator for Ornstein-Uhlenbeck processes driven by  doi: 10.1016/j.spa.2008.12.006 |
| [11] |
Hu Y, Long H. On the singularity of least squares estimator for mean-reverting  |
| [12] |
Janicki A, Weron A. Simulation and Chaotic Behavior of  |
| [13] | Jeong H, Tomber B, Albert R, et al. The large-scale organization of metabolic networks. Nature, 2000, 407: 378-382 |
| [14] |
Jiang H, Dong X. Parameter estimation for the non-stationary Ornstein-Uhlenbeck process with linear drift. Stat Papers, 2015, 56: 257-268
doi: 10.1007/s00362-014-0580-z |
| [15] |
Kessler M. Estimation of an ergodic diffusion from discrete observations. Scand J Statist, 1997, 24: 211-229
doi: 10.1111/1467-9469.00059 |
| [16] | Kutoyants Y. Statistical Inference for Ergodic Diffusion Processes. Heidelberg: Springer-Verlag, 2004 |
| [17] | Long H. Parameter estimation for a class of stochastic differential equations driven by small stable noises from discrete observations. Acta Math Sci, 2010, 30B(3): 645-663 |
| [18] |
Mandelbrot B. The Pareto-Lévy law and the distribution of income. Int Econ Rev, 1960, 1: 79-106
doi: 10.2307/2525289 |
| [19] |
Pan Y, Yan L. The least squares estimation for the  doi: 10.1007/s11009-018-9654-z |
| [20] | Sato K. Lévy Processes and Infinitely Divisible Distributions. Cambridge: Cambridge University Press, 1999 |
| [21] |
Shimizu Y. Local asymptotic mixed normality for discretely observed non-recurrent Ornstein-Uhlenbeck processes. Ann Inst Stat Math, 2012, 64: 193-211
doi: 10.1007/s10463-010-0307-4 |
| [22] |
Xu W, Wu C, Dong Y, Xiao W. Modeling Chinese stock returns with stable distribution. Math Comput Model, 2011, 54: 610-617
doi: 10.1016/j.mcm.2011.03.004 |
| [23] |
Zang Q, Zhang L. Asymptotic behaviour of the trajectory fitting estimator for reflected Ornstein-Uhlenbeck Processes. J Theor Probab, 2019, 32: 183-201
doi: 10.1007/s10959-017-0796-7 |
| [24] |
Zang Q, Zhu C. Asymptotic behaviour of parametric estimation for nonstationary reflected Ornstein-Uhlenbeck processes. J Math Anal Appl, 2016, 444: 839-851
doi: 10.1016/j.jmaa.2016.06.067 |
| [25] |
Zhang S, Zhang X. A least squares estimator for discretely observed Ornstein-Uhlenbeck processes driven by symmetric  doi: 10.1007/s10463-012-0362-0 |
| [26] |
Zhang X, Yi H, Shu H. Nonparametric estimation of the trend for stochastic differential equations driven by small  doi: 10.1016/j.spl.2019.03.012 |
| [27] |
Zhang X, Yi H, Shu H. Parameter estimation for non-stationary reflected Ornstein-Uhlenbeck processes driven by  doi: 10.1016/j.spl.2019.108617 |
| [28] |
Zhang X, Yi H, Shu H. Parameter estimation for certain nonstationary processes driven by  doi: 10.1080/03610926.2019.1630436 |
| [29] | Zolotarev V. One-Dimensional Stable Distribution. Providence: American Mathematical Society, 1986 |
| [1] | 费晨,费为银. 分布不确定下随机微分方程参数最小二乘估计[J]. 数学物理学报, 2019, 39(6): 1499-1513. |
| [2] | 王继霞, 肖庆宪. 非时齐扩散模型中扩散系数的局部估计[J]. 数学物理学报, 2015, 35(2): 332-344. |
| [3] | 陶宝. 负极值指标估计量的渐近性质[J]. 数学物理学报, 2014, 34(3): 611-618. |
| [4] | 邱瑾, 陆传荣. 一类统计量的乘积的渐近性质和几乎处处中心极限定理[J]. 数学物理学报, 2013, 33(3): 475-482. |
| [5] | 王成勇. 半参数平滑转换回归模型及其级数估计[J]. 数学物理学报, 2012, 32(4): 797-807. |
| [6] | 王宏宁, 俞焕然. 基于相依数据的非参数回归函数和尺度函数的联立稳健估计[J]. 数学物理学报, 2010, 30(3): 835-847. |
| [7] | 张军舰, 李国英. 上界型拟合优度检验[J]. 数学物理学报, 2010, 30(2): 344-357. |
| [8] | 张娟, 崔恒建. 缺失数据下EV模型的调整最小二乘估计[J]. 数学物理学报, 2009, 29(6): 1465-1476. |
| [9] | 尹长明, 李永明, 王朋炎. 广义线性模型中极大拟似然估计的强收敛速度[J]. 数学物理学报, 2009, 29(4): 1058-1064. |
| [10] | 李莉娜, 高付清. 方向数据核密度估计强一致相合性的收敛速度[J]. 数学物理学报, 2009, 29(3): 707-715. |
| [11] | 肖枝洪;刘禄勤. 不完全信息随机截尾广义线性模型的极大似然估计[J]. 数学物理学报, 2008, 28(3): 553-564. |
| [12] | 李国亮; 刘禄勤. 误差为鞅差序列的部分线性模型中估计的强相合性[J]. 数学物理学报, 2007, 27(5): 788-801. |
| [13] | 夏天; 唐年胜; 王学仁; 张文专. 非线性再生散度随机效应模型的渐近性质[J]. 数学物理学报, 2007, 27(1): 146-154. |
| [14] | 丁洁丽;陈希孺. 广义线性回归极大似然估计的强相合性[J]. 数学物理学报, 2006, 26(2): 168-173. |
| [15] | 吴群英. 混合线性模型M估计的强相合性[J]. 数学物理学报, 2005, 25(1): 41-46. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 100
|
|
|||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 64
|
|
|||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||
|