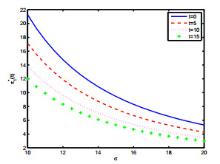
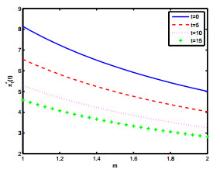
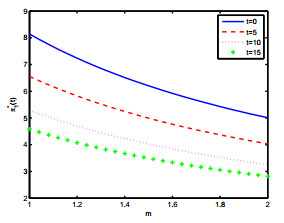
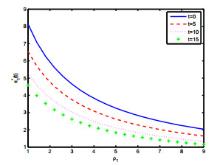
数学物理学报 ›› 2022, Vol. 42 ›› Issue (3): 943-960.
• 论文 • 上一篇
违约风险下目标收益型养老金计划的α-鲁棒最优投资策略
- 曲阜师范大学统计与数据科学学院 山东曲阜 273165
-
收稿日期:2021-08-23出版日期:2022-06-26发布日期:2022-05-09 -
通讯作者:赵永霞 E-mail:sy2syy@163.com;yongxiazhao@163.com -
作者简介:石媛, E-mail:sy2syy@163.com -
基金资助:国家自然科学基金(11501321);山东省自然科学基金(ZR2020MA035)
α-Robust Optimal Investment Strategy for Target Benefit Pension Plans Under Default Risk
- School of Statistics and Data Science, Qufu Normal University, Shandong Qufu 273165
-
Received:2021-08-23Online:2022-06-26Published:2022-05-09 -
Contact:Yongxia Zhao E-mail:sy2syy@163.com;yongxiazhao@163.com -
Supported by:the NSFC(11501321);the NSF of Shandong Province(ZR2020MA035)
摘要:
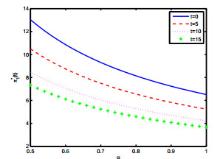
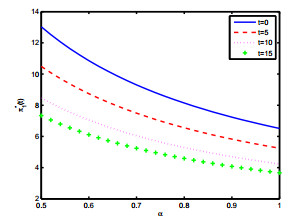
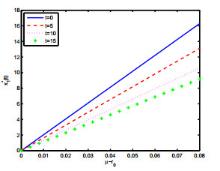
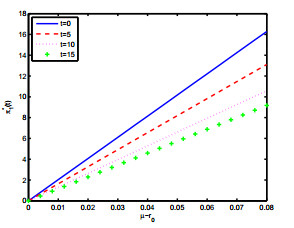
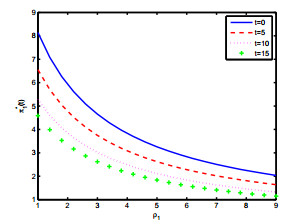
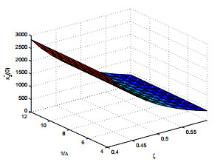
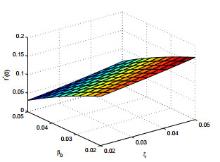
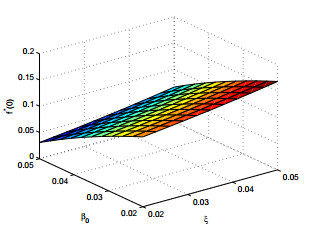
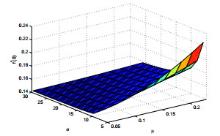
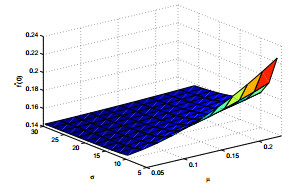
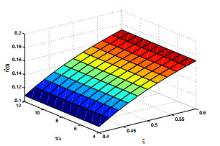
该文研究具有违约风险和模型不确定性的目标收益养老金计划的最优投资和收益支付问题.假定养老基金投资于无风险资产、可违约债券和股票,其中股票价格服从常弹性方差(CEV)模型.养老金的支付取决于计划的财务状况,且风险由不同代人分担.同时为保障退休之前发生死亡的养老金持有者权益,在模型中加入保费返还条款.此外,该文的模型允许养老金管理者有不同程度的模糊厌恶,而不是只考虑极端的模糊厌恶.应用随机控制方法,分别建立违约后和违约前两种情况下的Hamilton-Jacobi-Bellman方程,推导出α-鲁棒的最优投资策略和最优福利调整策略的闭型解.最后数值分析说明了金融市场参数对最优控制问题的影响.
中图分类号:
- O211.6
引用本文
石媛,赵永霞. 违约风险下目标收益型养老金计划的α-鲁棒最优投资策略[J]. 数学物理学报, 2022, 42(3): 943-960.
Yuan Shi,Yongxia Zhao. α-Robust Optimal Investment Strategy for Target Benefit Pension Plans Under Default Risk[J]. Acta mathematica scientia,Series A, 2022, 42(3): 943-960.
使用本文
| 1 |
Guan G H , Liang Z X . Optimal management of DC pension plan under loss aversion and Value-at-Risk constraints. Insurance: Mathematics and Economics, 2016, 69, 224- 237
doi: 10.1016/j.insmatheco.2016.05.014 |
| 2 |
Chen Z , Li Z F , Zeng Y , Sun J Y . Asset allocation under loss aversion and minimum performance constraint in a DC pension plan with inflation risk. Insurance: Mathematics and Economics, 2017, 75, 137- 150
doi: 10.1016/j.insmatheco.2017.05.009 |
| 3 | Wang S X , Rong X M , Zhao H . Optimal investment and benefit payment strategy under loss aversion for target benefit pension plans. Applied Mathematics and Computation, 2019, 346, 205- 218 |
| 4 |
He L , Liang Z X , Yuan F Y . Optimal DB-PAYGO pension management towards a habitual contribution rate. Insurance: Mathematics and Economics, 2020, 94, 125- 141
doi: 10.1016/j.insmatheco.2020.07.005 |
| 5 |
Gao J W . Optimal portfolios for DC pension plans under a CEV model. Insurance: Mathematics and Economics, 2009, 44, 479- 490
doi: 10.1016/j.insmatheco.2009.01.005 |
| 6 |
Li D P , Rong X M , Zhao H , Yi B . Equilibrium investment strategy for DC pension plan with default risk and return of premiums clauses under CEV model. Insurance: Mathematics and Economics, 2017, 72, 6- 20
doi: 10.1016/j.insmatheco.2016.10.007 |
| 7 |
Wang S X , Lu Y , Sanders B . Optimal investment strategies and intergenerational risk sharing for target benefit pension plans. Insurance: Mathematics and Economics, 2018, 80, 1- 14
doi: 10.1016/j.insmatheco.2018.02.003 |
| 8 |
Wang S X , Lu Y . Optimal investment strategies and risk-sharing arrangements for a hybrid pension plan. Insurance: Mathematics and Economics, 2019, 89, 46- 62
doi: 10.1016/j.insmatheco.2019.09.005 |
| 9 |
Wang P Q , Rong X M , Zhao H , Wang S X . Robust optimal investment and benefit payment adjustment strategy for target benefit pension plans under default risk. Journal of Computational and Applied Mathematics, 2021, 391, 113382
doi: 10.1016/j.cam.2021.113382 |
| 10 |
Wang P , Li Z F . Robust optimal investment strategy for an AAM of DC pension plans with stochastic interest rate and stochastic volatility. Insurance: Mathematics and Economics, 2018, 80, 67- 83
doi: 10.1016/j.insmatheco.2018.03.003 |
| 11 |
Zeng Y , Li D P , Chen Z , Yang Z . Ambiguity aversion and optimal derivative-based pension investment with stochastic income and volatility. Journal of Economic Dynamics and Control, 2018, 88, 70- 103
doi: 10.1016/j.jedc.2018.01.023 |
| 12 |
Heath C , Tversky A . Preference and belief: ambiguity and competence in choice under uncertainty. Journal of Risk and Uncertainty, 1991, 4 (1): 5- 28
doi: 10.1007/BF00057884 |
| 13 |
Li B , Li D P , Xiong D W . Alpha-robust mean-variance reinsurance-investment strategy. Journal of Economic Dynamics and Control, 2016, 70, 101- 123
doi: 10.1016/j.jedc.2016.07.001 |
| 14 |
Kang Z L , Li X , Li Z F , Zhu S S . Data-driven robust mean-CVaR portfolio selection under distribution ambiguity. Quantitative Finance, 2019, 19, 105- 121
doi: 10.1080/14697688.2018.1466057 |
| 15 |
Li B , Luo P , Xiong D W . Equilibrium strategies for alpha-maxmin expected utility maximization. SIAM Journal on Financial Mathematics, 2019, 10 (2): 394- 429
doi: 10.1137/18M1178542 |
| 16 |
Li D P , Bi J N , Hu M C . Alpha-robust mean-variance investment strategy for DC pension plan with uncertainty about jump-diffusion risk. RAIRO-Operations Research, 2021, 55, S2983- S2997
doi: 10.1051/ro/2020132 |
| 17 |
Marinacci M . Probabilistic sophistication and multiple priors. Econometrica, 2002, 70, 755- 764
doi: 10.1111/1468-0262.00303 |
| 18 |
Ghirardato P , Maccheroni F , Marinacci M . Differentiating ambiguity and ambiguity attitude. Journal of Economic Theory, 2004, 118, 133- 173
doi: 10.1016/j.jet.2003.12.004 |
| 19 |
Klibanoff P , Marinacci M , Mukerji S . A smooth model of decision making under ambiguity. Econometrica, 2005, 73, 1849- 1892
doi: 10.1111/j.1468-0262.2005.00640.x |
| 20 | Bielecki T , Jang I . Portfolio optimization with a defaultable security. Asia-Pacific Financial Markets, 2006, 13, 113- 127 |
| 21 | Duffie D , Singleton K J . Credit Risk: Pricing, Measurement, and Management. Princeton: Princeton University Press, 2003 |
| 22 | Bowers N L, Gerber H, Hickman J, Jones D, Nesbitt C. Actuarial Mathematics. Illinois: The Society of Actuaries, 1997 |
| [1] | 陈叶君,丁惠生. 无穷维随机微分方程的渐近概周期解[J]. 数学物理学报, 2023, 43(2): 341-354. |
| [2] | 赵彦军,孙晓辉,苏丽,李文轩. 具有Logistic增长和Beddington-DeAngelis发生率的随机SIRS传染病模型定性分析[J]. 数学物理学报, 2022, 42(6): 1861-1872. |
| [3] | 刘俊峰,毛磊,王志. 空间非齐次白噪声驱动的分数阶随机热方程的矩估计[J]. 数学物理学报, 2022, 42(4): 1186-1208. |
| [4] | 范楠,王才士,姬红. Bernoulli泛函上典则酉对合的扰动[J]. 数学物理学报, 2022, 42(4): 969-977. |
| [5] | 季锟鹏,彭幸春. 考虑通胀风险与最低绩效保障的损失厌恶型保险公司的最优投资与再保险策略[J]. 数学物理学报, 2022, 42(4): 1265-1280. |
| [6] | 陈密,聂昌伟,刘海燕. 一类离散相依索赔风险模型的随机分红问题[J]. 数学物理学报, 2022, 42(2): 631-640. |
| [7] | 吴晓,孔荫莹,郭圳滨. Polish空间上的折扣马氏过程量子化策略的渐近优化[J]. 数学物理学报, 2022, 42(2): 594-604. |
| [8] | 高志强. 依时变化的随机环境中的分枝随机游动的局部极限定理的二阶展开[J]. 数学物理学报, 2022, 42(1): 282-305. |
| [9] | 张传洲,李甜甜,焦樊.   |
| [10] | 李江,蓝桂杰,张树文,魏春金. 一类随机葡萄糖-胰岛素模型动力学分析[J]. 数学物理学报, 2021, 41(6): 1937-1949. |
| [11] | 刘丽雅,蒋达清. 具有一般反应函数与贴壁生长现象的随机恒化器模型的全局动力学行为[J]. 数学物理学报, 2021, 41(6): 1912-1924. |
| [12] | 张仲华,张倩. 转换机制下具有非线性扰动的随机SIVS传染病模型的定性分析[J]. 数学物理学报, 2021, 41(4): 1218-1234. |
| [13] | 丁毅,郭精军. 时变混合分数布朗运动下带交易费用的亚式期权定价[J]. 数学物理学报, 2021, 41(4): 1135-1146. |
| [14] | 林运国. 时间非齐次二态量子游荡的演化过程分析[J]. 数学物理学报, 2021, 41(4): 1097-1110. |
| [15] | 杨晓凤,董华,戴洪帅. 混合观测体系下谱负Lévy过程的Parisian破产问题[J]. 数学物理学报, 2021, 41(2): 548-561. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 197
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 188
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|