一类三维逆时热传导问题的数值求解

摘要/Abstract

摘要：

该文考虑一类三维逆时热传导问题的数值解法.基于有限差分时间离散，并结合伽辽金（Galerkin）方法对空间进行有限元离散，导出刚度矩阵及载荷向量，对热传导问题进行数值求解.针对反问题，利用分离变量法建立T时刻温度场与初始温度场之间的对应关系，给出了反演公式，并在一定先验假设条件下证明了反问题的局部稳定性.为克服反问题求解的不适定性，使用吉洪诺夫（Tikhonov）正则化和终值数据扰动正则化方法反演了初始温度场，通过数值实验验证了算法的有效性.

关键词: 三维逆时热传导问题, 有限元, 不适定性, 正则化方法

Abstract:

In this paper, we consider the numerical solution of a three-dimensional inverse heat conduction problem. Based on the finite difference and the finite element method, the stiffness matrix and load vector are derived to solve the heat conduction problem. We use the variable separation method to establish the corresponding relationship between the temperature field at time T and the initial temperature field for the inverse problem. The inversion formulation is obtained. The local stability for the inverse problems is proved under certain priori assumptions. To overcome the ill-posedness for solving the inverse problem, we used the Tikhonov regularization and perturbation regularization method to reconstruct the initial temperature field. We verified the effectiveness of the algorithm through several numerical experiments.

Key words: 3-D inverse heat conduction problem, Finite element, Ill-posedness, Regularization method

中图分类号:

O241.8

孟庆春,张磊. 一类三维逆时热传导问题的数值求解[J]. 数学物理学报, 2022, 42(1): 187-200.

Qingchun Meng,Lei Zhang. Numerical Solution of the Three-Dimensional Inverse Heat Conduction Problems[J]. Acta mathematica scientia,Series A, 2022, 42(1): 187-200.

图/表 8

表 1

图 1

表 2

图 2

表 3

图 3

表 4

图 4

参考文献 19

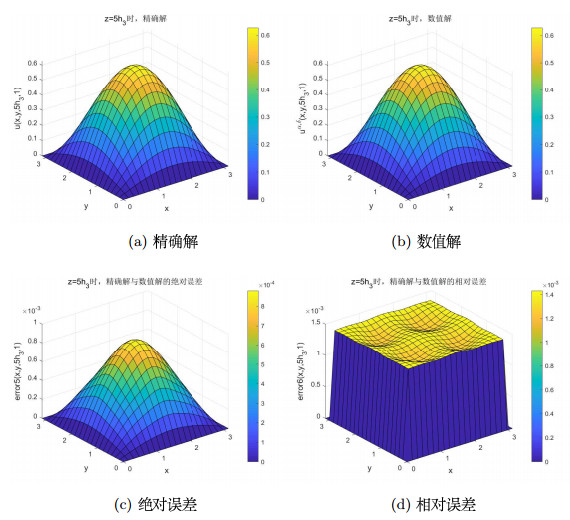
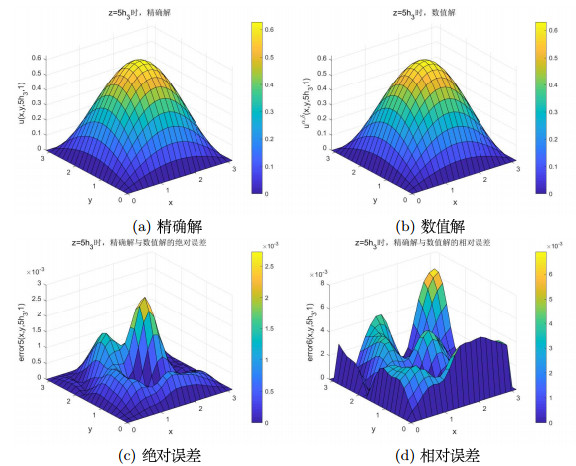
1	Hazanee A , Ismailov M I , Lesnic D . An inverse time-dependent source problem for the heat equation. Appl Numer Math, 2013, 69: 13- 33 doi: 10.1016/j.apnum.2013.02.004
2	Zhang J L , Sheng T T . Dynamic system method for solving inverse problems in heat conduction equations. J Comput Sci Eng, 2014, 11: 413- 417
3	Min T , Zang S Q , Chen S N . Source strength identification problem for the three-dimensional inverse heat conduction equations. Inverse Probl Sci Eng, 2020, 28 (6): 827- 838 doi: 10.1080/17415977.2019.1665663
4	Wagner B M , Fernando M R . A comparison of some inverse methods for estimating the initial condition of the heat equation. J Comput Appl Math, 1999, 103 (1): 145- 163 doi: 10.1016/S0377-0427(98)00249-0
5	Khalid M , Salim M , Zaman F D . Initial inverse problem in heat equation with Bessel operator. Int J Heat Mass Tran, 2002, 45 (14): 2959- 2965 doi: 10.1016/S0017-9310(02)00019-4
6	Yang T , Zhen W W , Xie J X . Reversing inverse problem of source term of heat conduction equation. Adv Appl Math, 2019, 8 (1): 105- 110 doi: 10.12677/AAM.2019.81012
7	Damirchi J , Yazdanian A R , Shamami T R . Numerical investigation of an inverse problem based on regularization method. Math Sci, 2019, 13 (3): 193- 199 doi: 10.1007/s40096-019-0288-2
8	Tuan N H , Binh T T , Minh N D . An improved regularization method for initial inverse problem in 2-D heat equation. Appl Math Model, 2015, 39 (2): 425- 437 doi: 10.1016/j.apm.2014.05.014
9	Chen H , Frankel J I , Keyhani M . Nonlinear inverse heat conduction problem of surface temperature estimation by calibration integral equation method. Numer Heat Tr B-Fund, 2018, 73 (5): 263- 291 doi: 10.1080/10407790.2018.1464316
10	Joachimiak M , Ciaikowski M . Nonlinear unsteady inverse boundary problem for heat conduction equation. Archives of Thermodynamics, 2017, 38 (2): 81- 100 doi: 10.1515/aoter-2017-0011
11	Chapko R , Mindrinos L . On the non-linear integral equation approach for an inverse boundary value problem for the heat equation. J Eng Math, 2019, 119 (2): 255- 268
12	Ma K Y , Prakash P , Deiveegan A . Generalized Tikhonov methods for an inverse source problem of the time-fractional diffusion equation. J Eng Math, 2018, 108: 39- 48
13	Liu C J , Wei T . Moving boundary identification for a two-dimensional inverse heat conduction problem. Inverse Probl Sci En, 2011, 19 (8): 1139- 1154 doi: 10.1080/17415977.2011.603084
14	Lewis R W , Nithiarasu P , Seetharamu K N . Fundamentals of the Finite Element Method for Heat and Fluid Flow. New York: John Wiley & Sons, 2004: 152- 155
15	孔祥谦. 有限单元法在传热学中的应用. 北京: 科学出版社, 1998: 29- 31
	Kong X Q . The Application of the Finite Element Method in Heat Transfer. Beijing: Science Press, 1998: 29- 31
16	Bourgeois H M , Kirsch A , Rundell W . Inverse problems for partial differential equations. Oberwolfach Reports, 2012, 9 (1): 611- 659 doi: 10.4171/OWR/2012/11
17	刘继军. 不适定问题的正则化方法及应用. 北京: 科学出版社, 2005: 132- 156
	Liu J J . The Regularization Methods and Applications of Ill-posed Problems. Beijing: Science Press, 2005: 132- 156
18	Liu J J . Numerical solution of forward and backward problem for 2-D heat conduction equation. J Comput Appl Math, 2002, 145 (2): 459- 482 doi: 10.1016/S0377-0427(01)00595-7
19	Tikhonov A N , Goncharsky A V , Stepanov V V , Yagola A G . Solutions of Ill-posed Problems. Washington, DC: Winston, 1977: 188- 196

T	J=10			J=20
T	$\left\\|e_{1}\right\\|_{L^{2}}$	$\left\\|e_{1}\right\\|_{L ^{\infty}}$	$\left\\|e_{2}\right\\|$	$\left\\|e_{1}\right\\|_{L^{2}}$	$\left\\|e_{1}\right\\|_{L ^{\infty}}$	$\left\\|e_{2}\right\\|$
2	0.0972	0.0087	0.0111	0.0961	0.0030	0.0039
4	0.1537	0.0137	0.0222	0.1514	0.0048	0.0077
6	0.1824	0.0163	0.0335	0.1790	0.0057	0.0116
8	0.1923	0.0172	0.0449	0.1881	0.0059	0.0155

T	J=30			J=40
T	$\left\\|e_{1}\right\\|_{L^{2}}$	$\left\\|e_{1}\right\\|_{L ^{\infty}}$	$\left\\|e_{2}\right\\|$	$\left\\|e_{1}\right\\|_{L^{2}}$	$\left\\|e_{1}\right\\|_{L ^{\infty}}$	$\left\\|e_{2}\right\\|$
2	0.0961	0.0030	0.0039	0.1435	0.0016	0.0020
4	0.1514	0.0048	0.0077	0.2260	0.0025	0.0041
6	0.1790	0.0057	0.0116	0.2669	0.0030	0.0061
8	0.1881	0.0059	0.0155	0.2803	0.0031	0.0082

δ	α = 0.0001			α = 0.0005
δ	$\left\\|e_{3}\right\\|_{L^{2}}$	$ \left\\|e_{3}\right\\|_{L ^{\infty}}$	$\left\\|e_{4}\right\\|$	$\left\\|e_{3}\right\\|_{L^{2}}$	$ \left\\|e_{3}\right\\|_{L ^{\infty}}$	$\left\\|e_{4}\right\\|$
0.001	0.3704	0.0119	0.0117	0.3165	0.0100	0.0100
0.005	0.4378	0.0134	0.0138	0.3815	0.0126	0.0121
0.01	0.5333	0.0198	0.0169	0.4628	0.0156	0.0146
0.05	1.3499	0.0734	0.0427	1.1337	0.0432	0.0359

δ	α = 0.001			α = 0.005
δ	$\left\\|e_{3}\right\\|_{L^{2}}$	$ \left\\|e_{3}\right\\|_{L ^{\infty}}$	$\left\\|e_{4}\right\\|$	$\left\\|e_{3}\right\\|_{L^{2}}$	$ \left\\|e_{3}\right\\|_{L ^{\infty}}$	$\left\\|e_{4}\right\\|$
0.001	0.2490	0.0080	0.0079	0.2778	0.0088	0.0088
0.005	0.3122	0.0100	0.0099	0.2147	0.0068	0.0068
0.01	0.3950	0.0122	0.0125	0.1381	0.0048	0.0044
0.05	1.0394	0.0332	0.0329	0.5074	0.0152	0.0160

α	T=4			T=6
α	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$
0.0001	0.2490	0.0080	0.0079	0.2778	0.0088	0.0088
0.0005	0.3122	0.0100	0.0099	0.2147	0.0068	0.0068
0.001	0.3950	0.0122	0.0125	0.1381	0.0048	0.0044
0.005	1.0394	0.0332	0.0329	0.0377	0.0011	0.0013

α	T=8			T=10
α	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$
0.0001	0.4306	0.0141	0.0154	0.5406	0.0201	0.0193
0.0005	0.3987	0.0130	0.0142	0.4987	0.0159	0.0178
0.001	0.3616	0.0117	0.0129	0.4513	0.0142	0.0161
0.005	0.0686	0.0023	0.0024	0.0789	0.0024	0.0028

α	T=20			T=30
α	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$
0.0001	1.0701	0.0332	0.0382	1.5610	0.0492	0.0557
0.0005	0.9424	0.0297	0.0336	1.1354	0.0359	0.0405
0.001	0.7844	0.0247	0.0280	0.6202	0.0196	0.0221
0.005	0.4205	0.0133	0.0150	2.9273	0.0926	0.1044

α	T=40			T=50
α	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$
0.0001	1.8839	0.0595	0.0672	1.6395	0.0519	0.0585
0.0005	0.5125	0.0162	0.0183	2.3469	0.0742	0.0837
0.001	1.0346	0.0327	0.0369	6.0408	0.1910	0.2154
0.005	9.2018	0.2910	0.3281	17.8107	0.5632	0.6350

α	T=4			T=6
α	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$
0.0001	0.6014	0.0454	0.0214	0.9492	0.0748	0.0338
0.0005	0.2497	0.0135	0.0089	0.3730	0.0185	0.0133
0.001	0.2042	0.0101	0.0073	0.3015	0.0144	0.0108
0.005	0.0210	0.0015	0.0007	0.0585	0.0031	0.0021

α	T=8			T=10
α	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$
0.0001	1.0726	0.0650	0.0382	1.1132	0.0726	0.0397
0.0005	0.4502	0.0205	0.0161	0.5333	0.0230	0.0190
0.001	0.3932	0.0199	0.0140	0.4762	0.0163	0.0170
0.005	0.0865	0.0044	0.0031	0.0953	0.0037	0.0034

α	T=20			T=30
α	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$
0.0001	1.1529	0.0486	0.0411	1.5826	0.0521	0.0564
0.0005	0.9618	0.0333	0.0343	1.1500	0.0356	0.0401
0.001	0.7997	0.0240	0.0285	0.6346	0.0203	0.0226
0.005	0.4066	0.0131	0.0145	2.9149	0.0922	0.1039

α	T=40			T=50
α	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$	$\left\\|e_{5}\right\\|_{L^{2}}$	$\left\\|e_{5}\right\\|_{L ^{\infty}}$	$\left\\|e_{6}\right\\|$
0.0001	1.8994	0.0617	0.0677	1.6546	0.0525	0.0590
0.0005	0.5265	0.0166	0.0188	2.3341	0.0738	0.0832
0.001	1.0211	0.0325	0.0364	6.0298	0.1907	0.2150
0.005	9.1925	0.2907	0.3278	17.8057	0.5631	0.6349

[1]	张宏武. 一类半线性椭圆方程柯西问题的正则化方法[J]. 数学物理学报, 2022, 42(1): 45-57.
[2]	杨晓侠,张厚超. Extended Fisher-Kolmogorov方程的间断有限元分析[J]. 数学物理学报, 2021, 41(6): 1880-1896.
[3]	王克彦,王奇生. 一类非线性双曲型方程扩展混合有限元方法的误差估计[J]. 数学物理学报, 2021, 41(2): 468-478.
[4]	李婷,黄鹏展. 向列相液晶流的模块grad-div稳定化有限元方法[J]. 数学物理学报, 2021, 41(2): 451-467.
[5]	杨帆,王乾朝,李晓晓. 识别Rayleigh-Stokes方程源项的分数阶Landweber迭代正则化方法[J]. 数学物理学报, 2021, 41(2): 427-450.
[6]	李伟,黄鹏展. 流体相互作用模型的粘性分离有限元方法[J]. 数学物理学报, 2020, 40(5): 1362-1380.
[7]	王俊俊,杨晓侠. 一类非线性抛物方程H¹-Galerkin混合有限元方法的高精度分析[J]. 数学物理学报, 2019, 39(4): 894-908.
[8]	葛志昊,葛媛媛. 几乎不可压缩线性弹性问题的多重网格Uzawa型混合有限元方法[J]. 数学物理学报, 2018, 38(5): 873-882.
[9]	文娟, 何银年, 黄鹏展, 李敏. Navier-Stokes方程几种稳定化有限元算法数值比较[J]. 数学物理学报, 2016, 36(3): 543-557.
[10]	陈传军, 赵鑫. 一类非线性对流扩散方程两重网格特征有限元方法及误差估计[J]. 数学物理学报, 2014, 34(3): 643-654.
[11]	杨宇博, 祝鹏, 尹云辉. 奇异摄动问题最优阶一致收敛的间断有限元分析[J]. 数学物理学报, 2014, 34(3): 716-726.
[12]	赵振宇, 由雷. 求解热源识别问题的修正吉洪诺夫正则化方法[J]. 数学物理学报, 2014, 34(1): 186-192.
[13]	石东洋, 王海红. 非线性Sobolev方程的经济型差分-流线扩散非协调有限元法[J]. 数学物理学报, 2013, 33(6): 1148-1161.
[14]	李磊, 孙萍, 罗振东. 抛物方程一种新混合有限元格式及误差分析[J]. 数学物理学报, 2012, 32(6): 1158-1165.
[15]	何斯日古楞, 李宏. 弹性动力学问题的混合间断时空有限元法[J]. 数学物理学报, 2012, 32(6): 1179-1190.