具有免疫时滞的分数阶HBV感染模型稳定性分析

摘要/Abstract

摘要：

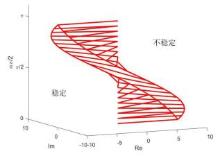
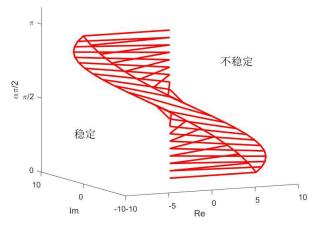
基于相关的病理知识，研究了具有免疫时滞和非线性发生率的分数阶HBV感染模型的稳定性问题.讨论了系统解的存在唯一性、正性和有界性.此外，利用泛函微分方程和Caputo分数阶导数的稳定性理论，通过分析模型在平衡点处超越特征方程根的分布情况，讨论了时滞对平衡点稳定性的影响.研究结果表明：时滞不影响无病平衡点的稳定性，但会诱发地方病平衡点的稳定性，并且在其附近产生小振幅的周期解.通过构造合适的Lyapunov函数，分析了无病平衡点的全局渐进稳定性.最后，利用分数阶时滞稳定性原理，设计相应线性控制器，对分数阶HBV感染模型进行有效控制.

关键词: 时滞, 分数阶, HBV, 稳定性

Abstract:

In this paper, we study the stability of fractional-order HBV (Hepatitis B Virus) infection model with immune delay and nonlinear incidence. Initially, the existence, uniqueness, positivity and boundedness of the model solutions are discussed. In addition, with the stability theory of functional differential equation, combining some new lemmas about Caputo fractional derivatives and some theories about fractional dynamic system, we discuss the in?uence of time delay on the stability of equilibrium point by analyzing the distribution of the characteristic equation roots on the equilibrium point. The results show that time delay does not a?ect the stability of disease-free equilibrium, while induces the stability of endemic equilibrium and produces periodic solutions with small amplitude nearby. Meanwhile, global asymptotic stability of the disease-free equilibrium is investigated by constructing a suitable Lyapunov function. Finally, using the fractional order delay stability principle, the corresponding linear controller is designed to effectively control the fractional order HBV infection model.

Key words: Time delay, Fractional order, HBV, Stability

中图分类号:

O175

李喜玲,高飞,李文琴. 具有免疫时滞的分数阶HBV感染模型稳定性分析[J]. 数学物理学报, 2021, 41(2): 562-576.

Xiling Li,Fei Gao,Wenqin Li. Stability Analysis of Fractional-Order Hepatitis B Virus Infection Model With Immune Delay[J]. Acta mathematica scientia,Series A, 2021, 41(2): 562-576.

图/表 1

参考文献 38

1	Marion P L, Robinson W S. Hepadna Viruses: Hepatitis B and Related Viruses//Cooper M, et al. Curr Topic Microbiol Immu, 1983, 105: 99-121
2	Lavanchy D . Worldwide epidemiology of HBV infection, disease burden, and vaccine prevention. J Clin Virol, 2005, 34 (Suppl 1): S1- S3
3	Nowak M A , Bonhoeffer S , Hill A M , et al. Viral dynamics in hepatitis B virus infection. Proc Natl Acad Sci, 1996, 93 (9): 4398- 4402 doi: 10.1073/pnas.93.9.4398
4	Nowak M A , Bangham C R M . Population dynamics of immune responses to persistent viruses. Science, 1996, 272 (5258): 74- 79 doi: 10.1126/science.272.5258.74
5	Min L Q , Su Y M , Kuang Y . Mathematical analysis of a basic virus infection model with application to HBV infection. Rocky MT J Math, 2008, 38 (5): 1573- 1585
6	Gourley S A , Kuang Y , Nagy J D . Dynamics of a delay differential equation model of hepatitis B virus infection. J Biol Dynam, 2008, 2 (2): 140- 153 doi: 10.1080/17513750701769873
7	马庆波, 向华. 具有时滞的HBV病毒动力学模型稳定性分析. 生物信息学, 2009, 7 (4): 326- 329 doi: 10.3969/j.issn.1672-5565.2009.04.022
	Ma Q B , Xiang H . Stability analysis of HBV virus dynamics model with delay. Bioinformatics, 2009, 7 (4): 326- 329 doi: 10.3969/j.issn.1672-5565.2009.04.022
8	Wang K F , Fan A J , Torres A . Global properties of an improved hepatitis B virus model. Nonlinear Anal-Real, 2010, 11 (4): 3131- 3138 doi: 10.1016/j.nonrwa.2009.11.008
9	Xie Q Z , Huang D W , Zhang S D , et al. Analysis of a viral infection model with delayed immune response. Appl Math Model, 2010, 34 (9): 2388- 2395 doi: 10.1016/j.apm.2009.11.005
10	Vargas-De-Leon C . Stability analysis of a model for HBV infection with cure of infected cells and intracellular delay. Appl Math Comput, 2012, 219 (1): 389- 398
11	Wang Y , Liu X N . Dynamical behaviors of a delayed HBV infection model with logistic hepatocyte growth, cure rate and CTL immune response. Japan J Ind Appl Math, 2015, 32 (3): 575- 593 doi: 10.1007/s13160-015-0184-6
12	靖晓洁, 赵爱民, 刘桂荣. 考虑部分免疫和环境传播的麻疹传染病模型的全局稳定性. 数学物理学报, 2019, 39A (1): 909- 917 doi: 10.3969/j.issn.1003-3998.2019.04.018
	Jing X J , Zhao A M , Liu G R . Global stability of a measles epidemic model with partial immunity and environmental transmission. Acta Math Sci, 2019, 39A (4): 909- 917 doi: 10.3969/j.issn.1003-3998.2019.04.018
13	Dokoumetzidis A , Macheras P . Fractional kinetics in drug absorption and disposition processes. Journal of Pharmacokinetics and Pharmacodynamics, 2009, 36 (2): 165- 178 doi: 10.1007/s10928-009-9116-x
14	Srivastava H M , Saad K M , Khader M M . An efficient spectral collocation method for the dynamic simulation of the fractional epidemiological model of the ebola virus. Chaos Soliton Fract, 2020, 140, 110174 doi: 10.1016/j.chaos.2020.110174
15	朱波, 刘立山. 带瞬时脉冲的分数阶非自制发展方程解的存在唯一性. 数学物理学报, 2019, 39A (1): 105- 113 doi: 10.3969/j.issn.1003-3998.2019.01.010
	Zhu B , Liu L S . Existence and uniqueness of the mild solutions for a class of fractional non-autonomous evolution equations with impulses. Acta Math Sci, 2019, 39A (1): 105- 113 doi: 10.3969/j.issn.1003-3998.2019.01.010
16	Stanislavsky A A . Memory effects and macroscopic manifestation of randomness. Phys Rev E, 2000, 61 (5): 4752- 4759 doi: 10.1103/PhysRevE.61.4752
17	Vargas-De-Leon C . Volterra-type Lyapunov functions for fractional-order epidemic systems. Commun Nonlinear Sci Numer Simul, 2015, 24, 75- 85 doi: 10.1016/j.cnsns.2014.12.013
18	Salman S M , Yousef A M . On a fractional-order model for HBV infection with cure of infected cells. Journal of the Egyptian Mathematical Society, 2017, 25 (4): 445- 451 doi: 10.1016/j.joems.2017.06.003
19	Ullah S , Khan M A , Farooq M . A new fractional model for the dynamics of the hepatitis B virus using the Caputo-Fabrizio derivative. Eur Phys J Plus, 2018, 133 (6): 237 doi: 10.1140/epjp/i2018-12072-4
20	Ullah S , Khan M A , Farooq M . Modeling and analysis of the fractional HBV model with Atangana-Baleanu derivative. Eur Phys J Plus, 2018, 133 (8): 313 doi: 10.1140/epjp/i2018-12120-1
21	高飞, 胡道楠, 童恒庆, 等. 分数阶Willis环脑迟发性动脉瘤时滞系统混沌分析. 物理学报, 2018, 67 (15): 303- 313
	Gao F , Hu D N , Tong H Q , et al. Chaotic analysis of fractional Willis delayed aneurysm system. Acta Phys Sin, 2018, 67 (15): 303- 313
22	Wang X H , Wang Z , Huang X , et al. Dynamic analysis of a delayed fractional-order SIR model with saturated incidence and treatment functions. Int J Bifurcat Chaos, 2018, 28 (14): 1850180 doi: 10.1142/S0218127418501808
23	Podlubny I . Fractional Differential Equations. New York: Academic Press, 1999
24	Diethelm K . The Analysis of Fractional Differential Equations: An Application-Oriented Exposition Using Differential Operators of Caputo type. New York: Springer, 2010
25	Huo R , Wang X L , Wu G R . Large existence and uniqueness of solutions for a class of fractional integral-differential equations with time-delay. Journal of Inner Mongolia Agricultural University(Natural Science Edition), 2014, (35): 169
26	Li Y , Chen Y Q , Podlubny I . Mittag-Leffler stability of fractional order nonlinear dynamic systems. Automatica, 2009, 45 (8): 1965- 1969 doi: 10.1016/j.automatica.2009.04.003
27	Li Y , Chen Y Q , Podlubny I . Stability of fractional-order nonlinear dynamic systems: Lyapunov direct method and generalized Mittag-Leffler stability. Comput Math Appl, 2010, 59 (5): 1810- 1821 doi: 10.1016/j.camwa.2009.08.019
28	Hu J B , Lu G P , Zhang S B , et al. Lyapunov stability theorem about fractional system without and with delay. Commun Nonlinear Sci, 2015, 20 (3): 905- 913 doi: 10.1016/j.cnsns.2014.05.013
29	Aguila-Camacho N , Duarte-Mermoud M A , Gallegos J A . Lyapunov functions for fractional order systems. Commun Nonlinear Sci, 2014, 19 (9): 2951- 2957 doi: 10.1016/j.cnsns.2014.01.022
30	张琪昌. 分岔与混沌理论及应用. 天津: 天津大学出版社, 2005
	Zhang Q C . Bifurcation and Chaos Theory and Its Application. Tianjin: Tianjin University Press, 2005
31	Cooke K L , Van Den Driessche P . On zeroes of some transcendental equations. Funkc Ekvacioj, 1986, 29 (1): 77- 90
32	Matignon D . Stability results for fractional differential equations with applications to control processing. Computational Engineering in Systems Applications, 1996, 2, 963- 968
33	庄科俊. 一类时滞乙肝病毒模型的稳定性分析. 中北大学学报(自然科学版), 2015, (2): 122- 125 doi: 10.3969/j.issn.1673-3193.2015.02.006
	Zhuang K J . Stability analysis for a hepatitis B virus model with time delay. Journal of North University of China(Natural Science Edition), 2015, (2): 122- 125 doi: 10.3969/j.issn.1673-3193.2015.02.006
34	Van den Driessche P , Watmough J . Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math Biosci, 2002, 180 (1/2): 29- 48
35	Tam J . Delay effect in a model for virus replication. Math Med Bilo: A Journal of the IMA, 1999, 16 (1): 29- 37 doi: 10.1093/imammb/16.1.29
36	Li M Y , Shu H . Global dynamics of an in-host viral model with intracellular delay. B Math Biol, 2010, 72 (6): 1492- 1505 doi: 10.1007/s11538-010-9503-x
37	Ruan S , Wei J . On the zeros of transcendental functions with applications to stability of delay differential equations with two delays. Dynam Cont Dis Ser A, 2003, 10, 863- 874
38	Lasalle J P . The Stability of Dynamical Systems. Philadelphia: SIMA, 1976

[1]	徐家发,罗洪林,刘立山. 一类具有p-Laplacian算子的分数阶差分方程边值问题的正解[J]. 数学物理学报, 2021, 41(2): 402-414.
[2]	任晶,翟成波. 基于变分法的回火分数阶脉冲微分系统分析[J]. 数学物理学报, 2021, 41(2): 415-426.
[3]	杨帆,王乾朝,李晓晓. 识别Rayleigh-Stokes方程源项的分数阶Landweber迭代正则化方法[J]. 数学物理学报, 2021, 41(2): 427-450.
[4]	郭改慧,刘晓慧. 一类自催化可逆生化反应模型的Hopf分支及其稳定性[J]. 数学物理学报, 2021, 41(1): 166-177.
[5]	杜丹丹,朱玉灿. K-框架和紧K-框架的算子扰动的稳定性[J]. 数学物理学报, 2021, 41(1): 29-38.
[6]	西宣宣,侯咪咪,周先锋. 非自治Caputo分数阶发展方程弱解的适定性与不变集[J]. 数学物理学报, 2021, 41(1): 149-165.
[7]	何泽荣,张智强,王阳. 一类非线性年龄等级结构种群模型的稳定性[J]. 数学物理学报, 2020, 40(6): 1712-1722.
[8]	马德香,ÖzbeklerAbdullah. 一类带强迫项的高阶半线性分数阶微分方程的广义Lyapunov不等式[J]. 数学物理学报, 2020, 40(6): 1537-1551.
[9]	李建利,李安然,魏重庆,李刚. 一类分数阶Schrödinger-Kirchhoff方程多重解的存在性[J]. 数学物理学报, 2020, 40(6): 1612-1621.
[10]	韩娅玲,向建林. 一类分数阶p-Laplace方程基态解的存在性及其渐进行为[J]. 数学物理学报, 2020, 40(6): 1622-1633.
[11]	李振杰,李磊. 带有凸非线性项的分数阶Laplace方程的解的对称性[J]. 数学物理学报, 2020, 40(5): 1224-1234.
[12]	吕静云,杨小远. 基于序方法的Hilfer分数阶积分微分方程的逼近能控性[J]. 数学物理学报, 2020, 40(5): 1282-1294.
[13]	朱凯旋,谢永钦,周峰,邓习军. 带有时滞项的复Ginzburg-Landau方程的拉回吸引子[J]. 数学物理学报, 2020, 40(5): 1341-1353.
[14]	李伟,黄鹏展. 流体相互作用模型的粘性分离有限元方法[J]. 数学物理学报, 2020, 40(5): 1362-1380.
[15]	周永辉. 一类具有时滞的非局部反应扩散方程非单调临界行波解的全局稳定性[J]. 数学物理学报, 2020, 40(4): 983-992.