1 引言
回顾测度吸引子和拓扑吸引子的概念, 一个f-向前不变集A称为测度吸引子(拓扑吸引子), 如果满足下列条件
1) 集合A的吸引盆B(A,f):={x:ωf(x)⊂A}(不引起混淆的情况下, 简记为B(A)) 具有正的Lebesgue 测度 (是一个剩余集, 即可数开的稠密子集的交集);
2) 不存在严格包含于A的向前不变集A0使得B(A0)具有正的 Lebesgue 测度 (是一个剩余集).
注意到, 如果A是一个拓扑吸引子, 则B(A)包含一个开区间. 拓扑吸引子必然是测度吸引子, 但反过来却不一定成立. 寻找各种不同的非正则吸引子一直是一个重要的议题. Lyubich 和 Milnor[19]证明了实二次 Fibonacci 多项式不存在非正则吸引子. Bruin 等[4]证明了一类具有 Fibonacci 组合性质的单峰映射具有 Lebesgue 测度为 0 的非正则吸引子, 这一结论后来被 Bruin[3]推广到了具有 Fibonacci-like 组合性质的单峰映射上. Bruin, Keller 和 Pierre[5]构造了一个临界点c的ω-极限集ω(c)为非正则吸引子的单峰映射. Bruin[3]证明了一类光滑的单峰映射不存在非正则吸引子. Li 和 Wang[16]引入了一类一般的 Fibonacci 单峰映射并讨论了非正则吸引子的存在性问题. Bruin 和 Todd[6] 展示了一个非正则吸引子的例子.
Cantor 吸引子是许多学者感兴趣的重要议题. 在文献[21]中, 一个具有正 Lebesgue 测度的不变 Cantor 集Λ的映射f被构造, 其中f除一个可数集B外是处处可微的且对任意的x∈Λ∖B有|f′(x)|=2. Blokh 和 Lyubich[1] 证明了临界点为c的S-单峰映射的测度吸引子必然是 Cantor 集ω(c)且拓扑熵htop(f|ω(c))=0, 这一结论后来被 Kozlovski[13]推广到了一类除临界点外是C3的单峰映射上. Bruin[3] 研究了S-单峰映射的吸引 Cantor 集存在的拓扑条件. Li 和 Shen[14]研究了一类光滑区间映射的 Cantor 吸引子的 Hausdorff 维数问题. 在文献[15]中, Li 和 Shen 构造了一个具有非正则吸引子的不可重整化映射.
在文献[10]中, 我们通过 Denjoy-like 手术构造了一簇C1单峰映射˜gβ, 使得˜gβ具有一个厚的双曲排斥不变 Cantor 集˜Aβ, 且˜Aβ包含一个非正则吸引子˜A∗β.˜gβ的C1光滑性是由临界点的原像集元素个数的精确估计以及插入区间长度的精确控制保证的. 然而, 以上构造的双曲排斥集˜Aβ是不可达的, 这是因为插入区间上只有可数个点的轨道能够进入到˜Aβ中.
一个有趣的问题是, 是否存在具有可达双曲排斥集的映射? 如果存在, 其光滑性与稳健性如何? 在本文中, 我们将构造一簇结构稳定的C1映射{fα}, 使得每个fα具有一个可达的双曲排斥不变 Cantor 集Aα, 且Aα具有正 Lebesgue 测度与正拓扑熵. 我们称一个集合A是可达的, 如果A的吸引盆B(A)与A的差集B(A)∖A具有正 Lebesgue 测度. 称一簇映射{fα}是稳健的或结构稳定的, 如果对不同的α和α′, 映射fα与fα′拓扑共轭, 即, 存在一个同胚映射h使得fα∘h=h∘fα′成立. 映射fα的构造类似于 Denjoy 反例的构造[8]以及具有正Lebesgue 测度的马蹄的构造[2]. 更详细地说, 我们考虑分段线性映射
其中,f在不连续点c=12处的值是模糊的. 对映射 (1.1) 实施 Denjoy-like 手术, 将不连续点c的原像集∪n≥0f−n(c)上的点替换成开区间, 控制好这些开区间的长度并在区间上定义映射. 未被开区间替换的点构成的集合Aα既是一个可达的双曲排斥不变 Cantor 集, 又是一个非正则吸引子.
本文的主要结论见以下定理.
定理 1.1 存在一簇结构稳定的C1映射fα,1<α<3, 使得fα具有可达的双曲排斥集Aα, 且Aα同时也是一个具有正 Lebesgue 测度的非正则 Cantor 吸引子.
注 1.1 1)fα一共具有三个吸引子: 不动点x0, Cantor 集Aα, 不动点x1. 其中,x0和x1都是fα的拓扑吸引子;
2)fα限制在非正则吸引子Aα上具有正的拓扑熵htop(fα|Aα)=log2, 这是因为对任意的x∈Aα有f′α(x)=2;
3)fα的光滑性做到了最好, 即映射fα不可能是C1+r的, 这是因为具有正的 Lebesgue 测度的双曲排斥 Cantor 集的映射不可能是C1+r的, 参见文献[7, 定理 2.6, p222];
4) 条件1<α<3不是本质的, 这只是为了便于我们定义插入的区间上的映射.
本文剩下内容结构布局如下: 在第 2 节中, 我们对映射 (1.1) 实施 Denjoy-like 手术, 给出本文的映射fα的构造方法. 在第 3 节中, 我们列出本文的主要结论并给出定理 1.1 的证明.
2 映射fα的构造
在本节中, 我们首先给出一些记号, 然后通过对映射 (1.1) 实施 Denjoy-like 手术, 构造出本文所需的映射fα. 手术的关键在于控制插入区间的长度和定义这些区间上的映射.
给定一个满足下列条件的正数序列{λn}+∞n=1
事实上, 满足条件 (2.1) 的序列大量存在, 比如, 可取λn=1n(n+1).
令
其中1<α<3, 则
这一性质对fα光滑性的证明至关重要.
定义
其中,c是分段线性映射 (1.1) 的不连续点. 将点x∈Cn标记为序列s(x)=snsn−1⋯s0, 其中si定义为
我们在不连续点c及其原像集上的点分别进行爆破插入一个开区间. 更详细地说, 我们将不连续点c用一个长度为 3 的开区间Jc:=(p,q)替换, 并且将被标记为s(x)=snsn−1⋯s0的点x∈C∖{c}用一个长度为bα,n的开区间Jsnsn−1⋯s0替换, 即|Jsnsn−1⋯s0|=bα,n. 注意到Fn=2n, 因此,
这意味着插入区间的总长度是有限的. 经过上述手术后, 我们将新得到的区间记为Ic:=[a,b]. 进一步地, 我们在a的左侧插入一个长度为 1 的区间I0:=[s,a), 在b的右侧插入一个长度为1的区间I1:=(b,t], 则[s,t]=I0∪Ic∪I1, 其中I0, Ic, I1依赖于参数α的选择. 我们将区间[s,t]记为Iα:=Aα∪Bα, 其中Bα是插入的区间的并集,Aα是Bα的补集.
对于给定序列snsn−1⋯s0, 设Jsnsn−1⋯s0=(un,vn), Jsn−1sn−2⋯s0=(un−1,vn−1), 根据文献[10,引理 1], 可以在区间Jsnsn−1⋯s0上定义一个端点导数等于 2 的C1保向微分同胚映射hsnsn−1⋯s0:Jsnsn−1⋯s0→Jsn−1sn−2⋯s0为
同理, 在区间(s,a)上, 可以定义一个端点导数等于 2 的C1保向微分同胚映射r1:(s,a)→(s,a)为
在区间(b,t)上, 可以定义一个端点导数等于 2 的C1保向微分同胚映射r2:(b,t)→(b,t)为
引理 2.1 映射r1有唯一的吸引不动点x0, 映射r2有唯一的吸引不动点x1.
证 我们只证r1的情形,r2的情形完全类似. 根据定义, 有
令r1(x)=x, x∈(s,a), 则
注意到s=a−1, 从而
因此 (2.2) 式成立当且仅当x=2a−12=s+a2, 从而r1有唯一的不动点, 记为x0. 由
可知
因此,x0是r1的唯一吸引不动点. 证毕.
在区间Jc=(p,q)上, 定义映射g:Jc→Iα为
其中
则g是端点导数等于 2 的C1满映射. 事实上, 映射g1:(p,p+1)→(b,t)是一个转折点为p+12的C1满映射, 且满足
映射g3:(q−1,q)→(s,a)是一个转折点为q−12的C1满映射q−12, 且满足
注意到
根据文献[10, 引理 1] 知,g2:(p+1,q−1)→(a,b)是一个严格单调递减的映射, 且满足
定义Bα上的映射φ:Bα→Bα为
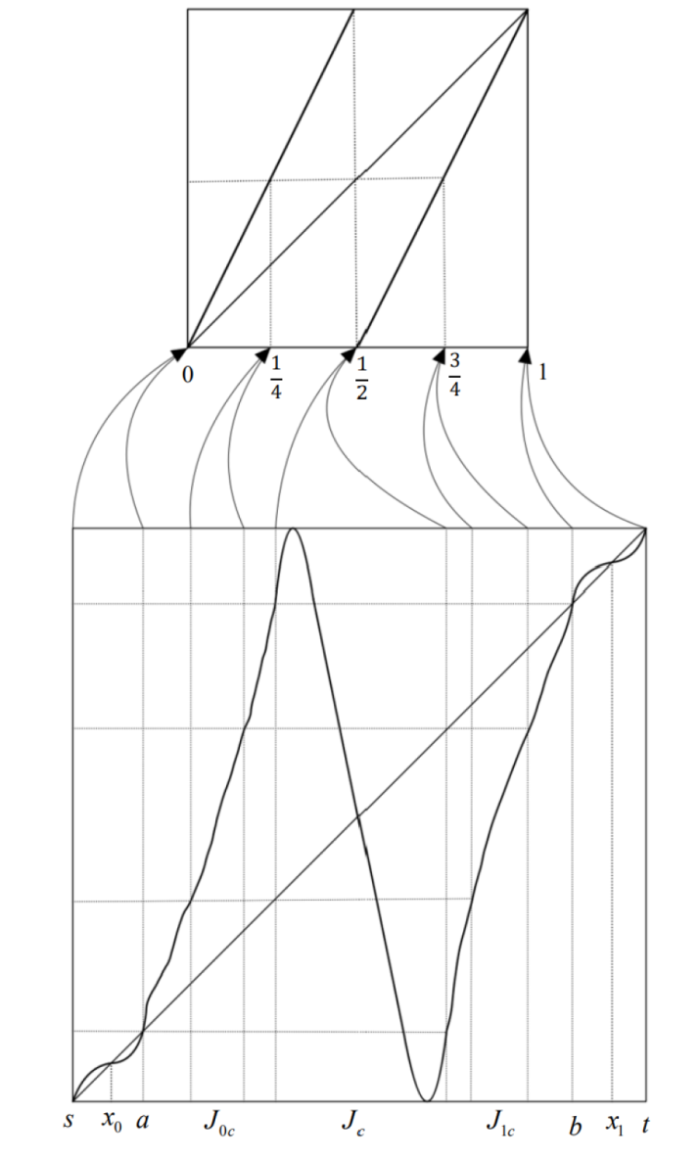
则φ是一个C1满映射. 由于Bα在区间Iα=Aα∪Bα上稠密, 所以映射φ(x)可以延拓到整个区间Iα上. 我们记区间Iα上的连续映射为fα. 映射fα的构造示意图如图1所示.
图1
注 2.1 根据fα的构造, 如果x不属于任何一个插入的区间, 即x∉Bα, 则x的轨道永远不会进入到Bα中, 这意味着Aα是向前不变的, 即fα(Aα)⊆Aα.
3 主要结论
在本节中, 我们将分析上一节构造的映射fα的一些性质, 进而得到本文的主要结论.
命题 3.1 对于任意的1<α<3, 映射fα是C1的, 且对任意的x∈Aα, 有f′(x)=2.
证 由于证明与文献[10, 定理 A]{ding2022thick} 证明完全类似, 因此这里不再重述. 证毕.
注 3.1 根据fα的构造,fα显然不是C2的. 假设Jsnsn−1⋯s0=(un,vn), 则
但是
这意味着f_\alpha不是C^2的. 事实上, 根据定义, 我们有
从而 (3.1)式成立. 注意到
因此,
命题 3.2 \mathcal{A}_\alpha是f_\alpha的一个具有正 Lebesgue 测度的、可达的双曲排斥 Cantor 集, 同时也是f_\alpha的一个非正则吸引子.
证 根据f_\alpha的构造, 被开区间替换的点集是单位区间上的可数稠密子集,\mathcal{A}_\alpha是区间\mathcal{I}_\alpha的闭子集, 且\mathcal{A}_\alpha中的任意点都可以被\mathcal{B}_\alpha中某些开区间的端点逼近, 所以\mathcal{A}_\alpha是一个 Lebesgue 测度为 1 的Cantor 集. 根据命题 3.1, 对任意的x\in \mathcal{A}_\alpha, 有f'(x)=2>1, 又\mathcal{A}_\alpha是一个向前不变集, 故\mathcal{A}_\alpha是双曲排斥的.
由于\mathcal{A}_\alpha\subseteq\mathbb{B}(\mathcal{A}_\alpha), 所以\mathcal{A}_\alpha的吸引盆\mathbb{B}(\mathcal{A}_\alpha)有正的 Lebesgue 测度. 注意到f在单位区间上是拓扑传递的, 这意味着f_\alpha|_{\mathcal{A}_\alpha}是拓扑传递的, 因此, 不存在真包含于\mathcal{A}_\alpha的向前不变闭集\mathcal{A}_{\alpha,0}使得\mathbb{B}(\mathcal{A}_{\alpha,0})有正的 Lebesgue 测度, 从而\mathcal{A}_\alpha是测度吸引子. 由于不存在开区间被\mathcal{A}_\alpha吸引, 所以\mathcal{A}_\alpha不是拓扑吸引子. 事实上,\mathcal{A}_\alpha只能吸引能够到达\mathcal{A}_\alpha的点, 即,\mathbb{B}(\mathcal{A}_\alpha)=\cup_{i=0}^{+\infty}f_\alpha^{-i}(\mathcal{A}_\alpha), 由\mathcal{A}_\alpha的无处稠密性及f_\alpha分段严格单调连续性知\mathbb{B}(\mathcal{A}_\alpha)不包含开区间.
注意到, 开区间在映射J_c=(p,q)在映射f_\alpha作用下被映为整个区间\mathcal{I}_\alpha. 当x\in (p+1, q-1)\subset J_c时, 有
故g_2是一个非奇异的同胚映射. 由于\mathcal{A}_\alpha\subset g_2((p+1,q-1))且\mathcal{A}_\alpha具有正 Lebesgue 测度, 所以g_2^{-1}(\mathcal{A}_\alpha)也具有正 Lebesgue 测度. 由于g_2^{-1}(\mathcal{A}_\alpha)\subset \mathbb{B}(\mathcal{A}_\alpha)且\mathcal{A}_\alpha\subset \mathbb{B}(\mathcal{A}_\alpha), 而g_2^{-1}(\mathcal{A}_\alpha)\subset J_c\subset \mathcal{B}_\alpha与\mathcal{A}_\alpha是互不相交的, 因此\mathbb{B}(\mathcal{A}_\alpha)\backslash \mathcal{A}_\alpha具有正 Lebesgue 测度, 即\mathcal{A}_\alpha是可达的. 证毕.
在探讨映射f_\alpha的结构稳定性之前, 我们将先研究一类区间映射的拓扑共轭问题.
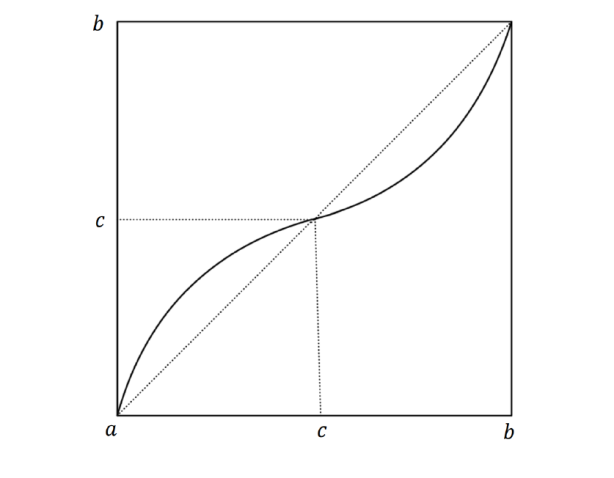
假设J:=[a,b]是一个闭区间,A(J)是满足下列性质的严格单调递增连续映射f的集合
1)f有三个不动点:a,\ b和c, 其中c\in(a,b)是f的吸引不动点;
2) 当x\in(a,c)时,f(x)>x; 当x\in(c,b)时,f(x)<x.
图2
引理 3.1 如果h_1\in A(J_1)和h_2\in A(J_2), 其中J_1和J_2是两个闭区间, 则h_1和h_2是拓扑共轭的.
证 令J_1:=[a_1,b_1]和J_2:=[a_2, b_2],c_1\in (a,b),\ c_2\in (a,b)分别是h_1和h_2的吸引不动点. 要证明h_1和h_2是拓扑共轭的, 只需证明h_1|_{[a_1, c_1]}拓扑共轭于h_2|_{[a_2,c_2]}及h_1|_{[c_1, b_1]}拓扑共轭于h_2|_{[c_2,b_2]}.
任意选择点t_1\in (a_1, c_1)和s_1\in (c_1, b_1), 有
上式给出了J_1的一个分割
其中,I_{0n}和I_{1n}分别定义为
选择任意点t_2\in (a_2, c_2),\ s_2\in (c_2, b_2), 选择任意的两个单调递增的同胚映射\phi_0: (t_1, h_1(t_1)]\rightarrow (t_2, h_2(t_2)]和\phi_1: (s_1, h_1(s_1)]\rightarrow (s_2, h_2(s_2)].定义映射\phi: J_1\rightarrow J_2为
则\phi是h_1和h_2的单调递增的拓扑共轭. 事实上, 对于x\in I_{0n}, 有
类似地, 对于x\in I_{1n}, 有
\phi在每个开区间(h_1^{n}(t_1),\ h_1^{n+1}(t_1)]和每个开区间(h_1^{n}(s_1),\ h_1^{n+1}(s_1)]上是严格单调递增且连续的, 这是因为\phi_0,\ h_1^n和h_2^n都是严格单调递增且连续的. 因此,\phi是区间J_1上的严格单调且连续的映射. 证毕.
命题 3.3 所有的f_\alpha,\ 1<\alpha<3是相互拓扑共轭的.
证 为了书写方便, 假设(\mathcal{I}_1,f_1)和(\mathcal{I}_2,f_2)是通过选择不同的\alpha构造的两个动力系统,\mathcal{A}^i为系统(\mathcal{I}_i,f_i)上的 Cantor 集,I_0^i为点0的左侧插入的区间,I_1^i为点 1 的右侧插入的区间, 其中i=1,\ 2.
要证明f_1拓扑共轭于f_2, 只需证存在一个单调递增的同胚映射\Phi:\mathcal{I}_1\rightarrow \mathcal{I}_2使得对x\in \mathcal{I}_1, 有f_2\circ\Phi(x)=\Phi\circ f_1(x).
由于f_1|_{I_0^1}\in A(I_0^1)和f_2|_{I_0^2}\in A(I_0^2), 根据引理 3.1,f_1|_{I_0^1}拓扑共轭于f_2|_{I_0^2}, 即存在一个单调递增的同胚映射\varphi_0:I_0^1\rightarrow I_0^2使得对任意的x\in I_0^1, 有
其中\varphi_0类似于引理 3.1 的证明中\phi的构造. 类似的, 存在一个单调递增的同胚映射\psi_0:I_1^1\rightarrow I_1^2使得对任意的x\in I_1^2, 有
其中\psi_0类似于引理 3.1 的证明中\phi的构造.
记
则A_n^i是区间\mathcal{I}_i上的一些互不相交的开区间构成的集合. 显然,A_n^1的元素个数等于A_n^2的元素个数, 即,\sharp(A_n^1)=\sharp(A_n^2). 事实上, 我们可以发现
假设
其中A_{n,1}^1,\ A_{n,2}^1,\ \cdots,\ A_{n,3^{n-1}}^1和A_{n,1}^2,\ A_{n,2}^2,\ \cdots,\ A_{n,3^{n-1}}^2在\mathcal{I}_1和\mathcal{I}_2中有相同的排列顺序, 则我们有
定义一个映射\varphi_{1,1}:A_{1,1}^1\rightarrow A_{1,1}^2使得对任意的x\in A_{1,1}^1, 有f_2\circ\varphi_{1,1}(x)=\varphi_{1,1}\circ f_1(x), 则\varphi_{1,1}是一个单调递增的同胚映射, 这是因为\varphi_0是单调递增的同胚映射且f_1|_{A_{1,1}^1}与f_2|_{A_{1,1}^2}有相同的单调性.
注意到, 如果A\in A_n^i, 则f_i(A)\in A_{n-1}^i. 对于一个区间A_{n,u}^1\in A_n^1,\ n>1, 我们假设f_1(A_{n,u}^1)=A_{n-1,v}^1\in A_{n-1}^1, 则A_{n,u}^2\in A_n^2且f_2(A_{n,u}^2)=A_{n-1,v}^2\in A_{n-1}^2. 通过递推, 我们可以定义一个单调递增的同胚映射\varphi_{n,u}:A_{n,u}^1\rightarrow A_{n,u}^2使得对任意的x\in A_{n,u}^1, 有
类似于A_n^i的讨论, 我们记
假设
进一步地, 定义一个单调递增的同胚映射\psi_{1,1}:B_{1,1}^1\rightarrow B_{1,1}^2使得对任意的x\in B_{1,1}^1, 有f_2\circ\psi_{1,1}(x)=\psi_0\circ f_1(x). 类似于\varphi_{n,u}的构造, 通过递推, 定义一个单调递增的同胚映射\psi_{n,u}:B_{n,u}^1\rightarrow B_{n,u}^2使得对任意的x\in B_{n,u}^1, 有
其中f_i(B_{n,u}^i)=B_{n-1,v}^i,\ i=1,2.
根据f_i的构造, 有
且\mathbb{B}(\mathcal{A}^i)在\mathcal{I}_i上是无处稠密的.
定义映射\Phi_0:\mathcal{I}_1\backslash\mathbb{B}(\mathcal{A}^1)\rightarrow \mathcal{I}_2\backslash\mathbb{B}(\mathcal{A}^2)为
将\Phi_0(x)延拓到区间\mathcal{I}_1上, 得到新的映射\Phi: \mathcal{I}_1 \rightarrow \mathcal{I}_2. 显然,\Phi(x)是单调递增的连续映射. 要证明f_1和f_2拓扑共轭, 只需证明
根据\Phi的定义, (3.3) 式对任意的x\in \mathcal{I}_1\backslash\mathbb{B}(\mathcal{A}^1)成立. 如果x\in \mathbb{B}(\mathcal{A}^1)是属于A_n^1或B_n^1的一个区间的端点, 则 (3.3) 式显然成立. 我们不妨假设x_0\in \mathbb{B}(\mathcal{A}^1)不是属于A_n^1或B_n^1的某个区间的端点, 由于\mathbb{B}(\mathcal{A}^1)在\mathcal{I}_1上是无处稠密的, 所以x_0可以被某些属于A_n^1或B_n^1的区间的端点无限趋近. 不妨假设\{y_m\}为属于A_n^1或B_n^1的区间的端点序列且当m\rightarrow +\infty时,y_m趋近于x_0, 则\Phi(y_m)为属于A_n^2或B_n^2的某些区间的端点构成的序列. 由于\Phi(x)和f_1(x)是连续的, 所以且当m\rightarrow +\infty时,\Phi(y_m)趋近于\Phi(x_0),f_1(y_m)趋近于f_1(x_0). 因此,
由于
所以
证毕.
定理 1.1 的证明 根据命题 3.1、命题 3.2 和命题 3.3, 定理 1.1 得证.
参考文献
Measurable dynamics ofS-unimodal maps of the interval
Horseshoe with Positive Measure
DOI:10.1007/BF01389849 URL [本文引用: 1]
Topological conditions for the existence of absorbing Cantor sets
DOI:10.1090/tran/1998-350-06 URL [本文引用: 3]
Wild Cantor attractors exist
Adding machines and wild attractors
DOI:10.1017/S0143385797086392
URL
[本文引用: 1]

We investigate the dynamics of unimodal maps f of the interval restricted\nto the omega limit set X of the critical point for cases where X is a\nCantor set. In particular, many cases where X is\na measure attractor of f are included. We give two classes of examples of\nsuch maps, both generalizing unimodal Fibonacci maps [LM,\nBKNS]. In all\ncases f_{|X} is a continuous factor of a generalized odometer (an adding\nmachine-like dynamical system), and at the same time f_{|X} factors onto\nan irrational circle rotation. In some of the examples we obtain irrational\nrotations on more complicated groups as factors.
Wild attractors and thermodynamic formalism
DOI:10.1007/s00605-015-0747-2 URL [本文引用: 1]
\alpha-limit sets and Lyapunov function for maps with one topological attractor
Thick hyperbolic repelling invariant Cantor sets and wild attractors
DOI:10.1088/1361-6544/acb18f
[本文引用: 4]

Let D be the set of \n \n \n β\n ∈\n (\n 1\n,\n 2\n ]\n \n \n such that f\n \n β\n is a symmetric tent map with finite critical orbit. For \n \n \n β\n ∈\n D\n \n \n, by operating Denjoy-like surgery on f\n \n β\n, we constructed a C\n 1 unimodal map \n \n \n \n \n g\n ˜\n \n β\n \n \n \n admitting a thick hyperbolic repelling invariant Cantor set which contains a wild attractor. The smoothness of \n \n \n \n \n g\n ˜\n \n β\n \n \n \n is ensured by the effective estimation of the preimages of the critical point as well as the prescribed lengths of the inserted intervals. D is dense in \n \n \n (\n 1\n,\n 2\n ]\n \n \n, and \n \n \n \n \n g\n ˜\n \n β\n \n \n \n can not be \n \n \n \n C\n \n 1\n +\n α\n \n \n \n \n because the hyperbolic repelling invariant Cantor set of \n \n \n \n C\n \n 1\n +\n α\n \n \n \n \n map has Lebesgue measure equal to zero.
Milnor attractors and topological attractors of a piecewise linear map.
DOI:10.1088/0951-7715/14/2/304 URL [本文引用: 1]
On Hausdorff dimension of unimodal attractors
DOI:10.1007/s00220-006-1540-9 URL [本文引用: 1]
Getting rid of the negative Schwarzian derivative condition
Hausdorff dimension of Cantor attractors in one-dimensional dynamics
DOI:10.1007/s00222-007-0083-9 URL [本文引用: 1]
The topological complexity of Cantor attractors for unimodal interval maps
DOI:10.1090/tran/2016-368-01 URL [本文引用: 1]
A new class of generalized Fibonacci unimodal maps
DOI:10.1088/0951-7715/27/7/1633 URL [本文引用: 1]
Conjugacy problem of strictly monotone maps with only one jump discontinuity
DOI:10.1007/s00025-019-1126-4
[本文引用: 1]

We provide several new q-congruences for truncated basic hypergeometric series with the base being an even power of q. Our results mainly concern congruences modulo the square or the cube of a cyclotomic polynomial and complement corresponding ones of an earlier paper containing q-congruences for truncated basic hypergeometric series with the base being an odd power of q. We also give a number of related conjectures including q-congruences modulo the fifth power of a cyclotomic polynomial and a congruence for a truncated ordinary hypergeometric series modulo the seventh power of a prime greater than 3.
Combinatorics, geometry and attractors of quasi-quadratic maps
DOI:10.2307/2118604 URL [本文引用: 1]
The Fibonacci unimodal map.
DOI:10.1090/jams/1993-06-02 URL [本文引用: 1]
On the concept of attractor
DOI:10.1007/BF01212280 URL [本文引用: 1]
A map with invariant Cantor set of positive measure. Nonlinear Analysis: Theory