1 引言
玻色-爱因斯坦凝聚 (BEC) 被称为世界物质第五态, 是指玻色子在温度接近绝对零度时达到的物质状态. 它由玻色和爱因斯坦在 1924-1925 年预测[9 ,13 ,14 ] , 并于 1995 年首次在物理实验中实现[1 ,10 ,12 ] .
在早期实验中, 玻色子在磁势阱中不能发生自旋. 1998 年, 利用光学偶极子阱, 人们首次在自旋 $^{23}\mathrm{Na}$ [21 ] . 在光阱中, 不同超精细态的粒子在空间中具有不同的角动量, 从而产生丰富的自旋结构. 自旋量子数为 $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ [15 ,16 ,22 ] . 特别地, 由于粒子自旋和粒子运动的相互影响会产生自旋轨道耦合 (SOC) 效应, 近年, Lin 等[18 ,19 ,20 ] 在中性原子的 BEC 实验中, 成功诱导了自旋轨道耦合旋量 BEC.
在平均场意义下, 温度 $T\ll $ $T_c$ $\mathcal{F}$ $\mathcal{F}+1$ $\Psi(\mathbf{x},t)= {(\psi_{-2}(\mathbf{x},t),\psi_{-1}(\mathbf{x},t), \psi_0(\mathbf{x},t),\psi_{1}(\mathbf{x},t),\psi_{2}(\mathbf{x},t))}^{T}$
(1.1) $ \begin{equation} \begin{split} {\rm i}\partial_{t}\psi_{\pm2} =&[H+\beta_{0}\rho(\Psi)\pm 2\beta_{1}F_{z}(\Psi)]\psi_{\pm2}+\beta_{1}F_{\mp}(\Psi)\psi_{\pm1} +\frac{\beta_{2}}{\sqrt{5}}A_{00}(\Psi)\bar{\psi}_{\mp2}\\&-\gamma({\rm i}\partial_{y}\psi_{\pm1}\mp \partial_{x}\psi_{\pm1}), \end{split} \end{equation} $
(1.2) $ \begin{equation} \begin{split} {\rm i}\partial_{t}\psi_{\pm1} =&[H+\beta_{0}\rho(\Psi)\pm\beta_{1}F_{z}(\Psi)]\psi_{\pm1}+\beta_{1}(\frac{\sqrt{6}}{2}F_{\mp}(\Psi)\psi_{0}+F_{\pm}(\Psi)\psi_{\pm2})\\& -\frac{\beta_{2}}{\sqrt{5}} A_{00}(\Psi)\bar{\psi}_{\mp1} -\gamma[{\rm i}\partial_{y}(\psi_{\pm2}+\frac{\sqrt{6}}{2}\psi_{0})\mp \partial_{x}(\frac{\sqrt{6}}{2}\psi_{0}-\psi_{\pm2})], \end{split} \end{equation} $
(1.3) $ \begin{equation} \begin{split} {\rm i}\partial_{t}\psi_{0} =&[H+\beta_{0}\rho(\Psi)]\psi_{0}+\frac{\sqrt{6}}{2}\beta_{1}[F_{+}(\Psi)\psi_{1}+F_{-}(\Psi)\psi_{-1}] +\frac{\beta_{2}}{\sqrt{5}}A_{00}(\Psi)\bar{\psi}_{0}\\ & -\frac{\sqrt{6}}{2}\gamma[{\rm i}\partial_{y}(\psi_{1}+\psi_{-1})- \partial_{x}(\psi_{-1}-\psi_{1})], \end{split} \end{equation} $
(1.4) $ \begin{equation} \psi_{\ell}(\mathbf{x},0) = \psi_{\ell}^{0}(\mathbf{x}),\quad \mathbf{x}\in \mathbb{R}^{d},\quad\ell = -2,-1,0,1,2. \end{equation} $
这里 $t$ $\mathbf{x}\in \mathbb{R} ^{d} (d=2,3)$ $\gamma$ $\rho$ $\beta_{2}, \beta_{1}, \beta_{0}$ $V(\mathbf{x})$ $V(\mathrm{x})$
其中 $\omega_{\nu}$ $\nu$ - 方向上 ($\nu=x,y,z$ ) 的势阱频率. $V(\mathbf{x})$
这里 $E_{\nu}=\frac{1}{2}\hbar^{2}\hat{q_{\nu}}^{2}$ $I_{\nu}$
波函数 $\Psi(\mathbf{x},t)$
(1.5) $\begin{equation}\mathcal{N}(t):=\mathcal{N}(\Psi(\cdot,t)):=\sum_{\ell=-2}^{2}\int_{\mathbb{R}^d}|\psi_{\ell}(\mathbf{x},t)|^{2}{\rm d}\mathbf{x} \equiv1,\quad t\geq 0; \end{equation}$
(1.6) $ \begin{equation} \mathcal{M}(t):=\mathcal{M}(\Psi(\cdot,t)):=\sum_{\ell=-2}^{2}\int_{\mathbb{R}^d}\ell|\psi_\ell(\mathbf{x},t)|^2{\rm d}\mathbf{x}\equiv M,\quad M\in[-2,2]; \end{equation} $
(1.7) $ \begin{equation} \begin{split} E(t):=\ &E(\Psi(\cdot, t))\\:=\ &\int_{\mathbb{R}^d}\bigg\{\sum\limits_{\ell=-2}^2\left(\frac{1}{2}|\nabla\psi_\ell|^2+V(\mathbf{x})|\psi_\ell|^2 )+\frac{\beta_0}{2}\rho^2+\frac{\beta_1}{2} \left(|F_+(\Psi)|^2+|F_z(\Psi)|^2 \right)\right)\\ &+\frac{\beta_2}{2}|A_{00}(\Psi)|^2-\gamma \left[{\rm i}(\partial_{y}\Psi)^{T} f_{x}{\bar{\Psi}}-(\partial_{x}\Psi)^{T} f_{y}{\bar{\Psi}} \right]\bigg\}{\rm d}\mathbf{x} \\ \equiv\ & E(\Psi(\cdot,0))=:E_0,\quad t\geq0, \end{split} \end{equation} $
其中 $\mathcal{N}$ $\mathcal{M}$ $\bar{\Psi}$ ${\Psi}^{T}$ $\Psi$ $E(\Psi)$ $E_{\rm kin}(\Psi)$ $E_{\rm pot}(\Psi)$ $E_{\rm spin}(\Psi)$ $E_{\rm soc}(\Psi)$
基态模拟是 BEC 研究的重要问题. 自旋轨道耦合 Spin-2 BEC 的基态 $\Phi_{g}(\mathbf{x})$
(1.8) $ \begin{equation} E\left(\Phi_{g}\right) = \min_{\Phi\in S}E(\Phi), \end{equation} $
(1.9) $ \begin{equation} S=\{\Phi = (\phi_{-2},\phi_{-1},\phi_{0},\phi_{1},\phi_{2})^{T}| N(\Phi) = 1, \mathcal{M}(\Phi) = M, E(\Phi)<\infty\}. \end{equation} $
近年已有大量的数值方法模拟 BEC 的基态. Bao 等早期采用直接最小化能量泛函法来求解基态[6 ] . 随后, Bao 和 Du 提出了一种简单且广泛适用的方法, 即正规梯度流法[4 ] 来计算 BEC 的基态. 对于旋转单组份 BEC 模型, Bao 等采用向后欧拉有限差分离散的连续梯度流法[8 ] 研究了模型的基态, 对称态和中心涡态以及能量和化学势图. Antoine 和 Tang 等提出了效率更高的预条件共轭梯度法 (PCG)[2 ] .
对于旋量 BEC 的基态模拟, Bao 和 Wang 为 Spin-1 BEC 构造了连续梯度流并对其采用 Crank-Nicolson 有限差分进行离散[7 ] . 随后, Bao 等利用离散正规梯度流法计算 Spin-1 和 Spin-2 BEC 的基态[3 ,5 ] , 其核心在于发掘投影系数间的隐含关系, 连同模型中的总质量和总磁场量守恒关系, 使得投影步骤所需的所有投影系数可被唯一确定. 此外, Cai 和 Liu 提出了模拟不带自旋轨道耦合项的 Spin-$\mathcal{F}$ [11 ,17 ] .
对于自旋轨道耦合旋量 BEC 模型, Yuan 等[23 ] 提出了投影梯度流法计算自旋轨道耦合 Spin-1 BEC 的基态. 该方法采用空间二阶有限差分和时间 Grank-Nicolson 方法离散连续梯度流, 具有总质量、总磁场量守恒及能量递减的性质. 然而, 自旋轨道耦合 Spin-2 BEC 模型具有复杂的自旋交换项和自旋单线态项, 其基态解在自旋轨道耦合效应下具有复杂的基态相, 可呈现细密的条纹状和晶格状两种图案. 这些特点对模拟自旋轨道耦合 Spin-2 BEC 基态的数值算法在精度与效率方面提出了新的挑战. 目前, 关于自旋轨道耦合 Spin-2 BEC 基态模拟的数值结果十分有限. 本文将带拉格朗日乘子的梯度流算法[11 ,17 ] 推广到自旋轨道耦合 Spin-2 BEC 的基态模拟. 该方法在每步迭代只需求解一个常系数椭圆方程(组), 易实施且效率高.
本文结构安排如下: 第 2 节介绍带拉格朗日乘子的正规梯度法, 给出该方法的梯度流方程及其投影系数的确定方法; 第 3 节介绍其离散格式; 第 4 节给出数值结果.
2 带拉格朗日乘子的正规梯度流法
自旋轨道耦合 Spin-2 BEC (1.1)-(1.3) 对应的连续正规梯度流 (CNGF) 定义为
(2.1) $ \begin{equation} \begin{split} \partial_{t}\phi_{\pm2} &=-(H+\beta_{0}\rho\pm 2\beta_{1}F_{z})\phi_{\pm2}-\beta_{1}F_{\mp}\phi_{\pm1} -\frac{\beta_{2}}{\sqrt{5}}A_{00}\bar{\phi}_{\mp2}\\ &\quad+\gamma({\rm i}\partial_{y}\phi_{\pm1}\mp\partial_{x}\phi_{\pm1})+(\mu\pm2\lambda)\phi_{\pm2}\\ &\triangleq -\left[g_{\pm2}(\Phi)-(\mu\pm2\lambda)\phi_{\pm2}\right],\quad t\geq 0, \end{split} \end{equation} $
(2.2) $ \begin{equation} \begin{split} \partial_{t}\phi_{\pm1} =&-(H+\beta_{0}\rho\pm\beta_{1}F_{z})\phi_{\pm1}-\beta_{1}\left(\frac{\sqrt{6}}{2}F_{\mp}\phi_{0}+F_{\pm}\phi_{\pm2}\right) +\frac{\beta_{2}}{\sqrt{5}}A_{00}\bar{\phi}_{\mp1} \\ &\quad+\gamma\left[{\rm i}\partial_{y}(\phi_{\pm2}+\frac{\sqrt{6}}{2}\phi_{0})\mp \partial_{x}\left(\frac{\sqrt{6}}{2}\phi_{0}-\phi_{\pm2}\right)\right]+(\mu\pm\lambda)\phi_{\pm1},\\ &\hspace{-0.4cm}\triangleq -\left[g_{\pm1}(\Phi)-(\mu\pm\lambda)\phi_{\pm1}\right], \end{split} \end{equation} $
(2.3) $ \begin{equation} \begin{split} \partial_{t}\phi_{0} =&-(H+\beta_{0}\rho)\phi_{0}-\frac{\sqrt{6}}{2}\beta_{1}(F_{+}\phi_{1}+F_{-}\phi_{-1}) -\frac{\beta_{2}}{\sqrt{5}}A_{00}\bar{\phi}_{0}\\ & +\frac{\sqrt{6}}{2}\gamma\left[{\rm i}\partial_{y}(\phi_{1}+\phi_{-1})-\partial_{x}(\phi_{-1}-\phi_{1})\right]+\mu\phi_{0}\\ &\hspace{-0.4cm}\triangleq -\left[g_{0}(\Phi)-\mu\phi_{0}\right]. \end{split} \end{equation} $
这里 $\mu(\Phi)$ $\lambda (\Phi)$ $\Phi$
联立 (2.1)-(2.3) 式并以 $\left(\mu(\Phi)+\ell\lambda(\Phi)\right)\phi_\ell \ (\ell=0,\pm1,\pm 2)$
(2.4) $ \begin{equation} \begin{cases}\mu(\Phi)=\frac{P(\Phi)Q(\Phi)-MR(\Phi)}{P(\Phi)-M^{2}},\\\lambda(\Phi)=\frac{R(\Phi)-MQ(\Phi)}{P(\Phi)-M^{2}}, \end{cases} \end{equation} $
这里及下文, 如无特殊说明, 范数 $\|\cdot\|$ $L^2$ $\|\cdot\|_{L^2}$ .
易知连续正规梯度流 (2.1)-(2.3) 式具有总质量守恒、总磁场量守恒和能量稳定性, 但由于其为非线性积分方程组, 直接离散求解往往计算量大. 为避免这一困难, 下面考虑其一阶分裂格式导出的 GFLM. 取时间步长 $\Delta t$ $t_{n} = n\Delta t (n = 0,1,2,\cdots )$ $ t_{n-1}\leq t \leq t_{n} $
(2.5) $ \begin{equation} \begin{split} \partial_{t}\phi_{\pm2} &=-(H+\beta_{0}\rho\pm 2\beta_{1}F_{z})\phi_{\pm2}-\beta_{1}F_{\mp}\phi_{\pm1} -\frac{\beta_{2}}{\sqrt{5}}A_{00}\bar{\phi}_{\mp2}\\ &\quad+\gamma({\rm i}\partial_{y}\phi_{\pm1}\mp\partial_{x}\phi_{\pm1})+(\mu^{n-1}\pm2\lambda^{n-1})\phi_{\pm2} \\ &\triangleq -\left[g_{\pm2}(\Phi)-(\mu^{n-1}\pm2\lambda^{n-1})\phi_{\pm2}\right], \end{split} \end{equation} $
(2.6) $ \begin{equation} \begin{split} \partial_{t}\phi_{\pm1} =&-(H+\beta_{0}\rho\pm\beta_{1}F_{z})\phi_{\pm1}-\beta_{1}\left(\frac{\sqrt{6}}{2}F_{\mp}\phi_{0}+F_{\pm}\phi_{\pm2}\right) +\frac{\beta_{2}}{\sqrt{5}}A_{00}\bar{\phi}_{\mp1} \\ &\quad+\gamma\left[{\rm i}\partial_{y}(\phi_{\pm2}+\frac{\sqrt{6}}{2}\phi_{0})\mp \partial_{x}\left(\frac{\sqrt{6}}{2}\phi_{0}-\phi_{\pm2}\right)\right]+(\mu^{n-1}\pm\lambda^{n-1})\phi_{\pm1},\\ &\hspace{-0.4cm}\triangleq -\left[g_{\pm1}(\Phi)-(\mu^{n-1}\pm\lambda^{n-1})\phi_{\pm1}\right], \end{split} \end{equation} $
(2.7) $ \begin{equation} \begin{split} \partial_{t}\phi_{0} =&-(H+\beta_{0}\rho)\phi_{0}-\frac{\sqrt{6}}{2}\beta_{1}(F_{+}\phi_{1}+F_{-}\phi_{-1}) -\frac{\beta_{2}}{\sqrt{5}}A_{00}\bar{\phi}_{0}\\ & +\frac{\sqrt{6}}{2}\gamma\left[{\rm i}\partial_{y}(\phi_{1}+\phi_{-1})-\partial_{x}(\phi_{-1}-\phi_{1})\right]+\mu^{n-1}\phi_{0}\\ &\hspace{-0.4cm}\triangleq -\left[g_{0}(\Phi)-\mu^{n-1}\phi_{0}\right]. \end{split} \end{equation} $
(2.8) $ \begin{equation} \phi_{\ell}(\mathbf{x},t_{n}):= \phi_{\ell}(\mathbf{x},t_{n}^{+}) = \alpha_{\ell}^{n}\phi_{\ell}(\mathbf{x},t_{n}^{-}),\quad \ell = -2,-1, \cdots,2, \end{equation} $
这里 $\phi_{\ell}(\mathbf{x},t_{n}^{\pm}) = \lim \limits_{t\rightarrow t_{n}^{\pm}}\phi_{\ell}(\mathbf{x},t)$ $\mu^{n-1}=\mu(\Phi^{n-1})$ $\lambda^{n-1}=\lambda(\Phi^{n-1})$ . 值得指出的是, 投影步骤等价于求解连续正规梯度流 (2.1)-(2.3) 式一阶分裂得到的第二个常微分方程组
(2.9) $ \begin{equation} \partial_{t}\phi_{\ell}(\mathbf{x},t)=\left[(\mu_{\Phi}(t)-\mu^{n-1})+\ell(\lambda_{\Phi}(t)-\lambda^{n-1})\right]\phi_{\ell}(\mathbf{x}, t),\quad \ell = -2,-1,\cdots,2, \end{equation} $
其中 $\mu_{\Phi}(t):=\mu\left(\Phi(\cdot,t) \right)$ $\lambda_{\Phi}(t):=\lambda\left(\Phi(\cdot,t) \right)$
这里 $\alpha_{\ell}^{n} (\ell = -2,-1,0,1,2)$ $n$ $\phi_{\ell}(\mathbf{x},t_n) (\ell = -2,-1,0,1, 2)$
(2.10) $ \begin{equation} \sum_{\ell=-2}^{2}(\alpha_{\ell}^{n})^{2}\|\phi_{\ell}(\cdot,t_{n}^{-})\|^2 = 1, \qquad \sum_{\ell=-2}^{2}\ell(\alpha_{\ell}^{n})^{2}\|\phi_{\ell}(\cdot,t_{n}^{-})\|^2 = M. \end{equation} $
因此, 为唯一确定所有投影系数 $\alpha_{\ell}^{n} (\ell = -2,-1,0,1,2)$ [11 ] 中对 $(\alpha_{\ell}^{n})^2$
(2.11) $ \begin{matrix} (\alpha_{\ell}^{n})^2&=&{\rm e}^{2\int_{t_{n-1}}^{t}\left[(\mu_{\Phi}(s)-\mu^{n-1})+\ell(\lambda_{\Phi}(s)-\lambda^{n-1})\right]{\rm d}s} \nonumber\\ &\approx&1+2\left[\int_{t_{n-1}}^{t}(\mu_{\Phi}(s)-\mu^{n-1}){\rm d}s+\ell\int_{t_{n-1}}^{t}(\lambda_{\Phi}(s)-\lambda^{n-1}){\rm d}s\right] \nonumber\\ &:=&c_{0} + \ell c_1. \end{matrix} $
将 (2.11) 式代入 (2.10) 式, 易得关于 $c_0,c_1$
(2.12) $ \begin{equation} m_0c_0+m_1c_1 = 1, \end{equation} $
(2.13) $ \begin{equation} m_1c_0+m_2c_1 = M, \end{equation} $
若假设至少有两个分量函数 $\phi_{\ell}(\cdot,t_{n}^{-}) (\ell = -2,-1,0,1,2)$
(2.14) $ \begin{equation} c_0=\frac{m_2-m_1M}{m_0m_2-m_1^2},\qquad c_1=\frac{m_0M-m_1}{m_0m_2-m_1^2}. \end{equation} $
(2.15) $ \begin{equation} \alpha_\ell^n={\rm e}^{\frac{c_0+\ell c_1-1}{2}}=\exp\left[\frac{m_2-m_1M+\ell(m_0M-m_1)}{m_0m_2-m_1^2}-\frac{1}{2} \right], \quad \ell=-2,-1,\cdots,2. \end{equation} $
3 离散格式
在势函数 $V(\mathbf{x})$ $|\mathbf{x} |\rightarrow+\infty$
将有界区域 $(a,b)\times (c,d)$ $h_{x} = (b-a)/J > 0$ $h_{y} = (d-c)/K >0$ $J,K$
设 $\Phi_{j,k}^{n} =(\phi_{j,k}^{n,-2},\phi_{j,k}^{n,-1},\phi_{j,k}^{n,0},\phi_{j,k}^{n,1},\phi_{j,k}^{n, 2})$ $\Phi(x_{j},y_{k},t_{n})$ $\Phi^{n}$ $t=t_{n}$ $\Phi_{j,k}^{n}$ . 引入常系数稳定项因子 $\varepsilon^n$
(3.1) $ \begin{equation} \begin{split} \frac{\phi^{*,\ell}_{j,k} - \phi^{n-1,\ell}_{j,k}}{\Delta t}&=\frac{1}{2}\nabla_{h}^{2}\phi^{*,\ell}_{j, k}-\varepsilon^{n-1}\phi^{*,\ell}_{j,k} +\varepsilon^{n-1} \phi^{n-1,\ell}_{j,k} + G^{n-1,\ell}_{j,k}, \end{split} \end{equation} $
(3.2) $ \begin{equation} \phi_{j,k}^{n,\ell} = \alpha_{\ell}^{n} \phi_{j,k}^{*,\ell},\quad \ell =-2,\cdots,2. \\ \phi^{0,\ell}_{j,k}=\phi_{\ell}(x_{j},y_{k},t=0),\end{equation} $
稳定项因子 $\varepsilon^{n}$
(3.3) $ \begin{equation} \varepsilon^n = \frac{1}{2}(b^{n}_{\max}+b^{n}_{\min}), \end{equation} $
这里 $b^{n}_{\max}$ $b^{n}_{\min}$
通过对 $\phi^{n-1,\ell}$
(3.4) $ \begin{equation} \widehat{\phi_{p,q}^{n-1,\ell}}=\frac{1}{JK}\sum_{j=0}^{J-1}\sum_{k=0}^{K-1}\phi_{j,k}^{n-1,\ell}{\rm e}^ {-{\rm i}\mu_p(x_j-a)}{\rm e}^{-{\rm i}\mu_q(y_k-c)}, \end{equation} $
这里 $\mu_{p}=\frac{2p\pi}{b-a}$ $\mu_{q}=\frac{2q\pi}{d-c}$ $p=-J/2,-J/2+1,\cdots,J/2-1$ $q=-K/2,-K/2+1,\cdots, K/2-1$ . 进而, 可得微分算子 $\nabla^{2}$ $\nabla_{h}^{2}$ .
(3.5) $ \begin{equation} \nabla_{h}^{2}\phi^{n-1,\ell}_{j,k}=-\sum_{p=-J/2}^{J/2-1}\sum_{q=-K/2}^{K/2-1}(\mu_{p}^{2}+\mu_{q}^{2}) \widehat{\phi_{p,q}^{n-1,\ell}}{\rm e}^{{\rm i}\mu_p(x_j-a)}{\rm e}^{{\rm i}\mu_q(y_k-c)}. \end{equation} $
(3.6) $ \begin{equation} \frac{\widehat{\phi_{pq}^{*,\ell}} - \widehat{\phi_{pq}^{n-1,\ell}}}{\Delta t}= -\left(\frac{\mu_{p}^{2}}{2} + \frac{\mu_{q}^{2}}{2} + \varepsilon^{n-1}\right)\widehat{\phi_{pq}^{\ast,\ell}} +\varepsilon^{n-1}\widehat{\phi_{pq}^{n-1,\ell}} +\widehat{G^{n-1,\ell}_{pq}}. \end{equation} $
(3.7) $ \begin{equation} \widehat{\phi_{pq}^{*,\ell}} = \frac{1}{1+\Delta t(\frac{\mu_{p}^{2}}{2} + \frac{\mu_{q}^{2}}{2}+\varepsilon^{n-1} )} \left(\widehat{\phi_{pq}^{n-1,\ell}} +\Delta t \varepsilon^{n-1}\widehat{\phi_{pq}^{n-1,\ell}} +\Delta t\widehat{G^{n-1, \ell}_{pq}} \right). \end{equation} $
通过对 (3.7) 式作逆快速傅里叶变换即得方程 (3.1) 的解.
4 数值实验
本章运用 GFLM 方法模拟自旋轨道耦合 Spin-2 BEC 在各参数下的基态. 对循环型体系 $(\beta_{1}>0)$ $\beta_{1}<0)$
其中 $\phi_g({\bf x})$
的基态或其近似 $\phi_{ho}({\bf x})=\frac{1}{\sqrt{\pi}}{\rm e}^{-(x^2+y^2)/2}$ . 除非特别说明, 本文取计算区域 $D=(-10,10)^2$ $J=K=128$ $V({\bf x})=0.5(x^2+y^2)$ .
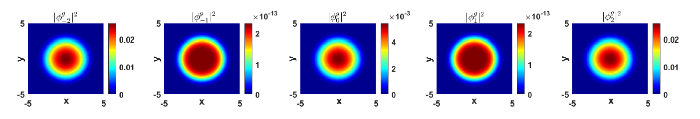
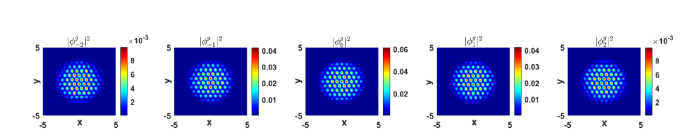
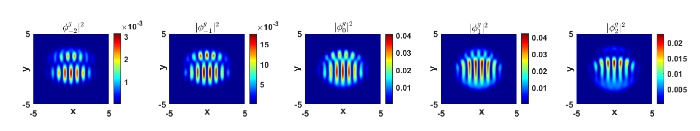
算例 1 (可行性测试) 取 $\beta_{0}=100$ $\beta_{1}=1$ $\beta_{2}=-2$ $M=0$ $\gamma=0$ . 应用 GFLM 方法求该参数下的基态解.
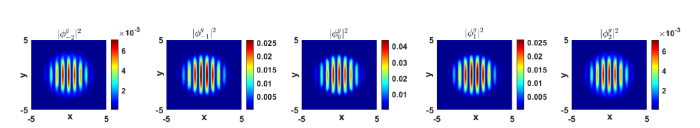
图1 是 GFLM 方法模拟算例 1 基态解的结果. 观察可得: (1) 基态解分量 $\phi_{-\ell}^g=\phi_{\ell}^g (\ell=1,2)$ $M=0$ $\phi_{-\ell}$ $\phi_{\ell}$ $\rm CGPEs$ $\phi_{-\ell}^g=\phi_{\ell}^g (\ell=1,2)$ $\gamma=0$
图1
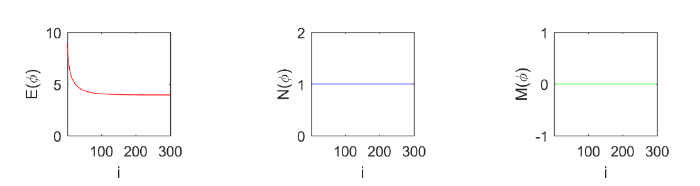
图2 表示计算过程中, 能量、总质量和总磁场量随迭代次数的变化情况. 观察可知: (1) 能量一直减少, 最后达到稳定状态; (2) 总质量 $N\equiv1$ $M\equiv0$
图2
图2
算例 1 中能量($E_{\phi}$ ) , 总质量($N_{\phi}$ ) 和总磁场量($M_{\phi}$ ) 随迭代次数的变化图
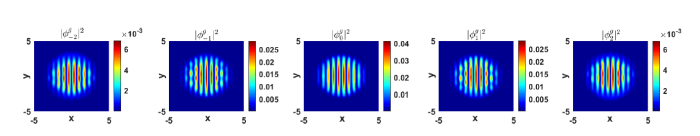
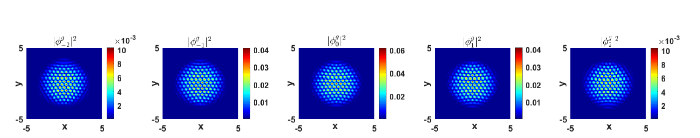
算例 2 (循环型体系下结果) 取 $\beta_{0}=100, \beta_{1}=1, \beta_{2}=2, M=0$ $\gamma=1.5, \gamma=2, \gamma=2.3, \gamma=2.4, \gamma=3, \gamma=4$
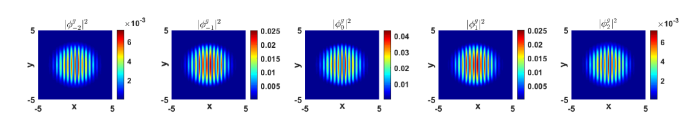
图3 -图8 是算例 2 中所得的具有循环型体系下自旋轨道耦合 Spin-2 BEC 基态. 观察可得: (1) 基态解分量 $\phi_{-\ell}^g$ $\phi_{\ell}^g (\ell=1,2)$ $M=0$ $\gamma$ $\gamma$
图3
图3
算例 2 中 $\gamma=1.5$
图4
图4
算例 2 中 $\gamma=2$
图5
图5
算例 2 中 $\gamma=2.3$
图6
图6
算例 2 中 $\gamma=2.4$
图7
图7
算例 2 中 $\gamma=3$
图8
图8
算例 2 中 $\gamma=4$
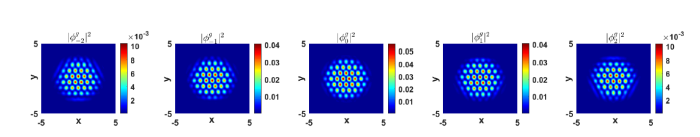
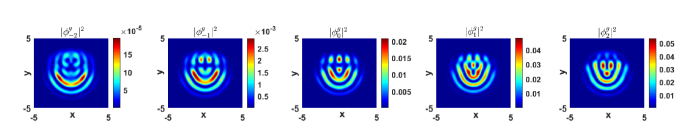
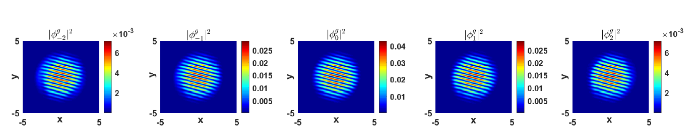
算例 3 (循环型体系下结果) 固定 $\beta_{0}=100, \beta_{1}=1, \beta_{2}=2$ $M=1, \gamma=1.5$ $M=1.2, \gamma=2$
图9 -图10 是算例 3 中所得自旋轨道耦合 Spin-2 BEC 的基态解. 观察可得: (1) $M\neq0$ $\phi_{-\ell}^g$ $\phi_{\ell}^g (\ell=1,2)$
图9
图9
算例 3 中 $M=1$ $\gamma=1.5$
图10
图10
算例 3 中 $M=1.2$ $\gamma=2$
由上可得: GFLM 方法可以有效模拟循环型体系下自旋轨道耦合 Spin-2 BEC 的基态解; 磁场量 $M=0$ $\gamma$
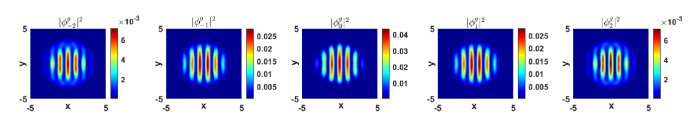
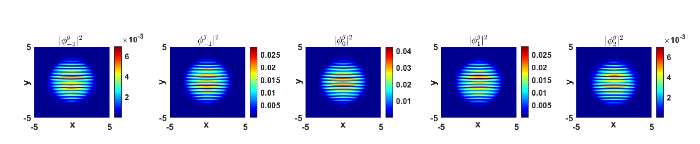
算例 4 (铁磁体系下结果) 取 $\beta_{0}=100, \beta_{1}=-1, \beta_{2}=-25, M=0$ $\gamma=1.5, \gamma=2, \gamma=3.2, \gamma=3.3, \gamma=3.5, \gamma=4$
图11 -图16 是算例 4 中所得自旋轨道耦合 Spin-2 BEC 的基态解. 观察可得: (1) 基态解分量 $\phi_{-\ell}^g$ $\phi_{\ell}^g (\ell=1,2)$ $M=0$ $\gamma$ $\gamma$
图11
图11
算例 4 中 $\gamma=1.5$
图12
图12
算例 4 中 $\gamma=2$
图13
图13
算例 4 中 $\gamma=3.2$
图14
图14
算例 4 中 $\gamma=3.3$
图15
图15
算例 4 中 $\gamma=3.5$
图16
图16
算例 4 中 $\gamma=4$
算例 5 (铁磁体系下结果) 固定 $\beta_{0}=100$ $\beta_{1}=-1$ $\beta_{2}=-25$ $M=0.6, \gamma=1.5$ $M=0.5, \gamma=2$
图17 -图18 是算例 5 中所得自旋轨道耦合强度的自旋轨道耦合 Spin-2 BEC 的基态解. 观察可得: (1) $M\neq0$ $\phi_{-\ell}^g$ $\phi_{\ell}^g (\ell=1,2)$
图17
图17
算例 5 中 $M=0.6$ $\gamma=1.5$
图18
图18
算例 5 中 $M=0.5$ $\gamma=2$
由上可得: GFLM 方法可有效模拟铁磁体系下自旋轨道耦合 Spin-2 BEC 的基态解; 磁场量 $M=0$ $\gamma$
参考文献
View Option
[1]
Anderson M H Ensher J R Matthewa M R et al. Observation of Bose-Einstein condensation in a dilute atomic vapor
Science , 1995 , 269 198 -201
PMID:17789847
[本文引用: 1]
A Bose-Einstein condensate was produced in a vapor of rubidium-87 atoms that was confined by magnetic fields and evaporatively cooled. The condensate fraction first appeared near a temperature of 170 nanokelvin and a number density of 2.5 x 10(12) per cubic centimeter and could be preserved for more than 15 seconds. Three primary signatures of Bose-Einstein condensation were seen. (i) On top of a broad thermal velocity distribution, a narrow peak appeared that was centered at zero velocity. (ii) The fraction of the atoms that were in this low-velocity peak increased abruptly as the sample temperature was lowered. (iii) The peak exhibited a nonthermal, anisotropic velocity distribution expected of the minimum-energy quantum state of the magnetic trap in contrast to the isotropic, thermal velocity distribution observed in the broad uncondensed fraction.
[2]
Antoine X Levitt A Tang Q Efficient spectral computation of the stationary states of rotating Bose-Einstein condensates by preconditioned nonlinear conjugate gradient methods
Journal of Computational Physics , 2017 , 343 92-109
[本文引用: 1]
[3]
Bao W Cai Y Mathematical models and numerical methods for spinor Bose-Einstein condensates
Communications in Computational Physics , 2018 , 24 899 -965
[本文引用: 1]
[4]
Bao W Du Q Computing the ground state solution of Bose-Einstein condensates by a normalized gradient flow
SIAM Journal on Scientific Computing , 2004 , 25 1674 -1697
DOI:10.1137/S1064827503422956
URL
[本文引用: 1]
[5]
Bao W Lim F Computing ground states of spin-1 Bose-Einstein condensates by the normalized gradient flow
SIAM Journal on Scientific Computing , 2008 , 30 1925 -1948
DOI:10.1137/070698488
URL
[本文引用: 1]
[6]
Bao W Tang W Ground state solution of Bose-Einstein condensate by directly minimizing the energy functional
Journal of Computational Physics , 2003 , 187 230 -254
DOI:10.1016/S0021-9991(03)00097-4
URL
[本文引用: 1]
[7]
Bao W Wang H A mass and magnetization conservervative and energy-diminishing numerical method for computing ground state of spin-1 Bose-Einstein condensates
SIAM Journal on Numerical Analysis , 2007 , 45 5 ): 2177 -2200
DOI:10.1137/070681624
URL
[本文引用: 1]
[8]
Bao W Wang H Markowich P A Ground, symmetric and central vortex states in rotating Bose-Einstein condensates
Communications in Mathematical Sciences , 2005 , 3 1 ): 57 -88
DOI:10.4310/CMS.2005.v3.n1.a5
URL
[本文引用: 1]
[10]
Bradley C C Sackett C A Tollett J J Hulet R G Evidence of Bose-Einstein condensation in an atomic gas with attractive interaction
Physical Review Letters , 1995 , 75 1687-1690
[本文引用: 1]
[11]
Cai Y Liu W Efficient and accurate gradient flow methods for computing ground states of spinor Bose-Einstein condensates
Journal of Computational Physics , 2021 , 433 110183
DOI:10.1016/j.jcp.2021.110183
URL
[本文引用: 3]
[12]
Davis K B Mewes M O Andrews M R et al. Bose-Einstein condensation in a gas of sodium atoms
Physical Review Letters , 1995 , 75 3969 -3973
PMID:10059782
[本文引用: 1]
[13]
Einstein A Quantentheorie des einatomigen idealen gases
Sitzungsberichte der Preussischen Akademie der Wissenschaften , 1924 , 22 261 -267
[本文引用: 1]
[14]
Einstein A Quantentheorie des einatomigen idealen gases, zweite abhandlung
Sitzungsberichte der Preussischen Akademie der Wissenschaften , 1925 , 1 3 -14
[本文引用: 1]
[17]
Liu W Cai Y Normalized gradient flow with Lagrange multiplier for computing ground states of Bose-Einstein condensates
SIAM Journal on Scientific Computing , 2021 , 43 1 ): B219 -B242
DOI:10.1137/20M1328002
URL
[本文引用: 2]
[18]
Lin Y J Compton R L Jimenez-Garcia K et al. Synthetic magmetic fields for ultracold neutral stoms
Nature , 2009 , 462 628 -632
DOI:10.1038/nature08609
[本文引用: 1]
[20]
Lin Y J Jimenez-Garcia K Spielman I B A spin-orbit-coupled Bose-Einstein condensates
Nature , 2011 , 471 83 -86
DOI:10.1038/nature09887
[本文引用: 1]
[21]
Stamper-Kurn D M Andrews M R Chikkatur A P et al. Optical confinement of a Bose-Einstein condensate
Physical Review Letters , 1998 , 80 2027 -2030
DOI:10.1103/PhysRevLett.80.2027
URL
[本文引用: 1]
[23]
Yuan Y Xu Z Tang Q Wang H The numerical study of the ground states of spin-1 Bose-Einstein condensates with spin-orbit-coupling
East Asian Journal on Applied Mathematics , 2018 , 8 3 ): 598 -610
DOI:10.4208/eajam
URL
[本文引用: 1]
Observation of Bose-Einstein condensation in a dilute atomic vapor
1
1995
... 玻色-爱因斯坦凝聚 (BEC) 被称为世界物质第五态, 是指玻色子在温度接近绝对零度时达到的物质状态. 它由玻色和爱因斯坦在 1924-1925 年预测[9 ,13 ,14 ] , 并于 1995 年首次在物理实验中实现[1 ,10 ,12 ] . ...
Efficient spectral computation of the stationary states of rotating Bose-Einstein condensates by preconditioned nonlinear conjugate gradient methods
1
2017
... 近年已有大量的数值方法模拟 BEC 的基态. Bao 等早期采用直接最小化能量泛函法来求解基态[6 ] . 随后, Bao 和 Du 提出了一种简单且广泛适用的方法, 即正规梯度流法[4 ] 来计算 BEC 的基态. 对于旋转单组份 BEC 模型, Bao 等采用向后欧拉有限差分离散的连续梯度流法[8 ] 研究了模型的基态, 对称态和中心涡态以及能量和化学势图. Antoine 和 Tang 等提出了效率更高的预条件共轭梯度法 (PCG)[2 ] . ...
Mathematical models and numerical methods for spinor Bose-Einstein condensates
1
2018
... 对于旋量 BEC 的基态模拟, Bao 和 Wang 为 Spin-1 BEC 构造了连续梯度流并对其采用 Crank-Nicolson 有限差分进行离散[7 ] . 随后, Bao 等利用离散正规梯度流法计算 Spin-1 和 Spin-2 BEC 的基态[3 ,5 ] , 其核心在于发掘投影系数间的隐含关系, 连同模型中的总质量和总磁场量守恒关系, 使得投影步骤所需的所有投影系数可被唯一确定. 此外, Cai 和 Liu 提出了模拟不带自旋轨道耦合项的 Spin-$\mathcal{F}$ [11 ,17 ] . ...
Computing the ground state solution of Bose-Einstein condensates by a normalized gradient flow
1
2004
... 近年已有大量的数值方法模拟 BEC 的基态. Bao 等早期采用直接最小化能量泛函法来求解基态[6 ] . 随后, Bao 和 Du 提出了一种简单且广泛适用的方法, 即正规梯度流法[4 ] 来计算 BEC 的基态. 对于旋转单组份 BEC 模型, Bao 等采用向后欧拉有限差分离散的连续梯度流法[8 ] 研究了模型的基态, 对称态和中心涡态以及能量和化学势图. Antoine 和 Tang 等提出了效率更高的预条件共轭梯度法 (PCG)[2 ] . ...
Computing ground states of spin-1 Bose-Einstein condensates by the normalized gradient flow
1
2008
... 对于旋量 BEC 的基态模拟, Bao 和 Wang 为 Spin-1 BEC 构造了连续梯度流并对其采用 Crank-Nicolson 有限差分进行离散[7 ] . 随后, Bao 等利用离散正规梯度流法计算 Spin-1 和 Spin-2 BEC 的基态[3 ,5 ] , 其核心在于发掘投影系数间的隐含关系, 连同模型中的总质量和总磁场量守恒关系, 使得投影步骤所需的所有投影系数可被唯一确定. 此外, Cai 和 Liu 提出了模拟不带自旋轨道耦合项的 Spin-$\mathcal{F}$ [11 ,17 ] . ...
Ground state solution of Bose-Einstein condensate by directly minimizing the energy functional
1
2003
... 近年已有大量的数值方法模拟 BEC 的基态. Bao 等早期采用直接最小化能量泛函法来求解基态[6 ] . 随后, Bao 和 Du 提出了一种简单且广泛适用的方法, 即正规梯度流法[4 ] 来计算 BEC 的基态. 对于旋转单组份 BEC 模型, Bao 等采用向后欧拉有限差分离散的连续梯度流法[8 ] 研究了模型的基态, 对称态和中心涡态以及能量和化学势图. Antoine 和 Tang 等提出了效率更高的预条件共轭梯度法 (PCG)[2 ] . ...
A mass and magnetization conservervative and energy-diminishing numerical method for computing ground state of spin-1 Bose-Einstein condensates
1
2007
... 对于旋量 BEC 的基态模拟, Bao 和 Wang 为 Spin-1 BEC 构造了连续梯度流并对其采用 Crank-Nicolson 有限差分进行离散[7 ] . 随后, Bao 等利用离散正规梯度流法计算 Spin-1 和 Spin-2 BEC 的基态[3 ,5 ] , 其核心在于发掘投影系数间的隐含关系, 连同模型中的总质量和总磁场量守恒关系, 使得投影步骤所需的所有投影系数可被唯一确定. 此外, Cai 和 Liu 提出了模拟不带自旋轨道耦合项的 Spin-$\mathcal{F}$ [11 ,17 ] . ...
Ground, symmetric and central vortex states in rotating Bose-Einstein condensates
1
2005
... 近年已有大量的数值方法模拟 BEC 的基态. Bao 等早期采用直接最小化能量泛函法来求解基态[6 ] . 随后, Bao 和 Du 提出了一种简单且广泛适用的方法, 即正规梯度流法[4 ] 来计算 BEC 的基态. 对于旋转单组份 BEC 模型, Bao 等采用向后欧拉有限差分离散的连续梯度流法[8 ] 研究了模型的基态, 对称态和中心涡态以及能量和化学势图. Antoine 和 Tang 等提出了效率更高的预条件共轭梯度法 (PCG)[2 ] . ...
Plancks gesetz und lichtquantenhypothese
1
1924
... 玻色-爱因斯坦凝聚 (BEC) 被称为世界物质第五态, 是指玻色子在温度接近绝对零度时达到的物质状态. 它由玻色和爱因斯坦在 1924-1925 年预测[9 ,13 ,14 ] , 并于 1995 年首次在物理实验中实现[1 ,10 ,12 ] . ...
Evidence of Bose-Einstein condensation in an atomic gas with attractive interaction
1
1995
... 玻色-爱因斯坦凝聚 (BEC) 被称为世界物质第五态, 是指玻色子在温度接近绝对零度时达到的物质状态. 它由玻色和爱因斯坦在 1924-1925 年预测[9 ,13 ,14 ] , 并于 1995 年首次在物理实验中实现[1 ,10 ,12 ] . ...
Efficient and accurate gradient flow methods for computing ground states of spinor Bose-Einstein condensates
3
2021
... 对于旋量 BEC 的基态模拟, Bao 和 Wang 为 Spin-1 BEC 构造了连续梯度流并对其采用 Crank-Nicolson 有限差分进行离散[7 ] . 随后, Bao 等利用离散正规梯度流法计算 Spin-1 和 Spin-2 BEC 的基态[3 ,5 ] , 其核心在于发掘投影系数间的隐含关系, 连同模型中的总质量和总磁场量守恒关系, 使得投影步骤所需的所有投影系数可被唯一确定. 此外, Cai 和 Liu 提出了模拟不带自旋轨道耦合项的 Spin-$\mathcal{F}$ [11 ,17 ] . ...
... 对于自旋轨道耦合旋量 BEC 模型, Yuan 等[23 ] 提出了投影梯度流法计算自旋轨道耦合 Spin-1 BEC 的基态. 该方法采用空间二阶有限差分和时间 Grank-Nicolson 方法离散连续梯度流, 具有总质量、总磁场量守恒及能量递减的性质. 然而, 自旋轨道耦合 Spin-2 BEC 模型具有复杂的自旋交换项和自旋单线态项, 其基态解在自旋轨道耦合效应下具有复杂的基态相, 可呈现细密的条纹状和晶格状两种图案. 这些特点对模拟自旋轨道耦合 Spin-2 BEC 基态的数值算法在精度与效率方面提出了新的挑战. 目前, 关于自旋轨道耦合 Spin-2 BEC 基态模拟的数值结果十分有限. 本文将带拉格朗日乘子的梯度流算法[11 ,17 ] 推广到自旋轨道耦合 Spin-2 BEC 的基态模拟. 该方法在每步迭代只需求解一个常系数椭圆方程(组), 易实施且效率高. ...
... 因此, 为唯一确定所有投影系数 $\alpha_{\ell}^{n} (\ell = -2,-1,0,1,2)$ [11 ] 中对 $(\alpha_{\ell}^{n})^2$
Bose-Einstein condensation in a gas of sodium atoms
1
1995
... 玻色-爱因斯坦凝聚 (BEC) 被称为世界物质第五态, 是指玻色子在温度接近绝对零度时达到的物质状态. 它由玻色和爱因斯坦在 1924-1925 年预测[9 ,13 ,14 ] , 并于 1995 年首次在物理实验中实现[1 ,10 ,12 ] . ...
Quantentheorie des einatomigen idealen gases
1
1924
... 玻色-爱因斯坦凝聚 (BEC) 被称为世界物质第五态, 是指玻色子在温度接近绝对零度时达到的物质状态. 它由玻色和爱因斯坦在 1924-1925 年预测[9 ,13 ,14 ] , 并于 1995 年首次在物理实验中实现[1 ,10 ,12 ] . ...
Quantentheorie des einatomigen idealen gases, zweite abhandlung
1
1925
... 玻色-爱因斯坦凝聚 (BEC) 被称为世界物质第五态, 是指玻色子在温度接近绝对零度时达到的物质状态. 它由玻色和爱因斯坦在 1924-1925 年预测[9 ,13 ,14 ] , 并于 1995 年首次在物理实验中实现[1 ,10 ,12 ] . ...
Spinor Bose condensates in optical traps
1
1998
... 在早期实验中, 玻色子在磁势阱中不能发生自旋. 1998 年, 利用光学偶极子阱, 人们首次在自旋 $^{23}\mathrm{Na}$ [21 ] . 在光阱中, 不同超精细态的粒子在空间中具有不同的角动量, 从而产生丰富的自旋结构. 自旋量子数为 $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ [15 ,16 ,22 ] . 特别地, 由于粒子自旋和粒子运动的相互影响会产生自旋轨道耦合 (SOC) 效应, 近年, Lin 等[18 ,19 ,20 ] 在中性原子的 BEC 实验中, 成功诱导了自旋轨道耦合旋量 BEC. ...
Spinor Bose-Einstein condensates
1
2012
... 在早期实验中, 玻色子在磁势阱中不能发生自旋. 1998 年, 利用光学偶极子阱, 人们首次在自旋 $^{23}\mathrm{Na}$ [21 ] . 在光阱中, 不同超精细态的粒子在空间中具有不同的角动量, 从而产生丰富的自旋结构. 自旋量子数为 $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ [15 ,16 ,22 ] . 特别地, 由于粒子自旋和粒子运动的相互影响会产生自旋轨道耦合 (SOC) 效应, 近年, Lin 等[18 ,19 ,20 ] 在中性原子的 BEC 实验中, 成功诱导了自旋轨道耦合旋量 BEC. ...
Normalized gradient flow with Lagrange multiplier for computing ground states of Bose-Einstein condensates
2
2021
... 对于旋量 BEC 的基态模拟, Bao 和 Wang 为 Spin-1 BEC 构造了连续梯度流并对其采用 Crank-Nicolson 有限差分进行离散[7 ] . 随后, Bao 等利用离散正规梯度流法计算 Spin-1 和 Spin-2 BEC 的基态[3 ,5 ] , 其核心在于发掘投影系数间的隐含关系, 连同模型中的总质量和总磁场量守恒关系, 使得投影步骤所需的所有投影系数可被唯一确定. 此外, Cai 和 Liu 提出了模拟不带自旋轨道耦合项的 Spin-$\mathcal{F}$ [11 ,17 ] . ...
... 对于自旋轨道耦合旋量 BEC 模型, Yuan 等[23 ] 提出了投影梯度流法计算自旋轨道耦合 Spin-1 BEC 的基态. 该方法采用空间二阶有限差分和时间 Grank-Nicolson 方法离散连续梯度流, 具有总质量、总磁场量守恒及能量递减的性质. 然而, 自旋轨道耦合 Spin-2 BEC 模型具有复杂的自旋交换项和自旋单线态项, 其基态解在自旋轨道耦合效应下具有复杂的基态相, 可呈现细密的条纹状和晶格状两种图案. 这些特点对模拟自旋轨道耦合 Spin-2 BEC 基态的数值算法在精度与效率方面提出了新的挑战. 目前, 关于自旋轨道耦合 Spin-2 BEC 基态模拟的数值结果十分有限. 本文将带拉格朗日乘子的梯度流算法[11 ,17 ] 推广到自旋轨道耦合 Spin-2 BEC 的基态模拟. 该方法在每步迭代只需求解一个常系数椭圆方程(组), 易实施且效率高. ...
Synthetic magmetic fields for ultracold neutral stoms
1
2009
... 在早期实验中, 玻色子在磁势阱中不能发生自旋. 1998 年, 利用光学偶极子阱, 人们首次在自旋 $^{23}\mathrm{Na}$ [21 ] . 在光阱中, 不同超精细态的粒子在空间中具有不同的角动量, 从而产生丰富的自旋结构. 自旋量子数为 $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ [15 ,16 ,22 ] . 特别地, 由于粒子自旋和粒子运动的相互影响会产生自旋轨道耦合 (SOC) 效应, 近年, Lin 等[18 ,19 ,20 ] 在中性原子的 BEC 实验中, 成功诱导了自旋轨道耦合旋量 BEC. ...
Bose-Einstein condensates in a uniform light-induced vector potential
1
2009
... 在早期实验中, 玻色子在磁势阱中不能发生自旋. 1998 年, 利用光学偶极子阱, 人们首次在自旋 $^{23}\mathrm{Na}$ [21 ] . 在光阱中, 不同超精细态的粒子在空间中具有不同的角动量, 从而产生丰富的自旋结构. 自旋量子数为 $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ [15 ,16 ,22 ] . 特别地, 由于粒子自旋和粒子运动的相互影响会产生自旋轨道耦合 (SOC) 效应, 近年, Lin 等[18 ,19 ,20 ] 在中性原子的 BEC 实验中, 成功诱导了自旋轨道耦合旋量 BEC. ...
A spin-orbit-coupled Bose-Einstein condensates
1
2011
... 在早期实验中, 玻色子在磁势阱中不能发生自旋. 1998 年, 利用光学偶极子阱, 人们首次在自旋 $^{23}\mathrm{Na}$ [21 ] . 在光阱中, 不同超精细态的粒子在空间中具有不同的角动量, 从而产生丰富的自旋结构. 自旋量子数为 $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ [15 ,16 ,22 ] . 特别地, 由于粒子自旋和粒子运动的相互影响会产生自旋轨道耦合 (SOC) 效应, 近年, Lin 等[18 ,19 ,20 ] 在中性原子的 BEC 实验中, 成功诱导了自旋轨道耦合旋量 BEC. ...
Optical confinement of a Bose-Einstein condensate
1
1998
... 在早期实验中, 玻色子在磁势阱中不能发生自旋. 1998 年, 利用光学偶极子阱, 人们首次在自旋 $^{23}\mathrm{Na}$ [21 ] . 在光阱中, 不同超精细态的粒子在空间中具有不同的角动量, 从而产生丰富的自旋结构. 自旋量子数为 $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ [15 ,16 ,22 ] . 特别地, 由于粒子自旋和粒子运动的相互影响会产生自旋轨道耦合 (SOC) 效应, 近年, Lin 等[18 ,19 ,20 ] 在中性原子的 BEC 实验中, 成功诱导了自旋轨道耦合旋量 BEC. ...
Spinor Bose gases: Symmetries, magnetism, and quantum dynamics
1
2013
... 在早期实验中, 玻色子在磁势阱中不能发生自旋. 1998 年, 利用光学偶极子阱, 人们首次在自旋 $^{23}\mathrm{Na}$ [21 ] . 在光阱中, 不同超精细态的粒子在空间中具有不同的角动量, 从而产生丰富的自旋结构. 自旋量子数为 $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ $\mathcal{F}$ [15 ,16 ,22 ] . 特别地, 由于粒子自旋和粒子运动的相互影响会产生自旋轨道耦合 (SOC) 效应, 近年, Lin 等[18 ,19 ,20 ] 在中性原子的 BEC 实验中, 成功诱导了自旋轨道耦合旋量 BEC. ...
The numerical study of the ground states of spin-1 Bose-Einstein condensates with spin-orbit-coupling
1
2018
... 对于自旋轨道耦合旋量 BEC 模型, Yuan 等[23 ] 提出了投影梯度流法计算自旋轨道耦合 Spin-1 BEC 的基态. 该方法采用空间二阶有限差分和时间 Grank-Nicolson 方法离散连续梯度流, 具有总质量、总磁场量守恒及能量递减的性质. 然而, 自旋轨道耦合 Spin-2 BEC 模型具有复杂的自旋交换项和自旋单线态项, 其基态解在自旋轨道耦合效应下具有复杂的基态相, 可呈现细密的条纹状和晶格状两种图案. 这些特点对模拟自旋轨道耦合 Spin-2 BEC 基态的数值算法在精度与效率方面提出了新的挑战. 目前, 关于自旋轨道耦合 Spin-2 BEC 基态模拟的数值结果十分有限. 本文将带拉格朗日乘子的梯度流算法[11 ,17 ] 推广到自旋轨道耦合 Spin-2 BEC 的基态模拟. 该方法在每步迭代只需求解一个常系数椭圆方程(组), 易实施且效率高. ...
