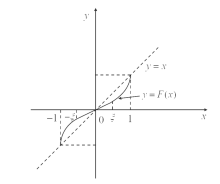
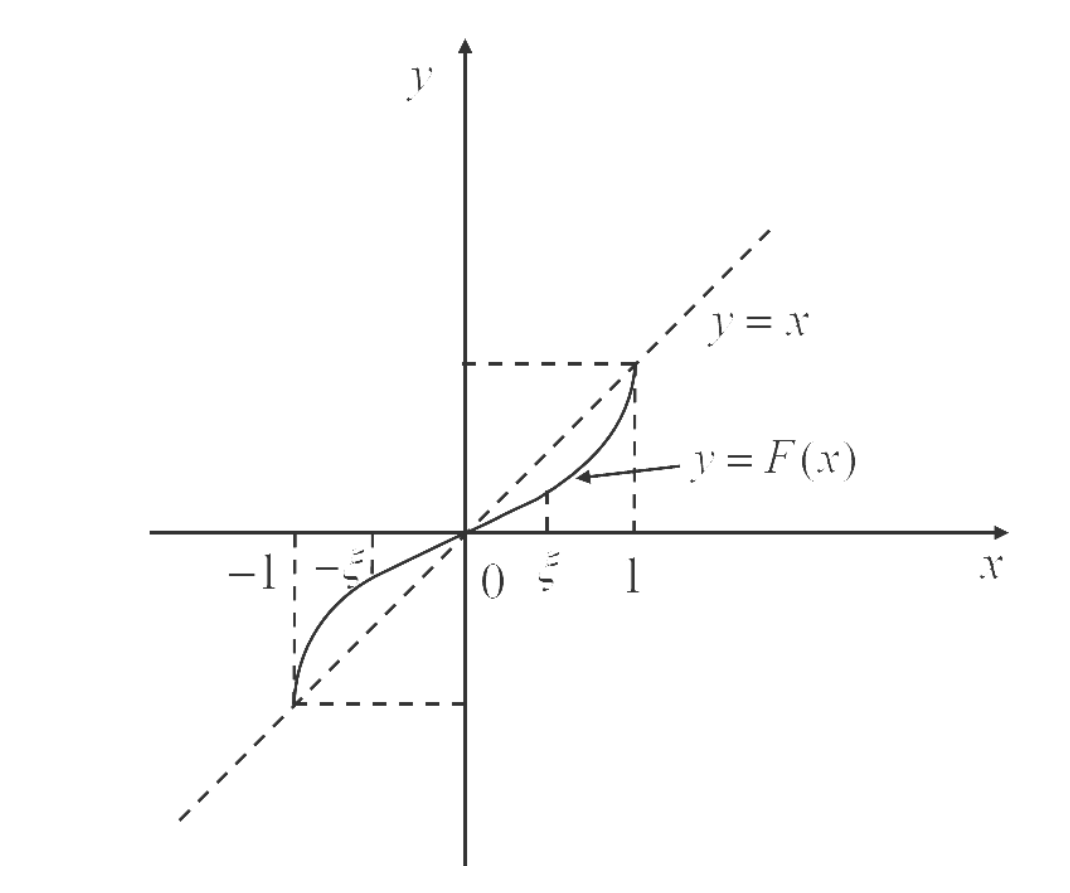
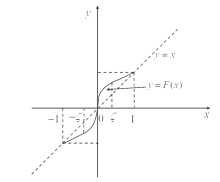
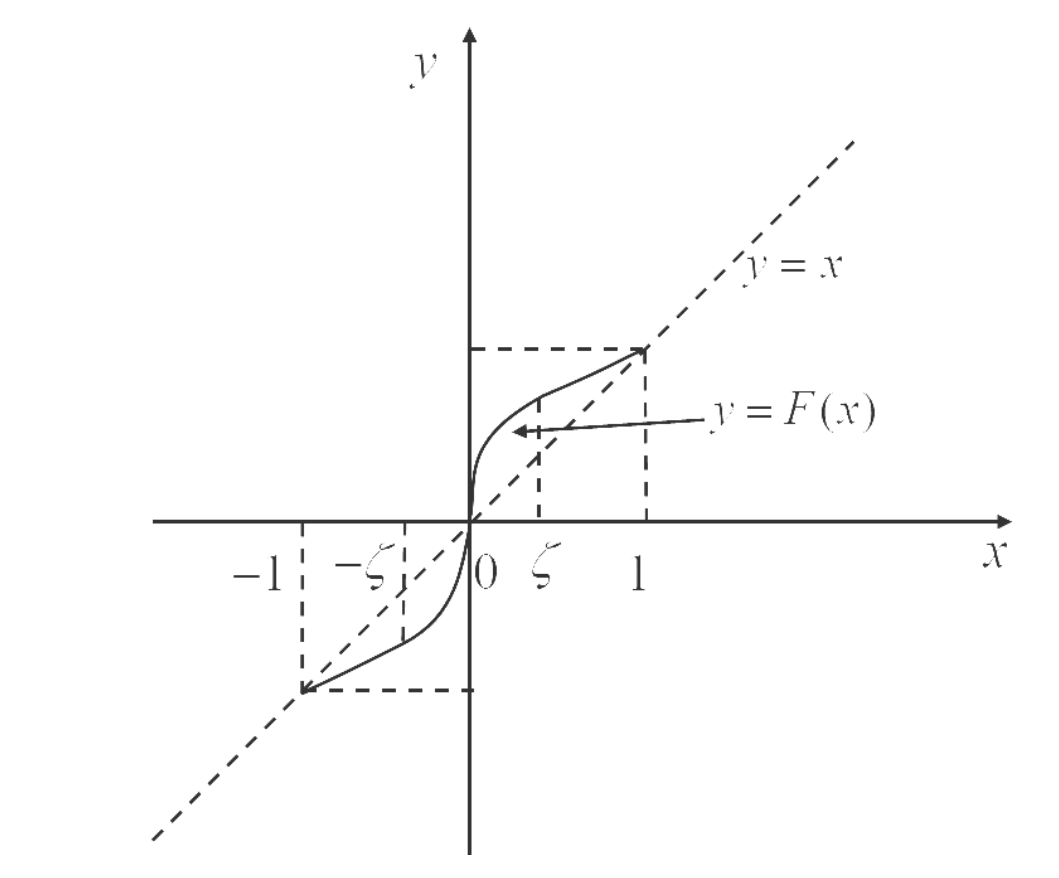
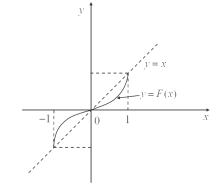
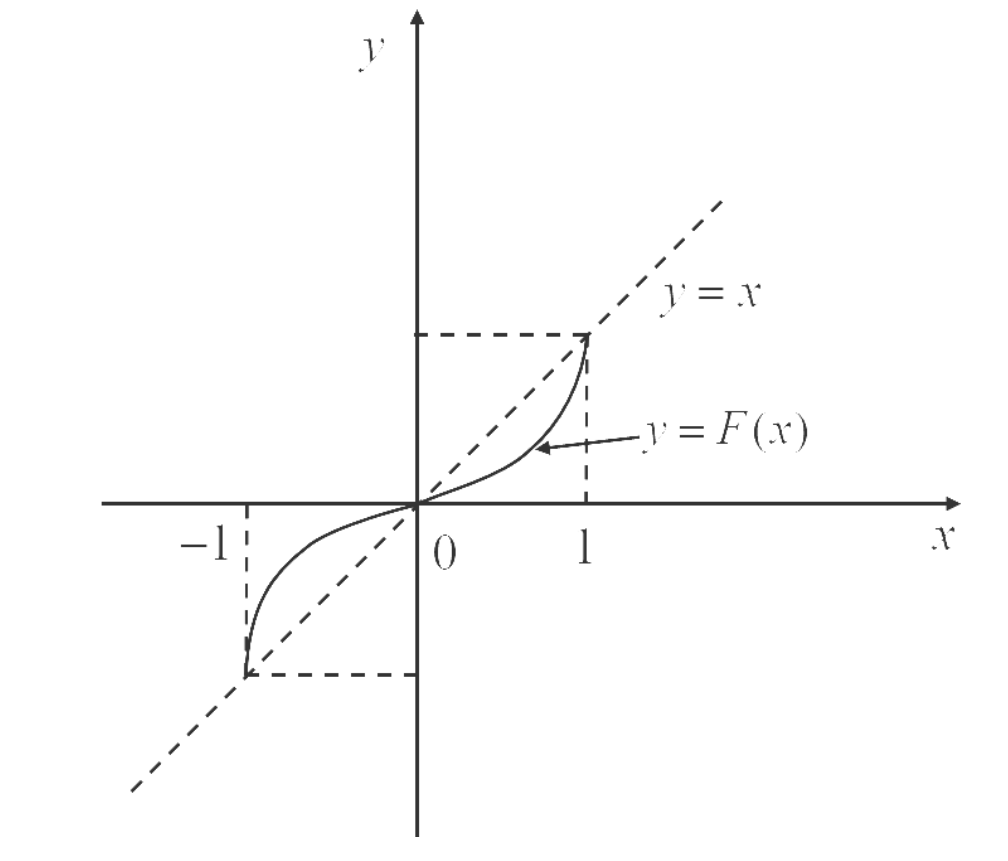
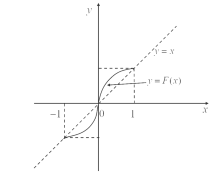
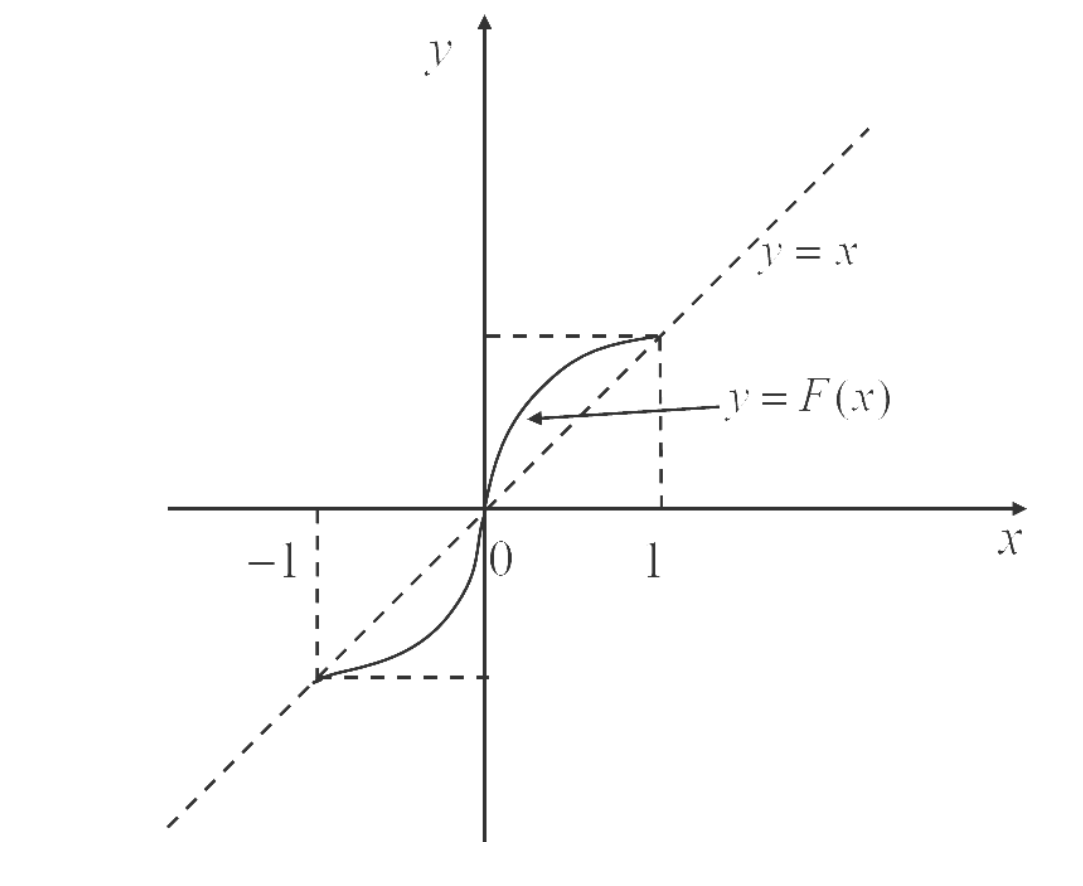
Acta mathematica scientia,Series A ›› 2024, Vol. 44 ›› Issue (2): 313-325.
Previous Articles Next Articles
Homeomorphic Solutions of Iterative Functional Equations
- 1School of Mathematics and Statistics, Hanshan Normal University, Guangdong Chaozhou 521041
2Faculty of Mathematics, Jiaxing University, Zhejiang Jiaxing 314001
-
Received:2022-08-11Revised:2023-10-14Online:2024-04-26Published:2024-04-07 -
Supported by:Zhejiang Provincial Natural Science(LY18A010017);National Natural Science Foundatjion of China(12026207);Doctoral Initiation Project of Hanshan Normal University(QD202210)
CLC Number:
- O175.23
Cite this article
Liu Jinghua, Li Lin. Homeomorphic Solutions of Iterative Functional Equations[J].Acta mathematica scientia,Series A, 2024, 44(2): 313-325.
share this article
| [1] | Baron K, Jarczyk W. Recent results on functional equations in a single variable perspectives and open problems. Aequationes Math, 2001, 61: 1-48 |
| [2] | Bessis D, Marmi S, Turchetti G. On the singularities of divergent majorant series arising from normal form theory. Rend Mat Appl, 1989, 9: 645-659 |
| [3] | Block L, Coven M. Topological conjugacy and transitivity for a class of piecewise monotone maps of the interval. Trans Amer Math Soc, 1987, 300: 297-306 |
| [4] | Chen Jingmin, Zhang Weinian. Leading coefficient problem for polynomial-like iterative equations. J Math Anal Appl, 2009, 349: 413-419 |
| [5] | Draga S, Morawiec J. Reducing the polynomial-like iteative equations order and a generalized Zoltán Boros' Problem. Aequationes Math, 2016, 90: 935-950 |
| [6] | Draga S, Morawiec J. Mean of iterates. Aequationes Math, 2019, 93: 21-35 |
| [7] | Fontich E. Transversal homoclinic points of a class of conservative diffeomorphisms. J Differential Equations, 1990, 87: 1-27 |
| [8] | Greenfield S J, Nussbaum R D. Dynamics of a quadratic map in two complex variables. J Differential Equations, 2001, 169: 57-141 |
| [9] |
Hu J, Sullivan D P. Topological conjugacy of circle diffeomorphisms. Ergodic Theory Dyn Syst, 1997, 17(1): 173-186
doi: 10.1017/S0143385797061002 |
| [10] | Jarczyk W. On an equation of linear iteration. Aequationes Math, 1996, 51: 303-310 |
| [11] | Kuczma M, Choczewski B, Ger R. Iterative Functional Equations. Encyclopedia Math Appl, Vol 32. Cambridge:Cambridge University Press, 1990 |
| [12] | Lanford O. A computer-assisted proof of the Feigenbaum conjectures. Bull Amer Math Soc (NS), 1982, 6: 427-434 |
| [13] |
Li L, Song W. Global solutions for leading coefficient problem of a general iterative equation. Results Math, 2015, 68(1/2): 247-260
doi: 10.1007/s00025-014-0432-0 |
| [14] | Li L, Zhang W M. Continuously decreasing solutions for polynomial-like iterative equations. Sci China Ser A, 2013, 56: 1051-1058 |
| [15] |
Li M R, Zhao H Y. Strongly convex solutions of polynomial-like iterative equation. J Math Anal Appl, 2021, 495(2): 124786
doi: 10.1016/j.jmaa.2020.124786 |
| [16] | Liu J H. Analytical invariant curves for a second order difference equation modeled from macroeconomics under the Brjuno condition. J Difference Equ Appl, 2017, 3: 648-656 |
| [17] | Liu J H, Yu Z H, Zhang W N. Invariant curves for a second order difference equation modeled from macroeconomics. J Difference Equ Appl, 2015, 9: 757-773 |
| [18] | Matkowski J, Weinian Z. Method of characteristic for function equations in polynomial form. Acta Math Sinica, 1997, 13: 421-432 |
| [19] | McCarthy P. The general exact bijective continuous solution of Feigenbaum's functional equation. Comm Math Phys, 1983, 91: 431-443 |
| [20] | Nabeya S. On the functional equation f(p+qx+rf(x))=a+bx+cf(x). Aequationes Math, 1974, 11: 199-211 |
| [21] | Parry W. Symbolic dynamics and transformations of the unit interval. Trans Amer Math Soc, 1966, 122: 368-378 |
| [22] | Song W, Li L. Continuously decreasing solutions for a general iterative equation. Acta Mathematica Scientia, 2018, 38B: 177-186 |
| [23] | Tabor J, Tabor J. On a linear iterative equation. Results Math, 1995, 27: 412-421 |
| [24] | Xu B, Zhang W N. Construction of continuous solutions and stability for the polynomial-like iterative equation. J Math Anal Appl, 2007, 325: 1160-1170 |
| [25] | Yang D L, Zhang W N. Characteristic solutions of polynomial-like iterative equations. Aequationes Math, 2004, 67: 80-105 |
| [26] | Yang L, Zhang J Z, The second type of Feigenbaum's functional equation. Sci China Ser A, 1986, 29: 1252-1262 |
| [27] | Zhang J Z, Yang L, Zhang W N. Some advances on functional equations. Adv Math (China), 1995, 24: 385-405 |
| [28] | Zhang W N. Discussion on the iterated equation Σni=1λifi(x)=F(x). Chinese Sci Bull, 1987, 32: 1444-1451 |
| [29] | Zhang W N. Discussion on the differentiable solutions of the iterated equation Σni=1λifi(x)=F(x). Nonlinear Anal, 1990, 15: 387-398 |
| [30] | Zhang W N, Baker J. Continuous solutions of a polynomial-like iterative equation with variable coefficients. Ann Polon Math, 2000, 73: 29-36 |
| [31] | Zhang W N, Nikodem K, Xu B. Convex solutions of polynomial-like iterative equations. J Math Anal Appl, 2006, 315: 29-40 |
| [32] | Zhang W M, Xu B, Zhang W N. Global solutions for leading coefficient problem of polynomial-like iterative equations. Results Math, 2013, 63: 79-93 |
| [33] | Zhang W M, Zhang W N. On continuous solutions of n-th order polynomial-like iterative equations. Publ Math Debrecen, 2010, 76: 117-134 |
| [34] |
Zhang W M, Zhang W N. C1 linearization for planar contractions. J Funct Anal, 2011, 260: 2043-2063
doi: 10.1016/j.jfa.2010.12.029 |
| [35] | Zhang W M, Zhang W N, Jarczyk W. Sharp regularity of linearization for C1,1 hyperbolic diffeomorphisms. Math Ann, 2014, 358: 69-113 |
| [1] | Zhiheng Yu,Xiaobing Gong. Polynomial Solutions of the Polynomial-Like Iterative Equation [J]. Acta mathematica scientia,Series A, 2019, 39(6): 1352-1364. |
| [2] | YANG Yu-Bo, ZHU Peng, YIN Yun-Hui. A Optimal Uniformly Convergent Discontinuous Galerkin Finite Element Method for Singularly Perturbed Problem [J]. Acta mathematica scientia,Series A, 2014, 34(3): 716-726. |
| [3] | YANG Zheng, JI Yuan-Yuan, MA He-Ping. Uniform Convergence of the Fourier Spectral Method for the Zakharov Equations [J]. Acta mathematica scientia,Series A, 2013, 33(3): 409-423. |
| [4] | YUN Dong-Fang, HUANG Shu-Xiang. On a Class of Singular Equations with Nonlinear Terms Depending on the Gradient [J]. Acta mathematica scientia,Series A, 2012, 32(5): 861-878. |
| [5] | ZHONG Ji-Yu, LI Xiao-Pei. On Set-valued Solutions of an Iterative Equation [J]. Acta mathematica scientia,Series A, 2011, 31(4): 970-977. |
| [6] | TU Tian-Liang, MO Jiong. Interpolatory Approximation to Harmonic Function with Boundary Data [J]. Acta mathematica scientia,Series A, 2010, 30(2): 397-404. |
| [7] | Peng Zhigang. The Properties of Several Classes of Analytic Functions with Missing Coefficients [J]. Acta mathematica scientia,Series A, 2008, 28(4): 661-669. |
| [8] | BANG Zhi-Gang, SU Feng-. Extreme Points and Support Points of a Family of Analytic Func tions [J]. Acta mathematica scientia,Series A, 2005, 25(3): 345-348. |
| [9] | Zhu Zhongyi, Wei Bocheng. Strong Uniform Convergence Rates for Nonparametric Estimations of Conditional Functional and its Derivatives [J]. Acta mathematica scientia,Series A, 1998, 18(4): 394-401. |
|