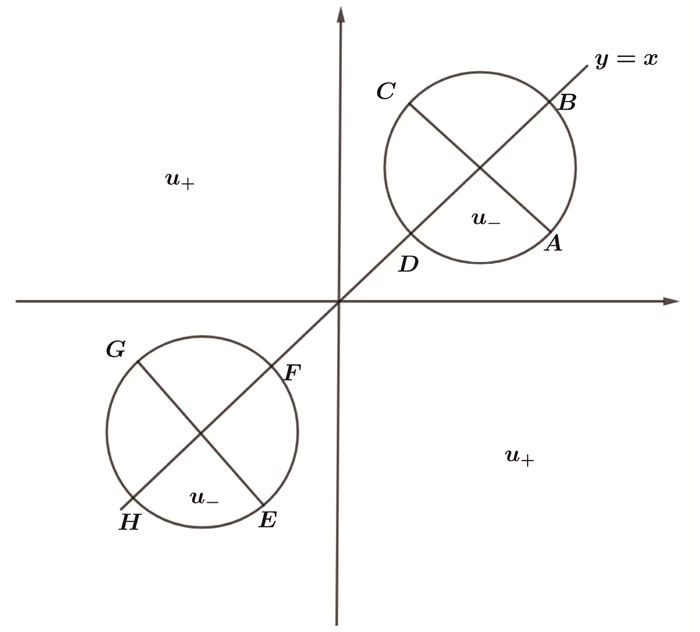
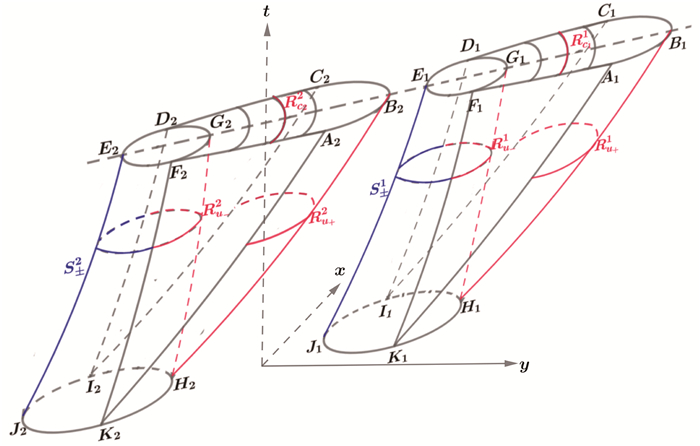
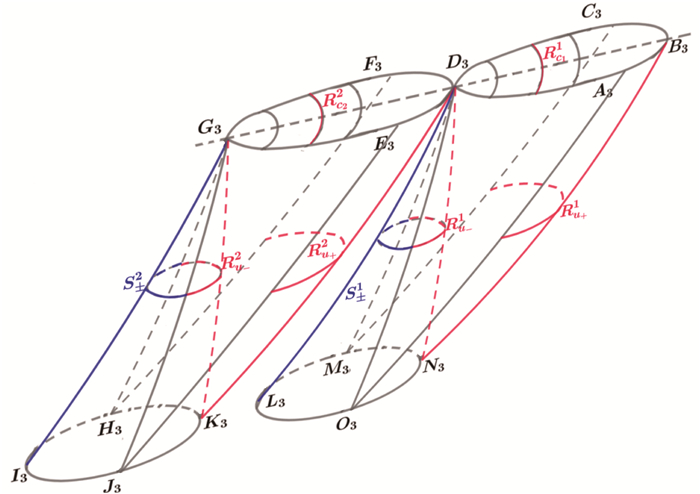
Acta mathematica scientia,Series A ›› 2022, Vol. 42 ›› Issue (4): 1122-1149.
Previous Articles Next Articles
Global Singular Structures of Non-Selfsimilar Riemann Solutions for Two Dimensional Non-homogeneous Burgers Equation
Yuan-an Zhao1,2( ),Gaowei Cao2,*(
),Gaowei Cao2,*( ),Xiaozhou Yang2(
),Xiaozhou Yang2( )
)
- 1 University of Chinese Academy of Sciences, Beijing 100049
2 Innovation Academy for Precision Measurement Science and Technology, Chinese Academy of Sciences, Wuhan 430071
-
Received:2021-05-27Online:2022-08-26Published:2022-08-08 -
Contact:Gaowei Cao E-mail:824141695@qq.com;gwcao@apm.ac.cn;xzyang@wipm.ac.cn -
Supported by:the NSFC(11701551);the NSFC(11971024);the NSFC(11471332)
CLC Number:
- O175
Cite this article
Yuan-an Zhao,Gaowei Cao,Xiaozhou Yang. Global Singular Structures of Non-Selfsimilar Riemann Solutions for Two Dimensional Non-homogeneous Burgers Equation[J].Acta mathematica scientia,Series A, 2022, 42(4): 1122-1149.
share this article
| 1 |
Conway E , Smoller J . Uniqueness and stability theorem for the generalized solution of the initial-value problem for a class of quasi-linear equations in several sapce variables. Arch Ration Mech Anal, 1967, 23 (5): 399- 408
doi: 10.1007/BF00276782 |
| 2 | Conway E , Smoller J . Global solutions of the Cauchy problem for Quasi-linear first-order equations in several spaces variables. Comm Pure Appl Math, 1996, 19: 95- 105 |
| 3 | Chang T , Hsiao L . The Riemann problem and interaction of waves in Gas Dynamics. NASA STI/Recon Technical Report A, 1989, 90: 40- 44 |
| 4 |
Chen G Q , Li D , Tan D . Structure of Riemann solutions for 2-dimensional scalar conservation laws. J Differential Equations, 1996, 127 (1): 124- 147
doi: 10.1006/jdeq.1996.0065 |
| 5 |
Cao G W , Xiang W , Yang X Z . Global structure of admissible solutions of multi-dimensional non-homogeneous scalar conservation law with Riemann-type data. J Differential Equations, 2017, 263 (2): 1055- 1078
doi: 10.1016/j.jde.2017.03.007 |
| 6 |
Guckenheimer J . Shocks and rarefactions in two space dimensions. Arch Ranon Mech Anal, 1975, 59 (3): 281- 291
doi: 10.1007/BF00251604 |
| 7 |
Kruzkov S N . First order quasilinear equations in several independent variables. Math USSR Sb, 1970, 10 (2): 217- 243
doi: 10.1070/SM1970v010n02ABEH002156 |
| 8 |
Liu T P . Nonlinear resonance for quasilinear hyperbolic equation. Journal of mathematical physics, 1987, 28 (11): 2593- 2602
doi: 10.1063/1.527751 |
| 9 |
Murray J D . On the Gunn effect and other physical examples of perturbed conservation equations. Journal of Fluid Mechanics, 1970, 44 (2): 315- 346
doi: 10.1017/S0022112070001854 |
| 10 |
Sheng W . Two-dimensional Riemann problem for scalar conservation laws. Journal of Differential Equations, 2002, 183 (1): 239- 261
doi: 10.1006/jdeq.2001.4124 |
| 11 |
Volpert A I . The space BV and quasilinear equations. Maths USSR Sbornik, 1967, 2: 225- 267
doi: 10.1070/SM1967v002n02ABEH002340 |
| 12 |
Wagner D H . The Riemann problem in two space dimensions for a single conservation law. SIAM Journal on Mathematical Analysis, 1983, 14 (3): 534- 559
doi: 10.1137/0514045 |
| 13 |
Yang X Z . Multi-dimensional Riemann problem of scalar conservation law. Acta Mathematica Scientia, 1999, 19 (2): 190- 200
doi: 10.1016/S0252-9602(17)30629-X |
| 14 | Yang X Z . The singular structure of non-selfsimilar global solutions of n dimensional Burgers equation. Acta Mathematica Applicatae Sinica, English Series, 2005, 3: 505- 518 |
| 15 | Yang X Z , Zhang T . Global smooth solution of multi-dimensional non-homogeneous conservation laws. Progress in Natural Science, 2004, 14 (10): 855- 862 |
| 16 | Zhang P , Zhang T . Generalized characteristic analysis and Guckenheimer structure. Journal of differential equations, 1999, 152 (2): 409- 430 |
| 17 | Zhang T , Zheng Y X . Two-dimensional Riemann problem for a single conservation law. Transactions of the American Mathematical Society, 1989, 312 (2): 589- 619 |
| [1] | Zhiqiang Shao. Concentration and Cavitation in the Pressureless Limit of Euler Equations of Compressible Fluid Flow with Damping and Friction [J]. Acta mathematica scientia,Series A, 2022, 42(4): 1150-1172. |
| [2] | Ning-An LAI, Wei XIANG, Yi ZHOU. GLOBAL INSTABILITY OF MULTI-DIMENSIONAL PLANE SHOCKS FOR ISOTHERMAL FLOW [J]. Acta mathematica scientia,Series A, 2022, 42(3): 887-902. |
| [3] | Jia Jia. The Two-Dimensional Steady Chaplygin Gas Flows Passing a Straight Wedge [J]. Acta mathematica scientia,Series A, 2021, 41(5): 1270-1282. |
| [4] | Yufeng Chen,Tingting Chen,Zhen Wang. The Existence of the Measure Solution for the Non-Isentropic Chaplygin Gas [J]. Acta mathematica scientia,Series A, 2020, 40(4): 833-841. |
| [5] | Qingling Zhang,Ying Ba. The Perturbed Riemann Problem for the Pressureless Euler Equations with a Flocking Dissipation [J]. Acta mathematica scientia,Series A, 2020, 40(1): 49-62. |
| [6] | Lijun Pan,Xinli Han,Tong Li. The Generalized Riemann Problem for Chromatography Equations with Delta Shock Wave [J]. Acta mathematica scientia,Series A, 2019, 39(6): 1300-1313. |
| [7] | Chen Tingting, Qu Aifang, Wang Zhen. The Two-Dimensional Riemann Problem for Isentropic Chaplygin Gas [J]. Acta mathematica scientia,Series A, 2017, 37(6): 1053-1061. |
| [8] | Li Huahui, Shao Zhiqiang. Vanishing Pressure Limit of Riemann Solutions to the Aw-Rascle Model for Generalized Chaplygin Gas [J]. Acta mathematica scientia,Series A, 2017, 37(5): 917-930. |
| [9] | Li Huahui, Shao Zhiqiang. Interactions of Delta Shock Waves for the Nonsymmetric Keyfitz-Kranzer System with Split Delta Functions [J]. Acta mathematica scientia,Series A, 2017, 37(4): 714-729. |
| [10] | SHAO Zhi-Qiang. The Riemann Problem with Delta Initial Data for the Aw-Rascle Traffic Model with Chaplygin Pressure [J]. Acta mathematica scientia,Series A, 2014, 34(6): 1353-1371. |
| [11] | LIU Xiao-Min, WANG Zhen. The Riemann Problem for a System of Nonlinear Elasticity of |Mixed Type [J]. Acta mathematica scientia,Series A, 2013, 33(5): 801-818. |
| [12] | ZHANG Ting-Ting. The Stability of the Riemann Solutions for Aw-Rascle Model [J]. Acta mathematica scientia,Series A, 2013, 33(2): 230-241. |
| [13] | Liu Fagui; Ge Yunfei; Li Caizhong. Linear Hyperbolic Systems with Discontinuous Coefficients [J]. Acta mathematica scientia,Series A, 2007, 27(1): 184-192. |
| [14] | CHEN Shou-Xin. Global Discontinuous Solutions to a Class of Generalized Riemann Problems for Quasilinear Hyperbolic Systems [J]. Acta mathematica scientia,Series A, 2003, 23(4): 419-430. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 157
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 75
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|