不等式的推广以及在加性时变时滞系统中的应用

RichHTML

PDF (PC)

Generalization of Inequality and Its Application in Additive Time-Varying Delay Systems

Abstract

Figures/Tables 6

References 28

Metrics

Comments

Recommended 10

Abstract

Cite this article

share this article

Figures/Tables 6

References 28

Related Articles 2

Metrics

Comments

Recommended 10

Abstract:

This article mainly studies the improvement problem of integral inequality and applies it to the stability study of additive time-varying delay systems: Firstly, the existing Wirtinger inequality is proven from the perspectives of monotonicity and adding positive terms using the method of constructing parameter functions. Secondly, we propose extended inequalities, including the extended Wirtinger inequality and the extended cross-convex inequality which is based on third-order matrices. Thirdly, utilizing these two extended inequalities, we provide criteria for determining the asymptotic stability of additive time-varying delay systems in the form of Linear Matrix Inequalities (LMIs). Finally, numerical examples are presented to demonstrate the effectiveness and superiority of the proposed method.

Key words: Additive time-varying delay system, Wirtinger inequality, Stability analysis

CLC Number:

O211

Fan Tianjiao, Feng Lichao, Yang Yanmei. Generalization of Inequality and Its Application in Additive Time-Varying Delay Systems[J].Acta mathematica scientia,Series A, 2024, 44(5): 1335-1351.

Trendmd

[1]	Chen D, Liu X W, Song Y L. Stability analysis of discrete-time system with slowly time-varying delays. Procedia Computer Science, 2022, 199: 1008-1015 doi: 10.1016/j.procs.2022.01.127
[2]	Cai L, Xiong L L, Zhang H Y. A generalized multiple integral inequality with application to time-varying delay systems. Procedia Computer Science, 2022, 199: 1268-1275
[3]	Fridman E. New Lyapunov-Krasovskii functionals for stability of linear retarded and neutral type systems. Systems and Control Letters, 2001, 43(4): 309-319
[4]	Gong C, Zhang X, Wu L G. Multiple-integral inequalities to stability analysis of linear time-delay systems. Journal of the Franklin Institute, 2017, 354(3): 1446-1463
[5]	González A. Improved results on stability analysis of time-varying delay systems via delay partitioning method and Finsler's lemma. Journal of the Franklin Institute, 2022, 359(14): 7632-7649
[6]	Gouaisbaut F, Peaucelle D. Delay-dependent stability analysis of linear time delay systems. IFAC Proceedings Volumes, 2006, 39(10): 54-59
[7]	Gyurkovics E. A note on Wirtinger-type integral inequalities for time-delay systems. Automatica, 2015, 61: 44-46
[8]	Han Q L. A discrete delay decomposition approach to stability of linear retarded and neutral systems. Automatica, 2009, 45(2): 517-524
[9]	Ji Y D, Ma X T, Wang L Y, et al. Novel stability criterion for linear system with two additive time-varying delays using general integral inqualities. AIMS Mathematics, 2021, 6(8): 8667-8680
[10]	Jin L, He Y, Jiang L. A novel integral inequality and its application to stability analysis of linear system with multiple time delays. Applied Mathematics Letters, 2022, 124: 107648
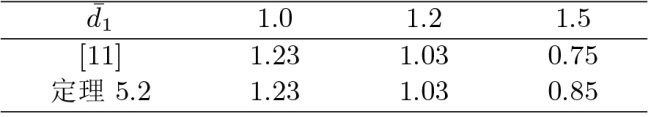
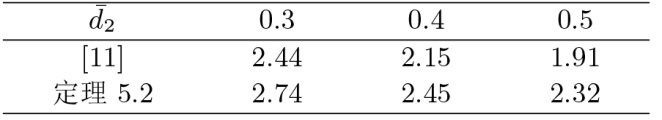
[11]	Jiao J M, Zhang R. An extended reciprocally convex matrix inequality and its application to stability analysis of systems with additive time-varying delays. Journal of the Franklin Institute, 2020, 357(4): 2282-2294
[12]	Li H F, Zhou B, Hou M Z, et al. On the time-varying Halanay inequality with applications to stability analysis of time-delay systems. Journal of the Franklin Institute, 2021, 358(10): 5488-5512
[13]	Liu K, Seuret A, Xia Y Q. Stability analysis of systems with time-varying delays via the second-order Bessel-Legendre inequality. Automatica, 2017, 76: 138-142
[14]	Liu P L. A delay decomposition approach to robust stability analysis of uncertain systems with time-varying delay. ISA Transactions, 2012, 51(6): 694-701
[15]	Park I, Lee J H, Park P G. New free-matrix-based integral inequality: Application to stability analysis of systems with additive time-varying delays. IEEE Access, 2020, 8: 125680-125691
[16]	Park P G, Ko J W, Jeong C K. Reciprocally convex approach to stability of systems with time-varying delays. Automatica, 2011, 47(1): 235-238
[17]	Seuret A, Gouaisbaut F. Jensen's and Wirtinger's inequalities for time-delay systems. IFAC Proceedings Volumes, 2013, 46(3): 343-348
[18]	Seuret A, Gouaisbaut F. Wirtinger-based integral inequality: Application to time-delay systems. Automatica, 2013, 49(9): 2860-2866
[19]	Tian J K, Ren Z R, Zhong S M. A new integral inequality and application to stability of time-delay systems. Applied Mathematics Letters, 2020, 101: 106058
[20]	Wu M, He Y, She J H. New delay-dependent stability criteria and stabilizing method for neutral systems. IEEE Transactions on Automatic Control, 2004, 49(12): 2266-2271
[21]	Wang C, Shen Y. Improved delay-dependent robust stability criteria for uncertain time delay systems. Applied Mathematics and Computation, 2011, 218(6): 2880-2888
[22]	Yang B, Yan Z F, Pan X J, et al. Improved stability criteria for linear systems with time-varying delays. Journal of the Franklin Institute, 2021, 358(15): 7804-7824 doi: 10.1016/j.jfranklin.2021.07.045
[23]	Zeng H B, Lin H C, He Y, et al. Hierarchical stability conditions for time-varying delay systems via an extended reciprocally convex quadratic inequality. Journal of the Franklin Institute, 2020, 357(14): 9930-9941
[24]	Zhi Y L, He Y, Zhang C K, et al. New method for stability of systems with time-varying delay via improved free-matrix-based integral inequality. IFAC-PapersOnLine, 2017, 50(1): 1281-1285
[25]	Zhao X, Lin C, Chen B, et al. Stability analysis for linear time-delay systems using new inequality based on the second-order derivative. Journal of the Franklin Institute, 2019, 356(15): 8770-8784 doi: 10.1016/j.jfranklin.2019.03.038
[26]	Zhang C K, He Y, Jiang L, et al. An improved summation inequality to discrete-time systems with time-varying delay. Automatica, 2016, 74: 10-15
[27]	Zhang H G, Liu Z W. Stability analysis for linear delayed systems via an optimally dividing delay interval approach. Automatica, 2011, 47(9): 2126-2129
[28]	Zhang X M, Han Q L, Seuret A, et al. An improved reciprocally convex inequality and an augmented Lyapunov-Krasovskii functional for stability of linear systems with time-varying delay. Automatica, 2017, 84: 221-226

Viewed

Full text

	From	local

	Times	33
	Rate	100%

Abstract

Just accepted	Online first	Issue

0	0	40

	From	Others

	Times	41
	Rate	100%

Cited

Web of Science	Crossref	ScienceDirect	Search for Citations in Google Scholar >>


This page requires you have already subscribed to WoS.

Shared

Discussed

[1]	Han Zhongjie, He Yiheng, Zhao Zhixue. Indirect Stabilization and Optimal Decay Rates of Weakly Coupled Plates with Various Types of Damping[J]. Acta mathematica scientia,Series A, 2023, 43(6): 1681 -1698 .
[2]	He Fen, Wang Zhen. Riemann Problem for a Class of Mixed-type Chemotaxis Models[J]. Acta mathematica scientia,Series A, 2024, 44(2): 354 -360 .
[3]	Chen Hao, Deng Xuemei, Bie Qunyi. Anisotropic Liouville Type Theorem for the Stationary Nematic Liquid Crystal Equations in $\mathbb{R}^{3}$ [J]. Acta mathematica scientia,Series A, 2024, 44(3): 661 -669 .
[4]	Chen Shangjie. Infinitely Many Large Energy Solutions for the Klein-Gordon-Born-Infeld Equation on $\mathbf{R}^3$ with Concave and Convex Nonlinearities[J]. Acta mathematica scientia,Series A, 2024, 44(3): 637 -649 .
[5]	Chang Jinhua, Wei Na. On the Schrödinger-Poisson System with Asymptotically Linear Term and Coulomb Potential[J]. Acta mathematica scientia,Series A, 2024, 44(3): 650 -660 .
[6]	Ding Xuanhao, Shao Changhui, Li Yongning. The Product of Volterra Operator and Toeplitz Operator[J]. Acta mathematica scientia,Series A, 2024, 44(4): 829 -836 .
[7]	Liu Wei, Xu Meizhen. Eigenvalues of a Class of Second-Order Differential Operator with Eigenparameters Dependent Internal Point Conditions[J]. Acta mathematica scientia,Series A, 2024, 44(4): 815 -828 .
[8]	Chen Junyou, Zhang Zhenliang. On Hausdorff Dimension of the Exceptional Sets of Partial Maximal Digits for Lüroth Expansion[J]. Acta mathematica scientia,Series A, 2024, 44(4): 847 -858 .
[9]	Jin Zhenfeng, Sun Hongrui, Zhang Weimin. Multiplicity and Asymptotic Behavior of Normalized Solutions for Kirchhoff-Type Equation[J]. Acta mathematica scientia,Series A, 2024, 44(4): 871 -884 .
[10]	Qian Yuting, Zhou Xueliang, Cheng Zhibo. Existence of Periodic Solutions for $\phi$ -Laplacian Rayleigh Equations with a Singularity[J]. Acta mathematica scientia,Series A, 2024, 44(5): 1183 -1193 .

Just accepted

Online first

Just accepted

Online first

[1]	Rui Bin,Xingxing Wang,Chunna Zeng. The Bonnesen-type Inequalities for Plane Closed Curves [J]. Acta mathematica scientia,Series A, 2022, 42(6): 1601-1610.
[2]	Meng Deng,Rui Xu. Stability Analysis of an HIV Infection Dynamic Model with CTL Immune Response and Immune Impairment [J]. Acta mathematica scientia,Series A, 2022, 42(5): 1592-1600.