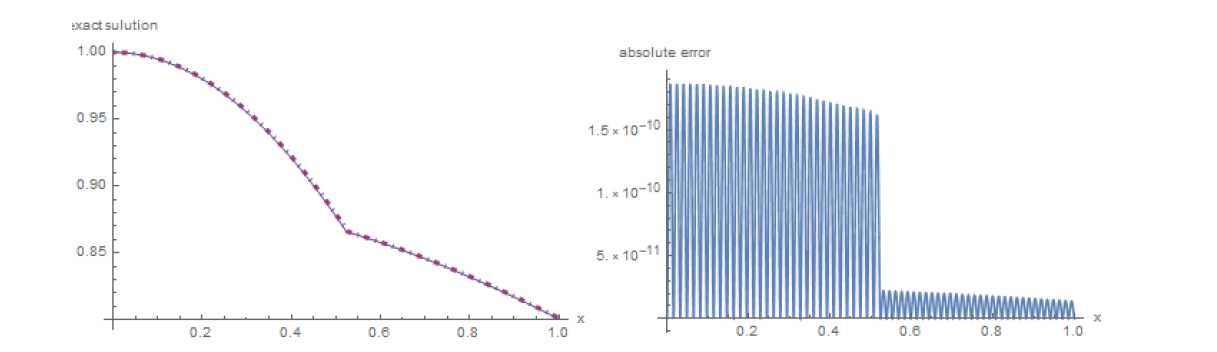
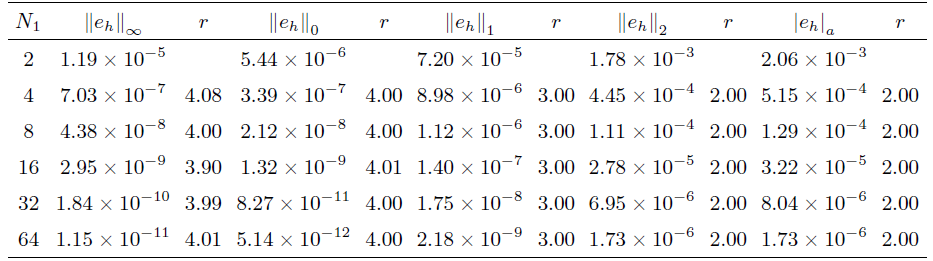
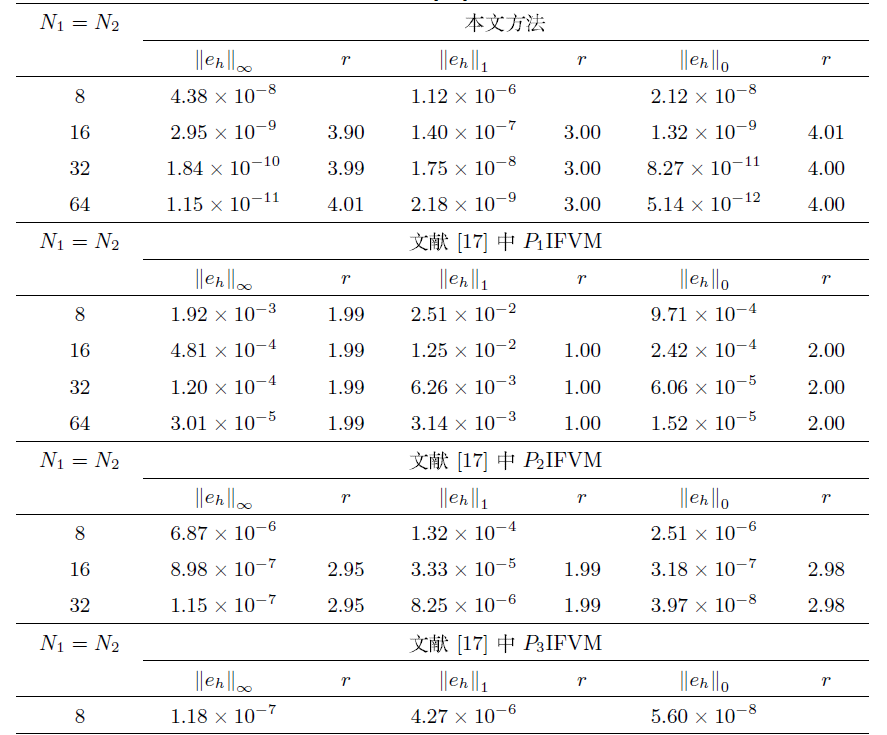
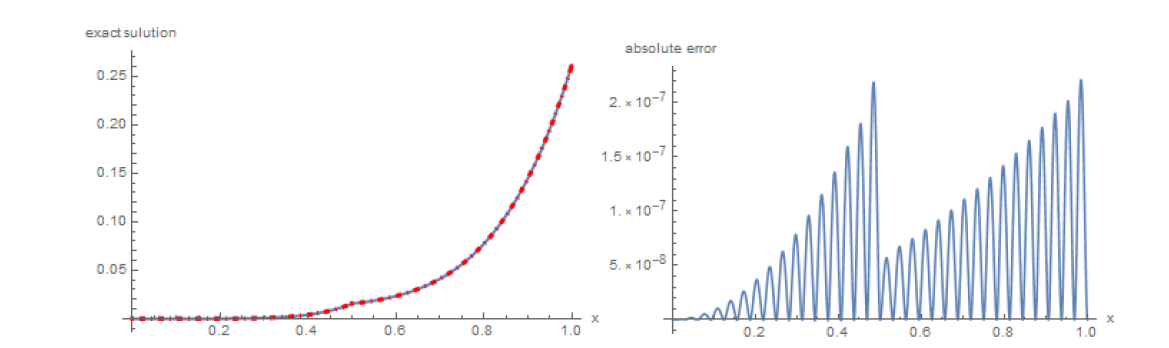
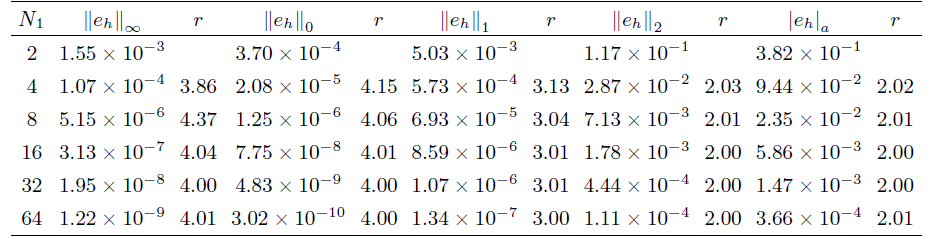
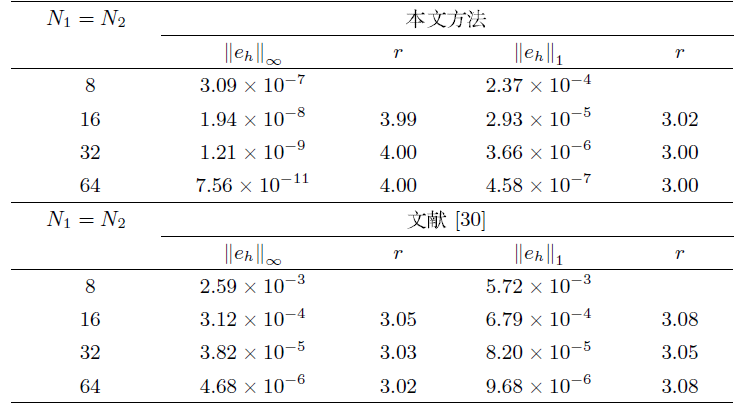
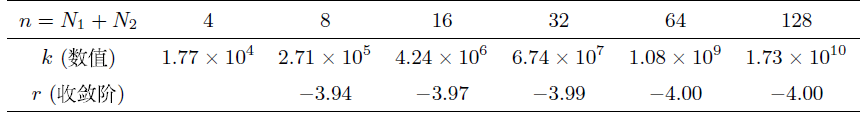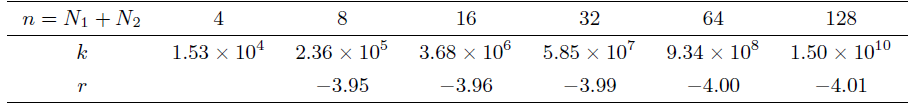Acta mathematica scientia,Series A ›› 2023, Vol. 43 ›› Issue (3): 883-895.
Previous Articles Next Articles
A Kind of Numerical Algorithm for Elliptic Interface Problem
- School of Mathematics and Sciences, Harbin Normal University, Harbin 150025
-
Received:2022-11-16Revised:2023-01-12Online:2023-06-26Published:2023-06-01 -
Contact:Jing Niu E-mail:qq63192678@126.com -
Supported by:Youth Fund of NSFC(12101164);Postgraduate Innovative Scientific Research Project of Harbin Normal University(HSDSSCX2022-40)
CLC Number:
- O241.8
Cite this article
Yu Yikang,Niu Jing. A Kind of Numerical Algorithm for Elliptic Interface Problem[J].Acta mathematica scientia,Series A, 2023, 43(3): 883-895.
share this article
| [1] |
Greengard L, Moura M. On the numerical evaluation of electrostatic fields in composite materials. Acta Numer, 1994, 3: 379-410
doi: 10.1017/S0962492900002464 |
| [2] | Peskin C. Numerical analysis of blood flow in the heart. J Comput Phys, 1977, 25: 220-252 |
| [3] |
Al-Smadi M, Arqub O A. Computational algorithm for solving Fredholm time-fractional partial integrodifferential equations of Dirichlet functions type with error estimates. Appl Math Comput, 2019, 342: 280-294
doi: 10.1016/j.amc.2018.09.020 |
| [4] |
Al-Smadi M, Arqub O A, Shawagfeh N, Momani S. Numerical investigations for systems of second-order periodic boundary value problems using reproducing kernel method. Appl Math Comput, 2016, 291: 137-148
doi: 10.1016/j.amc.2016.06.002 |
| [5] |
Albright J, Epshteyn Y, Medvinsky M, Xia Q. High-order numerical schemes based on difference potentials for 2D elliptic problems with material interfaces. Appl Numer Math, 2017, 111: 64-91
doi: 10.1016/j.apnum.2016.08.017 |
| [6] |
Babuska I, Caloz G, Osborn J. Special finite element methods for a class of second order elliptic problems with rough coefficients. SIAM J Numer Anal, 1994, 31: 945-981
doi: 10.1137/0731051 |
| [7] |
Epshteyn Y, Phippen S. High-order difference potentials methods for 1D elliptic type models. Appl Numer Math, 2015, 93: 69-86
doi: 10.1016/j.apnum.2014.02.005 |
| [8] |
Hellrung J, Wang L, Sifakis E, Teran J. A second order virtual node method for elliptic problems with interfaces and irregular domains in three dimensions. J Comput Phys, 2012, 231: 2015-2048
doi: 10.1016/j.jcp.2011.11.023 |
| [9] |
Lee C, Park J. Preconditioning for finite element methods with strain smoothing. Computers & Mathematics with Applications, 2023, 130: 41-57
doi: 10.1016/j.camwa.2022.11.018 |
| [10] |
Kalachikova U, Vasilyeva M, Harris I, et al. Generalized Multiscale Finite Element Method for scattering problem in heterogeneous media. J Comput Appl Math, 2023, 424: 114977
doi: 10.1016/j.cam.2022.114977 |
| [11] |
Guzman J, Sanchez M, Sarkis M. On the accuracy of finite element approximations to a class of interface problems. Math Comput, 2016, 85: 2071-2098
doi: 10.1090/mcom/2016-85-301 |
| [12] | Harari I, Dolbow J. Analysis of an efficient finite element method for embedded interface problems. Comput Math, 2010, 46: 205-211 |
| [13] |
Dong B Y, Feng X F, Lin Z L. An FE-FD method for anisotropic elliptic interface problems. SIAM J Sci Comput, 2020, 42: B1041-B1066
doi: 10.1137/19M1291030 |
| [14] |
Pan K J, Wu X X, Xu Y F, Yuan G W. An exact-interface-fitted mesh generator and linearity-preserving finite volume scheme for anisotropic elliptic interface problems. J Comput Phys, 2022, 463: 111293
doi: 10.1016/j.jcp.2022.111293 |
| [15] |
Cao W, Zhang X, Zhang Z. Superconvergence of immersed finite element methods for interface problems. Adv Comput Math, 2017, 43: 795-821
doi: 10.1007/s10444-016-9507-7 |
| [16] | He X, Lin T, Lin Y. Immersed finite element methods for elliptic interface problems with non-homogeneous jump conditions. Int J Numer Anal Model, 2011, 8: 284-301 |
| [17] |
Cao W, Zhang X, Zhang Z, Zou Q. Superconvergence of immersed finite volume methods for one-dimensional interface problems. J Sci Comput, 2017, 73: 543-565
doi: 10.1007/s10915-017-0532-6 |
| [18] | Liu K, Zou Q. Analysis of a special immersed finite volume method for elliptic interface problems. Int J Numer Anal Model, 2019, 16: 964-984 |
| [19] |
Liu W, Jun S, Zhang Y. Reproducing kernel particle method. Int J Numer Methods Fluids, 1995, 20: 1081-1106
doi: 10.1002/(ISSN)1097-0363 |
| [20] |
Yang J, Guan P, Fan C. Weighted reproducing kernel collocation method and error analysis for inverse Cauchy problems. Int J Appl Mech, 2016, 8: 1650030
doi: 10.1142/S1758825116500307 |
| [21] |
Yang J, Hsin W. Weighted reproducing kernel collocation method based on error analysis for solving inverse elasticity problems. Acta Mech, 2019, 230: 3477-3497
doi: 10.1007/s00707-019-02473-0 |
| [22] | Chen J, Wang H, Hu H. Reproducing kernel enhanced local radial basis collocation method. Int J Numer Methods Eng, 2008, 80: 600-627 |
| [23] |
Niu J, Xu M, Yao G M. An efficient reproducing kernel method for solving the allen-cahn equation. Appl Math Lett, 2019, 89: 78-84
doi: 10.1016/j.aml.2018.09.013 |
| [24] |
Niu J, Sun L X, Xu M Q, Hou J J. A reproducing kernel method for solving heat conduction equations with delay. Appl Math Lett, 2020, 100: 106036
doi: 10.1016/j.aml.2019.106036 |
| [25] |
Niu J, Jia Y T, Sun J D. A new piecewise reproducing kernel function algorithm for solving nonlinear hamiltonian systems. Appl Math Lett, 2023, 136: 108451
doi: 10.1016/j.aml.2022.108451 |
| [26] |
Sun L X, Niu J, Hou J J. A high order convergence collocation method based on the reproducing kernel for general interface problems. Appl Math Lett, 2021, 112: 106718
doi: 10.1016/j.aml.2020.106718 |
| [27] |
Yu Y, Niu J, Zhang J, Ning S Y. A reproducing kernel method for nonlinear C-q-fractional IVPs. Appl Math Lett, 2022, 125: 107751
doi: 10.1016/j.aml.2021.107751 |
| [28] | Yu Y K, Niu J, Yang X M, Cui M G. A broken reproducing kernel method for the multiple interface problems, Comput Appl Math, 2022, 41: 260 |
| [29] | 吴勃英, 林迎珍. 应用型再生核空间. 北京: 科学出版社, 2012 |
| Wu B, Lin Y Z. Applied Regenerative Nuclear Space. Beijing: Science Press, 2012 | |
| [30] |
杨学敏, 牛晶, 姚春华. 椭圆型界面问题的破裂再生核方法. 计算数学, 2022, 44(2): 217-232
doi: 10.12286/jssx.j2021-0791 |
|
Yang X M, Niu J, Yao C H. The method of fracture regeneration core for elliptical interface problem. Computational Mathematics, 2022, 44(2): 217-232
doi: 10.12286/jssx.j2021-0791 |
|
| [31] |
Hall C A, Meyer W W. Optimal error bounds for cubic spline interpolation. J Approx Theory, 1976, 16: 105-122
doi: 10.1016/0021-9045(76)90040-X |
| [32] |
Xu M, Zhao Z, Niu J, Lin Y. A simplified reproducing kernel method for 1-D elliptic type interface problems. J Comput Appl Math, 2019, 351: 29-40
doi: 10.1016/j.cam.2018.10.027 |
| [33] | 林群, 谢和虎. 有限元Aubin-Nitsche技巧新认识及其应用. 数学的实践与认识, 2011, 41(17): 247-258 |
| Lin Q, Xie H. An observation on Aubin-Nitsche lemma and its applications. Mathematics in Practice and Theory, 2011, 41(17): 247-258 |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 301
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 98
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|