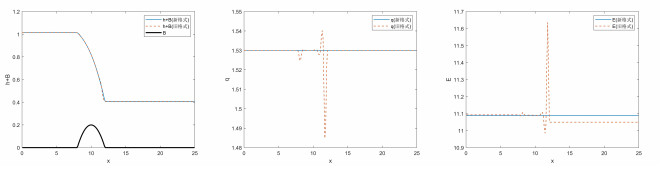
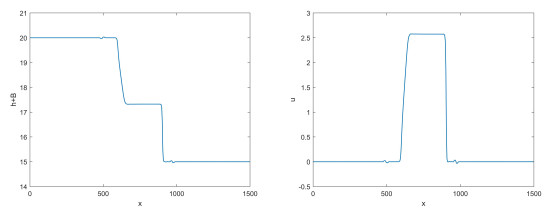
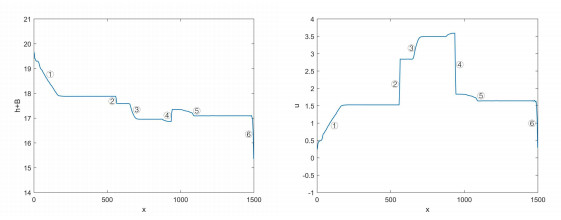
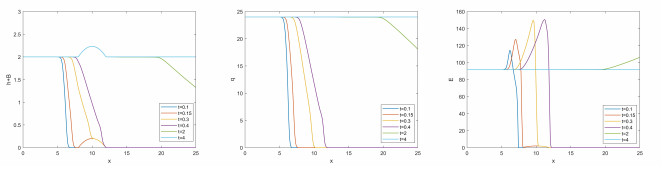
Acta mathematica scientia,Series A ›› 2022, Vol. 42 ›› Issue (3): 891-903.
Previous Articles Next Articles
Moving-Water Equilibria Preserving Central Scheme for the Saint-Venant System
Yiming Luo1( ),Dingfang Li1(
),Dingfang Li1( ),Min Liu1(
),Min Liu1( ),Jian Dong2,*(
),Jian Dong2,*( )
)
- 1 School of Mathematics and Statistics, Wuhan University, Wuhan 430072
2 College of Liberal Arts and Sciences, National University of Defense Technology, Changsha 410073
-
Received:2021-01-11Online:2022-06-26Published:2022-05-09 -
Contact:Jian Dong E-mail:luoyiming@whu.edu.cn;dfli@whu.edu.cn;liumin@whu.edu.cn;j.dong@whu.edu.cn -
Supported by:the National Key Research and Development Project(2017YFC0405901)
CLC Number:
- O241.82
Cite this article
Yiming Luo,Dingfang Li,Min Liu,Jian Dong. Moving-Water Equilibria Preserving Central Scheme for the Saint-Venant System[J].Acta mathematica scientia,Series A, 2022, 42(3): 891-903.
share this article
| 1 | Saint-Venant De . Théorie du mouvement non-permanent des eaux avec application aux crues des rivires et à lintroduction des marées dans leur lit. Comptes Rendus Hebdomadaires Des Séances De Lacadémie Des Sciences, 1871, 73 (99): 148- 154 |
| 2 |
Nessyahu H , Tadmor E . Non-oscillatory central differencing for hyperbolic conservation laws. J Comput Phys, 1990, 87 (2): 408- 463
doi: 10.1016/0021-9991(90)90260-8 |
| 3 | Touma R . Central unstaggered finite volume schemes for hyperbolic systems: applications to unsteady shallow water equations. Appl Math Comput, 2009, 213 (1): 47- 59 |
| 4 |
Touma R . Well-balanced central schemes for systems of shallow water equations with wet and dry states. Appl Math Model, 2016, 40 (4): 2929- 2945
doi: 10.1016/j.apm.2015.09.073 |
| 5 |
Dong J , Li D F . An effect non-staggered central scheme based on new hydrostatic reconstruction. Appl Math Comput, 2019, 372
doi: 10.1016/j.amc.2019.124992 |
| 6 |
Cheng Y Z , Kurganov A . Moving-water equilibria preserving central-upwind schemes for the shallow water equations. Commun Math Sci, 2016, 14 (6): 1643- 1663
doi: 10.4310/CMS.2016.v14.n6.a9 |
| 7 |
Cheng Y Z , Chertock A , Herty M , et al. A new approach for designing moving-water equilibria preserving schemes for the shallow water equations. J Sci Comput, 2019, 80 (1): 538- 554
doi: 10.1007/s10915-019-00947-w |
| 8 |
Liu X , Chen X , Jin S , et al. Moving-water equilibria preserving partial relaxation scheme for the Saint-Venant system. SIAM J Sci Comput, 2020, 42 (4): A2206- A2229
doi: 10.1137/19M1258098 |
| 9 |
Noelle S , Xing Y L , Shu C W . High-order well-balanced finite volume WENO schemes for shallow water equation with moving water. J Comput Phys, 2007, 226 (1): 29- 58
doi: 10.1016/j.jcp.2007.03.031 |
| 10 | Xing Y L , Shu C W , Noelle S . On the advantage of well-balanced schemes for moving-water equilibria of the shallow water equations. J Sci Comput, 2011, 48 (1): 339- 349 |
| 11 |
Xing Y L . Exactly well-balanced discontinuous Galerkin methods for the shallow water equations with moving water equilibrium. J Comput Phys, 2014, 257, 536- 553
doi: 10.1016/j.jcp.2013.10.010 |
| 12 |
Kurganov A , Petrova G . A second-order well-balanced positivity preserving central-upwind scheme for the Saint-Venant system. Commun Math Sci, 2007, 5 (1): 133- 160
doi: 10.4310/CMS.2007.v5.n1.a6 |
| 13 |
Chen G X , Noelle S . A new hydrostatic reconstruction scheme based on subcell reconstructions. SIAM J Numer Anal, 2017, 55 (2): 758- 784
doi: 10.1137/15M1053074 |
| 14 |
Jiang G S , Levy D , Lin C T , et al. High-resolution nonoscillatory central schemes with nonstaggered grids for hyperbolic conservation laws. SIAM J Numer Anal, 1998, 35 (6): 2147- 2168
doi: 10.1137/S0036142997317560 |
| 15 |
Touma R . Unstaggered central schemes with constrained transport treatment for ideal and shallow water magnetohydrodynamics. Appl Numer Math, 2010, 60 (7): 752- 766
doi: 10.1016/j.apnum.2010.02.006 |
| 16 | Touma R , Khankan S . Well-balanced unstaggered central schemes for one and two-dimensional shallow water equation systems. Appl Math Comput, 2012, 218 (10): 5948- 5960 |
| 17 |
Bollermann A , Chen G X , Kurganov A , et al. A well-balanced reconstruction for wet/dry fronts for the shallow water equations. J Sci Comput, 2013, 56 (2): 267- 290
doi: 10.1007/s10915-012-9677-5 |
| [1] | Mangmang Jian,Supei Zheng,Jianhu Feng,Mengqing Zhai. Well-Balanced Preserving of Entropy Stable Schemes for Shallow Water Equations [J]. Acta mathematica scientia,Series A, 2023, 43(2): 491-504. |
| [2] | Yuan Huang,Yue Zhi,Tong Kang,Ran Wang,Hong Zhang. Fully Discrete Finite Element Scheme for a Nonlinear Induction Heating Problem [J]. Acta mathematica scientia,Series A, 2022, 42(4): 1238-1255. |
| [3] | Haiyun Deng,Hui Liu,Wenjing Song. Finite Difference Scheme for the Nonhomogeneous Initial Boundary Value Problem of Critical Schrödinger Map [J]. Acta mathematica scientia,Series A, 2021, 41(5): 1311-1322. |
| [4] | Supei Zheng,Xia Xu,Jianhu Feng,Dou Jia. High Order Sign Preserving Entropy Stable Schemes [J]. Acta mathematica scientia,Series A, 2021, 41(5): 1296-1310. |
| [5] | Keyan Wang,Qisheng Wang. Error Estimates for Expanded Mixed Finite Element Methods for Nonlinear Hyperbolic Equation [J]. Acta mathematica scientia,Series A, 2021, 41(2): 468-478. |
| [6] | Zhihao Ge,Ruihua Li. Numerical Methods for the Critical Temperature and Gap Solution of Bogoliubov-Tolmachev-Shirkov Model [J]. Acta mathematica scientia,Series A, 2020, 40(6): 1699-1711. |
| [7] | Xiaolong Zhao,Meilan Qiu,Xijun Yu,Fang Qing,Shijun Zou. A Second-Order RKDG Method for Lagrangian Compressible Euler Equations on Unstructured Triangular Meshes [J]. Acta mathematica scientia,Series A, 2020, 40(5): 1354-1361. |
| [8] | Qin Zhou,Yin Yang. Adaptive Mesh Method for Solving a Second-Order Hyperbolic Equation [J]. Acta mathematica scientia,Series A, 2019, 39(4): 942-950. |
| [9] | Jian Dong. Research on the Application of Central Scheme in Saint-Venant System [J]. Acta mathematica scientia,Series A, 2019, 39(2): 372-385. |
| [10] | Zhihao Ge,Yuanyuan Ge. Multigrid Uzawa-Type Mixed Finite Element Methods for Nearly Incompressible Linear Elasticity Problem [J]. Acta mathematica scientia,Series A, 2018, 38(5): 873-882. |
| [11] | Ge Zhihao, Cao Jiwei. A New Absolutely Stable hp Discontinuous Galerkin Methodfor the Reaction-Diffusion Problem [J]. Acta mathematica scientia,Series A, 2018, 38(2): 385-394. |
| [12] | Chen Hongbin, Gan Siqing, Xu Da, Peng Yulong. A Formally Second-Order BDF Compact ADI Difference Scheme for the Two-Dimensional Fractional Evolution Equation [J]. Acta mathematica scientia,Series A, 2017, 37(5): 976-992. |
| [13] | Chen Chuanjun, Zhang Xiaoyan, Zhao Xin. A Two-Grid Finite Volume Element Approximation for One-Dimensional Nonlinear Parabolic Equations [J]. Acta mathematica scientia,Series A, 2017, 37(5): 962-975. |
| [14] | Wang Heyuan. The Dynamical Behaviors and the Numerical Simulation of a Five-Mode Lorenz-Like System of the MHD Equations for a Two-Dimensional Incompressible Fluid on a Torus [J]. Acta mathematica scientia,Series A, 2017, 37(1): 199-216. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 140
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 121
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|