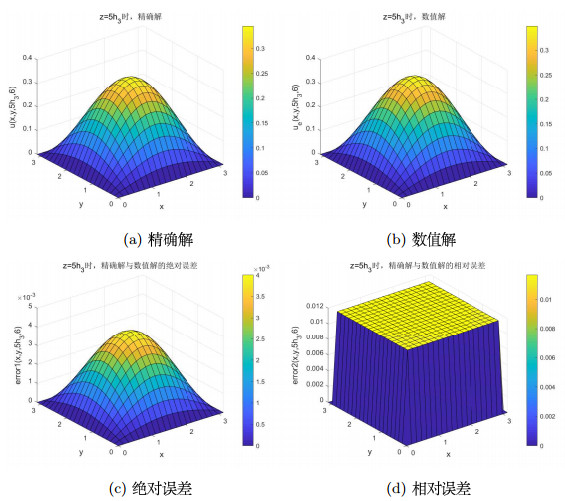
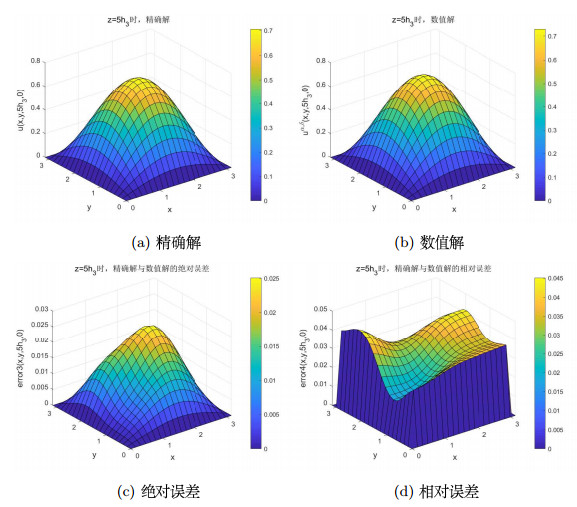
Acta mathematica scientia,Series A ›› 2022, Vol. 42 ›› Issue (1): 187-200.
Previous Articles Next Articles
Numerical Solution of the Three-Dimensional Inverse Heat Conduction Problems
Qingchun Meng1,2,Lei Zhang1,*( )
)
- 1 Department of Mathematical Sciences, Heilongjiang University, Harbin 150080
2 Institute of Applied Mathematics and Fundamental Sciences, Lviv Polytechnic National University, Lviv, Ukraine 79013
-
Received:2021-01-11Online:2022-02-26Published:2022-02-23 -
Contact:Lei Zhang E-mail:zhanglei@hlju.edu.cn -
Supported by:the NSFC(11871198);the NSFC(11801116);the Fundamental Research Funds for the Universities of Heilongjiang Province-Youth Innovation Team(RCYJTD201804);the Fundamental Research Funds for the Central Universities(3072020CFT2401)
CLC Number:
- O241.8
Cite this article
Qingchun Meng,Lei Zhang. Numerical Solution of the Three-Dimensional Inverse Heat Conduction Problems[J].Acta mathematica scientia,Series A, 2022, 42(1): 187-200.
share this article
"
| T | J=10 | J=20 | |||||
| 2 | 0.0972 | 0.0087 | 0.0111 | 0.0961 | 0.0030 | 0.0039 | |
| 4 | 0.1537 | 0.0137 | 0.0222 | 0.1514 | 0.0048 | 0.0077 | |
| 6 | 0.1824 | 0.0163 | 0.0335 | 0.1790 | 0.0057 | 0.0116 | |
| 8 | 0.1923 | 0.0172 | 0.0449 | 0.1881 | 0.0059 | 0.0155 | |
| T | J=30 | J=40 | |||||
| 2 | 0.0961 | 0.0030 | 0.0039 | 0.1435 | 0.0016 | 0.0020 | |
| 4 | 0.1514 | 0.0048 | 0.0077 | 0.2260 | 0.0025 | 0.0041 | |
| 6 | 0.1790 | 0.0057 | 0.0116 | 0.2669 | 0.0030 | 0.0061 | |
| 8 | 0.1881 | 0.0059 | 0.0155 | 0.2803 | 0.0031 | 0.0082 | |
"
| δ | α = 0.0001 | α = 0.0005 | |||||
| 0.001 | 0.3704 | 0.0119 | 0.0117 | 0.3165 | 0.0100 | 0.0100 | |
| 0.005 | 0.4378 | 0.0134 | 0.0138 | 0.3815 | 0.0126 | 0.0121 | |
| 0.01 | 0.5333 | 0.0198 | 0.0169 | 0.4628 | 0.0156 | 0.0146 | |
| 0.05 | 1.3499 | 0.0734 | 0.0427 | 1.1337 | 0.0432 | 0.0359 | |
| δ | α = 0.001 | α = 0.005 | |||||
| 0.001 | 0.2490 | 0.0080 | 0.0079 | 0.2778 | 0.0088 | 0.0088 | |
| 0.005 | 0.3122 | 0.0100 | 0.0099 | 0.2147 | 0.0068 | 0.0068 | |
| 0.01 | 0.3950 | 0.0122 | 0.0125 | 0.1381 | 0.0048 | 0.0044 | |
| 0.05 | 1.0394 | 0.0332 | 0.0329 | 0.5074 | 0.0152 | 0.0160 | |
"
| α | T=4 | T=6 | |||||
| 0.0001 | 0.2490 | 0.0080 | 0.0079 | 0.2778 | 0.0088 | 0.0088 | |
| 0.0005 | 0.3122 | 0.0100 | 0.0099 | 0.2147 | 0.0068 | 0.0068 | |
| 0.001 | 0.3950 | 0.0122 | 0.0125 | 0.1381 | 0.0048 | 0.0044 | |
| 0.005 | 1.0394 | 0.0332 | 0.0329 | 0.0377 | 0.0011 | 0.0013 | |
| α | T=8 | T=10 | |||||
| 0.0001 | 0.4306 | 0.0141 | 0.0154 | 0.5406 | 0.0201 | 0.0193 | |
| 0.0005 | 0.3987 | 0.0130 | 0.0142 | 0.4987 | 0.0159 | 0.0178 | |
| 0.001 | 0.3616 | 0.0117 | 0.0129 | 0.4513 | 0.0142 | 0.0161 | |
| 0.005 | 0.0686 | 0.0023 | 0.0024 | 0.0789 | 0.0024 | 0.0028 | |
| α | T=20 | T=30 | |||||
| 0.0001 | 1.0701 | 0.0332 | 0.0382 | 1.5610 | 0.0492 | 0.0557 | |
| 0.0005 | 0.9424 | 0.0297 | 0.0336 | 1.1354 | 0.0359 | 0.0405 | |
| 0.001 | 0.7844 | 0.0247 | 0.0280 | 0.6202 | 0.0196 | 0.0221 | |
| 0.005 | 0.4205 | 0.0133 | 0.0150 | 2.9273 | 0.0926 | 0.1044 | |
| α | T=40 | T=50 | |||||
| 0.0001 | 1.8839 | 0.0595 | 0.0672 | 1.6395 | 0.0519 | 0.0585 | |
| 0.0005 | 0.5125 | 0.0162 | 0.0183 | 2.3469 | 0.0742 | 0.0837 | |
| 0.001 | 1.0346 | 0.0327 | 0.0369 | 6.0408 | 0.1910 | 0.2154 | |
| 0.005 | 9.2018 | 0.2910 | 0.3281 | 17.8107 | 0.5632 | 0.6350 | |
"
| α | T=4 | T=6 | |||||
| 0.0001 | 0.6014 | 0.0454 | 0.0214 | 0.9492 | 0.0748 | 0.0338 | |
| 0.0005 | 0.2497 | 0.0135 | 0.0089 | 0.3730 | 0.0185 | 0.0133 | |
| 0.001 | 0.2042 | 0.0101 | 0.0073 | 0.3015 | 0.0144 | 0.0108 | |
| 0.005 | 0.0210 | 0.0015 | 0.0007 | 0.0585 | 0.0031 | 0.0021 | |
| α | T=8 | T=10 | |||||
| 0.0001 | 1.0726 | 0.0650 | 0.0382 | 1.1132 | 0.0726 | 0.0397 | |
| 0.0005 | 0.4502 | 0.0205 | 0.0161 | 0.5333 | 0.0230 | 0.0190 | |
| 0.001 | 0.3932 | 0.0199 | 0.0140 | 0.4762 | 0.0163 | 0.0170 | |
| 0.005 | 0.0865 | 0.0044 | 0.0031 | 0.0953 | 0.0037 | 0.0034 | |
| α | T=20 | T=30 | |||||
| 0.0001 | 1.1529 | 0.0486 | 0.0411 | 1.5826 | 0.0521 | 0.0564 | |
| 0.0005 | 0.9618 | 0.0333 | 0.0343 | 1.1500 | 0.0356 | 0.0401 | |
| 0.001 | 0.7997 | 0.0240 | 0.0285 | 0.6346 | 0.0203 | 0.0226 | |
| 0.005 | 0.4066 | 0.0131 | 0.0145 | 2.9149 | 0.0922 | 0.1039 | |
| α | T=40 | T=50 | |||||
| 0.0001 | 1.8994 | 0.0617 | 0.0677 | 1.6546 | 0.0525 | 0.0590 | |
| 0.0005 | 0.5265 | 0.0166 | 0.0188 | 2.3341 | 0.0738 | 0.0832 | |
| 0.001 | 1.0211 | 0.0325 | 0.0364 | 6.0298 | 0.1907 | 0.2150 | |
| 0.005 | 9.1925 | 0.2907 | 0.3278 | 17.8057 | 0.5631 | 0.6349 | |
| 1 |
Hazanee A , Ismailov M I , Lesnic D . An inverse time-dependent source problem for the heat equation. Appl Numer Math, 2013, 69: 13- 33
doi: 10.1016/j.apnum.2013.02.004 |
| 2 | Zhang J L , Sheng T T . Dynamic system method for solving inverse problems in heat conduction equations. J Comput Sci Eng, 2014, 11: 413- 417 |
| 3 |
Min T , Zang S Q , Chen S N . Source strength identification problem for the three-dimensional inverse heat conduction equations. Inverse Probl Sci Eng, 2020, 28 (6): 827- 838
doi: 10.1080/17415977.2019.1665663 |
| 4 |
Wagner B M , Fernando M R . A comparison of some inverse methods for estimating the initial condition of the heat equation. J Comput Appl Math, 1999, 103 (1): 145- 163
doi: 10.1016/S0377-0427(98)00249-0 |
| 5 |
Khalid M , Salim M , Zaman F D . Initial inverse problem in heat equation with Bessel operator. Int J Heat Mass Tran, 2002, 45 (14): 2959- 2965
doi: 10.1016/S0017-9310(02)00019-4 |
| 6 |
Yang T , Zhen W W , Xie J X . Reversing inverse problem of source term of heat conduction equation. Adv Appl Math, 2019, 8 (1): 105- 110
doi: 10.12677/AAM.2019.81012 |
| 7 |
Damirchi J , Yazdanian A R , Shamami T R . Numerical investigation of an inverse problem based on regularization method. Math Sci, 2019, 13 (3): 193- 199
doi: 10.1007/s40096-019-0288-2 |
| 8 |
Tuan N H , Binh T T , Minh N D . An improved regularization method for initial inverse problem in 2-D heat equation. Appl Math Model, 2015, 39 (2): 425- 437
doi: 10.1016/j.apm.2014.05.014 |
| 9 |
Chen H , Frankel J I , Keyhani M . Nonlinear inverse heat conduction problem of surface temperature estimation by calibration integral equation method. Numer Heat Tr B-Fund, 2018, 73 (5): 263- 291
doi: 10.1080/10407790.2018.1464316 |
| 10 |
Joachimiak M , Ciaikowski M . Nonlinear unsteady inverse boundary problem for heat conduction equation. Archives of Thermodynamics, 2017, 38 (2): 81- 100
doi: 10.1515/aoter-2017-0011 |
| 11 | Chapko R , Mindrinos L . On the non-linear integral equation approach for an inverse boundary value problem for the heat equation. J Eng Math, 2019, 119 (2): 255- 268 |
| 12 | Ma K Y , Prakash P , Deiveegan A . Generalized Tikhonov methods for an inverse source problem of the time-fractional diffusion equation. J Eng Math, 2018, 108: 39- 48 |
| 13 |
Liu C J , Wei T . Moving boundary identification for a two-dimensional inverse heat conduction problem. Inverse Probl Sci En, 2011, 19 (8): 1139- 1154
doi: 10.1080/17415977.2011.603084 |
| 14 | Lewis R W , Nithiarasu P , Seetharamu K N . Fundamentals of the Finite Element Method for Heat and Fluid Flow. New York: John Wiley & Sons, 2004: 152- 155 |
| 15 | 孔祥谦. 有限单元法在传热学中的应用. 北京: 科学出版社, 1998: 29- 31 |
| Kong X Q . The Application of the Finite Element Method in Heat Transfer. Beijing: Science Press, 1998: 29- 31 | |
| 16 |
Bourgeois H M , Kirsch A , Rundell W . Inverse problems for partial differential equations. Oberwolfach Reports, 2012, 9 (1): 611- 659
doi: 10.4171/OWR/2012/11 |
| 17 | 刘继军. 不适定问题的正则化方法及应用. 北京: 科学出版社, 2005: 132- 156 |
| Liu J J . The Regularization Methods and Applications of Ill-posed Problems. Beijing: Science Press, 2005: 132- 156 | |
| 18 |
Liu J J . Numerical solution of forward and backward problem for 2-D heat conduction equation. J Comput Appl Math, 2002, 145 (2): 459- 482
doi: 10.1016/S0377-0427(01)00595-7 |
| 19 | Tikhonov A N , Goncharsky A V , Stepanov V V , Yagola A G . Solutions of Ill-posed Problems. Washington, DC: Winston, 1977: 188- 196 |
| [1] | Zhang Hongwu. One Regularization Method for a Cauchy Problem of Semilinear Elliptic Equation [J]. Acta mathematica scientia,Series A, 2022, 42(1): 45-57. |
| [2] | Xiaoxia Yang,Houchao Zhang. Discontinuous Galerkin Finite Element Analysis of for the Extended Fisher-Kolmogorov Equation [J]. Acta mathematica scientia,Series A, 2021, 41(6): 1880-1896. |
| [3] | Ting Li,Pengzhan Huang. A Modular grad-div Stabilized Finite Element Method for Nematic Liquid Crystal Flow [J]. Acta mathematica scientia,Series A, 2021, 41(2): 451-467. |
| [4] | Fan Yang,Qianchao Wang,Xiaoxiao Li. Fractional Landweber Iterative Regularization Method to Identify Source Term for the Rayleigh-Stokes Equation [J]. Acta mathematica scientia,Series A, 2021, 41(2): 427-450. |
| [5] | Keyan Wang,Qisheng Wang. Error Estimates for Expanded Mixed Finite Element Methods for Nonlinear Hyperbolic Equation [J]. Acta mathematica scientia,Series A, 2021, 41(2): 468-478. |
| [6] | Wei Li,Pengzhan Huang. A Viscosity-Splitting Finite Element Method for the Fluid-Fluid Interaction Problem [J]. Acta mathematica scientia,Series A, 2020, 40(5): 1362-1380. |
| [7] | Zhongjian Zhao,Shaochun Chen. A Triangular Prism Finite Element for the Second-Order Elliptic Mixed Problem [J]. Acta mathematica scientia,Series A, 2020, 40(3): 684-693. |
| [8] | Junjun Wang,Xiaoxia Yang. Superconvergence Analysis of an H1-Galerkin Mixed Finite Element Method for Nonlinear Parabolic Equation [J]. Acta mathematica scientia,Series A, 2019, 39(4): 894-908. |
| [9] | Zhihao Ge,Yuanyuan Ge. Multigrid Uzawa-Type Mixed Finite Element Methods for Nearly Incompressible Linear Elasticity Problem [J]. Acta mathematica scientia,Series A, 2018, 38(5): 873-882. |
| [10] | Zhang Houchao, Wang Junjun, Shi Dongyang. A Type of New Lower Order Nonconforming Mixed Finite Elements Methods for the Extended Fisher-Kolmogorov Equation [J]. Acta mathematica scientia,Series A, 2018, 38(3): 571-587. |
| [11] | Zhang Houchao, Shi Dongyang. Superconvergence Analysis of a Lower Order Mixed Finite Element Method for Nonlinear Fourth-Order Hyperbolic Equation [J]. Acta mathematica scientia,Series A, 2016, 36(4): 656-671. |
| [12] | Wen Juan, He Yinnian, Huang Pengzhan, Li Min. Numerical Investigations on Several Stabilized Finite Element Methods for the Navier-Stokes Problem [J]. Acta mathematica scientia,Series A, 2016, 36(3): 543-557. |
| [13] | CHEN Chuan-Jun, ZHAO Xin. Analysis of a Two-grid Method for Characteristic Finite Element Solutions of Nonlinear Convection-diffusion Equations [J]. Acta mathematica scientia,Series A, 2014, 34(3): 643-654. |
| [14] | YANG Yu-Bo, ZHU Peng, YIN Yun-Hui. A Optimal Uniformly Convergent Discontinuous Galerkin Finite Element Method for Singularly Perturbed Problem [J]. Acta mathematica scientia,Series A, 2014, 34(3): 716-726. |
| [15] | WEN Juan, HE Yin-Nian, WANG Xue-Min, HUO Mi-Hui. TWO-LEVEL MULTISCALE FINITE ELEMENT METHODS FOR THE STEADY NAVIER-STOKES PROBLEM [J]. Acta mathematica scientia,Series A, 2014, 34(3): 960-972. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 182
|
|
|||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 114
|
|
|||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||
|