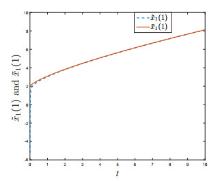
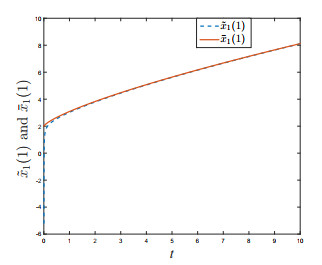
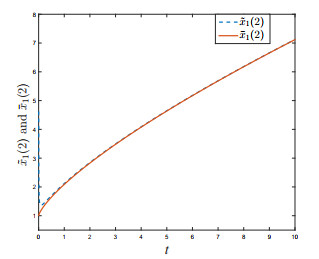
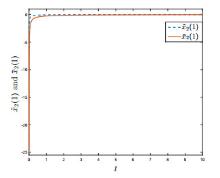
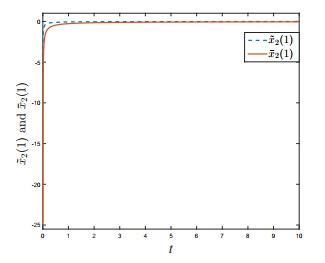
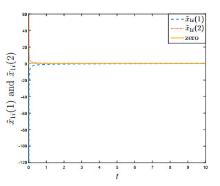
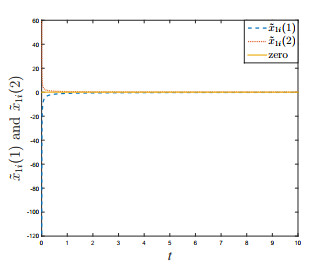
Acta mathematica scientia,Series A ›› 2021, Vol. 41 ›› Issue (5): 1529-1544.
Previous Articles Next Articles
On the Observer Design for Fractional Singular Linear Systems
Zaiyong Feng1,2( ),Ning Chen3,*(
),Ning Chen3,*( ),Yongpeng Tai4,Zhengrong Xiang2(
),Yongpeng Tai4,Zhengrong Xiang2( )
)
- 1 Department of Basic Sciences, Nanjing Institute of Railway Technology, Nanjing 210031
2 School of Automation, Nanjing University of Science and Technology, Nanjing 210094
3 College of Mechanical and Electronic Engineering, Nanjing Forestry University, Nanjing 210037
4 College of Automobile and Traffic Engineering, Nanjing Forestry University, Nanjing 210037
-
Received:2020-09-24Online:2021-10-26Published:2021-10-08 -
Contact:Ning Chen E-mail:zyfeng-97040@163.com;chenning@njfu.edu.cn -
Supported by:the NSFC(61873128);the NSF of the Jiangsu Higher Education Institution of China(17KJB120008);the Jiangsu Rail Transit Control Engineering Technology Research and Development Center(KFJ2010);the Qing Lan Project of the Jiangsu Higher Education Institutions;Qing Lan Project of Nanjing Institute of Railway Technology
CLC Number:
- TP13
Cite this article
Zaiyong Feng,Ning Chen,Yongpeng Tai,Zhengrong Xiang. On the Observer Design for Fractional Singular Linear Systems[J].Acta mathematica scientia,Series A, 2021, 41(5): 1529-1544.
share this article
| 1 | Podlubny I . Fractional Differential Equations. New York: Academic Press, 1999 |
| 2 |
Diethelm K , Ford N J . Analysis of fractional differential equations. Journal of Mathematical Analysis and Applications, 2002, 265 (2): 229- 248
doi: 10.1006/jmaa.2000.7194 |
| 3 |
Daftardar-Gejji V , Babakhani A . Analysis of a system of fractional differential equations. Journal of Mathematical Analysis and Applications, 2004, 293 (2): 511- 522
doi: 10.1016/j.jmaa.2004.01.013 |
| 4 |
Lakshmikantham V . Theory of fractional functional differential equations. Nonlinear Analysis Theory Methods and Applications, 2008, 69 (10): 3337- 3343
doi: 10.1016/j.na.2007.09.025 |
| 5 | Kilbas A A, Srivastava H M, Trujillo J J. Theory and Applications of Fractional Differential Equations. New York: Elsevier, 2006 |
| 6 | Du M, Wang Z, Hu H. Measuring memory with the order of fractional derivative. Scientific Reports, 2013. DOI: 10.1038/srep03431 |
| 7 | Wang Z H . Fractiona calculus: A mathematical method of describing the memory characteristic and intermediate process. Scientific Chinese, 2011, 3, 76- 78 |
| 8 |
Koeller R . Applications of fractional calculus to the theory of viscoelasticity. Transactions of the Asme Journal of Applied Mechanics, 1984, 51 (2): 299- 307
doi: 10.1115/1.3167616 |
| 9 | Nadzharyan T A , Kostrov S A , Stepanov G V . Fractional rheological models of dynamic mechanical behavior of magnetoactive elastomers in magnetic fields. Polymer: The International Journal for the Science and Technology of Polymers, 2018, 142, 316- 329 |
| 10 | Wang Z H , Hu H Y . Stability of a linear oscillator with damping force of fractional-order derivative. Sci China Ser G, 2009, 39 (10): 1495- 1502 |
| 11 | 蒲亦非. 分数阶微积分在现代信号分析与处理中应用的研究[D]. 成都: 四川大学, 2006 |
| PU Y F. Research on Application of Fractional Calculus to Latest Signal Analysis and Processing[D]. Chengdu: Sichuan University, 2006 | |
| 12 | N'Doye I, Zasadzinski M, Darouach M. Stabilization of Singular Fractional-order Systems: an LMI Approach[C]//Raymond G, et al. 18th Mediterranean Conference on Control and Automation, MED'10. Marrakech, Morocco: IEEE, 2010: 209-213 |
| 13 |
N'Doye I , Darouach M , Zasadzinski M . Robust stabilization of uncertain descriptor fractional-order systems. Automatica, 2013, 49 (6): 1907- 1913
doi: 10.1016/j.automatica.2013.02.066 |
| 14 | Marir S , Chadli M , Bouagada D . New admissibility conditions for singular linear continuous-time fractional-order systems. Journal of the Franklin Institute, 2016, 354 (2): 752- 766 |
| 15 |
Yao Y U , Zhuang J , Chang Y S . Sufficient and necessary condition of admissibility for fractional-order singular system. Acta Automatica Sinica, 2013, 39 (12): 2160- 2164
doi: 10.1016/S1874-1029(14)60003-3 |
| 16 |
Lin C , Chen B , Shi P . Necessary and sufficient conditions of observer-based stabilization for a class of fractional-order descriptor systems. Systems and Control Letters, 2018, 112, 31- 35
doi: 10.1016/j.sysconle.2017.12.004 |
| 17 |
Kaczorek T . Descriptor fractional linear systems with regular pencils. Asian Journal of Control, 2013, 15 (4): 1051- 1064
doi: 10.1002/asjc.579 |
| 18 | Feng Z Y , Chen N . On the existence and uniqueness of the solution of linear fractional differential-algebraic system. Mathematical Problems in Engineering, 2016, 1, 1- 9 |
| 19 | Sajewski L. Descriptor Fractional Continuous-Time Linear System and Its Solution Comparison of Three Different Methods//Szewczyk R, Zieliński C, Kaliczyńska M, et al. International Conference Automation. Switzerland: Springer, 2017: 45-57 |
| 20 |
Kaczorek T . Singular fractional linear systems and electrical circuits. International Journal of Applied Mathematics and Computer Science, 2011, 21 (2): 379- 384
doi: 10.2478/v10006-011-0028-8 |
| 21 | N'Doye I, Darouach M, Zasadzinski M. Observers Design for Singular Fractional-order Systems//2011 50th IEEE Conference on Decision and Control and European Control Conference. Orlando, FL: IEEE, 2011: 4017-4022 |
| 22 |
Zhan T , Ma S . Reduced-order observer design with unknown input for fractional order descriptor nonlinear systems. Transactions of the Institute of Measurement and Control, 2019, 41 (13): 3705- 3713
doi: 10.1177/0142331219834990 |
| 23 | Campbell S L . Singular Systems of Differential Equations. London: Pitman Publishing, 1980 |
| 24 | Debnath L , Bhatta D . Integral Transforms and Their Applications. New York: CRC Press, 2007 |
| 25 | Dai L. Singular Control Systems. Berlin: Springer, 1989 |
| 26 | Duan G R. Analysis and Design of Descriptor Linear Systems. New York: Springer, 2010 |
| [1] | Menglan Liao,Bin Guo. Asymptotic Stability of Weak Solutions to Wave Equation with Variable Exponents and Strong Damping Term [J]. Acta mathematica scientia,Series A, 2020, 40(1): 146-155. |
| [2] | Gengen Zhang,Wansheng Wang,Aiguo Xiao. Asymptotic Estimation of the Trapezoidal Method for a Class of Neutral Differential Equation with Variable Delay [J]. Acta mathematica scientia,Series A, 2019, 39(3): 560-569. |
| [3] | Jing Cui,Qiuju Liang,Nana Bi. Asymptotic Stability of Impulsive Neutral Stochastic Functional Differential Equation Driven by Fractional Brownian Motion [J]. Acta mathematica scientia,Series A, 2019, 39(3): 570-581. |
| [4] | Zhong Yue, Yuan Qian. The Asymptotic Stability of the Zero Solution for A Nonlinear Volterra Equation [J]. Acta mathematica scientia,Series A, 2015, 35(5): 878-883. |
| [5] | Luo Ricai, Xu Honglei, Wang Wusheng. Globally Asymptotic Stability of A New Class of Neutral Neural Networks with Time-Varying Delays [J]. Acta mathematica scientia,Series A, 2015, 35(3): 634-640. |
| [6] | LIU Dan-Hong, XU Gen-Qi. Study on the Diabetic Population Development of Early Warning Modeling [J]. Acta mathematica scientia,Series A, 2014, 34(3): 495-511. |
| [7] | WU Shi-Liang, LIU San-Yang. Global Asymptotic Stability of Bistable Traveling Wave Front in Reaction-diffusion Systems [J]. Acta mathematica scientia,Series A, 2010, 30(2): 440-448. |
| [8] | ZHAO Li-Qin. Necessary and Sufficient Conditions for the Global Weak Attractivity and Global Attractivity of a Class of Nonlinear Differential Equations [J]. Acta mathematica scientia,Series A, 2009, 29(3): 529-537. |
| [9] |
Xu Weijian;.
Stability and Hopf Bifurcation of an SIS Model with Species Logistic Growth and Saturating Infect Rate [J]. Acta mathematica scientia,Series A, 2008, 28(3): 578-584. |
| [10] |
Gao Shujing ;Chen Lansun.
ermanence and Global Stability for Single-species Model with Three Life Stages and Time Delay |
| [11] | CHEN Die, ZHANG Yu-Min, LIAO Xiao-Cuan-. Stability for Neutral Stochastic Functional Differential Equatio ns [J]. Acta mathematica scientia,Series A, 2005, 25(3): 323-330. |
| [12] | Shen Gengcheng Gao Guozhu Li Lifeng. Asymptotic Stability Perturbing Scalar Functional Differential Equations with Unbounded Delay [J]. Acta mathematica scientia,Series A, 1999, 19(5): 493-500. |
| [13] | Song Xinyu. ON THE NONAUTONOMOUS PREDATOR-PREY LOTKA-VOLTERRA DIFFUSION SYSTEMS [J]. Acta mathematica scientia,Series A, 1997, 17(S1): 200-205. |
| [14] | Luo Yuehu, Feng Dexing. On Asymptotic Stability for C0 Semigroups [J]. Acta mathematica scientia,Series A, 1997, 17(4): 396-402. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 136
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 166
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|