一种非结构网格上求解拉格朗日形式可压缩欧拉方程的二阶RKDG方法

Acta mathematica scientia,Series A ›› 2020, Vol. 40 ›› Issue (5): 1354-1361.

Previous Articles Next Articles

A Second-Order RKDG Method for Lagrangian Compressible Euler Equations on Unstructured Triangular Meshes

Xiaolong Zhao¹,Meilan Qiu²,Xijun Yu^3,*(),Fang Qing³,Shijun Zou³

¹ Beijing Computational Science Research Center, Graduate School of China Academy of Engineering Physics, Beijing 100193
² School of Mathematics and Statistics, Huizhou University, Guangdong Huizhou 516007
³ Laboratory of Computational Physics, Institute of Applied Physics and Computational Mathematics, Beijing 100088

Received:2018-12-12 Online:2020-10-26 Published:2020-11-04
Contact: Xijun Yu E-mail:yuxj@iapcm.ac.cn
Supported by:
the NSFC(11571002);the NSFC(11772067);the NSFC(11702028);the NSFC(U1930402);the NSF of Guangdong Province(2018A030310038);the CAEP Foundation of China(CX2019032)

Abstract

Abstract:

This paper takes advantages of the Discontinuous Galerkin (DG) method and Lagrangian scheme to present a second-order Runge-Kutta(RK) DG method for solving Lagrangian compressible Euler equations on unstructured triangular meshes. The method is more succinct than other fully Lagrangian schemes with the Jacobian matrix associated with the map between Lagrangian and Eulerian spaces, the solver of vertex velocity in the method has good adaptability for many problems. Numerical examples are presented to illustrate the robustness and second-order accuracy of the scheme.

Key words: Lagrange scheme, Unstructured triangular meshes, RKDG method

CLC Number:

O241.82

Xiaolong Zhao,Meilan Qiu,Xijun Yu,Fang Qing,Shijun Zou. A Second-Order RKDG Method for Lagrangian Compressible Euler Equations on Unstructured Triangular Meshes[J].Acta mathematica scientia,Series A, 2020, 40(5): 1354-1361.

Trendmd

Figures/Tables 4

References 8

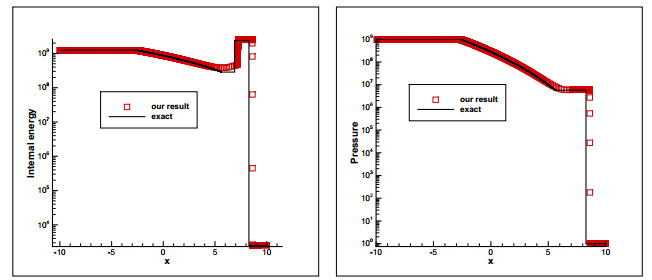
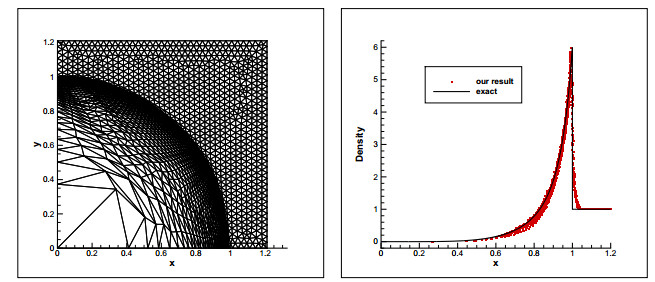
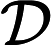1	Cockburn B , Shu C W . The Runge-Kutta discontinuous Galerkin method for conservation laws Ⅱ:general framework. Math Comp, 1989, 52, 411- 435
2	Luo H , Baum J D , Löhner R . On the computation of multi-material flows using ALE formulation. J Comput Phys, 2004, 194, 304- 328 doi: 10.1016/j.jcp.2003.09.026
3	Luo H , Baum D , Löhner R . A hermite WENO-based limiter for discontinuous Galerkin method on unstructured grids. J Comput Phys, 2007, 225 (1): 686- 713
4	Cheng J , Shu C W . A high order ENO conservative Lagrangian type scheme for the compressible Euler equations. J Comput Phys, 2007, 227, 1567- 1596 doi: 10.1016/j.jcp.2007.09.017
5	Cheng J , Shu C W . Positivity-preserving Lagrangian scheme for multi-material compressible flow. J Comput Phys, 2014, 257, 143- 168 doi: 10.1016/j.jcp.2013.09.047
6	Maire P H , Abgrall R , Breil J , et al. A Cell-Centered Lagrangian scheme for two-dimensional compressible flow problems. SIAM J Sci Comput, 2007, 29, 1781- 1824 doi: 10.1137/050633019
7	Boscheri W , Dumbser M , Balsara D S . High order Lagrangian ADER-WENO schemes on unstructured meshes-application of several node solvers to hydrodynamics and magnetohydrodynamics. Int J Numer Meth Fluids, 2014, 76, 737- 778 doi: 10.1002/fld.3947
8	Zhao X , Yu X , Duan M , et al. An arbitrary Lagrangian-Eulerian RKDG method for compressible Euler equations on unstructured meshes:Single-material flow. J Comput Phys, 2019, 396, 451- 469 doi: 10.1016/j.jcp.2019.07.015

$h({\cal D}(t))$	$L^{1}$ error	order	$L^{2}$ error	order	$L^{\infty}$ error	order
7.07E-002	2.1029E-002		2.4996E-002		6.8346E-002
3.51E-002	5.5261E-003	1.9280	6.7907E-003	1.8800	1.7204E-002	1.9900
1.76E-002	1.4303E-003	1.9498	1.8440E-003	1.8806	4.6818E-003	1.8776
8.31E-003	3.6687E-004	1.9630	5.0124E-004	1.8793	1.4688E-003	1.8724

[1]	Qin Zhou,Yin Yang. Adaptive Mesh Method for Solving a Second-Order Hyperbolic Equation [J]. Acta mathematica scientia,Series A, 2019, 39(4): 942-950.
[2]	Jian Dong. Research on the Application of Central Scheme in Saint-Venant System [J]. Acta mathematica scientia,Series A, 2019, 39(2): 372-385.
[3]	Zhihao Ge,Yuanyuan Ge. Multigrid Uzawa-Type Mixed Finite Element Methods for Nearly Incompressible Linear Elasticity Problem [J]. Acta mathematica scientia,Series A, 2018, 38(5): 873-882.
[4]	Ge Zhihao, Cao Jiwei. A New Absolutely Stable hp Discontinuous Galerkin Methodfor the Reaction-Diffusion Problem [J]. Acta mathematica scientia,Series A, 2018, 38(2): 385-394.
[5]	Chen Hongbin, Gan Siqing, Xu Da, Peng Yulong. A Formally Second-Order BDF Compact ADI Difference Scheme for the Two-Dimensional Fractional Evolution Equation [J]. Acta mathematica scientia,Series A, 2017, 37(5): 976-992.
[6]	Chen Chuanjun, Zhang Xiaoyan, Zhao Xin. A Two-Grid Finite Volume Element Approximation for One-Dimensional Nonlinear Parabolic Equations [J]. Acta mathematica scientia,Series A, 2017, 37(5): 962-975.
[7]	Wang Heyuan. The Dynamical Behaviors and the Numerical Simulation of a Five-Mode Lorenz-Like System of the MHD Equations for a Two-Dimensional Incompressible Fluid on a Torus [J]. Acta mathematica scientia,Series A, 2017, 37(1): 199-216.

A Second-Order RKDG Method for Lagrangian Compressible Euler Equations on Unstructured Triangular Meshes

RichHTML

PDF (PC)

Abstract

Cite this article

share this article

Figures/Tables 4

References 8

Related Articles 7

Metrics

Comments

Recommended 10