广义的(3+1)维Kadomtsev-Petviashvili方程的动力分析及其行波解

Abstract

Abstract:

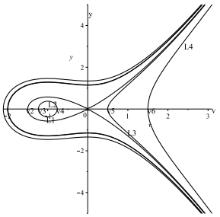
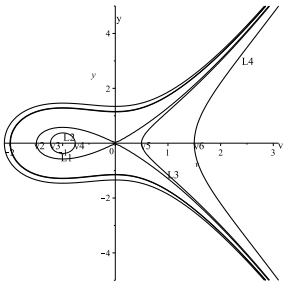
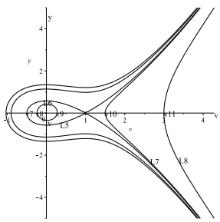
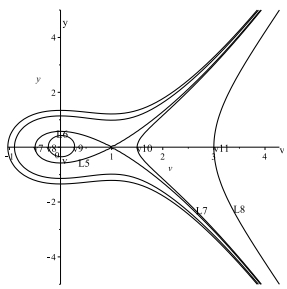
Dynamical analysis and explicit solutions for generalized (3+1)-dimension Kadomtsev-Petviashvili equation have been carried out. The singular solution is obtained by the ansatz method, the bifurcation phase portraits and corresponding explicit solution are also constructed by the approach of dynamical analysis.

Key words: Generalized (3+1)-dimension Kadomtsev-Petviashvili equation, Ansatz method, Bifurcation analysis, Phase portraits, Traveling wave solutions

CLC Number:

O175.29

Xue Zhang,Yuhuai Sun. Dynamical Analysis and Traveling Wave Solutions for Generalized (3+1)-Dimensional Kadomtsev-Petviashvili Equation[J].Acta mathematica scientia,Series A, 2019, 39(3): 501-509.

Trendmd

Figures/Tables 4

References 26

1	Adair D , Jaeger M . Simulation of tapered rotating beams with centrifugal stiffening using the adomian decomposition method. Applied Mathematical Modelling, 2016, 40: 3230- 3241 doi: 10.1016/j.apm.2015.09.097
2	Hasseine A , Bart H J . Adomian decomposition method solution of population balance equations for aggregation, nucleation, growth and breakup processes. Applied Mathematical Modelling, 2015, 39: 1975- 1984 doi: 10.1016/j.apm.2014.09.027
3	Aski F S , Seyed J N , Mohammadian E , Asgari A . Application of adomian decomposition method for micropolor flow in a porous channel. Propulsion and Power Research, 2014, 3: 15- 21 doi: 10.1016/j.jppr.2014.01.004
4	Reza M . Numerical solution of the Laplace equation in annulus by Adomian decomposition method. Chaos Solitons and Fractals, 2008, 36: 138- 143 doi: 10.1016/j.chaos.2006.06.016
5	Ein M S . Hierarchy of kissing numbers for exceptional Lie symmetry groups in high energy physics. Chaos Solitons and Fractals, 2008, 35: 420- 422 doi: 10.1016/j.chaos.2007.06.121
6	Biswas A , Yildirim A , Hayat T , et al. Soliton perturbation theory for the generalized klein-gordon equation with full nonlinearity. Proceedings of the Romanian Academy, 2012, 13: 32- 41
7	Hutchinson A J , Mason D P . Lie symmetry methods applied to the turbulent wake of a symmetric self-propelled body. Applied Mathematical Modelling, 2016, 40: 3062- 3080 doi: 10.1016/j.apm.2015.10.007
8	Song M , Rong Z L , Essaid Z , Anjan B . Singular solitons and bifurcation analysis of quadratic nonlinear klein-gordon equation. Applied Mathematical Information Science, 2013, 4: 1333- 1340
9	Triki H , Yildirim A , Hayat T , et al. Topological and non-topological soliton solutions of the bretherton equation. Proceedings of the Romanian Academy, 2012, 13: 688- 696
10	Triki H , Crutcher S , Yildirim A , et al. Bright and dark solitons of the modified complex ginzburg-landau equation with parabolic and dualpower law nonlinearity. Romanian Reports in Physics, 2012, 64: 367- 380
11	Chang S H . Convergence of variational iteration method applied two-point diffusion problems. Applied Mathematical Modelling, 2016, 40: 6805- 6810 doi: 10.1016/j.apm.2016.02.024
12	Salkuyeh D K , Tavakoli A . Interpolated variational iteration method for initial value problems. Applied Mathematical Modelling, 2016, 40: 3979- 3990 doi: 10.1016/j.apm.2015.10.037
13	Khuri S A , Sayfy A . A novel fixed point scheme proper setting of variational iteration method for BVPs. Applied Mathematical Letter, 2015, 48: 75- 84 doi: 10.1016/j.aml.2015.03.017
14	Concli F , Gorla C . Numerical modeling of the power losses in geared transmissions: Windage, churning and cavitation simulations with a new integrated approach that drastically reduces the computational effort. Tribology International, 2016, 103: 58- 68 doi: 10.1016/j.triboint.2016.06.046
15	Zhang M X , Li Y X , Li Y , et al. Numerical simulations on the effect of sloshing on liquid flow maldistribution of randomly packed column. Applied Thermal Engineering, 2017, 112: 585- 594 doi: 10.1016/j.applthermaleng.2016.10.049
16	Cheshmehzangi A . Multi-spatial environmental performance evaluation towards integrated urban design: A procedural approach with computational simulations. Journal of Cleaner Production, 2016, 139: 1085- 1093 doi: 10.1016/j.jclepro.2016.08.151
17	Kadomtsev B B , Petviashvili V . On the stability of solitary waves in weakly dispersive media. Soviet Physics Doklady, 1970, 15: 539- 541
18	Li D , Lin J , Liu X . Soliton deflexion for (1+3)-D Kadomtsev-Petviashvili equation. Commun Nonlinear Sci Numer Simul, 2009, 14: 3548- 3553 doi: 10.1016/j.cnsns.2009.02.007
19	Dorizzi B , Grammaticos B , Ramani A , Winternitz P . Are all the equations of the Kadomtsev-Petviashvili hierarchy integrable?. Journal of Mathematical Physics, 1986, 27: 2848- 2852 doi: 10.1063/1.527260
20	Lv N , Mei J Q , Zhang H Q . New explicit solutions for(3+1)-dimensional Kadomtsev-Petviashvili (KP) equation. International Journal of Nonlinear Science, 2011, 11: 506- 512
21	Peng Y Z , Krishnan E V . Exact travelling wave solutions to the (3+1)-dimensional Kadomtsev-Petviashvili equation. Acta Physical Polonica, 2005, 108: 421- 428 doi: 10.12693/APhysPolA.108.421
22	Alam M N , Akbar M A . Application of new generalized $(\frac{{G'}}{G})$-expansion method to the (3+1)-dimensional Kadomtsev-Petviashvili equation. Italian Journal of Pure and Applied Mathematics, 2016, 36: 1- 14
23	Yang R . Bifurcation analysis of a diffusive predator-prey system with Crowley-Martin functional response and delay. Chaos Solitons and Fractals, 2017, 95: 131- 139 doi: 10.1016/j.chaos.2016.12.014
24	Tang X , Song Y . Bifurcation analysis and Turing instability in a diffusive predator-prey model with herd behavior and hyperbolic mortality. Chaos Solitons and Fractals, 2015, 81: 303- 314 doi: 10.1016/j.chaos.2015.10.001
25	Chow S N , Hale J K . Method of Bifurcation Theory. New York: Springer-Verlag, 1982
26	Guckenheimer J , Homes P . Nonlinear Oscillations, Dynamical Systems and Bifurcations of Vector Fields. New York: Springer-Verlag, 1999

[1]	Penghong Zhong,Ganshan Yang,Xuan Ma. Global Existence and Self-Similar Blowup of Landau-Lifshitz-Gilbert Equation on Hyperbolic Space [J]. Acta mathematica scientia,Series A, 2019, 39(3): 461-474.
[2]	Kai Li,Han Yang,Fan Wang. Study on Weak Solution and Strong Solution of Incompressible MHD Equations with Damping in Three-Dimensional Systems [J]. Acta mathematica scientia,Series A, 2019, 39(3): 518-528.
[3]	Xianglin Han,Weigang Wang,Jiaqi Mo. Generalized Solution to the Singular Perturbation Problem for a Class of Nonlinear Differential-Integral Time Delay Reaction Diffusion System [J]. Acta mathematica scientia,Series A, 2019, 39(2): 297-306.
[4]	Yanbing Yang,Wei Lian,Shaobin Huang,Runzhang Xu. Finite Time Blow up of Solutions for Nonlinear Wave Equation with General Nonlinearity for Arbitrarily Positive Initial Energy [J]. Acta mathematica scientia,Series A, 2018, 38(6): 1239-1244.
[5]	Lin Zheng,Shu Wang,Linrui Li. Local Classical Solutions of 3D Fluid-Particle Interaction Model: The Flowing Regime [J]. Acta mathematica scientia,Series A, 2018, 38(6): 1173-1192.
[6]	Wenbo Wang,Quanqing Li. Existence and Concentration of Nontrivial Solutions for the Fractional Schrödinger Equations with Sign-Changing Steep Well Potential [J]. Acta mathematica scientia,Series A, 2018, 38(5): 893-902.
[7]	Zhao Yuanzhang, Ma Xiangru. Blow-Up Analysis for a Nonlocal Reaction-Diffusion Equation with Variant Diffusion Coefficient [J]. Acta mathematica scientia,Series A, 2018, 38(4): 750-769.
[8]	Shao Guangming, Chai Xiaojuan. Approximation of the Incompressible Navier-Stokes-Fourier System by the Artificial Compressibility Method [J]. Acta mathematica scientia,Series A, 2017, 37(6): 1070-1084.
[9]	Li Huahui, Shao Zhiqiang. Vanishing Pressure Limit of Riemann Solutions to the Aw-Rascle Model for Generalized Chaplygin Gas [J]. Acta mathematica scientia,Series A, 2017, 37(5): 917-930.
[10]	Liu Shifang, Ma Qiaozhen. Existence of Strong Global Attractors for Damped Suspension Bridge Equations with History Memory [J]. Acta mathematica scientia,Series A, 2017, 37(4): 684-697.
[11]	Li Huahui, Shao Zhiqiang. Interactions of Delta Shock Waves for the Nonsymmetric Keyfitz-Kranzer System with Split Delta Functions [J]. Acta mathematica scientia,Series A, 2017, 37(4): 714-729.
[12]	Qiu Hua, Zhang Yingshu, Fang Shaomei. The Global Existence Result of a Leray-α-Oldroyd Model to the Incompressible Viscoelastic Flow [J]. Acta mathematica scientia,Series A, 2017, 37(1): 102-112.
[13]	Gao Xinchun, Zhou Jian, Tian Miaoqing. Global Boundedness and Asymptotic Behavior in an Attraction-Repulsion Chemotaxis System with Logistic Source [J]. Acta mathematica scientia,Series A, 2017, 37(1): 113-121.
[14]	Qin Dongdong, Li Yunyang, Tang Xianhua. Schrödinger Equation with Asymptotically Linear Nonlinearity and Spectrum Point Zero [J]. Acta mathematica scientia,Series A, 2016, 36(6): 1103-1116.
[15]	Geng Fan, Li Ruizhai, Ge Xiangyu. Long Time Behavior of Boussinesq Type Equation with Damping Term [J]. Acta mathematica scientia,Series A, 2016, 36(6): 1196-1210.

Dynamical Analysis and Traveling Wave Solutions for Generalized (3+1)-Dimensional Kadomtsev-Petviashvili Equation

RichHTML

PDF (PC)

Abstract

Cite this article

share this article

Figures/Tables 4

References 26

Related Articles 15

Metrics

Comments

Recommended 10