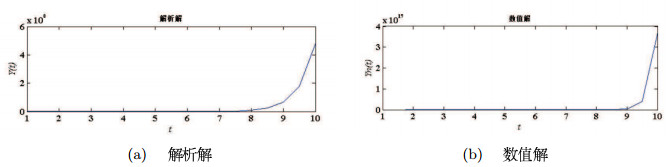
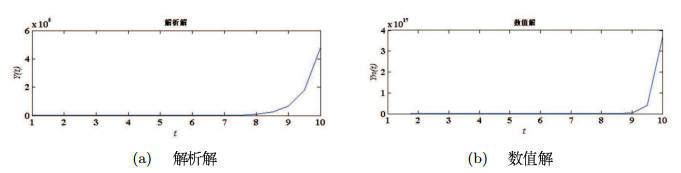
数学物理学报 ›› 2022, Vol. 42 ›› Issue (3): 826-838.
一类自变量分段连续系统的振动性分析
- 哈尔滨师范大学数学科学学院 哈尔滨 150025
-
收稿日期:2021-06-23出版日期:2022-06-26发布日期:2022-05-09 -
通讯作者:高建芳 E-mail:09151108@163.com -
基金资助:国家自然科学基金(12001143);哈尔滨师范大学学术创新项目(HSDSSCX2020-33)
Oscillation Analysis of a Kind of Systems with Piecewise Continuous Arguments
- School of Mathematical Sciences, Harbin Normal University, Harbin 150025
-
Received:2021-06-23Online:2022-06-26Published:2022-05-09 -
Contact:Jianfang Gao E-mail:09151108@163.com -
Supported by:the NSFC(12001143);the Academic Innovation Project of Harbin Normal University(HSDSSCX2020-33)
摘要:
该文主要运用
中图分类号:
- O241.8
引用本文
刘莹,高建芳. 一类自变量分段连续系统的振动性分析[J]. 数学物理学报, 2022, 42(3): 826-838.
Ying Liu,Jianfang Gao. Oscillation Analysis of a Kind of Systems with Piecewise Continuous Arguments[J]. Acta mathematica scientia,Series A, 2022, 42(3): 826-838.
使用本文
| 1 |
Cooke K L , Wiener J . Retarded differential equations with piecewise constant delays. J Math Anal Appl, 1984, 99, 265- 297
doi: 10.1016/0022-247X(84)90248-8 |
| 2 |
Song M H , Liu M Z . Numerical stability and oscillation of the Runge-Kutta methods for equation                         |
| 3 | Chen F . Oscillatory and asymptotic behaviour of odd order delay differential equations with impluses. J Math Sci, 2013, 15, 258- 273 |
| 4 |
Habibi S . Estimates on the dimension of an exponential attractor for a delay differential equation. Math Slovaca, 2014, 64, 1237- 1248
doi: 10.2478/s12175-014-0272-0 |
| 5 |
Á Garab , Pituk M , Stavroulakis I P . A sharp oscillation criterion for a linear delay differential equation. Appl Math Lett, 2019, 93, 58- 65
doi: 10.1016/j.aml.2019.01.042 |
| 6 | Durina J , Jadlovská I . A sharp oscillation result for second-order half-linear noncanonical delay differential equations. Electron J Qual Theory Differ Equa, 2020, 2020 (46): 1- 14 |
| 7 | Sethi A K , Tripathy A K . On oscillatory second order differential equations with variable delays. Palestine J Math, 2021, 3 (1): 11- 24 |
| 8 |
Pullan M C . Linear optimal control problems with piecewisw analytic solutions. J Math Anal Appl, 1996, 197, 207- 226
doi: 10.1006/jmaa.1996.0016 |
| 9 |
Shah S M , Wiener J . Advanced differential equations with piecewise constant argument deviations. Int J Math Sci, 1983, 6, 671- 703
doi: 10.1155/S0161171283000599 |
| 10 |
Song M H , Yang Z W , Liu M Z . Stability of  doi: 10.1016/j.camwa.2005.02.002 |
| 11 |
Akhmet M U . On the reduction principle for differential equations with piecewise constant argument of generalized type. J Math Anal Appl, 2007, 336, 646- 663
doi: 10.1016/j.jmaa.2007.03.010 |
| 12 |
Ozturk I , Bozkurt F . Stability analysis of a population model with piecewisw constant arguments. Nonlinear Anal Real World Appl, 2011, 12, 1532- 1545
doi: 10.1016/j.nonrwa.2010.10.011 |
| 13 | Gao J F . Numerical oscillation and non-oscillation for differential equation with piecewise continuous arguments of mixed type. Appl Math Comput, 2017, 299, 16- 27 |
| 14 |
Wiener J , Cooke K L . Oscillations in systems of differential equations with piecewise constant argument. J Math Anal Appl, 1989, 137, 221- 239
doi: 10.1016/0022-247X(89)90286-2 |
| 15 |
Luo Z G , Shen J H . New results on oscillation for delay differential equations with piecewise constant argument. Comput Math Appl, 2003, 45, 1841- 1848
doi: 10.1016/S0898-1221(03)90005-8 |
| 16 |
Wang Q , Zhu Q Y , Liu M Z . Stability and oscillations of numerical solutions for differential equations with piecewise continuous arguments of alternately advanced and retarded type. J Comput Appl Math, 2011, 235, 1542- 1552
doi: 10.1016/j.cam.2010.08.041 |
| 17 | Zhang C , Li T , Saker S H . Oscillation of fourth order delay differential equations. J Math Sci, 2014, 201, 322- 335 |
| 18 |
Džurina J , Jadlovská I . A note on oscillation of second-order delay differential equations. Appl Math Lett, 2017, 69, 126- 132
doi: 10.1016/j.aml.2017.02.003 |
| 19 |
Grace S R , Jadlovská I , Zafer A . Oscillation criteria for odd-order nonlinear delay differential equations with a middle term. Math Methods Appl Sci, 2017, 40, 5147- 5160
doi: 10.1002/mma.4377 |
| 20 | Moaaz O , Mahmoud E E , Alharbi W R . Third-order neutral delay differential equations: New iterative criteria for oscillation. J Funct Spaces, 2020, 2020 (1): 1- 8 |
| 21 |
Liu M Z , Gao J F , Yang Z W . Oscillation analysis of numerical solution in the                          |
| 22 |
Liu M Z , Gao J F , Yang Z W . Preservation of oscillation of the Runge-Kutta method for equation                         doi: 10.1016/j.camwa.2009.07.030 |
| 23 |
Gao J F , Liu S M . Oscillation analysis of numerical solutions in the  doi: 10.1002/mma.3458 |
| 24 |
Gao J F , Shi T T , Song F Y . Preservation of oscillation in the Runge-Kutta method for a type of advanced differential equation. Numer Funct Anal Optim, 2015, 36, 1420- 1430
doi: 10.1080/01630563.2015.1070863 |
| 25 | Györi I , Ladas G . Oscillation Theory of Delay Equations with Applications. Oxford: Clarendon Press, 1991: 32- 197 |
| 26 |
Song M H , Yang Z W , Liu M Z . Stability of  doi: 10.1016/j.camwa.2005.02.002 |
| [1] | 张萍,杨甲山,覃桂茳. 时间轴上二阶非线性非自治延迟动力系统的振动性[J]. 数学物理学报, 2022, 42(3): 839-850. |
| [2] | 李文娟,汤获,俞元洪. 具有次线性中立项的二阶阻尼微分方程的振动准则[J]. 数学物理学报, 2022, 42(1): 58-69. |
| [3] | 仉志余,赵成,李宇宇. 时间尺度上带超线性中立项的二阶时滞动力方程的振动性[J]. 数学物理学报, 2021, 41(6): 1838-1852. |
| [4] | 覃桂茳,杨甲山. 具拟线性中立项的二阶变时滞动力方程的振动定理[J]. 数学物理学报, 2021, 41(5): 1492-1503. |
| [5] | 闫朝琳,高建芳. 混合型脉冲微分方程的数值振动性分析[J]. 数学物理学报, 2020, 40(4): 993-1006. |
| [6] | 罗李平,罗振国,曾云辉. 一类非线性脉冲中立抛物型分布参数系统的振动条件[J]. 数学物理学报, 2020, 40(3): 784-795. |
| [7] | 李继猛,杨甲山. 具非线性中立项的二阶延迟微分方程的Philos型准则[J]. 数学物理学报, 2020, 40(1): 169-186. |
| [8] | 张晓建. 具非线性中立项的广义Emden-Fowler微分方程的振动性[J]. 数学物理学报, 2018, 38(4): 728-739. |
| [9] | 罗李平, 罗振国, 邓义华. 脉冲扰动对非线性时滞双曲型分布参数系统振动的影响[J]. 数学物理学报, 2018, 38(2): 313-321. |
| [10] | 李文娟, 汤获, 俞元洪. 中立型Emden-Fowler微分方程的振动性[J]. 数学物理学报, 2017, 37(6): 1062-1069. |
| [11] | 王云竹, 高建芳. 一类非线性延迟微分方程θ-方法的数值解振动分析[J]. 数学物理学报, 2017, 37(2): 342-351. |
| [12] | 杨甲山. 时间测度链上具正负系数的二阶阻尼动力方程的振动准则[J]. 数学物理学报, 2014, 34(2): 393-408. |
| [13] | 殷慰萍. 一类复蒙日-安培方程Dirichlet问题数值解探讨(四)[J]. 数学物理学报, 2013, 33(4): 646-654. |
| [14] | 陈大学. 具有振动系数的二阶非线性中立型时滞动力方程的有界振动性[J]. 数学物理学报, 2013, 33(1): 98-113. |
| [15] | 李同兴, 韩振来, 张承慧, 孙一冰. 时间尺度上三阶Emden-Fowler动力方程的振动准则[J]. 数学物理学报, 2012, 32(1): 222-232. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 139
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 107
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|