| [1] Hayman W K. Meromorphic Functions. Oxford: Clarendon Press, 1964
[2] Laine I. Nevanlinna Theory and Complex Differential Equations. Berlin, New York: Walter de Gruyter, 1993
[3] 仪洪勋, 杨重骏. 亚纯函数的唯一性理论. 北京: 科学出版社, 1995
[4] Heittokangas J, et al. Complex difference equations of malmquist type. Comput Methods Funct Theory, 2001, 1(1): 27--39
[5] Gundersen G, et al. Meromorphic solutions of generalized schroder equations. Aequations Math, 2002, 63: 110--135
[6] Zheng X M, Chen Z X. On properties of 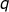 -difference equations. Acta Mathematica Scientia, 2012, 32B(2): 724--734
[7] Goldstein R. Some results on factorization of meromorphic functions. J London Math Soc, 1971, 4(2): 357--364
[8] Wang J. Growth and poles of meromorphic solutions of some complex difference equations. J Math Anal Appl, 2011, 379: 367--377
[9] Halburd R G, Korhonen R J. Finite-order meromorphic solutions and the discrete Painlev\'e equations. Proc London Math Soc, 2007, 94: 443--474
[10] Bergweiler W, Ishizaki K, Yanagihara N. Meromorphic solution of some functional equations. Methods Appl Anal, 1998, 5(3): 248--258
[11] Hayman W K. Angular value distribution of power series with gaps. Proc Lond Math Soc, 1972, 24: 590--624 |