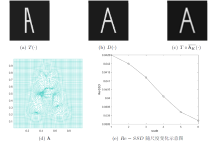
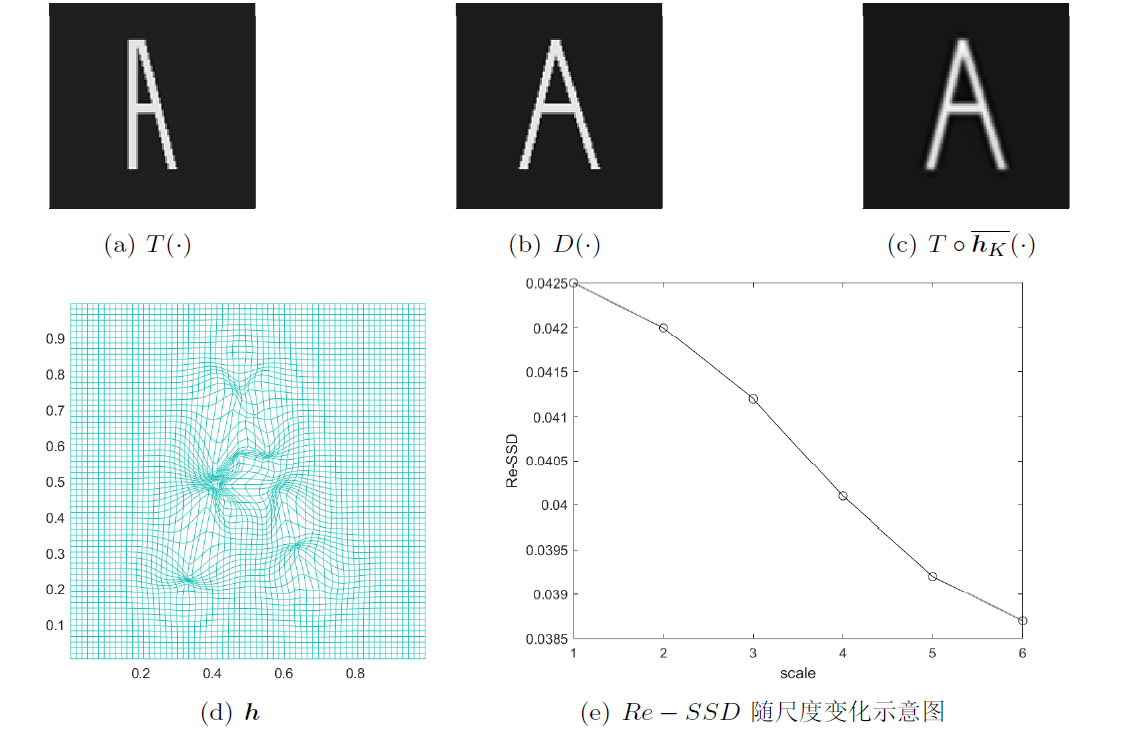
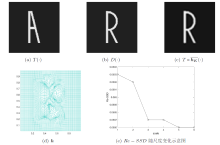
数学物理学报 ›› 2024, Vol. 44 ›› Issue (6): 1665-1688.
基于拟共形理论的分数阶多尺度微分同胚图像配准
- 武汉理工大学数学系 武汉 430070
-
收稿日期:2023-10-18修回日期:2024-04-30出版日期:2024-12-26发布日期:2024-11-22 -
通讯作者:*韩欢, Email: hanhuan11@whut.edu.cn -
作者简介:王慧楠, Email:whn@whut.edu.cn -
基金资助:国家自然科学基金(11901443);湖北省自然科学基金(2022CFB379)
Multi-Scale Approach for Diffeomorphic Image Registration with Fractional-Order Regularization Based on Quasiconformal Theory
- Department of Mathematics, Wuhan University of Technology, Wuhan 430070
-
Received:2023-10-18Revised:2024-04-30Online:2024-12-26Published:2024-11-22 -
Supported by:National Natural Science Foundation of China(11901443);Natural Science Foundation of Hubei Province of China(2022CFB379)
摘要:
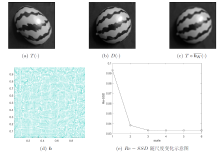
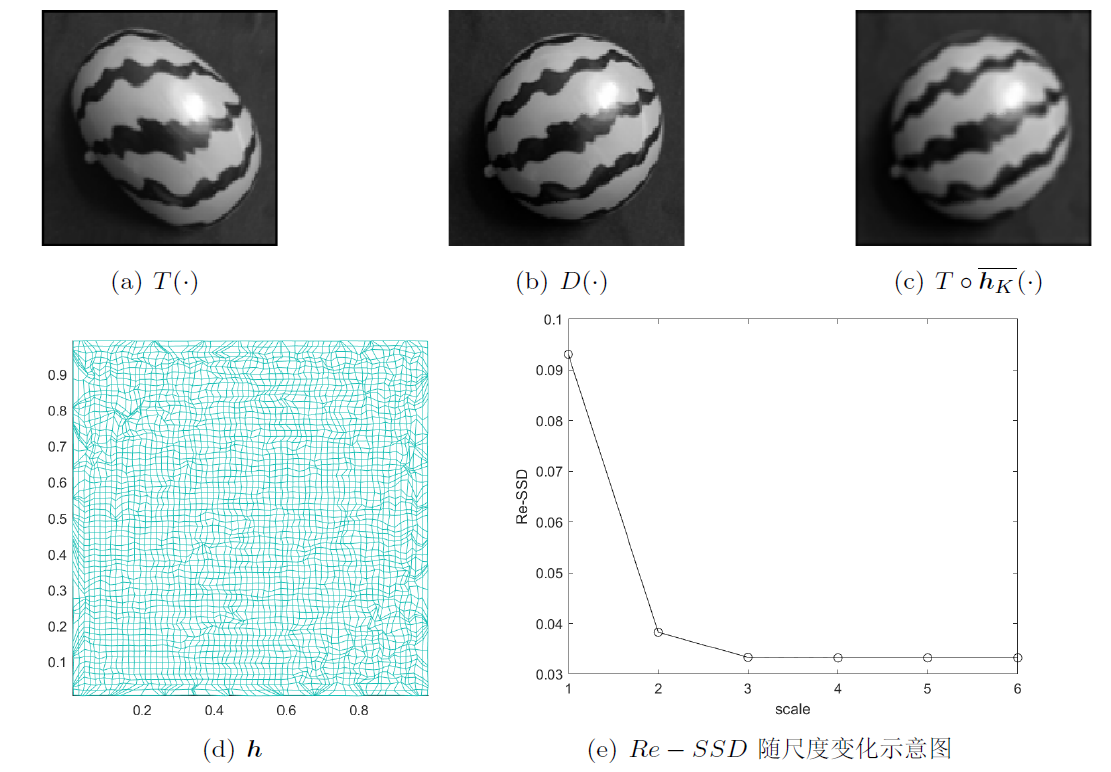
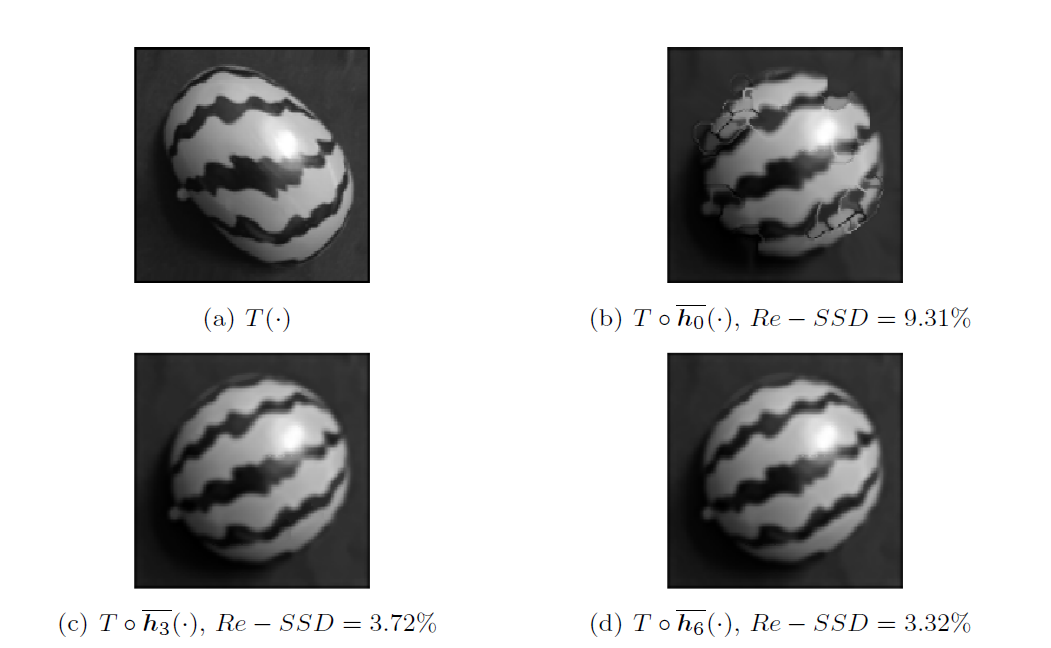
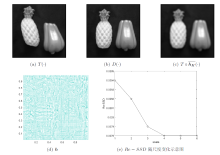
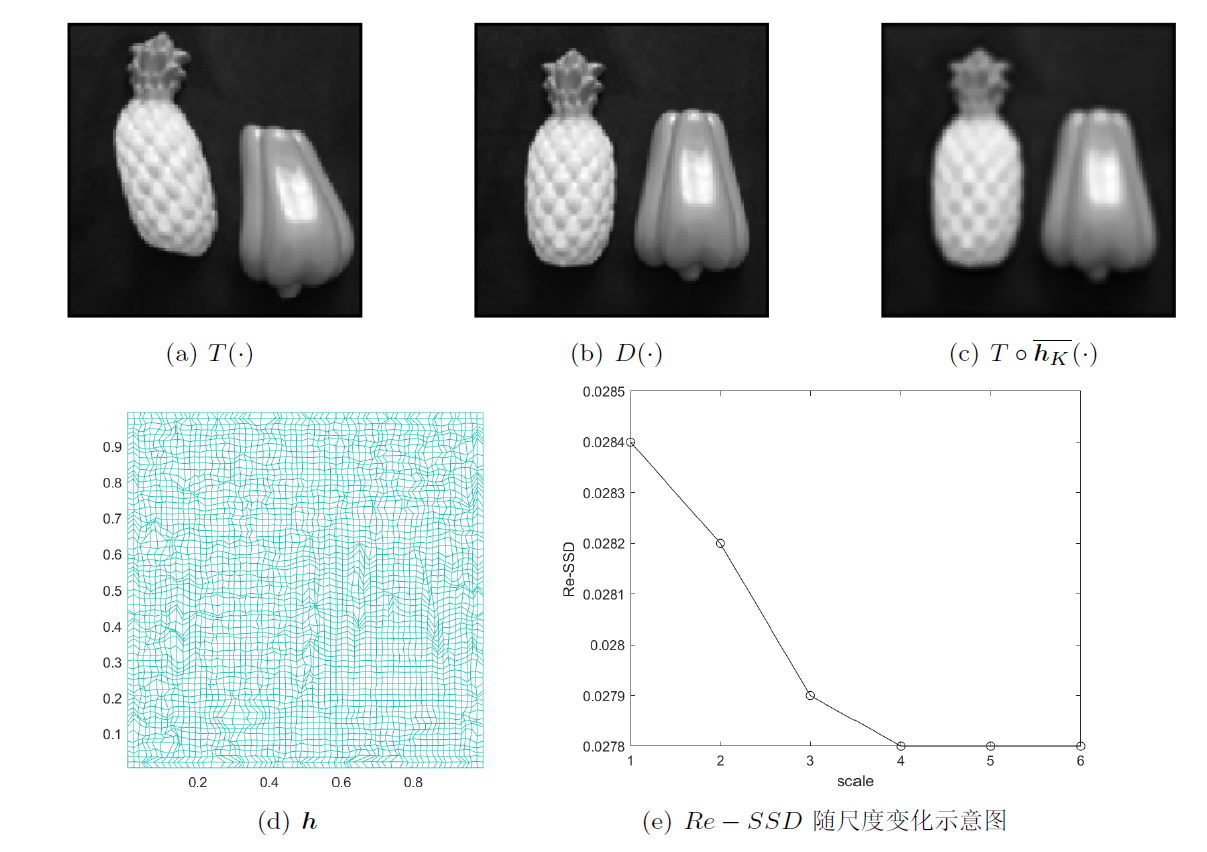
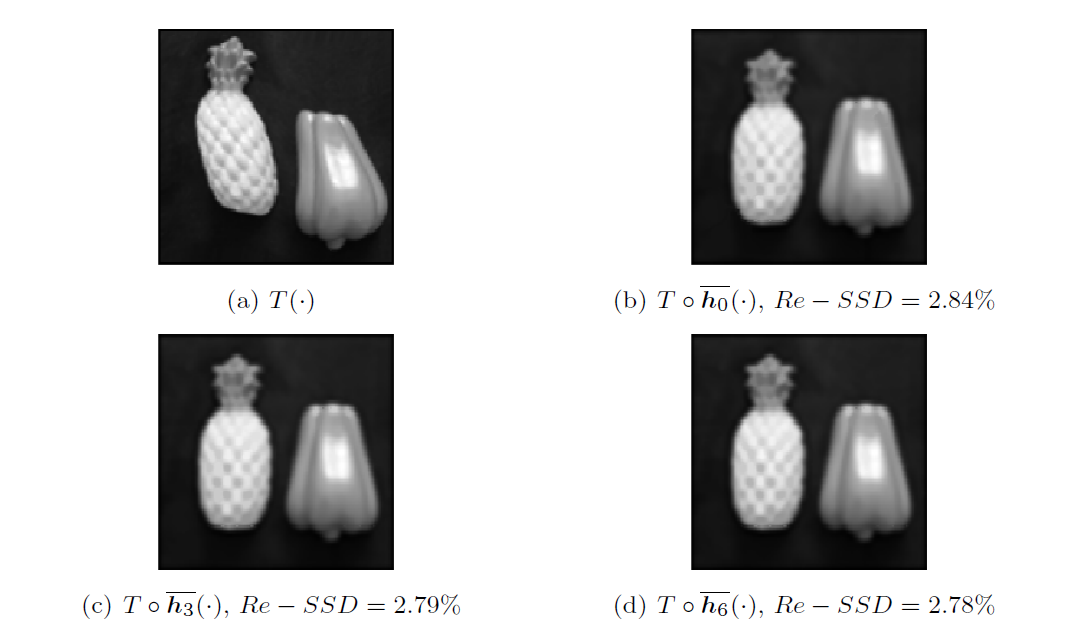
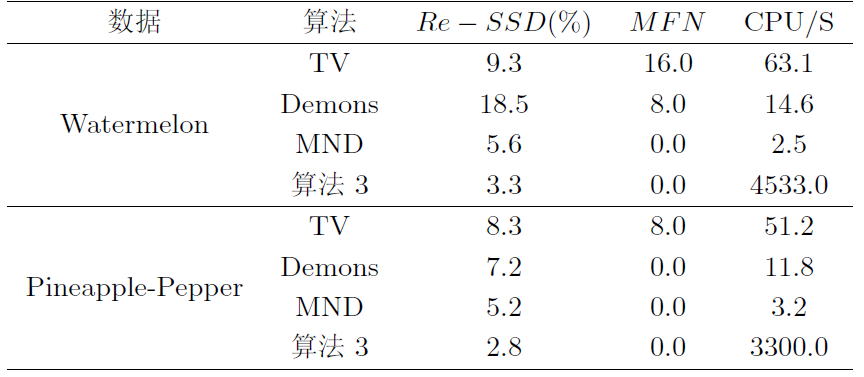
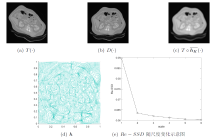
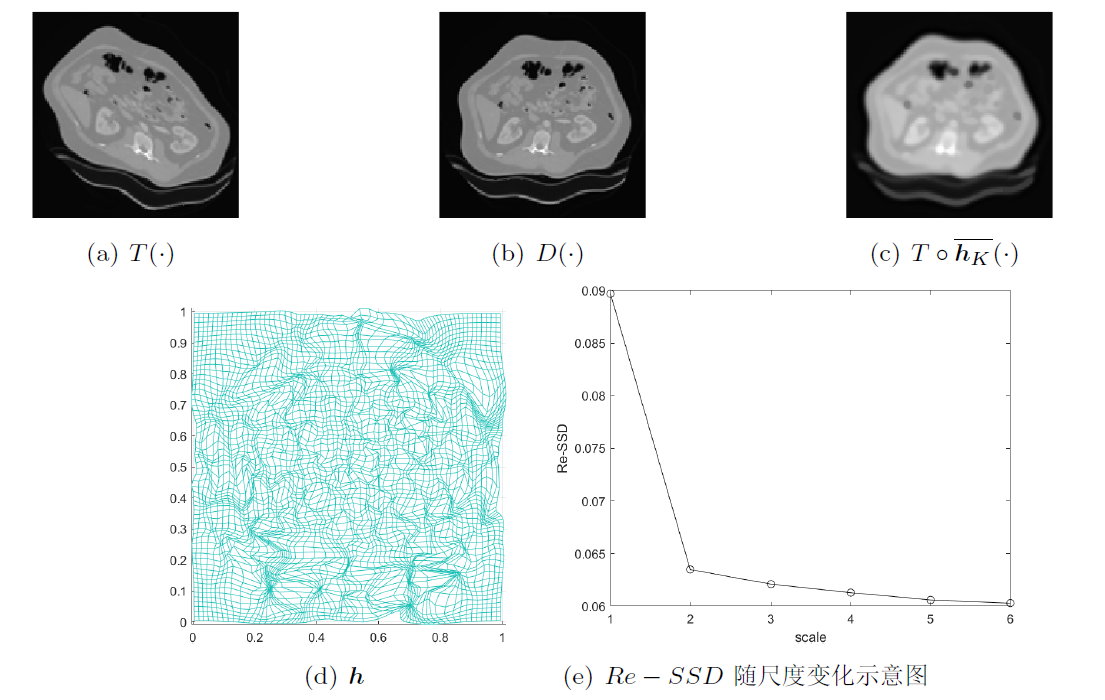
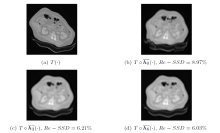
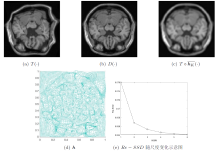
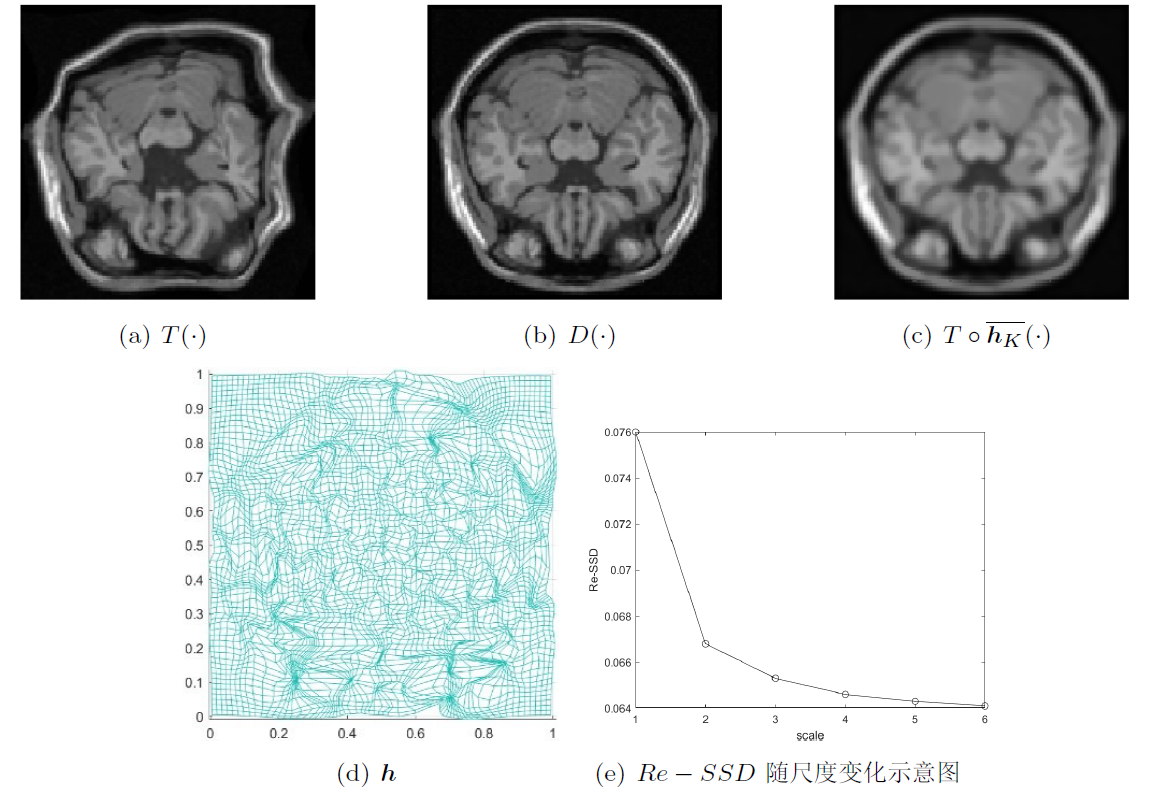
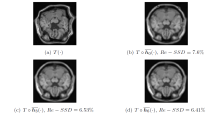
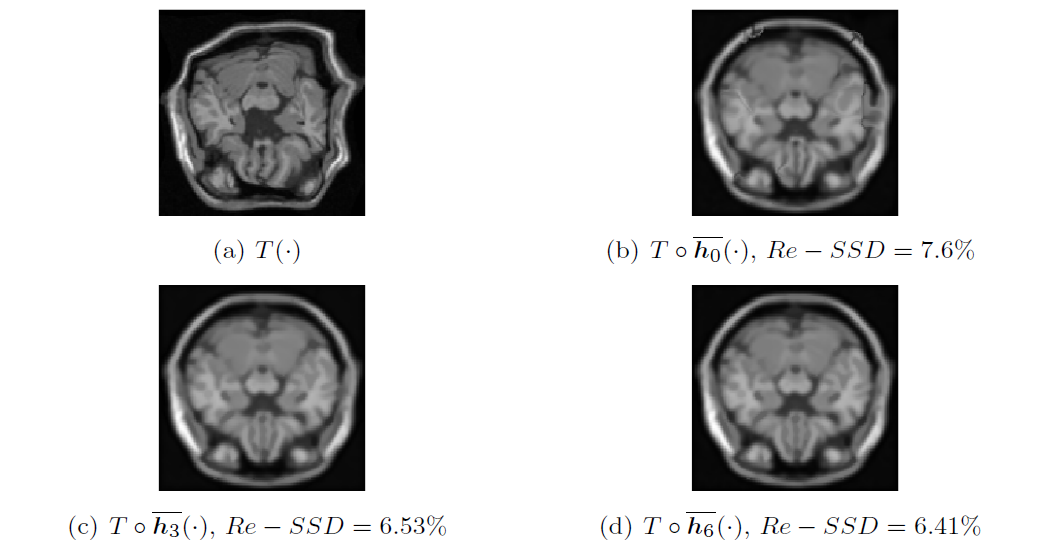
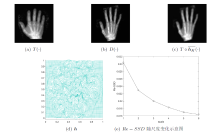
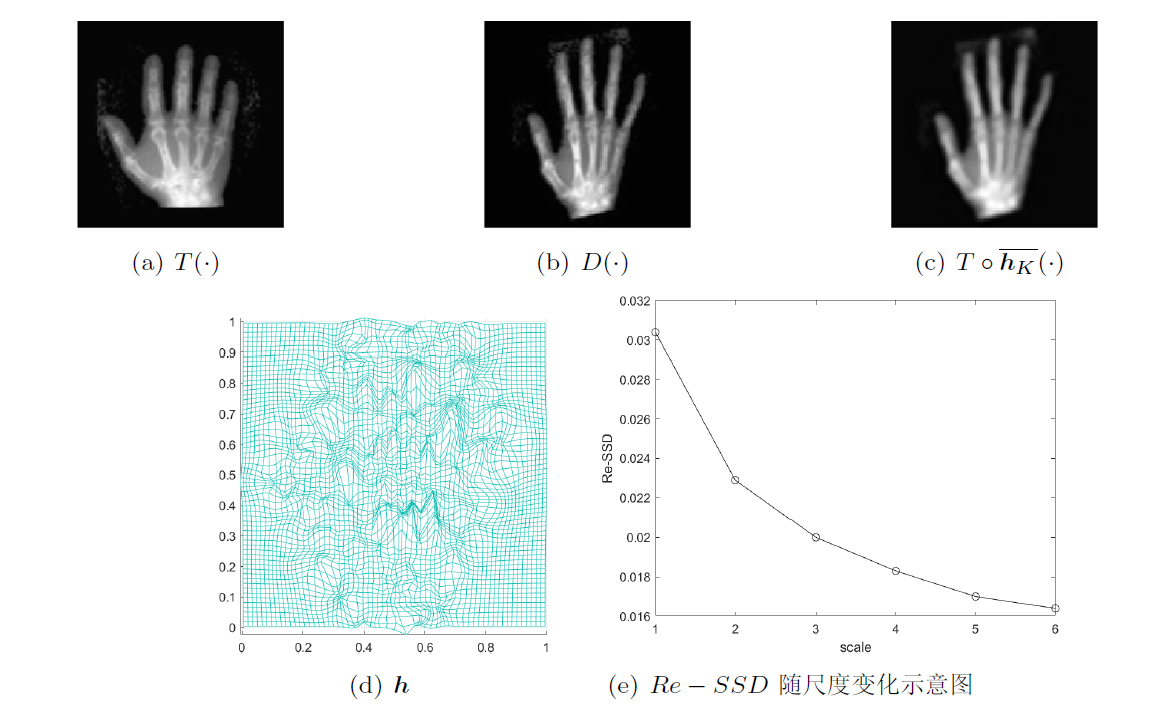
图像配准领域存在着两大挑战: (1) 网格重叠现象; (2) 贪婪配准问题不适定. 针对这两大挑战, 该文提出了一个基于拟共形理论的多尺度分数阶微分同胚图像配准模型, 该模型在无网格重叠及先验正则项的前提下, 得到了相似性度量泛函的一个光滑极小值点. 此外, 该文证明了所提模型解的存在性及多尺度方法的收敛性, 并通过数值实验验证了所提算法能有效避免网格重叠并得到较好的配准结果.
中图分类号:
- TP391
引用本文
王慧楠, 韩欢. 基于拟共形理论的分数阶多尺度微分同胚图像配准[J]. 数学物理学报, 2024, 44(6): 1665-1688.
Wang Huinan, Han Huan. Multi-Scale Approach for Diffeomorphic Image Registration with Fractional-Order Regularization Based on Quasiconformal Theory[J]. Acta mathematica scientia,Series A, 2024, 44(6): 1665-1688.
使用本文
| [1] | Brown L G. A survey of image registration techniques. ACM Computing Surveys, 1992, 24(4): 325-376 |
| [2] | Lester H, Arridge S R. A survey of hierarchical non-linear medical image registration. Pattern Recognition, 1998, 32(1): 129-149 |
| [3] |
Maintz J B, Viergever M A. A survey of medical image registration. Medical Image Analysis, 1998, 2(1): 1-36
doi: 10.1016/s1361-8415(01)80026-8 pmid: 10638851 |
| [4] |
Mohamed A, Zacharaki E I, Shen D, et al. Deformable registration of brain tumor images via a statistical model of tumor-induced deformation. Medical Image Analysis, 2006, 10(5): 752-763
pmid: 16860588 |
| [5] |
Sotiras A, Davatzikos C, Paragios N. Deformable medical image registration: a survey. IEEE Transactions on Medical Imaging, 2013, 32(7): 1153-1190
doi: 10.1109/TMI.2013.2265603 pmid: 23739795 |
| [6] | Zitova B, Flusser J. Image registration methods: a survey. Image and Vision Computing, 2003, 21(11): 977-1000 |
| [7] | Budhiraja A, Dupuis P, Maroulas V. Large deviations for stochastic flows of diffeomorphisms. Bernoulli, 2010, 16(1): 234-257 |
| [8] | Chen Y M, Shi J L, Rao M, et al. Deformable multi-modal image registration by maximizing rényi's statistical dependence measure. Inverse Problems and Imaging, 2015, 9(1): Article 79 |
| [9] | Chen Y M, Ye X J. Inverse consistent deformable image registration. The Legacy of Auadi Ramakrishnan in the Mathematical Sciences, 2010: 419-440 |
| [10] | Yang X M, Shen C M, Li F, et al. A combination of the total variation filter and a fourth-order filter for image registration. Mathematical Problems in Engineering, 2015, 1: 235134 |
| [11] | Zhang D P, Chen K. A novel diffeomorphic model for image registration and its algorithm. Journal of Mathematical Imaging and Vision, 2018, 60: 1261-1283 |
| [12] | Zhang J P, Chen K. Variational image registration by a total fractional-order variation model. Journal of Computational Physics, 2015, 293: 442-461 |
| [13] | Zhang J, Chen K, Yu B. An improved discontinuity-preserving image registration model and its fast algorithm. Applied Mathematical Modelling, 2016, 40(23/24): 10740-10759 |
| [14] | Zhang J, Chen K, Yu B. A novel high-order functional based image registration model with inequality constraint. Computers and Mathematics with Applications, 2016, 72(12): 2887-2899 |
| [15] | Machado J T, Kiryakova V, Mainardi F. Recent history of fractional calculus. Communications in Nonlinear Science and Numerical Simulation, 2011, 16(3): 1140-1153 |
| [16] | Abdeljawad T. On conformable fractional calculus. Journal of Computational and Applied Mathematics, 2015, 279: 57-66 |
| [17] | Han H. A variational model with fractional-order regularization term arising in registration of diffusion tensor image. Inverse Problems and Imaging, 2018, 12(6): 1263-1291 |
| [18] | Metzler R, Klafter J. The random walk's guide to anomalous diffusion: a fractional dynamics approach. Physics Reports, 2000, 339(1): 1-77 |
| [19] | Han H, Wang Z Q. An alternating direction implicit scheme of a fractional-order diffusion tensor image registration model. Applied Mathematics and Computation, 2019, 356: 105-118 |
| [20] | Haber E, Modersitzki J. Numerical methods for volume preserving image registration. Inverse Problems, 2004, 20(5): 1621-1638 |
| [21] | Lui L M, Thiruvenkadam S, Wang Y L, et al. Optimized conformal surface registration with shape-based landmark matching. SIAM Journal on Imaging Sciences, 2010, 3(1): 52-78 |
| [22] | Lui L M, Wong T W, Zeng W, et al. Optimization of surface registrations using Beltrami holomorphic flow. Journal of Scientific Computing, 2012, 50(3): 557-585 |
| [23] | Lam K C, Lui L M. Landmark and intensity-based registration with large deformations via quasi-conformal maps. SIAM Journal on Imaging Sciences, 2014, 7(4): 2364-2392 |
| [24] | Han H, Wang Z P, Zhang Y M. Multi-scale approach for two-dimensional diffeomorphic image registration. Multiscale Modeling and Simulation, 2021, 19(4): 1538-1572 |
| [25] | Lehto O, Virtanen K I. Quasiconformal Mappings in the Plane. New York: Springer, 1973 |
| [26] | Gardiner F P, Lakic N. Quasiconformal Teichmuller Theory. Providence RI: American Mathematical Society, 2000 |
| [27] | Ahlfors L V. Lectures on Quasiconformal Mappings. Providence RI: American Mathematical Society, 2006 |
| [28] | Aubert G, Kornprobst P, Aubert G. Mathematical Problems in Image Processing:Partial Differential Equations and the Calculus of Variations. New York: Springer, 2006 |
| [29] | Han H, Wang Z P. A diffeomorphic image registration model with fractional-order regularization and Cauchy-Riemann constraint. SIAM Journal on Imaging Sciences, 2020, 13(3): 1240-1271 |
| [30] | Hou T L, Tang T, Yang J. Numerical analysis of fully discretized Crank-Nicolson scheme for fractional-in-space Allen-Cahn equations. Journal of Scientific Computing, 2017, 72: 1214-1231 |
| [31] | Tian W Y, Zhou H, Deng W H. A class of second order difference approximations for solving space fractional diffusion equations. Mathematics of Computation, 2015, 84(294): 1703-1727 |
| [32] | Barrett R, Berry M, Chan T F, et al. Templates for the Solution of Linear Systems: Building Blocks for Iterative Methods. Philadelphia: Society for Industrial and Applied Mathematics, 1994 |
| [33] | Paige C C, Saunders M A. Solution of sparse indefinite systems of linear equations. SIAM journal on numerical analysis, 1975, 12(4): 617-629 |
| [34] | Burger M, Modersitzki J, Ruthotto L. A hyperelastic regularization energy for image registration. SIAM Journal on Scientific Computing, 2013, 35(1): 132-148 |
| [35] | Kelley C T. Iterative Methods for Optimization. Philadelphia: Society for Industrial and Applied Mathematics, 1999 |
| [36] | Nocedal J, Wright S J. Numerical Optimization. Berlin: Springer, 2006 |
| [37] | Sun W, Yuan Y X. Optimization Theory and Methods:Nonlinear Programming. Berlin: Springer, 2006 |
| [38] | Modersitzki J. FAIR: Flexible Algorithms for Image Registration. Philadelphia: Society for Industrial and Applied Mathematics, 2009 |
| [39] | Pock T, Urschler M, Zach C, et al. A duality based algorithm for TV-L1-optical-flow image registration//International Conference on Medical Image Computing and Computer-Assisted Intervention. Berlin: Springer, 2007: 511-518 |
| [40] | Kroon D J. Multimodality Non-rigid Demon Algorithm Image Registration. MatlabCentral. http://www.mathworks.com/matlabCentral/fileexchange/21451-multimodality-non-rigid-demon-algorithm-image-registration, 2008 |
| [41] | Kroon D J, Slump C H. MRI modalitiy transformation in demon registration//IEEE International Symposium on Biomedical Imaging: From Nano to Macro. Boston: IEEE, 2009: 963-966 |
| [1] | 张伟, 陈柯元, 毋祎, 倪晋波. 多项 Caputo 分数阶微分方程 Dirichlet 问题 Lyapunov 型不等式[J]. 数学物理学报, 2024, 44(6): 1433-1444. |
| [2] | 张怡然, 黎定仕. 脉冲分数阶格点系统的不变测度[J]. 数学物理学报, 2024, 44(6): 1563-1576. |
| [3] | 高晓茹, 李建军, 徒君. 一类带有时变系数的分数阶扩散方程解的爆破性[J]. 数学物理学报, 2024, 44(5): 1230-1241. |
| [4] | 王俊杰. 空间分数阶 KGS 方程组的辛差分格式[J]. 数学物理学报, 2024, 44(5): 1319-1334. |
| [5] | 张潇, 张宏武. 分数阶椭圆方程反边值问题的分数 Tikhonov 正则化方法[J]. 数学物理学报, 2024, 44(4): 978-993. |
| [6] | 宾茂君, 施翠云. 半线性Riemann-Liouville分数阶发展方程反馈时间最优控制[J]. 数学物理学报, 2024, 44(3): 687-698. |
| [7] | 孙小春, 吴育联, 徐郜婷. 分数阶不可压缩 Navier-Stokes-Coriolis 方程解的整体适定性[J]. 数学物理学报, 2024, 44(3): 737-745. |
| [8] | 周悦, 杨燕. 分数阶 Fourier 变换的测不准原理[J]. 数学物理学报, 2024, 44(2): 257-264. |
| [9] | 罗肖义, 韩欢, 张贻民. 基于ADI格式的多尺度图像分解[J]. 数学物理学报, 2024, 44(1): 160-172. |
| [10] | 王文霞. 带有 p-Laplacian 算子的分数阶非线性积分边值问题的唯一正解与和算子方法[J]. 数学物理学报, 2023, 43(6): 1731-1743. |
| [11] | 徐斐, 张勇. 分数阶 Burgers 方程时间周期弱解的唯一性与渐近稳定性[J]. 数学物理学报, 2023, 43(6): 1710-1722. |
| [12] | 李仁华, 王征平. 含强制位势的分数阶薛定谔泊松方程的正规化解[J]. 数学物理学报, 2023, 43(6): 1723-1730. |
| [13] | 唐炎娟. 分数阶抛物方程整体解的径向对称性与单调性[J]. 数学物理学报, 2023, 43(5): 1409-1416. |
| [14] | 丁自娟,韩欢. 基于多尺度方法的多模态微分同胚图像配准[J]. 数学物理学报, 2023, 43(5): 1620-1640. |
| [15] | 杨帆, 曹英, 李晓晓. 时空分数阶扩散波动方程的初值识别问题[J]. 数学物理学报, 2023, 43(2): 377-398. |
|