新 (3+1) 维 KP 方程的退化解与相互作用解

数学物理学报 ›› 2024, Vol. 44 ›› Issue (6): 1520-1536.

新 (3+1) 维 KP 方程的退化解与相互作用解

郭艳凤^1,^*(),崔静易¹(),肖海军¹(),张景军²()

¹中国地质大学 (武汉) 数学与物理学院武汉 430074
²嘉兴大学数据科学学院浙江嘉兴 314001

收稿日期:2024-01-30 修回日期:2024-04-29 出版日期:2024-12-26 发布日期:2024-11-22
通讯作者: ^*郭艳凤,Email: guoyan_feng@163.com
作者简介:崔静易, Email: cuijingyi@cug.edu.cn;|肖海军, Email: xiaohj@cug.edu.cn;|张景军, Email: zjj_math@aliyun.com
基金资助:
国家自然科学基金(11861013);国家自然科学基金(11771183);国家自然科学基金(12261053);广西科技基地与人才专项(AD21238019)

Degeneration Behaviors of Solutions and Hybrid Solutions for the New (3+1)-Dimensional KP Equation

Guo Yanfeng^1,^*(),Cui Jingyi¹(),Xiao Haijun¹(),Zhang Jingjun²()

¹School of Mathematics and Physics, China University of Geosciences, Wuhan 430074
²College of Data Science, Jiaxing University, Zhejiang Jiaxing 314001

Received:2024-01-30 Revised:2024-04-29 Online:2024-12-26 Published:2024-11-22
Supported by:
NSFC(11861013);NSFC(11771183);NSFC(12261053);Guangxi Science and Technology Base and Talent Special Project(AD21238019)

摘要/Abstract

摘要：

该文主要研究了由 Wazwaz 于 2022 年首次提出的新 (3+1) 维 KP 方程的非线性波解. 基于 Hirota 双线性形式, 利用模共振技术由$ N $-孤子解得到 $ P $-呼吸解. 然后, 利用参数极限法, 根据参数的特殊关系, 对同宿呼吸解和 $ N $-孤子解进行退化得到了 Lump 解. 最后, 从 $ N $-孤子解的部分退化出发, 研究了由呼吸解、孤子解和 Lump 解组成的相互作用解. 该文通过可视化图形展示了这些解的动力学特性.

关键词: 新 $ (3+1) $ 维 KP 方程, $ P $-呼吸解, 退化行为, Lump 解, 相互作用解

Abstract:

We concentrate on the nonlinear wave solutions of the new (3+1)-dimensional KP equation, which was firstly proposed by Wazwaz in 2022. Based on the Hirota bilinear form, the $ P $-breathing solutions are mainly obtained from the $ N $-soliton solutions utilizing the module resonance technique. Then, using parameter limit approach, the Lump solutions are derived by degenerating behaviors of the homoclinic breathing solutions and $ N $-soliton solutions on the basis of the special relations of parameters. In addition, from the partial degeneration of the $ N $-soliton solutions, some hybrid solutions are investigated by the interaction solutions among the breathing, soliton and Lump solutions.

Key words: New (3+1)-dimensional KP equation, $ P $-Breathing solutions, Degeneration behaviors, $ Q $-Lump solutions, Hybrid solutions

中图分类号:

0175.29

郭艳凤, 崔静易, 肖海军, 张景军. 新 (3+1) 维 KP 方程的退化解与相互作用解[J]. 数学物理学报, 2024, 44(6): 1520-1536.

Guo Yanfeng, Cui Jingyi, Xiao Haijun, Zhang Jingjun. Degeneration Behaviors of Solutions and Hybrid Solutions for the New (3+1)-Dimensional KP Equation[J]. Acta mathematica scientia,Series A, 2024, 44(6): 1520-1536.

图/表 14

图1

图2

图3

图4

图5

图6

图7

图8

图9

图10

图11

图12

图13

图14

参考文献 33

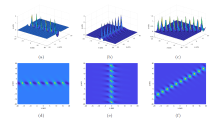
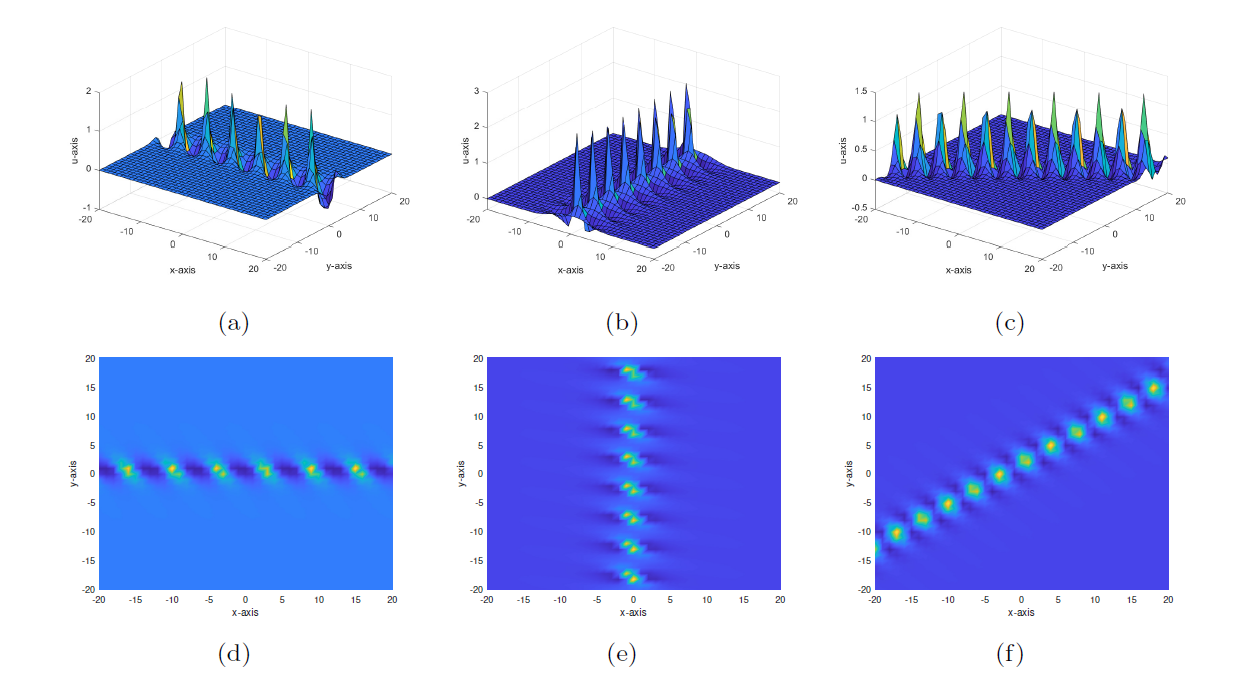
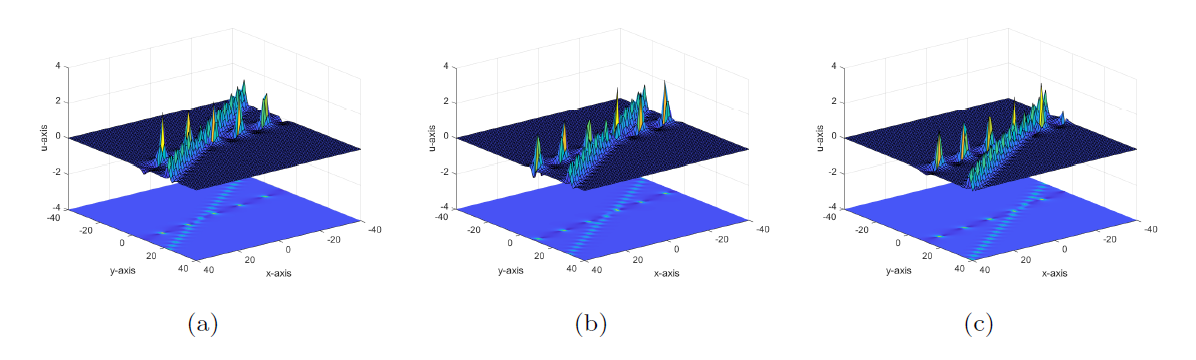
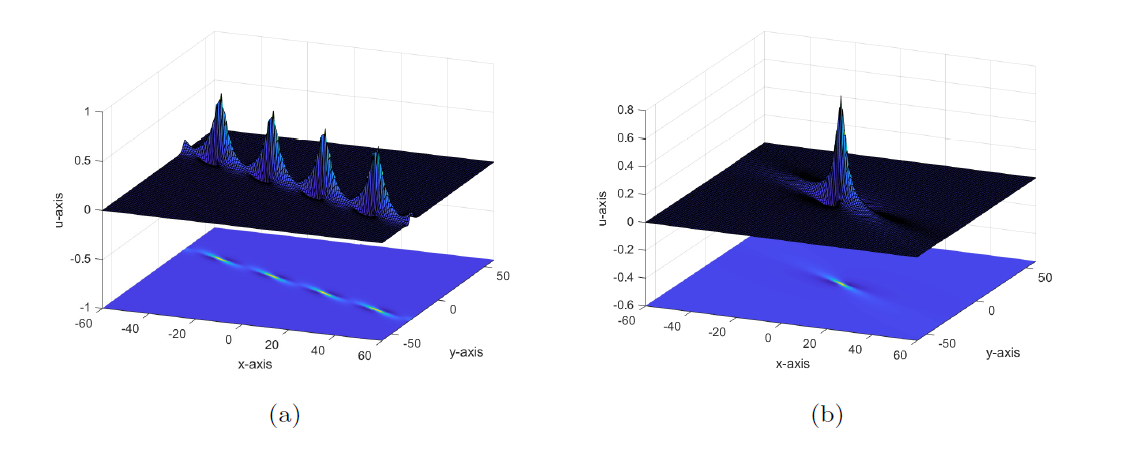
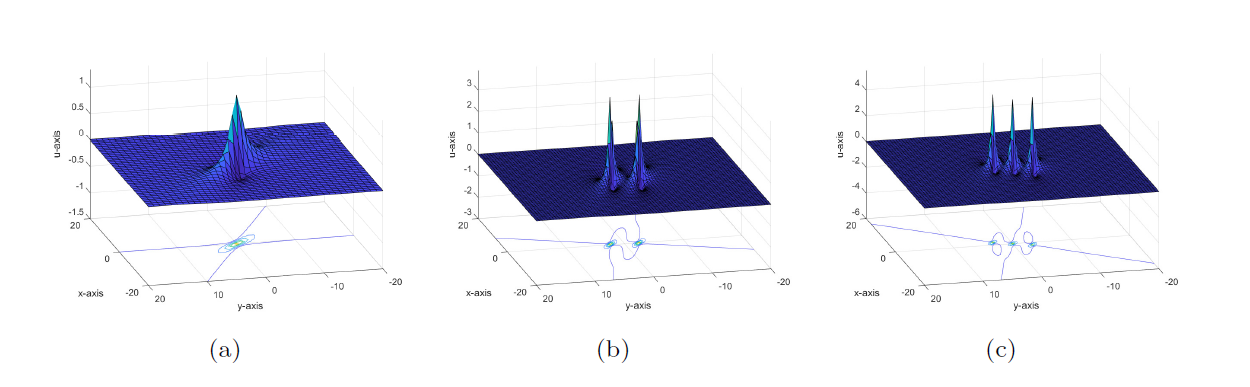
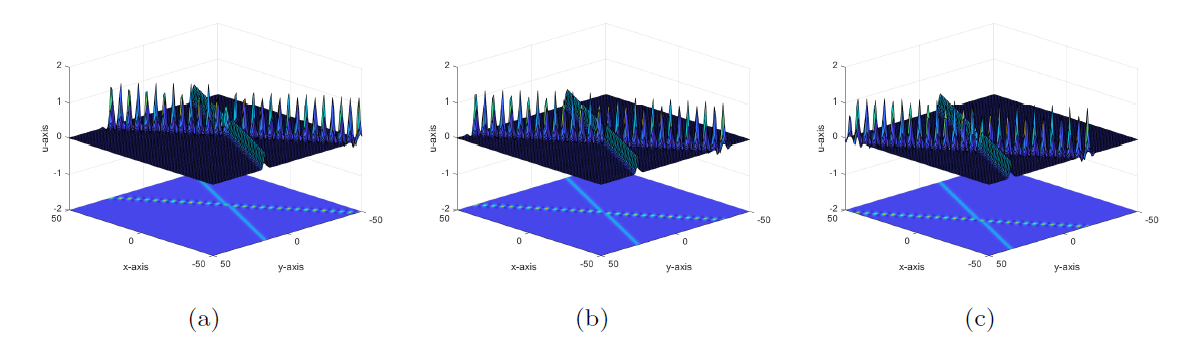
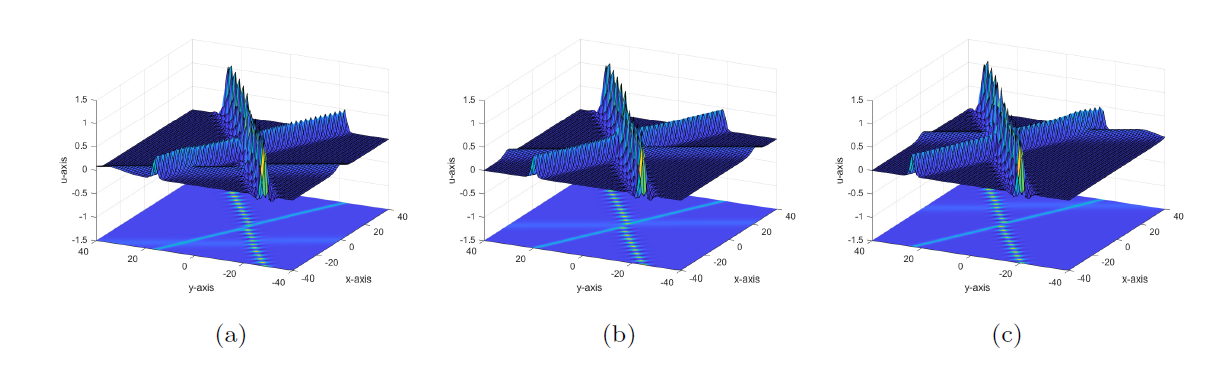
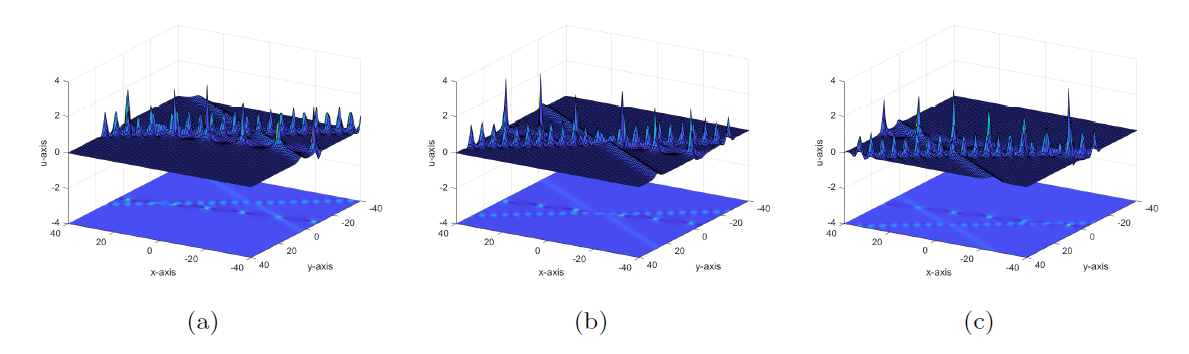
[1]	Hirota R. Exact solution of the Korteweg-de vries equation for multiple collisions of solitons. Phys Rev Lett, 1971, 27(18): 1192-1194
[2]	Staruszkiewicz A. A nonlinear modification of the Schrödinger equation. Acta Phys Pol B, 1983, 14(12): 907-909
[3]	Wazwaz A M. Painlevé analysis for new (3+1)-dimensional Boiti-Leon-Manna-Pempinelli equations with constant and time-dependent coefficients. Int J Numer Methods Heat Fluid Flow, 2020, 30(9): 4259-4266
[4]	Zhao Z H, Dai Z D, Wang C J. Extend three-wave method for the (1+2)-dimensional Ito equation. Appl Math Comput, 2010, 217(5): 2295-2300
[5]	Wazwaz A M. Integrable (3+1)-dimensional Ito equation: variety of lump solutions and multiple-soliton solutions. Nonlinear Dyn, 2022, 109(3): 1929-1934
[6]	Ma W X. Lump solutions to the Kadomtsev-Petviashvili equation. Phys Lett A, 2015, 379(36): 1975-1978
[7]	Chen S J, Lü X. Lump and lump-multi-kink solutions in the (3+1)-dimensions. Commun Nonlinear Sci Numer Simul, 2022, 109: 106103
[8]	Seadawy A R, Ahmed S, Rizvi S T, Ali K. Various forms of lumps and interaction solutions to generalized Vakhnenko Parkes equation arising from high-frequency wave propagation in electromagnetic physics. J Geom Phys, 2022, 176: 104507
[9]	Tan W, Dai Z D, Yin Z Y. Dynamics of multi-breathers, N-solitons and M-lump solutions in the (2+1)-dimensional KdV equation. Nonlinear Dyn, 2019, 96(2): 1605-1614
[10]	Wang H, Wang Y. Breather waves, rogue waves and complexiton solutions for a Zakharov-Kuznetsov equation. J Geom Phys, 2021, 167: 104286
[11]	Li L X. Degeneration of solitons for a (3+1)-dimensional generalized nonlinear evolution equation for shallow water waves. Nonlinear Dyn, 2022, 108(2): 1627-1640
[12]	Zhao Z L, He L C. M-lump,high-order breather solutions and interaction dynamics of a generalized (2+1)-dimensional nonlinear wave equation. Nonlinear Dyn, 2020, 100(3): 2753-2765
[13]	Li L X, Dai Z D, Cheng B T. Degeneration of N-soliton solutions for a (3+1)-dimensional nonlinear model in shallow water waves. Nonlinear Dyn, 2023, 111(2): 1667-1683
[14]	Zhou X, Ilhan O A, Manafian J, et al. N-lump and interaction solutions of localized waves to the (2+1)-dimensional generalized KDKK equation. J Geom Phys, 2021, 168: 104312
[15]	Hirota R. Exact envelope-soliton solutions of a nonlinear wave equation. J Math Phys, 1973, 14(7): 805-809
[16]	Cui J Y, Li D L, Zhang T F. Symmetry reduction and exact solutions of the (3+1)-dimensional nKdV-nCBS equation. Appl Math Lett, 2023, 144: 108718
[17]	Ma W X. N-soliton solutions and the Hirota conditions in (2+1)-dimensions. Opt Quant Electron, 2020, 52(12): Article 511
[18]	Zhao X, Tian B, Du X X, et al. Bilinear Bäcklund transformation, kink and breather-wave solutions for a generalized (2+1)-dimensional Hirota-Satsuma-Ito equation in fluid mechanics. Eur Phys J Plus, 2021, 136(2): 1-9
[19]	Chen X, Guo Y F, Zhang T F. Some new kink type solutions for the new (3+1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Nonlinear Dyn, 2023, 111(1): 683-695
[20]	Sulaiman T A, Yusuf A, Abdeljabbar A, Alquran M. Dynamics of lump collision phenomena to the (3+1)-dimensional nonlinear evolution equation. J Geom Phys, 2021, 169: 104347
[21]	Guo Y F, Dai Z D, Guo C X. Lump solutions and interaction solutions for (2+1)-dimensional KPI equation. Front Math China, 2022, 17(5): 875-886
[22]	Ma H C, Chen Q X, Deng A P. Soliton molecules and some novel hybrid solutions for the (2+1)-dimensional generalized Konopelchenko-Dubrovsky-Kaup-Kupershmidt equation. Commun Theor Phys, 2020, 72(9): 095001
[23]	Zhang W J, Xia T C. Solitary wave, M-lump and localized interaction solutions to the (4+1)-dimensional Fokas equation. Phys Scr, 2020, 95(4): 045217
[24]	Wazwaz A M, Alatawi N S, Albalawi W, El-Tantawy S A. Painlevé analysis for a new (3+1)-dimensional KP equation: Multiple-soliton and lump solutions. Euro Phys Lett, 2022, 140(5): 52002
[25]	Ahmad S, Khan S A, Hadi F. Damped Kadomtsev-Petviashvili equation for weakly dissipative solitons in dense relativistic degenerate plasmas. Commun Theor Phys, 2017, 68(6): 783
[26]	Fogaca D A, Navarra F S, Ferreira Filho L G. Kadomtsev-Petviashvili equation in relativistic fluid dynamics. Commun Nonlinear Sci Numer Simul, 2013, 18(2): 221-235
[27]	Ma W X, Batwa S, Manukure S. Dispersion-managed lump waves in a spatial symmetric KP model. E Asian J Appl Math, 2023, 13(2): 246-256
[28]	Ma W X. Lump waves in a spatial symmetric nonlinear dispersive wave model in (2+1)-dimensions. Mathematics, 2023, 11(22): 4664
[29]	Wang H. Lump and interaction solutions to the (2+1)-dimensional Burgers equation. Appl Math Lett, 2018, 85: 27-34
[30]	Ma W X. A novel kind of reduced integrable matrix mKdV equations and their binary Darboux transformations. Mod Phys Lett B, 2022, 36(20): 2250094
[31]	Ma W X. Linear superposition of Wronskian rational solutions to the KdV equation. Commun Theor Phys, 2021, 73(6): 065001
[32]	Ma W X. Four-component integrable hierarchies of Hamiltonian equations with ($ m+n+2 $)th-order Lax pairs. Theor Math Phys, 2023, 216(2): 1180-1188
[33]	Ma W X. Novel Liouville integrable Hamiltonian models with six components and three signs. Chin J Phys, 2023, 86: 292-299