目前, 分数阶微分方程的算法还不够成熟, 主要表现在算法需要长时间的计算历程和大空间域的计算, 成熟的算法较少, 主要集中在有限差分法与有限元方法. 因此发展新的数值算法, 提高计算效率, 解决分数阶微分方程计算量大和存储量过大等问题是学者们迫切关注的课题.

分数阶偏微分方程在物理学中广泛应用, 它们是反常扩散、信号处理和控制、图像处理和地震分析的重要数学模型. 人们对分数阶微分方程的不同数学模型的数值解非常感兴趣^[1⇓⇓-4]. 与整数阶偏微分问题相比, 处理分数阶导数是相对复杂的问题, 部分算法还存在计算时间长, 存储空间大, 精度不够, 效率不高等问题. 因此对于分数阶偏微分问题的研究还处于发展阶段. 分数阶对流-扩散方程是分数阶偏微分方程重要的一支, 源自 Montroll 和 Weiss 于 1965 年提出的连续时间随机游走模型. 此类方程在化工, 物理, 生物和医疗等领域引起普遍关注, 是流体力学, 气体动力学等重要的数学模型, 具备广阔的应用前景. 由于解析法很难得到对流-扩散方程的解析解, 对流扩散问题的有效数值解总是计算数学中一个重要的研究内容. 林等人 ^[5]应用了有限差分和谱近似方法来求解该方程. 陈等人^[6] 提出了一种小波方法来研究变系数时间分数阶对流扩散模型. Saadatmandi 等人^[7]提出了 Sinc-Legendre 配置法来求解此类方程. Yaseen 等人 ^[8]基于三次三角样条提出了有限差分方法来求解时间分数阶对流-扩散方程. 任等人^[9] 提出了四阶外推紧致差分方法来求解带有空间变系数的时间分数阶对流扩散模型. 应用配置法求解变系数时间分数阶对流-扩散方程. 还有配置法 ^[10,11], B-样条方法, 有限点等其他方法均用来求解此类方程^{[12⇓⇓⇓-16]}.

在本文中, 我们将研究具有变系数的时间分数阶对流-扩散模型

其初边值条件为

在这里, $\Omega\times J=[0,1]\times[T]$, $c(x)$, $d(x)$, $\zeta_{0}(t)$, $\zeta_{1}(t)$, $\phi(x)$ 是连续的. $0<\alpha<1$, $D_{t}^{\alpha}u(x,t)$ 为 $u(x,t)$ 的 Caputo 分数阶导数

崔明根教授和他的同事们^[17]从 1980 年成为利用再生核方法求解线性非线性问题的先导. 有许多研究者提出利用再生核方法求解各类模型. 李等人 ^[18] 通过分段再生核方法求解线性摄动延迟微分方程. 梅等人 ^[19] 结合再生核方法求解非线性微分方程. 吕等人 ^[20]和 Sahihi 等人 ^[21]运用再生核方法求解边值问题. 一些学者基于再生核方法求解热扩散方程^[22,23], 高阶扩散方程^[24], 分数阶扩散方程等 ^[25⇓-27].本文利用有限差分法逼近 Caputo 分数阶导数, 得到了该方程的半离散格式. 最后, 给出了再生核的方法.

本文包括介绍在内, 可分为 5 个部分. 第 2 部分是预备知识, 构造了再生核空间 $W_{2}^{3}[0,1]$, 随后将方程的边值条件齐次化, 得到了该方程的半离散格式. 在第 3 部分中, 我们利用再生核的方法得到了方程的近似解, 证明了该方法的稳定性和一致收敛性. 在第 4 部分中, 我们给出了三个数值例子, 以证明了该方法的有效性. 最后, 我们给本文作了一个简短的结论.

首先将上述方程的边值条件 $u(0,t)=\zeta_{0}(t)$, $u(1,t)=\zeta_{1}(t)$ 齐次化, 可得

其中 $f(x,t)=r(x,t)-D_{t}^{\alpha}h(x,t)-c(x)\frac{\partial h(x,t)}{\partial x}-d(x)\frac{\partial^{2} h(x,t)}{\partial x^{2}}$, $h(x,t)=x\zeta_{1}(t)+(1-x)\zeta_{0}(t)$.

随后利用有限差分法得到方程的半离散格式.

引理2.1^[8] 对任意的 $0<\alpha<1$, $b_{j}$ 满足如下性质

(1) $b_{j}>0, j=0,1,\cdots,n$,

(2) $1=b_{0}>b_{1}>\cdots>b_{n}$, $b_{n} \rightarrow 0, (n\rightarrow \infty)$,

(3) $\sum\limits_{j=0}^{n}(b_{j}-b_{j+1})=1$.

令 $\Delta t=\frac{1}{N}$$(N\in\mathbb{N})$ 是时间步长, $t_{n}=n \Delta t$, $(n=0$, $1$, $\cdots$, $N)$. 为了简化, 定义 $u^{n}(x)=u(x,t_{n})$, Caputo 分数阶导数 $D_{t}^{\alpha}u(x,t_{n+1})$ 的近似公式表示如下

这里 $a(\Delta t, \alpha)=\frac{1}{\Gamma(2-\alpha)(\Delta t)^{\alpha}}$, $b_{j}=(j+1)^{1-\alpha}-j^{1-\alpha}$.

将公式 (2.4) 代入公式 (2.1) 中, 则公式 (2.1) 可转化为如下形式

下面建立并求解本节中所用到的再生核空间及再生核函数.

定义2.1 再生核空间 $H_{2}^{3}[0,1]$ 为

其内积和范数为

定理2.1$R_{x}(y)$ 为 $H_{2}^{3}[0,1]$ 的再生核函数, 则其表达式为

这里

定义2.2 再生核空间 $W_{2}^{1}[0,1]$ 的定义为

其内积和范数表示为

定理2.2$Q_{x}(y)$ 是 $W_{2}^{1}[0,1]$ 的再生核函数, 其中

引入一个线性算子 $L:H_{2}^{3}[0,1]\rightarrow W_{2}^{1}[0,1]$, 对 $\forall u^{n+1}(x) \in H_{2}^{3}[0,1]$ 有

则方程 (2.5) 为

其中

下面将证明 $L$ 为线性有界算子.

定理3.1$L$ 为从空间 $H_{2}^{3}[0,1]$ 映射到 $W_{2}^{1}[0,1]$ 的线性有界算子.

证 根据定义 2.1, 可知

由 (3.2) 式, 可得

因为 $a(\Delta t,\alpha)$, $c(x)$ 和 $d(x)$ 是连续的, 所以它们在 $[0,1]$ 上有界. 此外由再生性, 可知

总之,

随后,

这里 $c$, $c_{1}$ 为常数.

令$\{x_{i}\}_{i=1}^{\infty}$为[0,1]上的稠密点集, $\psi_{x}(y)=L^\ast Q_{x}(y)$,其中$L^\ast$为$L$的共轭算子. 且$\psi_{i}(x)=L_{y}R_{x}(y)\mid_{y=x_{i}}$.

定理3.2$\{\psi_{i}(x)\}_{i=1}^{\infty}$ 在 $H_{2}^{3}[0,1]$ 中完备.

证 令 $\langle u(x),\psi_{i}(x)\rangle_{H_{2}^{3}[0,1]}=0, (i=1, 2, \cdots)$, $\forall$$u(x) \in H_{2}^{3}[0,1]$, 则

因为 $\{x_{i}\}_{i=1}^{\infty}$ 在 [0,1] 中稠密, 可以得到 $(Lu)(x)=0$. 又由 $L^{-1}$ 存在, 可得 $u(x)=0$.

在空间 $H_{2}^{3}[0,1]$ 中对 ${\{\psi_{i}}(x)\}_{i=1}^{\infty}$ 进行施密特正交化, 则可以得到 ${\{\overline{\psi_{i}}(x)}\}_{i=1}^{\infty}$,

这里 $\beta_{ik}$ 为正交系数.

定理3.3 假定 $u^{n+1}(x)$ 是方程 (3.2) 的真解, 则 $u^{n+1}(x)$ 被表示为

证

其中 $\{x_{i}\}_{i=1}^{\infty}$ 为稠密点集.

我们对精确解 $u^{n+1}(x)=\sum\limits_{i=1}^{\infty}\sum\limits_{k=1}^{i}\beta_{ik}F^{n+1}(x_{k})\overline{\psi}_{i}(x)$, 取 $m$ 项截断, 则可以得到近似解 $u_{m}^{n+1}(x)$,

引理3.1 假定 $u^{n+1}(x)$ 为方程 (3.2) 的精确解, $u^{n+1}_{m}(x)$ 为方程 (3.2) 的近似解. $\{x_{k}\}_{k=1}^{\infty}$ 是稠密点集, 则 $Lu^{n+1}(x_{k})=Lu^{n+1}_{m}(x_{k})$.

证 由 (3.4) 式我们知道

另一方面,

因此, $\sum\limits_{k=1}^{i}\beta_{ik}F^{n+1}(x_{k})=\sum\limits_{k=1}^{i}\beta_{ik}Lu^{n}_{m}(x_{k})$. 可得知 $Lu^{n}_{m}(x_{k})=F^{n+1}(x_{k})$.

定理3.4 若 $\{x_{i}\}_{i=1}^{\infty}$ 是稠密点集, 则 $\left\|u^{n+1}(x)-u^{n+1}_{m}(x)\right\|_{H_{2}^{3}[0,1]}\rightarrow0$, $(m\rightarrow\infty)$.

证 令 $B=\{u^{n+1}_{m}(x)|\left\|u^{n+1}_{m}(x)\right\|_{H_{2}^{3}[0,1]}^{2}\leq c\}$ 为 [0,1] 上的紧集且 $c>0$ 为一个常数. 由于 $\{u^{n+1}_{m}(x)\}_{m=1}^{\infty}\in B$, 则有 $p^{n+1}(x)\in B$ 和收敛子列

由引理 3.1, 可知

因此

则 $Lp^{n+1}(x_{i})=F^{n+1}(x_{i})$.

由于 $\{x_{i}\}_{i=1}^{\infty}$ 的稠密性, 有 $Lp^{n+1}(x)=F^{n+1}(x)$. 因此 $p^{n+1}(x)$ 等于精确解 $u^{n+1}(x)$, 故有

进而 $Lu^{n+1}_{m}(x)\rightarrow Lu^{n+1}(x)$. 又因 $L^{-1}$ 存在, 可知

定理3.5 $u^{n+1}(x)$ 是 (3.2) 式的解, 则由本文方法所得到的解是稳定的.

证 令 $u^{n+1}(x)$, $\overline{u}^{n+1}(x)$ 分别是下述两个方程的解

其中 $\varepsilon(x)\rightarrow0$ 是一个扰动.

$u^{n+1}(x)$ 和 $\overline{u}^{n+1}(x)$ 可被表示为

令 $\tilde{\varepsilon}=\max\limits_{0\leq x\leq1}\varepsilon(x),$

则上述两方程的近似解可表示为

可知

又因

则可得到如下式子

根据定理 3.4 知

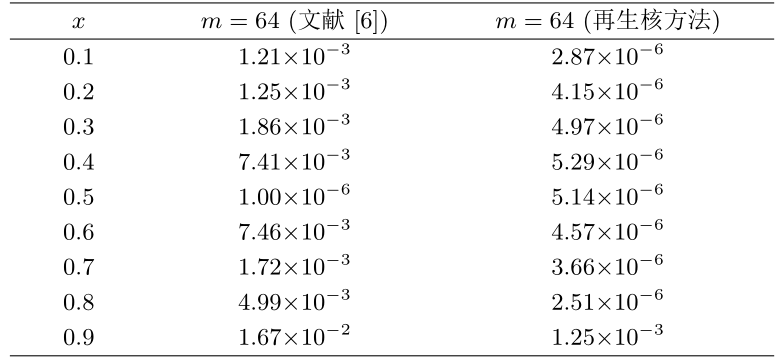
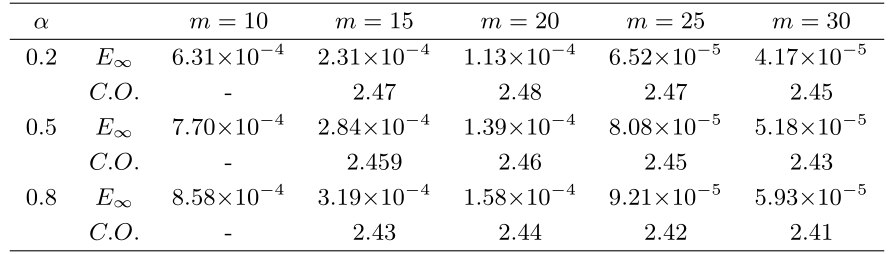
在本节中, 通过算例证明了方法的有效性, 数值运算结果由 Mathmatica 11.0 给出. 最大绝对误差 $E_{\infty}$, 绝对误差 $e_{m,n}(x)$, 及收敛阶 $C.O.$ 计算公式如下

${\bf 例 4.1}$ 考虑下面时间分数阶对流-扩散方程

其边值条件为

其初值条件为

精确解为 $u(x,t)=x^{2}+2\frac{\Gamma(\alpha+1)}{\Gamma(2\alpha+1)}t^{2\alpha}$, $r(x,t)=2t^{\alpha}+2x^{2}+2$. 当 $\alpha=0.5$, $t=0.5$ 且 $m=64$ 时, 本方法的运算结果与小波方法[6] 进行比较, 运算结果见表 1. 表 2 展示了当 $n=6$, $\alpha=0.2$, $0.5$, $0.8$ 和 $m=10$, $15$, $20$, $25$, $30$ 时的最大绝对误差和收敛阶. 最后当 $m = 5$, $\alpha = 0.5$, $n=10$, $15$, $20$, $25$, $30$ 时的绝对误差如图 1 所示.

${\bf 例 4.2}$ 考虑如下的时间分数阶对流-扩散方程

初边值条件为

这里 $r(x,t)=\frac{2t^{\alpha}}{\Gamma(3-\alpha)}+2x-2$, $u(x,t)=x^{2}+t^{2}$ 是精确解, 令 $T=1$, $n=5$, 表 3 展示了我们的方法在 $\alpha=0.2$, $0.5$, $0.8$, $m=10$, $15$, $20$, $25$, $30$ 下的绝对误差和收敛阶. 当 $\alpha=0.7$ 和 $m=20$ 时, $n=5$, $10$, $15$, $20$ 时在点 $x=0.2$, $0.4$, $0.6$, $0.8$ 处的绝对误差如表 4 所示. 图 2 展示了当 $m=20$, $t=1$, $\alpha=0.25$, $0.5$, $0.75$ 时的绝对误差. 表 5 展示了当 $T=2$, $\alpha=0.5$ 时的误差结果, 并与 Mercer[28] 的方法进行比较, 从而证得所提方法的有效性.

${\bf 例 4.3}$ 考虑此时间分数阶对流-扩散方程

边值条件为

初值条件为

精确解是 $u(x,t)=t^{2}\sin(2\pi x)$, $f(x,t)=\frac{2}{\Gamma(3-\alpha)}t^{2-\alpha}\sin(2\pi x)+4\pi^{2}t^{2}\sin(2 \pi x)$. 当 $\alpha=0.7$, $t=0.1$ 时, 由本方法得到的数值结果与配点法 ^[10]的对比情况如表 6 所示. 图 3 展示了真解 $u(x,t)$, 当 $m=50$, $t=0.3$, $0.5$, $0.7$, $0.9$ 时的近似解 $u^{n+1}_{50}(x)$, $(n=3$,$5$,$7$,$9)$ 如图 4 所示. 通过观察发现, 当 $t$ 取定即 $n$ 固定时, 近似解 $u^{n+1}_{50}(x)$ 图形的变化趋势和真解的图形吻合.

针对变系数的时间分数阶对流-扩散方程, 首先, 使用有限差分法, 将时间变量离散化, 得到了该方程的半离散格式. 之后再利用再生核方处理空间变量, 得到了方程的精确解 $u(x,t_{n})$. 然后将精确解 $u(x,t_{n})$ 取 $m$ 项截断, 得到近似解 $u_{m}(x,t_{n})$. 通过证明, 知道该方法是稳定的. 最后, 给出了几个数值例子, 并与其他文献中的方法进行了比较, 表明该算法是有效的. 在未来的工作中, 可结合再生核方法与 $\varepsilon$-最优解概念来求解更高阶的微分方程, 也可用于求解积分方程, 界面问题等. 结合再生核方法与有限差分法也可被用来求解高维的时间分数阶对流-扩散方程及其他的分数阶偏微分方程. 同时, 也可将再生核方法与其他经典的数值方法相结合来提高算法的有效性.