孤立子波在光波散射、流体力学、激波、场论、神经网络、量子力学中都有很广泛的应用^[1-8].近来，相关学者已经研究了许多求解孤立子波的方法.例如:齐次平衡法、辅助方程法、双曲函数法、椭圆函数法、(G'/G)展开法、Riccati函数法、符号计算法等^[9-13].当前, 求解非线性问题的方法已不断深化.很多科学研究者, 例如Abid等^[14], Hovhannisyan等^[15], Recke等^[16]和Graef等^[17]讨论了有关非线性问题.研究者也对一类非线性问题的激波、孤波、激光脉冲和大气物理等方面的模型作了讨论^[18-29].本文是涉及近代物理中的一个被广泛重视的非线性NNV (Nizhnik-Novikov-Veselov)动力系统微分模型, 利用了有效而简捷的泛函分析广义变分迭代方法得到了系统模型的孤立子波渐近行波解.这种方法具有广泛的应用前景.

考虑如下类广义非线性NNV动力扰动系统模型^{[10, 11]}:

(1.1) $ \begin{align} p_{t}+p_{xxx}-a_{1}pq_{x}-a_{2}qp_{y} = \varepsilon F_{1}(p, q), \end{align} $

(1.2) $ \begin{align} p_{x}-a_{3}q_{y} = \varepsilon F_{2}(p, q). \end{align} $

上式中$ \varepsilon>0 $为摄动参数, $ t, x, y $分别为时间和空间变量, $ a_{i} (i = 1, 2, 3) $为常数, $ F_{i} (i = 1, 2) $为扰动函数, 它为其变量在对应区域内是充分光滑有界函数.事实上, 在流体力学、凝聚态物理、光学和理论物理等学科中的很多应用问题都涉及到上述两个变量函数$ p, q $的三阶偏微分系统模型.一般来说, 对于这类偏微分系统模型, 难以得到其精确解的解析表示式.本文用一个简捷而有效的泛函分析变分原理的方法来求得模型(1.1)–(1.2)的解析的行波渐近解.

先对模型(1.1)–(1.2)作如下行波变换

(1.3) $ \begin{align} z = b_{1}x+b_{2}y+b_{1}t, \end{align} $

上式中$ b_{i}\ (i = 1, 2, 3) $为常数.由(1.1)–(1.2)式便得到关于$ z $的广义非线性动力系统:

(1.4) $ \begin{align} b^{3}_{1}p_{zzz}-b_{3}p_{z}-a_{1}b_{1}pq_{z}-a_{2}b_{1}qp_{z} = \varepsilon F_{1}(p, q), \end{align} $

(1.5) $ \begin{align} b_{1}p_{z}-a_{3}b_{1}q_{z} = \varepsilon F_{2}(p, q). \end{align} $

现先考虑系统(1.4)–(1.5)中的扰动项$ F_{1}(p, q) = 0, F_{2}(p, q) = 0 $的典型非线性NNV动力系统模型的情形:

(2.1) $ \begin{align} b^{3}_{1}p_{zz}-b_{3}p_{z}-a_{1}b_{1}pq_{z}-a_{2}b_{2}qp_{z} = 0, \end{align} $

(2.2) $ \begin{align} b_{1}p_{z}-a_{3}b_{2}q_{z} = 0. \end{align} $

由(2.1)–(2.2)式得到

$ b^{3}_{1}p_{zz}-b_{3}p-\frac{b_{1}(a_{1}b_{1}+a_{2}b_{2})}{2a_{3}b_{2}}p^{2}+D_{2}p = D_{1}, $

$ q = \frac{b_{1}}{a_{3}b_{2}}p+D_{2}. $

上式中$ D_1, D_2 $为任意常数, 不妨设其为零, 于是

(2.3) $ \begin{align} b^{3}_{1}p_{zz}-b_{3}p-\frac{b_{1}(a_{1}b_{1}+a_{2}b_{2})}{2a_{3}b_{2}}p^{2} = 0, \end{align} $

(2.4) $ \begin{align} q = \frac{b_{1}}{a_{3}b_{2}}p. \end{align} $

再用双曲函数待定系数法来求得方程(2.3)的孤立子波解.设

(2.5) $ \begin{align} p(z) = A_{1}{\rm sech}\ z+A_{2}{\rm sech}^{2}z+B_{0}\tanh z, \end{align} $

上式中$ A_{1}, A_{2}, B_{0} $为待定常数.由(2.5)式, 有

(2.6) $ \begin{align} p_{z} = -A_{1}{\rm sech}\ z\tanh z-2A_{2}{\rm sech}^{2}z\tanh z+B_{0}\tanh\ z, \end{align} $

(2.7) $ \begin{align} p_{zz} = A_{1}{\rm sech}\ z+4A_{2}{\rm sech}^{2}z-2A_{1}{\rm sech}^{3}z-6A_{2}{\rm sech}^{4}z -2B_{0}{\rm sech}^{2}z\tanh z. \end{align} $

将(2.5)–(2.7)式代入(2.3)式, 合并同类项并令对应项系数为零得

$ (b^{3}_{1}-b_{3})A_{1} = 0, {\quad} (4b^{3}_{1}-b_{3})A_{2}-\frac{b_{1}(a_{1}b_{1}+a_{2}b_{2})}{2a_{3}b_{2}}A^{2}_{1}+B^{2}_{0} = 0, $

$ (\frac{b_{1}(a_{1}b_{1}+a_{2}b_{2})}{2a_{3}b_{2}}A_{2}+b^{3}_{1})A_{1} = 0, {\quad} \frac{b_{1}(a_{1}b_{1}+a_{2}b_{2})}{2a_{3}b_{2}}A^{2}_{2}+6b^{3}_{1}A_{2} = 0, $

$ b_{3}B_{0} = 0, {\quad} \frac{b_{1}(a_{1}b_{1}+a_{2}b_{2})}{2a_{3}b_{2}}B^{2}_{0} = 0. $

因此得到

$ A_{1} = 0, \ \ A_{2} = -\frac{12a_{3}b^{2}_{1}b_{2}}{a_{1}b_{1}+a_{2}b_{2}}, \ \ B_{0} = 0, \ \ b_{3} = 4b^{3}_{1}. $

由此便得到行波变换(1.3)为

(2.8) $ \begin{align} z = b_{1}x+b_{2}y-4b^{3}_{1}t. \end{align} $

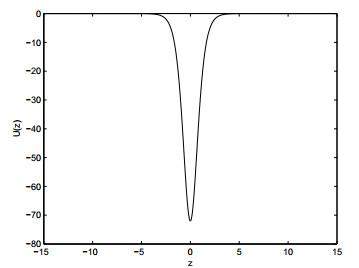
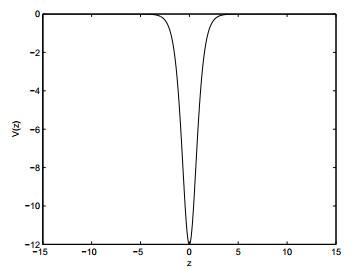
于是由(2.5)式, 得到系统(2.3)–(2.4)的一组孤立子波解$ p = U(z), q = V(z) $:

(2.9) $ \begin{align} U(z) = -\frac{12a_{3}b^{2}_{1}b_{2}}{a_{1}b_{1}+a_{2}b_{2}}{\rm sech}^{2}z, \end{align} $

(2.10) $ \begin{align} V(z) = -\frac{12b^{3}_{1}}{a_{1}b_{1}+a_{2}b_{2}}{\rm sech}^{2}z. \end{align} $

取$ a_{1} = -1, a_{2} = 1, a_{3} = 3, b_{1} = 1, b_{2} = 2 $, 由(2.9)和(2.10)式可分别决定$ p = U(z), $$ q = V(z) $的孤立子波曲线如图 1, 图 2所示.

在上一节中, 我们是对典型非线性NNV系统模型求出了其行波解.现在用泛函分析广义变分法来构造非线性扰动动力系统(1.4)–(1.5)的渐近解.

先引入一组泛函$ M_{i}[p, q] (i = 1, 2) $ (参见文献[30, 31]):

(3.1) $ \begin{align} M_{1}[p, q] = p-\int^{\tau}_{-\infty}\lambda_{1}(r)[b^{3}_{1}p_{rrr}-b_{3}p_{r} -a_{1}b_{1}\overline{p}\overline{q}_{r}-a_{2}b_{2}\overline{q}\overline{p}_{r} -\varepsilon F_{1}(\overline{p}, \overline{q})]{\rm d}r, \end{align} $

(3.2) $ \begin{align} M_{2}[p, q] = q-\int^{\tau}_{-\infty}\lambda_{2}(r)[b_{1}p_{r}-a_{3}b_{2}q_{r} -\varepsilon F_{2}(\overline{p}\overline{q})]{\rm d}r, \end{align} $

上式$ \overline{p}, \overline{q} $分别为$ p, q $的限制变量^{[30, 31]}, $ \lambda_{i}\ (i = 1, 2) $是Lagrange乘子.

计算泛函(3.1)–(3.2)的广义变分$ \delta M_{i}\ (i = 1, 2) $:

$ \begin{eqnarray*} \delta M_{1}& = &\delta p-[\lambda_{1}(b^{3}_{1}\delta p-b_{3}\delta p)]|_{r = z} +[\lambda_{1r}b^{3}_{1}\delta p_{r}]|_{r = z}+[\lambda_{1rr}b^{3}_{1}\delta p]|_{r = z} \\ && +\int^{z}_{-\infty}[b^{3}_{1}\lambda_{1rrr}-b_{3}\lambda_{1r}]\delta p{\rm d}r, \end{eqnarray*} $

$ \delta M_{2} = \delta q+a_{3}b_{2}[\lambda_{2}\delta q]|_{r = z} +\int^{z}_{-\infty}\lambda_{2r}\delta q{\rm d}r. $

按照泛函分析变分原理^{[30, 31]}, 令$ M_{i}\ (i = 1, 2) $的广义变分为零$ \delta M_{i} = 0\ (i = 1, 2) $, 可得

(3.3) $ \begin{align} b^{3}_{1}\lambda_{1rrr}-b_{3}\lambda_{1} = 0, \ \ \lambda_{1}|_{r = z} = \lambda_{1r}|_{r = z} = 0, \ \ \lambda_{1rr}|_{r = z} = \frac{1}{b^{3}_{1}}, \end{align} $

(3.4) $ \begin{align} \lambda_{2r} = 0, \ \ \lambda_{2}|_{r = z} = -\frac{1}{a_{3}b_{2}}. \end{align} $

显然由(3.3)–(3.4)式有

(3.5) $ \begin{align} \lambda_{1}(r) = \sum^{3}\limits_{i = 1}C_{i}\exp(\delta_{i}r), \ \ \lambda_{2}(r) = -\frac{1}{a_{3}b_{2}}, \end{align} $

其中

(3.6) $ \begin{align} \delta_{1} = \sqrt[3]{4}, \ \ \delta_{2} = \frac{\sqrt[3]{4}(-1+\sqrt{3}{\rm i})}{2}, \ \ \delta_{3} = \frac{\sqrt[3]{4}(-1-\sqrt{3}{\rm i})}{2}, \end{align} $

(3.7) $ \begin{align} C_{1} = \frac{1}{3\sqrt[3]{4}a^{2}_{1}}, \ \ C_{2} = \frac{\sqrt{3}-{\rm i}}{6\sqrt[3]{4}a^{2}_{1}}, \ \ C_{3} = \frac{\sqrt{3}+{\rm i}}{6\sqrt[3]{4}a^{2}_{1}}. \end{align} $

由(3.1)–(3.5)式, 构造系统(1.4)–(1.5)孤立子波渐近解的迭代关系式($ n = 0, 1, \cdots $):

(3.8) $ \begin{align} \begin{array}[b]{rl} p_{n+1}(z) = &{ } p_{n}(z) -\int^{z}_{-\infty}\bigg[\sum^{3}\limits_{i = 1}C_{i}\exp(\delta_{i}r)\bigg][b^{3}_{1}(p_{nrrr}-4p_{nr})\\ &{ } -a_{1}b_{1}p_{n}q_{nr}-a_{2}b_{2}p_{nr}-\varepsilon F_{1}(p_{n}, q_{n})]{\rm d}r, \end{array} \end{align} $

(3.9) $ \begin{align} q_{n+1}(z) = q_{n}(z) -\frac{1}{a_{3}b_{2}}\int^{z}_{-\infty}[b_{1}p_{nr}-a_{3}b_{2}q_{br} -\varepsilon F_{2}(p_{n}, q_{n})]{\rm d}r, \end{align} $

上式中$ \delta_{i}, C_{i} $由(3.6)和(3.7)式表示, $ (p_{0}(z), q_{0}(z)) $为系统(1.4)–(1.5)的孤立子波渐近解的初始迭代.

现取孤立子波渐近解的初始迭代为系统(1.4)–(1.5)的一组孤立子波解(2.9)–(2.10).即

(3.10) $ \begin{align} p_{0}(z) = -\frac{12a_{3}b^{2}_{1}b_{2}}{a_{1}b_{1}+a_{2}b_{2}}{\rm sech}^{2}z, \end{align} $

(3.11) $ \begin{align} q_{0}(z) = -\frac{12b^{3}_{1}}{a_{1}b_{1}+a_{2}b_{2}}{\rm sech}^{2}z, \end{align} $

由迭代式(3.8), (3.9), 依次可得到序列$ (p_{n}(z), q_{n}(z)) $.再由泛函分析不动点理论不难证明$ \lim\limits_{n\rightarrow \infty}p_{n} $及$ \lim\limits_{n\rightarrow \infty}q_{n} $在$ z\in[-M, M] $上一致地成立^{[32, 33]}, 上式中$ M $为任意大的正常数.

由迭代式(3.8), (3.9), 取$ p(z) = \lim\limits_{n\rightarrow \infty}p_{n}(z), q(z) = \lim\limits_{n\rightarrow \infty}q_{n}(z) $.显然它就是微分系统(1.4)–(1.5)的一组孤立子波精确解^{[30, 31]}.因此行波变换式(2.8), $ (p(b_{1}x+b_{2}y-4b^{3}t), $$ q(b_{1}x+b_{2}y-4b^{3}t)) $就是非线性广义扰动动力系统模型(1.1)–(1.2)的一组孤立子波行波解.而且$ (p_{n}(b_{1}x+b_{2}y-4b^{3}t), q_{n}(b_{1}x+b_{2}y-4b^{3}t)) $就是非线性广义扰动动力系统模型(1.1)–(1.2)的一组第$ n $次孤立子波行波渐近解, 再由泛函分析变分原理的Eular定理^[32-33]知, 本方法得到的渐近解具有较高的近似度.

考虑如下的一个特殊的动力系统模型

(4.1) $ \begin{equation} p_z(t)+p_{zzz}+pq_{z}-qp_{z} = \varepsilon\sin p, \end{equation} $

(4.2) $ \begin{equation} -p_{z}-3q_{z} = \varepsilon\cos q, \end{equation} $

由(3.10)–(3.11)式, 动力系统(4.1)–(4.2)的一个孤立子波渐近解的初始近似$ (p_{0}(z), q_{0}(z)) $是

(4.3) $ \begin{equation} p_{0}(z) = -36{\rm sech}^{2}z, \end{equation} $

(4.4) $ \begin{equation} q_{0}(z) = -12{\rm sech}^{2}z. \end{equation} $

用泛函分析广义变分迭代法，由(3.8)–(3.9)式可得动力系统模型(4.1)–(4.2)孤立子波渐近解的一次渐近解$ (p_{1}(z), q_{1}(z)) $为

(4.5) $ \begin{equation} p_{1}(z) = -36{\rm sech}^{2}z +\varepsilon\int^{z}_{-\infty}\bigg[\sum^{3}\limits_{i = 1} C_{i}\exp(\delta_{i}r)\bigg]\sin(-36{\rm sech}^{2}r){\rm d}r-\varepsilon\sin p_{0}, \end{equation} $

(4.6) $ \begin{equation} q_{1}(z) = -12{\rm sech}^{2}z+\varepsilon\int^{z}_{-\infty}\int^{z}_{-\infty} [\cos(12{\rm sech}^{2}r)]{\rm d}r-\varepsilon\cos q_{0}, \end{equation} $

上式中$ \delta_{1} = \sqrt[3]{4}, $$ \delta_{2} = \frac{\sqrt[3]{4}(-1+\sqrt{3}{\rm i})}{2}, $$ \delta_{3} = \frac{\sqrt[3]{4}(-1+-\sqrt{3}{\rm i})}{2}, $$ C_{1} = \frac{1}{3\sqrt[3]{4}}, $$ C_{2} = \frac{\sqrt{3}-{\rm i}}{6\sqrt[3]{4}}, $$ C_{3} = -\frac{\sqrt{3}+{\rm i}}{6\sqrt[3]{4}}. $

再由迭代式(3.8)–(3.9)和(4.5)–(4.6), 可得到系统模型(4.1)–(4.2)的一组孤立子波的二阶渐近解$ (p_{2}(z), q_{2}(z)) $:

(4.7) $ \begin{eqnarray} p_{2}(z)& = &{ } -36{\rm sech}^{2}z +\varepsilon\int^{z}_{-\infty}\bigg[\sum^{3}\limits_{i = 1} C_{i}\exp(\delta_{i}r)\bigg]\sin(-36{\rm sech}^{2}r){\rm d}r{}\\ &&{ } -\int^{z}_{-\infty}\bigg[\sum^{3}\limits_{i = 1}C_{1}\exp(\delta_{i}r)\bigg] [a^{3}_{i = 1} (p_{1rrr}-4p_{1r})+p_{1}q_{1r}-2q_{1}-\varepsilon \sin p_{1}]{\rm d}r, \end{eqnarray} $

(4.8) $ \begin{eqnarray} q_{2}(z)& = &-12{\rm sech}^{2}z+\varepsilon\int^{z}_{-\infty} [\cos(12{\rm sech}^{2}r)]{\rm d}r -\int^{z}_{-\infty}[b_{1}p_{1r}-a_{3} b_{2}q_{1r}-\varepsilon\cos q_{1}]{\rm d}r. \end{eqnarray} $

上式中$ p_{1}, q_{1} $由(4.5), (4.6)式表示.

由(2.8)式利用行波变换式$ s = x+2y-4t $代入(4.5)–(4.8)式, 便得到非线性广义扰动动力系统模型(4.1)–(4.2)的孤立子波一阶、二阶渐近行波解$ (p_{1asy}(x, y, t), q_{1asy}(x, y, t)) $及$ (p_{2asy}(x, y, t), $$ q_{2asy}(x, y, t)) $:

$ \begin{eqnarray*} p_{1asy}(x, y, t)& = &-36{\rm sech}^{2}(x+2y+4t) \\ &&+\varepsilon\int^{(x+2y+4t)}_{-\infty} \bigg[\sum^{3}\limits_{i = 1} C_{i}{\rm exp}(\delta_{i}r)\bigg]\sin(-36{\rm sech}^{2}(x+2y+4t)){\rm d}r -\varepsilon\sin p_{0}, \\ q_{1asy}(x, y, t)& = &-12{\rm sech}^{2}(x+2y+4t) +\varepsilon\int^{x+2y+4t}_{-\infty} [\cos(12{\rm sech}^{2}r)]{\rm d}r-\varepsilon\cos q_{0}, \\ p_{2asy}(x, y, t)& = &-36{\rm sech}^{2}(x+2y+4t) +\varepsilon\int^{x+2y+4t}_{-\infty} \bigg[\sum^{3}\limits_{i = 1} C_{i}{\rm exp}(\delta_{i}r)\bigg]\sin(-36{\rm sech}^{2}r){\rm d}r\\ &&-\int^{x+2y+4t}_{-\infty}\int^{x+2y+4t}_{-\infty}[a^{3}_{i = 1} (p_{1rrr}-4p_{1r})+p_{1}q_{1r}-2q_{1}-\varepsilon \sin p_{1}]{\rm d}r, \\ q_{2asy}(x, y, t)& = &-12{\rm sech}^{2}(x+2y+4t) +\varepsilon\int^{x+2y+4t}_{-\infty}[\cos(12{\rm sech}^{2}r)]{\rm d}r\\ &&-\int^{x+2y+4t}_{-\infty}[b_{1}p_{1r}-a_{3} b_{2}q_{1r}-\varepsilon\cos q_{1}]{\rm d}r. \end{eqnarray*} $

上式中$ p_1, q_1 $分别由(4.5), (4.6)式表示.

继续用本泛函分析变分迭代法, 可以得到非线性广义扰动动力系统模型(4.1)–(4.2)的一组任意$ n $次孤立子波$ p_{nasy}(x, y, t), q_{nasy}(x, y, t) $渐近行波解.由摄动理论和变分原理^{[32, 33]}知, 得到的渐近解在自变量任意的有限的区域内的$ n $次孤立子波渐近行波解$ p_{nasy}(x, y, t) $, $ q_{nasy}(x, y, t) $与系统模型的精确解$ p(x, y, t), q(x, y, t) $有如下的渐近估计式:

$ \begin{array}{c}p(x, y, t) = p_{nasy}(x, y, t)+O(\varepsilon^{n+1}), \ \ 0<\varepsilon\ll 1, \\ q(x, y, t) = q_{nasy}(x, y, t)+O(\varepsilon^{n+1}), \ \ 0<\varepsilon\ll 1. \end{array} $

广义泛函分析变分迭代法求扰动动力系统模型的孤立子波的渐近解是一个简捷有效的方法.由它得到的渐近解不同于简单的离散数值解.因为它还可以进行解析运算，它还可继续对模型解作定性, 定量的分析.例如通过广义扰动动力系统模型的孤立子波的渐近解, 进行某些微分，积分等解析运算，可以得到其它相关的物理量，从而扩大了对相应物理量的研究范围.另外，本文选取初始近似是用非扰动情形下的典型系统的孤立子波解.它决定了广义扰动动力系统模型比较快地得对应的孤立子波在所要求的精度范围内的渐近解析解.