1 引言
2 模型介绍
表 1 模型的参数定义
| 参数 | 定义 |
| 出生率 | |
| 与潜伏者接触的传播概率 | |
| 与感染者接触的传播概率 | |
| 有意识的易感者接触率调节因子 | |
| 易感者由无意识转为有意识的速率 | |
| 潜伏者转为隔离者的转移率 | |
| 潜伏者转为感染者的转移率 | |
| 感染者被隔离的速率 | |
| 接受治疗的速率 | |
| 治疗失败的速率 | |
| 自然死亡率 | |
| 因病死亡率 |
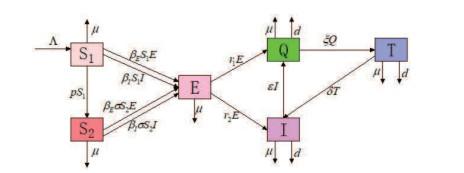
图 1
根据模型的传播机制图得如下模型
3 正性和不变集
模型中考虑的种群是人类, 给出模型的正性和不变集是非常重要的. 现将系统(2.1) 改写成矩阵的形式
其中
容易得到
根据文献[18]中的引理2可知, 对于任意的
对于系统(2.1), 不难得到
因此
是系统(2.1) 的不变集. 我们将在不变集
4 平衡点分析
4.1 无病平衡点与基本再生数
令系统(2.1) 的右端等于零, 则可以得到系统唯一的无病平衡点
下面利用基本再生矩阵的方法(参见文献[19]), 计算系统(2.1) 的基本再生数.
令
其中
其中
则基本再生数为
其中
4.2 地方病平衡点的存在性
设
由方程(4.5) 的前两个式子可得
由方程(4.5) 的最后一个式子可得
把(4.7) 式代入方程(4.5) 的第四式, 有
由方程(4.5) 的第五个式子可得
把(4.9) 式代入(4.8) 式, 则有
因为
由(4.6) 和(4.10) 式可得
将(4.11) 式代入(4.12) 式中, 有
直接求导得
令
故
所以
则
又
且
由函数
定理4.1 对于系统(2.1), 总存在一个无病平衡点
5 平衡点的全局稳定性
5.1 无病平衡点的全局稳定性
定理5.1 对于系统(2.1), 当
证 无病平衡点
则系统(2.1) 可被改写为
定义Lyapunov函数
其中
其中
令
结合(5.2) 式, 函数
故当
5.2 地方病平衡点的全局稳定性
定理5.2 对于系统(2.1), 当
证 地方病平衡点
记
系统(2.1) 可被改写为
定义Lyapunov函数
在(5.10) 式中, 只有变量
从上式可以分别求出
因此可得
根据算术平均数与几何平均数之间的关系可知, 当
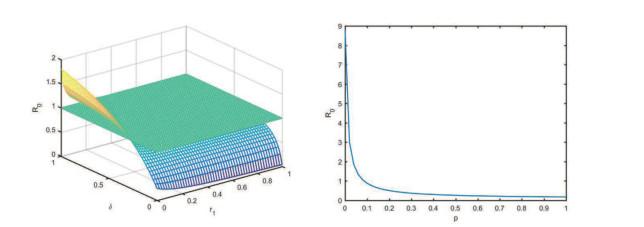
6 数值模拟
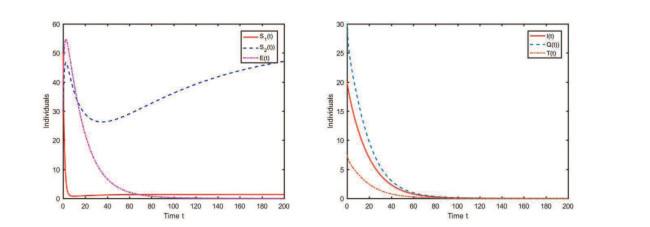
为了进一步解释说明前面得到的结论, 下面进行数值模拟. 选取的参数如下:
图 2
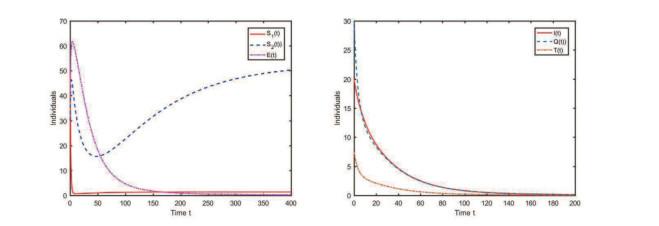
图 3
图 4
7 结论
本文建立了一类具有隔离和不完全治疗的传染病模型, 并研究了传染病的动力学行为. 通过再生矩阵的方法, 我们确定了模型的基本再生数
文中前面给出了基本再生数
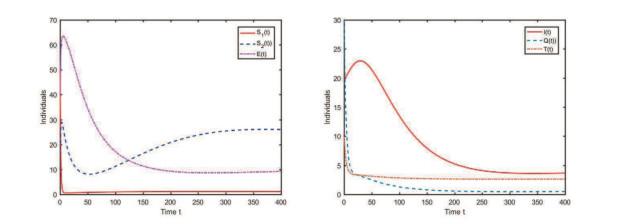
图 5
参考文献
A pneumonia outbreak associated with a new coronavirus of probable bat origin
DOI:10.1038/s41586-020-2012-7 [本文引用: 1]
Early transmission dynamics in Wuhan, China, of Noval Coronavirus-infected Pneumonia
DOI:10.1056/NEJMoa2001316 [本文引用: 1]
Stability analysis and control strategies for worm attack in mobile networks via a VEIQS propagation model
DOI:10.1016/j.amc.2019.124584 [本文引用: 1]
一类受媒体报道影响的SEIS传染病模型的定性分析
An epidemic model with saturated media/psychological impact
基于一类时滞动力学系统对新型冠状病毒肺炎疫情的建模和预测
Modeling and prediction for the trend of outbreak of NCP based on a time-delay dynamic system (in Chinese)
新型冠状病毒肺炎疫情下武汉及周边地区何时复工? 数据驱动的网络模型分析
When will be the resumption of work in wuhan and its surrounding areas during COVID-19 epidemic? A data-driven network modeling analysis (in Chinese)
Modeling and stability of epidemic model with free-living pathogens growing in the environment
Dynamics of a stochastic heroin epidemic model
一类带治疗项的非局部扩散SIR传染病模型的行波解
DOI:10.3969/j.issn.1003-3998.2020.01.008
Traveling waves in a nonlocal dispersal SIR epidemic model with treatment
DOI:10.3969/j.issn.1003-3998.2020.01.008
一类具有随机扰动的非自治SIRI流行病模型的动力学行为
DOI:10.3969/j.issn.1003-3998.2020.01.017 [本文引用: 1]
Dynamics of a nonautonomous SIRI epidemic model with random perturbations
DOI:10.3969/j.issn.1003-3998.2020.01.017 [本文引用: 1]
Global stability for an HIV/AIDS epidemic model with different latent stages and treatment
DOI:10.1016/j.apm.2012.04.013 [本文引用: 1]
The serial interval of COVID-19 among publicly reported confirmed cases
DOI:10.1101/2020.02.19.20025452 [本文引用: 1]
一类具有接种和隔离治疗的结核病模型的稳定性
DOI:10.3969/j.issn.1673-5196.2016.03.030 [本文引用: 2]
Stability of a tuberculosis model with vaccination and isolation treatment
DOI:10.3969/j.issn.1673-5196.2016.03.030 [本文引用: 2]
武汉市COVID-19疫情与易感人群软隔离强度关系分析
Analysis of the relationship between transmission of COVID-19 in Wuhan and soft quarantine intensity in susceptible population
COVID-19疫情时滞模型构建与确诊病例驱动的追踪隔离措施分析
Modelling COVID-19 epidemic with time delay and analyzing the strategy of confirmed cases-driven contact tracing followed by quarantine
Global stability of two models with incomplete for tuberculosis
Global stability of an epidemic model with incomplete treatment and vaccination
DOI:10.1155/2012/530267 [本文引用: 2]
Permanence and positive periodic solution for the single-species nonautonomous delay diffusive model
Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission
DOI:10.1016/S0025-5564(02)00108-6 [本文引用: 1]