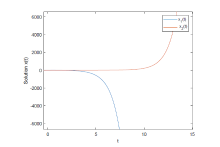
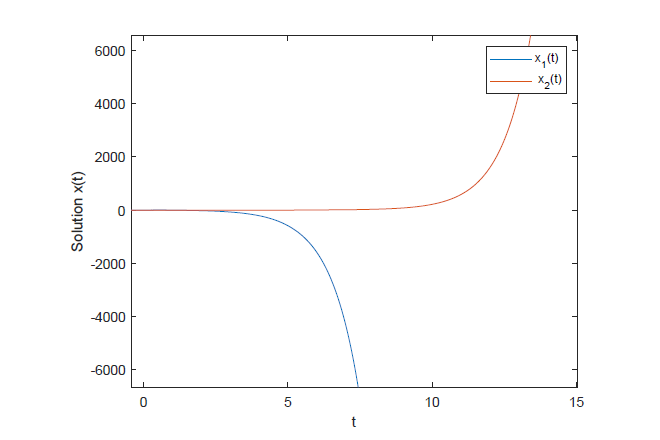
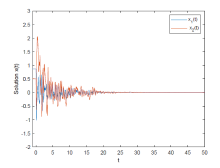
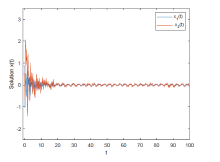
Acta mathematica scientia,Series A ›› 2024, Vol. 44 ›› Issue (6): 1607-1616.
Previous Articles Next Articles
Event-Triggered Control for a Class of Stochastic Time-Delay Nonlinear Systems
- College of Mathematics and Statistics, Hubei Normal University, Hubei Huangshi 435000
-
Received:2023-11-13Revised:2024-04-16Online:2024-12-26Published:2024-11-22 -
Supported by:Hubei Outstanding Youth Foundation(2021CFA080)
CLC Number:
- O231.2
Cite this article
Tang Jun, Wu Ailong. Event-Triggered Control for a Class of Stochastic Time-Delay Nonlinear Systems[J].Acta mathematica scientia,Series A, 2024, 44(6): 1607-1616.
share this article
| [1] | Wang P, Wang R, Su H. Stability of time-varying hybrid stochastic delayed systems with application to aperiodically intermittent stabilization. IEEE Transactions on Cybernetics, 2021, 52(9): 9026-9035 |
| [2] | Mao W, Hu L, Mao X. Razumikhin-type theorems on polynomial stability of hybrid stochastic systems with pantograph delay. Discrete and Continuous Dynamical Systems-Series B, 2020, 25(8): 3217-3232 |
| [3] | Meng Q, Ma Q, Zhou G. Adaptive output feedback control for stochastic uncertain nonlinear time-delay systems. IEEE Transactions on Circuits and Systems II: Express Briefs, 2022, 69(7): 3289-3293 |
| [4] | Zhou B, Luo W. Improved Razumikhin and Krasovskii stability criteria for time-varying stochastic time-delay systems. Automatica, 2018, 89: 382-391 |
| [5] | Sontag E D. Smooth stabilization implies coprime factorization. IEEE Transactions on Automatic Control, 1989, 34(4): 435-443 |
| [6] | Liu L, Yin S, Gao H, et al. Adaptive partial-state feedback control for stochastic high-order nonlinear systems with stochastic input-to-state stable inverse dynamics. Automatica, 2015, 51: 285-291 |
| [7] | Li X, Li P. Input-to-state stability of nonlinear systems: Event-triggered impulsive control. IEEE Transactions on Automatic Control, 2021, 67(3): 1460-1465 |
| [8] | Lu J, She Z, Feng W, et al. Stabilizability of time-varying switched systems based on piecewise continuous scalar functions. IEEE Transactions on Automatic Control, 2018, 64(6): 2637-2644 |
| [9] | 王长有, 李楠, 蒋涛, 等. 一类具有时滞及反馈控制的非自治非线性比率依赖食物链模型. 数学物理学报, 2022, 42A(1): 245-268 |
| Wang C Y, Li N, Jiang T, et al. On a nonlinear non-autonomous ratio-dependent food chain model with delays and feedback controls. Acta Math Sci, 2022, 42A(1): 245-268 | |
| [10] | 黄明辉, 刘君. 一类具有多变时滞的非线性微分系统的周期解与稳定性. 应用数学学报, 2022, 45(2): 294-306 |
| Huang M H, Liu J. Periodic solutions and stability of a class of nonlinear differential system with variable delays. Acta Mathematicae Applicatae Sinica, 2022, 45(2): 294-306 | |
| [11] | Seuret A, Briat C. Stability analysis of uncertain sampled-data systems with incremental delay using looped-functionals. Automatica, 2015, 55: 274-278 |
| [12] | Fridman E. Tutorial on Lyapunov-based methods for time-delay systems. European Journal of Control, 2014, 20(6): 271-283 |
| [13] | Min H, Xu S, Zhang B, et al. Output-feedback control for stochastic nonlinear systems subject to input saturation and time-varying delay. IEEE Transactions on Automatic Control, 2018, 64(1): 359-364 |
| [14] | Min H, Xu S, Zhang B, et al. Globally adaptive control for stochastic nonlinear time-delay systems with perturbations and its application. Automatica, 2019, 102: 105-110 |
| [15] | Ma L, Wang Z, Liu Y, et al. A note on guaranteed cost control for nonlinear stochastic systems with input saturation and mixed time-delays. International Journal of Robust and Nonlinear Control, 2017, 27(18): 4443-4456 |
| [16] | Ge X, Han Q L, Ding L, et al. Dynamic event-triggered distributed coordination control and its applications: A survey of trends and techniques. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2020, 50(9): 3112-3125 |
| [17] | Zhu W, Wang D, Liu L, et al. Event-based impulsive control of continuous-time dynamic systems and its application to synchronization of memristive neural networks. IEEE Transactions on Neural Networks and Learning Systems, 2017, 29(8): 3599-3609 |
| [18] | Zhang X M, Han Q L, Zhang B L. An overview and deep investigation on sampled-data-based event-triggered control and filtering for networked systems. IEEE Transactions on Industrial Informatics, 2016, 13(1): 4-16 |
| [19] | Ding L, Han Q L, Ge X, et al. An overview of recent advances in event-triggered consensus of multiagent systems. IEEE Transactions on Cybernetics, 2017, 48(4): 1110-1123 |
| [20] | Huong D C, Trinh H. Event-triggered state estimation for recurrent neural networks with unknown time-varying delays. International Journal of Robust and Nonlinear Control, 2022, 32(11): 6267-6281 |
| [21] | Huang Y, Deng F, Wan F. Event-triggered control for stochastic systems with multiple delays. International Journal of Robust and Nonlinear Control, 2023, 33(1): 641-658 |
| [22] | Liu Y A, Tang S, Liu Y, et al. Extended dissipative sliding mode control for nonlinear networked control systems via event-triggered mechanism with random uncertain measurement. Applied Mathematics and Computation, 2021, 396: 125901 |
| [23] | Wu X, Mu X. Event-triggered control for networked nonlinear semi-markovian jump systems with randomly occurring uncertainties and transmission delay. Information Sciences, 2019, 487: 84-96 |
| [24] | Wang Y, Xie L, De Souza C E. Robust control of a class of uncertain nonlinear systems. Systems & Control Letters, 1992, 19(2): 139-149 |
| [25] | Li B, Wang Z, Han Q L. Input-to-state stabilization of delayed differential systems with exogenous disturbances: The event-triggered case. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2017, 49(6): 1099-1109 |
| [26] | Guan Z H, Hill D J, Shen X. On hybrid impulsive and switching systems and application to nonlinear control. IEEE Transactions on Automatic Control, 2005, 50(7): 1058-1062 |
| [1] | Fan Tianjiao, Feng Lichao, Yang Yanmei. Generalization of Inequality and Its Application in Additive Time-Varying Delay Systems [J]. Acta mathematica scientia,Series A, 2024, 44(5): 1335-1351. |
| [2] | Fu Qinhong, Xiong Lianglin, Zhang Haiyang, Qin Ya, Quan Shenai. Sliding Mode Control for Time-Varying Delay Systems with Semi-Markov Jump [J]. Acta mathematica scientia,Series A, 2023, 43(2): 549-562. |
| [3] | Zaiyun Zhang,Zhenhai Liu,Youjun Deng. Global Existence and General Decay for a Nonlinear Viscoelastic Equation with Time-Varying Delay and Velocity-Dependent Material Density [J]. Acta mathematica scientia,Series A, 2021, 41(6): 1684-1704. |
| [4] | Qinghua Zhou,Li Wan,Jie Liu. Global Attracting Set for Neutral Type Hopfield Neural Networks with Time-Varying Delays [J]. Acta mathematica scientia,Series A, 2019, 39(4): 823-831. |
| [5] | Huang Zuda. Positive Pseudo Almost Periodic Solutions for a Delayed Nicholson's Blowflies Model with a Feedback Control [J]. Acta mathematica scientia,Series A, 2016, 36(3): 558-568. |
| [6] | Abdujelil Abdurahman, Jiang Haijun, Teng Zhidong. Exponential Synchronization for Impulsive Cohen-Grossberg Neural Networks with Mixed Time-Varying Delays [J]. Acta mathematica scientia,Series A, 2015, 35(3): 545-557. |
| [7] | LI Jun-Min. Adaptive Iterative Learning Control for Nonlinearly Parameterized Systems with Time-Varying Delays [J]. Acta mathematica scientia,Series A, 2011, 31(3): 682-690. |
| [8] | Xia Xuewen. Wavelet Properties of Linear Stochastic System [J]. Acta mathematica scientia,Series A, 1998, 18(S1): 144-148. |
|