具有局部阻尼的二维 Mindlin-Timoshenko 板系统的镇定

Abstract

Abstract:

In this paper, the two-dimensional Mindlin-Timoshenko plate system with local damping is studied. First, the original system is transformed into an abstract Cauchy problem, and the well posedness of the system is obtained by using operator semigroup theory. With the help of the frequency domain stability results of the linear system, the uniform exponential stability of the system is obtained by introducing geometric optical conditions and multiplier techniques.

Key words: Mindlin-Timoshenko plate system, Local damping, Piecewise multiplier method, Uniform exponential stability.

CLC Number:

O231.4

Zhang Chunguo, Sun Baonan, Fu Yuzhi, Yu Xin. Stabilization of 2-D Mindlin Timoshenko Plate Systems with Local Damping[J].Acta mathematica scientia,Series A, 2024, 44(4): 946-959.

Trendmd

Figures/Tables 1

References 16

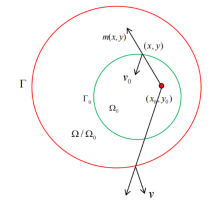
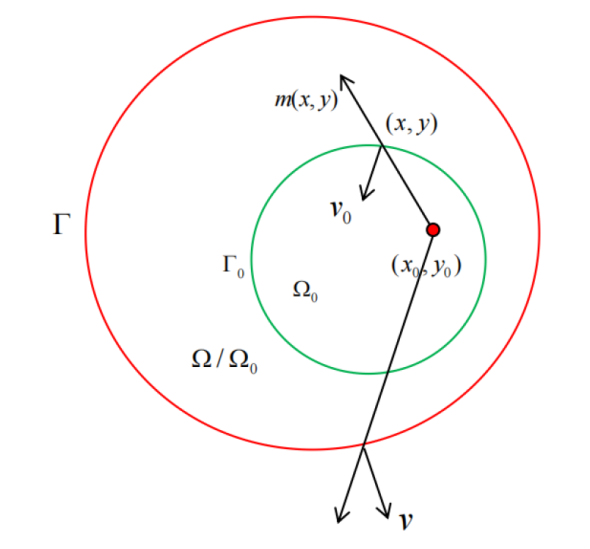
[1]	Lagnese J E. Boundary Stabilization of Thin Plates. Philadelhia, PA: Society for Industrial and Applied Mathematics, 1989
[2]	Nicaise S. Internal stabilization of a Mindlin-Timoshenko model by interior feedbacks. Mathematical Control and Related Fields, 2011, 1(3): 331-352
[3]	Rivera J E M, Oquendo H P O. Asymptotic behavior of a Mindlin-Timoshenko plate with viscoelastic dissipation on the boundary. Funkcialaj Ekvacioj, 2003, 46(3): 363-382
[4]	Dalsen G V. Stabilization of a thermoelastic Mindlin-Timoshenko plates model revisited. Zeitschrift fur angewandte Mathematik und Physik, 2013, 64(4): 1305-1325
[5]	Dalsen G V. Polynomial decay rate of a thermoelastic Mindlin-Timoshenko plates model with Dirichlet boundary conditions. Zeitschrift fur angewandte Mathematik und Physik, 2015, 66(1): 113-128
[6]	Louis T. Indirect stabilization of a Mindlin-Timoshenko plates. Journal of Mathematical Analysis and Applications, 2017, 449(2): 1880-1891
[7]	章春国, 付煜之, 刘宇标. 二维 Mindlin-Timoshenko 板系统的稳定性与最优性. 数学物理学报, 2021, 41A(5): 1465-1491
	Zhang C G, Fu Y Z, Liu Y B. Stability and optimality of 2-D Mindlin-Timoshenko plate system. Acta Math Sci, 2021, 41A(5): 1465-1491
[8]	Liu Y, Zhang C, Chen T. Stabilization of 2-d Mindlin-Timoshenko plates with localized acoustic boundary feedback. Journal of Industrial and Management Optimization, 2022, 18(2): 1009-1034
[9]	Liu K. Locally distributed control and damping for the conservative systems. SIAM Journal on Control and Optimization, 1997, 35(5): 1574-1590
[10]	Martinez P. A new method to obtain decay rate estimates for dissipative systems. ESAIM: Control, Optimisation and Calculus of Variations, 1999, 4: 419-444
[11]	Liu K, Liu Z. Exponential decay of energy of the Euler-Bernoulli beam with locally distributed Kelvin-Voigt damping. SIAM Journal on Control and Optimization, 1998, 36(3): 1086-1098
[12]	Fatiha A B. Piecewise multiplier method and nonlinear integral inequalities for Petrowsky equation with nonlinear dissipation. Journal of Evolution Equations, 2006, 6(1): 95-112
[13]	Hong G, Hong H. Stabilization of transmission system of Kirchhoff plates and wave equations with a localized Kelvin-Voigt damping. Journal of Evolution Equations, 2021, 20: 2239-2264
[14]	Pazy A. Semigroups of Linear Operators and Applications to Partial Differential Equations. New York: Springer Science and Business Media, 2012
[15]	Adams R A. Sobolev Spaces. New York: Acadamic Press, 1975
[16]	Huang F. Characteristic conditions for exponential stability of linear dynamical systems in Hilbert spaces. Ann of Differ Eqs, 1985, 1: 43-56

Stabilization of 2-D Mindlin Timoshenko Plate Systems with Local Damping

RichHTML

PDF (PC)

Abstract

Cite this article

share this article

Figures/Tables 1

References 16

Related Articles 11

Metrics

Comments

Recommended 10

[1]	Gao Caixia, Zhao Dongxia. The Delayed Control and Input-to-State Stability of ARZ Traffic Flow Model with Disturbances [J]. Acta mathematica scientia,Series A, 2024, 44(4): 960-977.
[2]	Bai Jinyan, Chai Shugen. Stabilization of Degenerate Wave Equations with Delayed Boundary Feedback [J]. Acta mathematica scientia,Series A, 2024, 44(1): 133-139.
[3]	Han Zhongjie, He Yiheng, Zhao Zhixue. Indirect Stabilization and Optimal Decay Rates of Weakly Coupled Plates with Various Types of Damping [J]. Acta mathematica scientia,Series A, 2023, 43(6): 1681-1698.
[4]	Zhang Zhipeng, Zhang Liang. Controllability of a Nonlinear Parabolic Systems with Drift-Diffusion Term [J]. Acta mathematica scientia,Series A, 2023, 43(6): 1744-1758.
[5]	Pang Yuting, Zhao Dongxia. The PDP Feedback Control and Exponential Stabilization of a Star-Shaped Open Channels Network System [J]. Acta mathematica scientia,Series A, 2023, 43(6): 1803-1813.
[6]	Pang Yuting,Zhao Dongxia,Zhao Xin,Gao Caixia. The PDP Boundary Control for a Class of 2$\times$2 Hyperbolic Partial Differential System [J]. Acta mathematica scientia,Series A, 2023, 43(5): 1471-1482.
[7]	Peng Qingqing,Zhang Zhifei. Global Attractor for a Coupled System of Wave and Euler-Bernoulli Plate Equation with Boundary Weak Damping [J]. Acta mathematica scientia,Series A, 2023, 43(4): 1179-1196.
[8]	Chunguo Zhang,Yuzhi Fu,Yubiao Liu. Stability and Optimality of 2-D Mindlin-Timoshenko Plate System [J]. Acta mathematica scientia,Series A, 2021, 41(5): 1465-1491.
[9]	Dongxia Fan,Dongxia Zhao,Na Shi,Tingting Wang. The PDP Feedback Control and Stability Analysis of a Diffusive Wave Equation [J]. Acta mathematica scientia,Series A, 2021, 41(4): 1088-1096.
[10]	Zhang Chunguo, Liu Yubiao, Liu Weiwei. Boundary Optimal Control for the Timoshenko Beam [J]. Acta mathematica scientia,Series A, 2018, 38(3): 454-466.
[11]	Xiong Jun, Li Junmin, He Chao. Fuzzy Boundary Control Design for a Class of First-Order Hyperbolic PDEs [J]. Acta mathematica scientia,Series A, 2017, 37(3): 469-477.