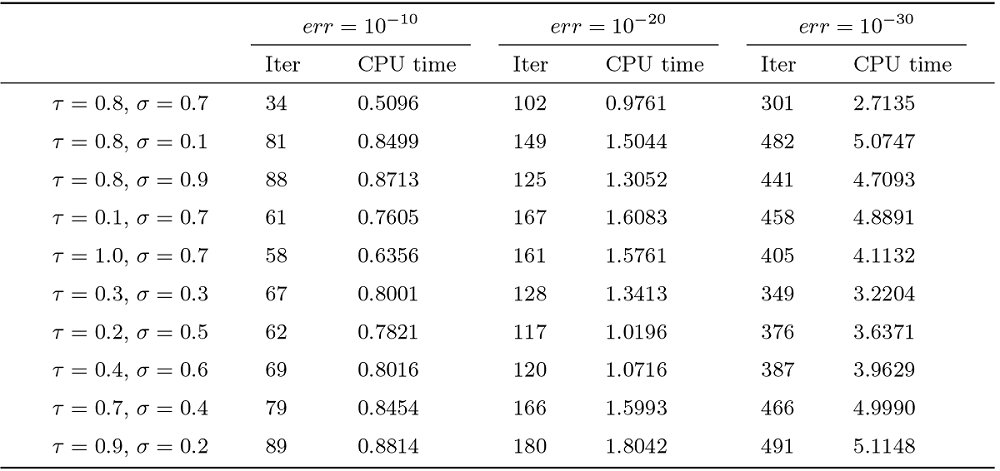
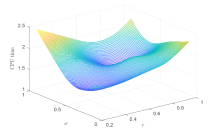
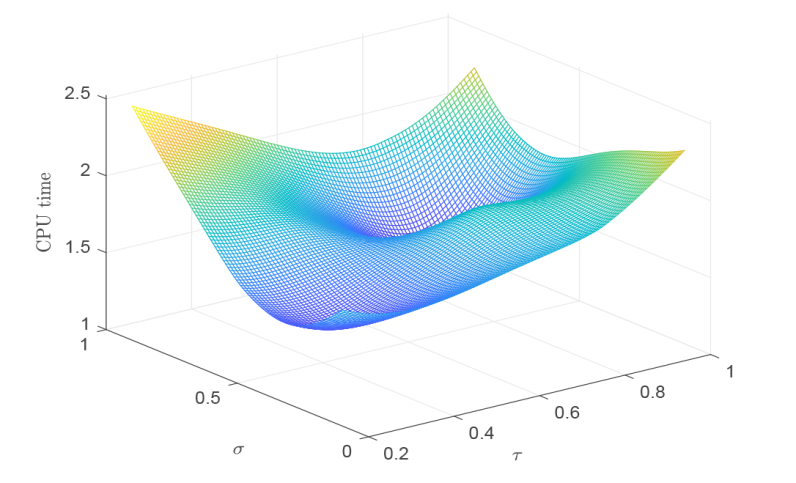
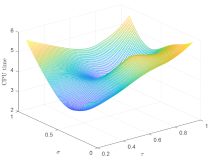
Acta mathematica scientia,Series A ›› 2024, Vol. 44 ›› Issue (4): 1080-1091.
Previous Articles Next Articles
A Golden Ratio Primal-Dual Algorithm for a Class of Nonsmooth Saddle Point Problems
- School of Mathematics and Statistics, Chongqing Technology and Business University, Chongqing 400067
-
Received:2023-06-05Revised:2024-03-25Online:2024-08-26Published:2024-07-26 -
Supported by:NSFC(11471059);NSF of Chongqing(cstc2021jcyj-msxmX0721);Team Building Project for Graduate Tutors in Chongqing(yds223010);Innovation Project for Graduate Students of Chongqing(CYS240567);Project of Chongqing Technology and Business University(ZDPTTD201908)
CLC Number:
- O224
Cite this article
Nie Jialin, Long Xianjun. A Golden Ratio Primal-Dual Algorithm for a Class of Nonsmooth Saddle Point Problems[J].Acta mathematica scientia,Series A, 2024, 44(4): 1080-1091.
share this article
| [1] | Bauschke H H, Combettes P. Convex Analysis and Monotone Operators Theory in Hilbert Spaces. New York: Springer-Verlag, 2020 |
| [2] | Chambolle A, Pock T. A first-order primal-dual algorithm for convex problems with applications to imaging. J Math Imaging Vis, 2011, 40: 120-145 |
| [3] | Combettes P, Pesquet J C. Proximal splitting methods in signal processing// Bauschke H, Burachik R, Combetles P, et al. Fixed-Point Algorithms for Inverse Problems in Science and Engineering. New York: Springer-Verlag, 2011: 185-212 |
| [4] | Uzawa H. Iterative methods for concave programming// Arrow K J, Hurwicz L, Uzawa H, eds. Studies in Linear and Nonlinear Programming. Stanford, CA: Stanford University Press, 1958, 6: 154-165 |
| [5] | Esser E, Zhang X, Chan T F. A general framework for a class of first order primal-dual algorithms for convex optimization in imaging science. SIAM J Imaging Sci, 2010, 3: 1015-1046 |
| [6] | He B, You Y, Yuan X. On the convergence of primal-dual hybrid gradient algorithm. SIAM J Imaging Sci, 2014, 7(4): 2526-2537 |
| [7] | Chambolle A, Pock T. On the ergodic convergence rates of a first-order primal-dual algorithm. Math Program, 2016, 159(1): 253-287 |
| [8] | Condat L. A primal-dual splitting method for convex optimization involving Lipschitzian, proximable and linear composite terms. J Optim Theory Appl, 2013, 158(2): 460-479 |
| [9] | Malitsky Y. Golden ratio algorithms for variational inequalities. Math Program, 2020, 184(1): 383-410 |
| [10] | Xu Y. Iteration complexity of inexact augmented Lagrangian methods for constrained convex programming. Math Program, 2021, 185: 89-117 |
| [11] | 周丹青, 常小凯, 杨俊峰. 一类新的黄金比率原始对偶算法. 高等学校计算数学学报, 2022, 44: 97-106 |
| Zhou D Q, Chang X K, Yang J F. A new Golden ratio primal-dual algorithm. Numerical Math A J Chinese Univ, 2022, 44: 97-106 | |
| [12] | Zhu Y, Liu D, Tran-Ding Q. New primal-dual algorithms for a class of nonsmooth and nonlinear convex-concave minimax problems. SIAM J Optim, 2022, 32(4): 2580-2611 |
| [13] | Hamedani E Y, Aybat N S. A primal-dual algorithm with line search for general convex-concave saddle point problems. SIAM J Optim, 2021, 31: 1299-1329 |
| [14] | Rockafellar R, Wets R. Variational Analysis. Berlin: Springer, 2004 |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 57
|
|
|||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 61
|
|
|||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||
|