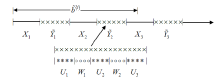
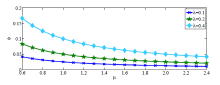
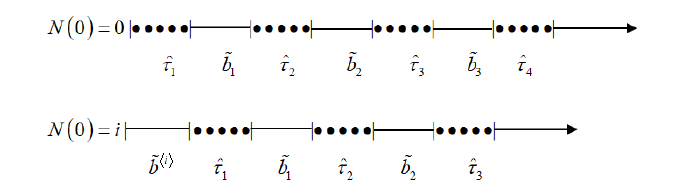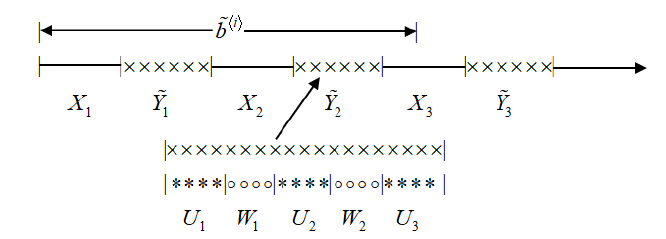Acta mathematica scientia,Series A ›› 2023, Vol. 43 ›› Issue (2): 625-645.
Previous Articles Next Articles
Analysis of M/G/1 Repairable Queueing System with a Replaceable Repair Facility, N -policy and Delayed Uninterrupted Single Vacation
He Yaxing,Tang Yinghui( ),Liu Qionglin
),Liu Qionglin
- School of Mathematical Sciences, Sichuan Normal University, Chengdu 610068
-
Received:2022-05-12Revised:2022-10-17Online:2023-04-26Published:2023-04-17 -
Supported by:NSFC(71571127);Special Project For Subject Construction of Sichuan Normal University(XKZX2021-04)
CLC Number:
- O213.2
Cite this article
He Yaxing, Tang Yinghui, Liu Qionglin. Analysis of
share this article
| [1] |
Avi-Itzhak B, Naor P. Some queueing problems with service station subject to server breakdown. Operations Research, 1963, 11: 303-320
doi: 10.1287/opre.11.3.303 |
| [2] |
Federgruen A, Green L. Queueing systems with service interruptions. Operations Research, 1986, 34: 752-768
doi: 10.1287/opre.34.5.752 |
| [3] |
史定华, 田乃硕. 服务台可修的GI/M(M/PH)/1排队系统. 应用数学学报, 1995, 18(1): 44-50
doi: 10.12387/C1995006 |
|
Shi D H, Tian N S. Repairable GI/M(M/PH)/1 queueing system. Acta Mathematicae Applicatae Sinica, 1995, 18(1): 44-50
doi: 10.12387/C1995006 |
|
| [4] | 李泉林. 服务台可修的M/SM/(PH/SM)/1排队系统. 应用数学, 1996, 9(4): 422-428 |
| Li Q L. M/SM(PH/SM)/1 repairable queueing system. Mathematica Applicatae, 1996, 9(4): 422-428 | |
| [5] |
曹晋华, 程侃. 服务台可修的M/G/1排队系统分析. 应用数学学报, 1982, 5(2): 113-127
doi: 10.12387/C1982016 |
|
Cao J H, Cheng K. Analysis of M/G/1 queueing system with repairable service station. Acta Mathematicae Applicatae Sinica, 1983, 5(2): 113-127
doi: 10.12387/C1982016 |
|
| [6] | 曹晋华. 服务设备可修的机器服务模型分析. 数学研究与评论, 1985, 5(4): 89-96 |
| Cao J H. Service model analysis of repairable service equipment. Journal of Mathematical Research and Exposition, 1985, 5(4): 89-96 | |
| [7] |
Tang Y H. Revisiting the model of servicing machines with repairable service facility-A analyzing idea and some new results. Acta Mathematicae Applicatae Sinica, English Series, 2010, 26(4): 557-566
doi: 10.1007/s10255-008-8029-6 |
| [8] | 唐应辉, 赵玮. 可修排队系统可靠性指标的分解特性. 运筹学学报, 2004, 8(4): 73-84 |
| Tang Y H, Zhao W. The decomposition properties of reliablility indices in repairable queueing systems. Operations Research Transactions, 2004, 8(4): 73-84 | |
| [9] |
Tang Y H. A single-server M/G/1 queueing system subject to breakdowns-some reliability and queueing problems. Microelectronics and Reliability, 1997, 37(2): 315-321
doi: 10.1016/S0026-2714(96)00018-2 |
| [10] | Tang Y H, Mu Y C, Yu M M. Analysis of reliability on M/G/1 repairable queueing sysyem with p-entering discipline during second type failure time-Some reliability indices. Journal of Systems Engineering, 2012, 27(4): 559-567 |
| [11] |
唐应辉, 唐小我. 推广的M/G(M/G)/1(M/G)可修排队系统(II)-一些可靠性指标. 系统工程理论与实践, 2000, 20(2): 84-91
doi: 10.12011/1000-6788(2000)2-84 |
|
Tang Y H, Tang X W. The generalized M/G(M/G)/1(M/G) repairable queueing system(II)-Some reliability indices. System Engineering-Theory and Practice, 2000, 20(2): 84-91
doi: 10.12011/1000-6788(2000)2-84 |
|
| [12] | Tang Y H. Some reliability problems arising in the repairable queueing system single delay vacation. Journal of Systems Science and Systems Engineering, 2001, 10(3): 306-314 |
| [13] |
Wang H, Wang T Y, Peam W L. Optimal control of the N policy M/G/1 queueing system with server breakdowns and general startup times. Applied Mathematical Modelling, 2007, 31(10): 2199-2212
doi: 10.1016/j.apm.2006.08.016 |
| [14] |
Wang H, Kuo C C, Ke C. Optimal control of the D-policy M/G/1 queueing system with server breakdowns. American Journal of Applied Sciences, 2008, 5(5): 565-573
doi: 10.3844/ajassp.2008.565.573 |
| [15] |
Wu W Q, Tang Y H, Yu M M, Jiang Y. Reliability analysis of a k-out-of-n: G repairable system with single vacation. Applied Mathematical Modelling, 2014, 38(19/20): 6075-6097
doi: 10.1016/j.apm.2014.05.020 |
| [16] | 钟瑶, 唐应辉. 具有两类失效模式的D -策略M/G/1可修排队系统最优控制策略D∗. 运筹学学报, 2020, 24(1): 40-56 |
| Zhong Y, Tang Y H. Analysis of the M/G/1 repairable queueing system with D-policy and two types of failure modes. Operations Research Transactions, 2020, 24(1): 40-56 | |
| [17] |
旷欣宇, 唐应辉. 带启动时间与双阈值(m,N) -策略的M/G/1可修排队系统的最优控制策略. 运筹与管理, 2021, 30(10): 64-70
doi: 10.12005/orms.2021.0315 |
|
Kuang X Y, Tang Y H. Optimal control policy for the M/G/1 repairable queueing system with random start-up time and bi-level threshold (m,N)-policy. Operations Research and Management Science, 2021, 30(10): 64-70
doi: 10.12005/orms.2021.0315 |
|
| [18] |
唐应辉, 余妙妙, 付永红. Geom/G1,G2(Geom/G)/1/1可修Erlang消失系统的可靠性指标及其计算机仿真分析. 系统工程理论与实践, 2010, 30(2): 347-355
doi: 10.12011/1000-6788(2010)2-347 |
|
Tang Y H, Yu M M, Fu Y H. Reliability indices of Geom/G1,G2(Geom/G)/1/1 repairable Erlang loss system and computer simulation analysis. System Engineering-Theory and Practice, 2010, 30( 2): 347-355
doi: 10.12011/1000-6788(2010)2-347 |
|
| [19] |
Tang Y H, Yu M M, Li C L. Geom/G1,G2/1/1 repairable Erlang loss system with catastrophe and second optional service. Journal of Systems Science and Complexity, 2011, 24(3): 554-564
doi: 10.1007/s11424-011-8339-2 |
| [20] |
Tang Y H, Yu M M, Yun X, Huang S J. Reliability indices of discrete-time GeoX/G/1 queueing system with unreliable service station and multiple adaptive delayed vacations. Journal of Systems Science and Complexity, 2012, 25(6): 1122-1135
doi: 10.1007/s11424-012-1062-9 |
| [21] |
兰绍军, 唐应辉. 具有Bernoulli反馈和Min(N,D) -策略控制的Geo(λ1,λ2)/G/1离散时间可修排队的可靠性分析. 系统科学与数学, 2016, 36(11): 2070-2086
doi: 10.12341/jssms12974 |
|
Lan S J, Tang Y H. Reliability analysis of discrete-time Geo(λ1,λ2)/G/1 repairable queue with Bernoulli feedback and Min(N,D)-policy. Journal of Systems Science and Mathematical Sciences, 2016, 36(11): 2070-2086
doi: 10.12341/jssms12974 |
|
| [22] |
Lan S J, Tang Y H. Analysis of D-policy discrete-time Geo/G/1 queue with second J-optional service and unreliable server. RAIRO-Operations Research, 2017, 51(1): 101-122
doi: 10.1051/ro/2016006 |
| [23] |
Lan S J, Tang Y H. Performance and reliability analysis of a repairable discrete-time Geo/G/1 queue with Bernoulli feedback and randomized policy. Applied Stochastic Models in Business and Industry, 2017, 33(5): 522-543
doi: 10.1002/asmb.v33.5 |
| [24] |
Lan S J, Tang Y H. An unreliable discrete-time retrial queue with probabilistic preemptive priority, balking customers and replacements of repair times. AIMS Mathematics, 2020, 5(5): 4322-4344
doi: 10.3934/math.2020276 |
| [25] |
刘金银, 唐应辉, 朱亚丽, 等. 具有温储备失效和延迟修理的M/G/1可修排队系统的可靠性指标. 系统工程理论与实践, 2015, 35(2): 413-423
doi: 10.12011/1000-6788(2015)2-413 |
|
Liu J Y, Tang Y H, Zhu Y L, et al. Reliability indices of M/G/1 repairable queueing system with warm standby failure and delayed repair. System Engineering-Theory and Practice, 2015, 35(2): 413-423
doi: 10.12011/1000-6788(2015)2-413 |
|
| [26] | 唐应辉, 刘金银, 余妙妙. N -控制策略且温储备失效M/G/1可修排队. 系统工程学报, 2015, 30(6): 852-864 |
| Tang Y H, Liu J Y, Yu M M. M/G/1 repairable with N-policy and warm standby failure. Journal of System Engineering, 2015, 30(6): 852-864 | |
| [27] |
唐应辉, 朱亚丽, 吴文青. 具有温储备失效的M/G/1可修排队系统. 系统工程理论与实践, 2014, 34(4): 944-950
doi: 10.12011/1000-6788(2014)4-944 |
|
Tang Y H, Zhu Y L, Wu W Q. M/G/1 repairable queueing system with warm standby failure. System Engineering-Theory and Practice, 2014, 34(4): 944-950
doi: 10.12011/1000-6788(2014)4-944 |
|
| [28] |
高丽君, 唐应辉. N -控制策略且温储备失效M/G/1可修排队的可靠性指标. 运筹与管理, 2018, 27(10): 102-112
doi: 10.12005/orms.2018.0237 |
|
Gao L J, Tang Y H. Reliability indices of M/G/1 repairable queue with N-policy and warm standby failure. Operations Research and Management Science, 2018, 27(10): 102-112
doi: 10.12005/orms.2018.0237 |
|
| [29] | 蔡晓丽, 唐应辉. 具有温储备失效特征和单重休假Min(N,V) -控制策略M/G/1的可修排队系统. 应用数学学报, 2017, 40(5): 692-701 |
| Cai X L, Tang Y H. M/G/1 repairable queueing system with warm standby failure and Min(N,V)-policy Based on Single vacation. Acta Mathematicae Applicatae Sinica, 2017, 40(5): 692-701 | |
| [30] |
刘仁彬, 唐应辉. 修理设备可更换且修理有延迟的两不同型部件并联可修系统. 高校应用数学学报, 2006, 21(2): 127-140
doi: 10.1007/BF02791345 |
|
Liu R B, Tang Y H. Two-different-units paralleled repairable system with a replaceable facility and repair delay. Applied Mathematics-A Journal of Chinese Universities, 2006, 21(2): 127-140
doi: 10.1007/BF02791345 |
|
| [31] |
Yu M M, Tang Y H, FuY H, Pan L M, Tang X W. A deteriorating repairable system with stochastic lead time and replaceable repair facility. Computers and Industrial Engineering, 2012, 62(2): 609-615
doi: 10.1016/j.cie.2011.11.023 |
| [32] | 唐应辉, 冯慧侠, 吴文青. 修理设备可更换的M/G/1可修排队系统分析. 系统工程学报, 2013, 28(6): 830-839 |
| Tang Y H, Feng H X, Wu W Q. Analysis of M/G/1 repairable queueing system with a replacement repair facility. Journal of Systems Engineering, 2013, 28(6): 830-839 | |
| [33] |
唐应辉, 朱亚丽, 吴文青. 修理设备可更换的N -策略M/G/1可修排队系统分析. 系统工程理论与实践, 2014, 34(3): 746-755
doi: 10.12011/1000-6788(2014)3-746 |
|
Tang Y H, Zhu Y L, Wu W Q. Analysis of M/G/1 repairable queueing system with N-policy and a replaceable repair facility. Systems Engineering-Theory and Practice, 2014, 34(3): 746-755
doi: 10.12011/1000-6788(2014)3-746 |
|
| [34] | 吴文青, 唐应辉, 兰绍军. 修理设备可更换的k/n(G)表决可修系统. 数学学报, 2016, 59(6): 799-820 |
| Wu W Q, Tang Y H, Lan S J. Analysis of k/n(G) repairable system with replaceable repair facility. Acta Mathematica Sinica, 2016, 59(6): 799-820 | |
| [35] |
Wu W Q, Tang Y H, Yu M M, Jiang Y, Liu H. Reliability analysis of a k-out-of n:G system with general repair times and replaceable repair equipment. Quality Technology and Quantitative Management, 2018, 15(2): 274-300
doi: 10.1080/16843703.2016.1226712 |
| [36] | 潘取玉, 唐应辉. 在延迟Min(N,D) -控制策略下修理设备可更换的M/G/1可修排队系统及最优控制策略. 数学物理学报, 2018, 38A(5): 1014-1031 |
| Pan Q Y, Tang Y H. Analysis of M/G/1 repairable queueing system and optimal control policy with a replaceable repair facility under delay Min(N,D)-policy. Acta Mathematica Scientia, 2018, 38A(5): 1014-1031 | |
| [37] | 魏瑛源, 唐应辉. 基于修理设备可更换和修理延迟策略的两不同型部件冷贮备可修系统. 工程数学学报, 2020, 37(4): 423-441 |
| Wei Y Y, Tang Y H. Two units cold standby repairable system with a replaceable repair facility and delay repair. Journal of Engineering Mathematics, 2020, 37(4): 423-441 | |
| [38] | 何亚兴, 唐应辉. 具有N -策略和延迟单重休假且休假不中断的M/G/1排队系统. 应用数学, 2021, 34(1): 130-145 |
| He Y X, Tang Y H. M/G/1 Queueing system with N-policy and Delayed Single vacation without interruption. Mathematica Applicata, 2021, 34(1): 130-145 | |
| [39] | 唐应辉, 唐小我. 排队论-基础与分析技术. 北京: 科学出版社, 2006 |
| Tang Y H, Tang X W. Queueing Theory-Foundations and Analysis Techniques. Beijing: Science Press, 2006 | |
| [40] | 曹晋华, 程侃. 可靠性数学引论(修订版). 北京: 高等教育出版社, 2006 |
| Cao J H, Cheng K. Introdution to Reliability Mathematics (revised edition). Beijing: Higher Education Press, 2006 |
| [1] | Quyu Pan,Yinghui Tang. Analysis of M/G/1 Repairable Queueing System and Optimal Control Policy with a Replaceable Repair Facility Under Delay Min(N, D)-Policy [J]. Acta mathematica scientia,Series A, 2018, 38(5): 1014-1031. |
|