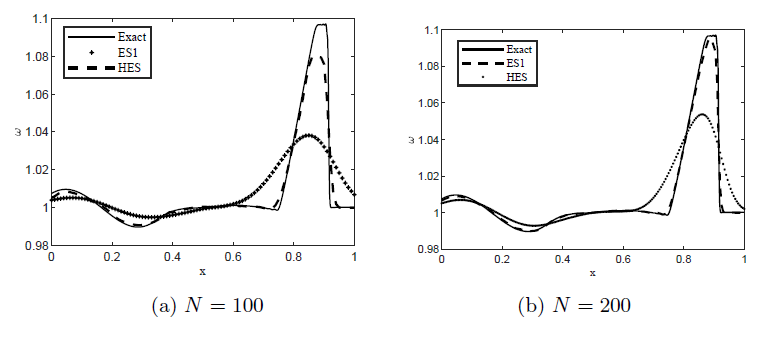
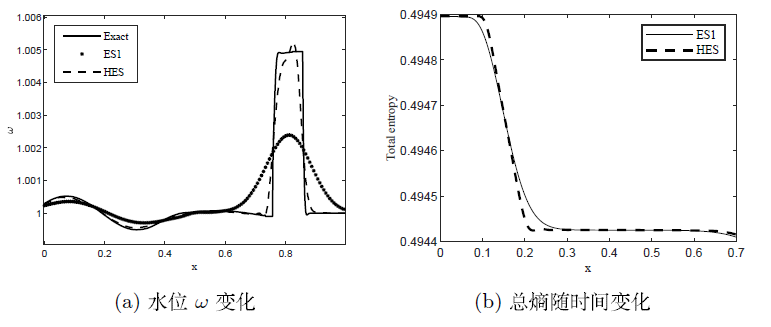
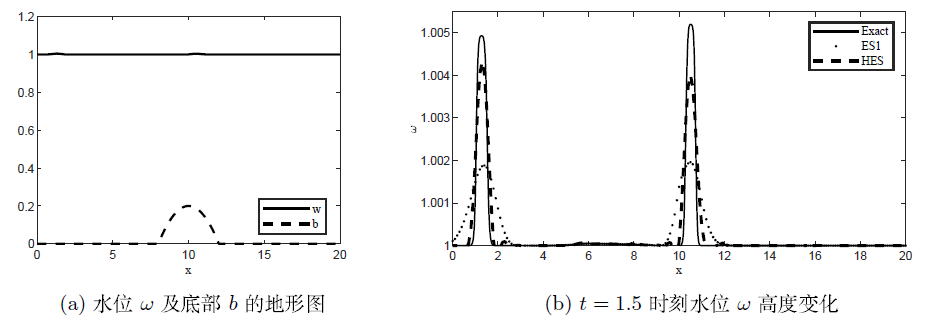
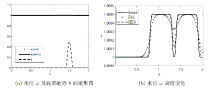
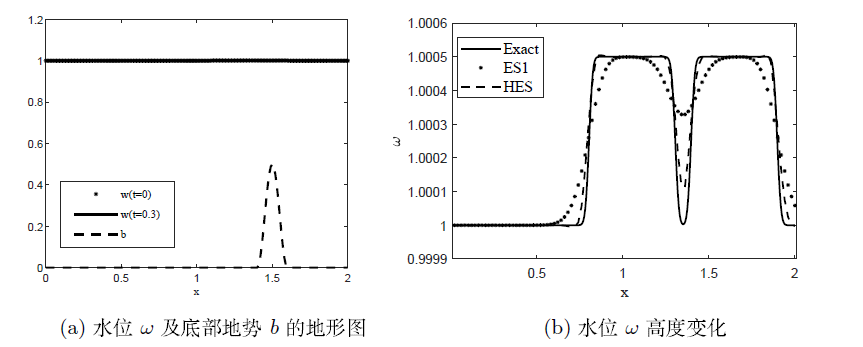
| [1] |
Lin G F, Lai J S, Guo W D. Performance of high-resolution TVD schemes for 1D dam-break simulations. J Chin Inst Eng, 2005, 28(5): 771-782
doi: 10.1080/02533839.2005.9671047
|
| [2] |
Xing Y L, Shu C W. High order finite difference WENO schemes with the exact conservation property for the shallow water matrixs. J Comput Phys, 2005, 208(1): 206-227
doi: 10.1016/j.jcp.2005.02.006
|
| [3] |
Kesserwani G, Liang Q H. A conservative high-order discontinuous Galerkin method for the shallow water matrixs with arbitrary topography. Int J Numer Meth Eng, 2011, 86(1): 47-69
doi: 10.1002/nme.v86.1
|
| [4] |
郑素佩, 李霄, 赵青宇, 等. 求解二维浅水波方程的旋转混合格式. 应用数学和力学, 2022, 43(2): 176-186
|
|
Zheng S P, Li X, Zhao Q Y, et al. A rotated mixed scheme for solving 2D shallow water matrixs. Appl Math Mech, 2022, 43(2): 176-186
|
| [5] |
Kurganov A, Levy D. Central-upwind schemes for the Saint-Venant system. Math Model Anal, 2002, 36(3): 397-425
|
| [6] |
Liu X, Albright J, Epshteyn Y, et al. Well-balanced positivity preserving central-upwind scheme with a novel wet/dry reconstruction on triangular grids for the Saint-Venant system. J Comput Phys, 2018, 374: 213-236
doi: 10.1016/j.jcp.2018.07.038
|
| [7] |
Kurganov A, Noelle S, Petrov A G. Semidiscrete central-upwind schemes for hyperbolic conservation laws and Hamilton-Jacobi matrixs. SIAM J Sci Comput, 2001, 23(3): 707-740
doi: 10.1137/S1064827500373413
|
| [8] |
董建. 中心格式在 Saint-Venant 方程组上的应用研究. 数学物理学报, 2019, 39A(2): 372-385
|
|
Dong J. Research on the application of central scheme in Saint-Venant system. Acta Math Sci, 2019, 39A(2): 372-385
|
| [9] |
Tadmor E. The numerical viscosity of entropy stable schemes for systems of conservation laws. I. Math Comput, 1987, 49(179): 91-103
|
| [10] |
Fjordholm U S, Mishra S, Tadmor E. Well-balanced and energy stable schemes for the shallow water matrixs with discontinuous topography. J Comput Phys, 2011, 230(14): 5587-5609
doi: 10.1016/j.jcp.2011.03.042
|
| [11] |
张海军, 封建湖, 程晓晗, 等. 带源项浅水波方程的高分辨率熵稳定格式. 应用数学和力学, 2018, 39(8): 935-945
|
|
Zhang H J, Feng J H, Cheng X H, et al. An entropy stable scheme for shallow water matrixs with source terms. Appl Math Mech, 2018, 39(8): 935-945
|
| [12] |
Fjordholm U S, Mishra S, Tadmor E. Arbitrarily high-order accurate entropy stable essentially non-oscillatory schemes for systems of conservation laws. SIAM J Numer Anal, 2012, 50(2): 544-573
doi: 10.1137/110836961
|
| [13] |
郑素佩, 建芒芒, 封建湖, 等. 保号WENO-AO型中心迎风格式. 计算物理, http://kns.cnki.net/kcms/detail/11.2011.O4.20220322.1639.008.html
|
|
Zheng S P, Jian M M, Feng J H, et al. Sign preserving WENO-AO-type central upwind schemes. Chinese J Comput Phys, http://kns.cnki.net/kcms/detail/11.2011.O4.20220322.1639.008.html
|
| [14] |
Lax P D. Weak solutions of nonlinear hyperbolic matrixs and their numerical computation. Commun Pur Appl Math, 1954, 7(1): 159-193
doi: 10.1002/(ISSN)1097-0312
|
| [15] |
Fjordholm U S, Ray D. A sign preserving WENO reconstruction method. J Sci Comput, 2016, 68(1): 42-63
doi: 10.1007/s10915-015-0128-y
|
| [16] |
Shu C W. Essentially non-oscillatory and weighted essentially non-oscillatory schemes. Acta Numer, 2020, 29: 701-762
doi: 10.1017/S0962492920000057
|
| [17] |
郑素佩, 徐霞, 封建湖, 等. 高阶保号熵稳定格式. 数学物理学报, 2021, 41A(5): 1296-1310
|
|
Zheng S P, Xu X, Feng J H, et al. High order sign preserving stable schemes. Acta Math Sci, 2021, 41A(5): 1296-1310
|
| [18] |
徐霞. 求解双曲守恒律方程的任意阶保号熵稳定格式研究. 西安:长安大学, 2021
|
|
Xu X. The Research of Arbitrary High-Order Sign Prserving Entropy Stable Schemes for Hyperbolic Conservation Laws. Xi'an: Chang'an University, 2021
|
| [19] |
Leveque R J. Balancing source terms and flux gradients in high-resolution Godunov methods: the quasi-steady wave-propagation algorithm. J Comput Phys, 1998, 146(1): 346-365
doi: 10.1006/jcph.1998.6058
|
| [20] |
罗一鸣, 李订芳, 刘敏, 等. 一种维持 Saint-Venant 方程组移动稳态解的中心格式. 数学物理学报, 2022, 42A(3): 891-903
|
|
Luo Y M, Li D F, Liu M, et al. Moving-water equilibria preserving central scheme for the Saint-Venant system. Acta Math Sci, 2022, 42A(3): 891-903
|
 ),Zheng Supei(
),Zheng Supei( ),Feng Jianhu(
),Feng Jianhu( ),Zhai Mengqing(
),Zhai Mengqing( )
)