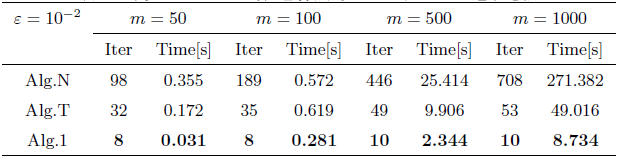
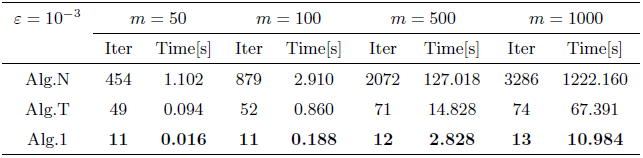
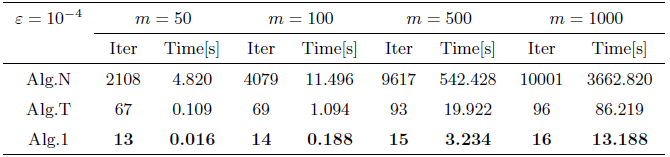
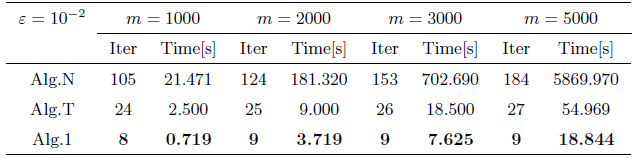
Acta mathematica scientia,Series A ›› 2023, Vol. 43 ›› Issue (1): 274-290.
Previous Articles Next Articles
A New Tseng-like Extragradient Algorithm for Common Solutions of Variational Inequalities and Fixed Point Problems
- School of Mathematical Science, Sichuan Normal University, Chengdu 610066
-
Received:2022-05-23Revised:2022-09-22Online:2023-02-26Published:2023-03-07
CLC Number:
- O244
Cite this article
Duan Jie, Xia Fuquan. A New Tseng-like Extragradient Algorithm for Common Solutions of Variational Inequalities and Fixed Point Problems[J].Acta mathematica scientia,Series A, 2023, 43(1): 274-290.
share this article
| [1] | Goldstein A A. Convex programming in Hilbert space. Bull New Ser Am Math Soc, 1964, 70(5): 109-112 |
| [2] | Korpelevich G M. The extragradient method for finding saddle points and other problems. Ekonomikai Matematicheskie Metody, 1976, 12: 747-756 |
| [3] |
Tseng P. A modified forward-backward splitting method for maximal monotone mapping. SIAM J Control Optim, 2000, 38(2): 431-446
doi: 10.1137/S0363012998338806 |
| [4] |
Cai G, Yekini S, Iyiola O S. Inertial Tseng's extragradient method for solving variational inequality problems of pseudo-monotone and non-lipschitz operators. J Ind Manag Optim, 2021, 1: 1-30
doi: 10.3934/jimo.2005.1.1 |
| [5] |
Moudafi A. Viscosity approximation methods for fixed-points problems. J Math Anal Appl, 2000, 241: 46-55
doi: 10.1006/jmaa.1999.6615 |
| [6] | Yamada I. The hybrid steepest descent method for the variational inequality problem over the intersection of fixed point sets of nonexpansive mappings//Butnariu D, Censor Y, Reich S, Eds. Inherently Parallel Algorithms in Feasibility and Optimization and Their Application. New York: Elservier, 2001: 473-504 |
| [7] | Halpern B. Fixed points of nonexpanding maps. Bull Amer Math, 1967, 73: 957-961 |
| [8] |
Ishikawa S. Fixed points by a new iteration method. Proc Amer Math Soc, 1974, 44: 147-150
doi: 10.1090/S0002-9939-1974-0336469-5 |
| [9] |
Marino G, Xu H K. A general iterative method for nonexpansive mappings in Hilbert spaces. J Math Anal Appl, 2006, 318: 43-52
doi: 10.1016/j.jmaa.2005.05.028 |
| [10] |
Tian M. A general iterative method for nonexpansive mappings in Hilbert spaces. Nonlinear Anal, 2010, 73(3): 689-694
doi: 10.1016/j.na.2010.03.058 |
| [11] |
Ke Y F, Ma C F. The generalized viscosity implicit rules of nonexpansive mappings in Hilbert spaces. Fixed Point Theory Appl, 2015, 2015: 190
doi: 10.1186/s13663-015-0439-6 |
| [12] |
Nadezhkina N, Takahashi W. Weak convergence theorem by an extragradient method for nonexpansive mappings and monotone mappings. J Optim Theory Appl, 2006, 128(1): 191-201
doi: 10.1007/s10957-005-7564-z |
| [13] |
Thong D V, Hieu D V. Inertial subgradient extragradient algorithms with line-search process for solving variational inequality problems and fixed point problems. Numer Algor, 2019, 80(4): 1283-1307
doi: 10.1007/s11075-018-0527-x |
| [14] |
Ceng L C, Shang M J. Hybrid inertial subgradient extragradient methods for variational inequalities and fixed point problems involving asymptotically nonexpansive mappings. Optimization, 2021, 70(4): 715-740
doi: 10.1080/02331934.2019.1647203 |
| [15] | Goebel K, Reich S. Uniform Convexity, Hyperbolic Geometry, and Nonexpansive Mappings. New York: Marcel Dekker, 1984 |
| [16] | Cottle R W, Yao J C. Pseudo-monotone complementarity problems in Hilbert space. J Optim Theory Appl, 1992, 72(2): 281-295 |
| [17] |
Xu H K. Iterative algorithms for nonlinear operators. J London Math Soc, 2002, 66: 240-256
doi: 10.1112/S0024610702003332 |
| [18] |
Xu H K, Kim T H. Convergence of hybrid steepest-descent methods for variational inequalities. J Optim Theory Appl, 2003, 119(1): 185-201
doi: 10.1023/B:JOTA.0000005048.79379.b6 |
| [19] | Glowinski R, Lions J L, Trémolières R. Numerical Analysis of Variational Inequalities. Amsterdam: Elsevier, 1981 |
| [20] |
Iusem A, Otero R G. Inexact versions of proximal point and augmented lagrangian algorithms in Banach spaces. Numer Funct Anal Optim, 2001, 22: 609-640
doi: 10.1081/NFA-100105310 |
| [21] |
Denisov S V, Semenov V V, Chabak L M. Convergence of the modified extragradient method for variational inequalities with non-lipschitz operators. Cybern Syst Anal, 2015, 51: 757-765
doi: 10.1007/s10559-015-9768-z |
| [22] |
He B S, Liao L Z. Improvements of some projection methods for monotone nonlinear variational inequalities. J Optim Theory Appl, 2002, 112(1): 111-128
doi: 10.1023/A:1013096613105 |
| [23] | Sun D F. A projection and contraction method for the nonlinear complementarity problem and it's extensions. Math Numer Sinica, 1994, 16(3): 183-194 |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 118
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 90
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|