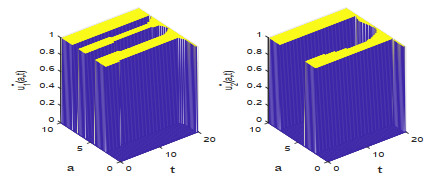
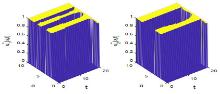
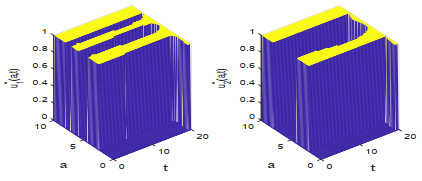
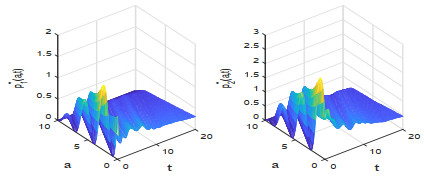
Acta mathematica scientia,Series A ›› 2022, Vol. 42 ›› Issue (1): 228-244.
Previous Articles Next Articles
Optimal Harvesting in a Competing System of Hierarchical Age-Structured Populations
- Institute of Operational Research and Cybernetics, Hangzhou Dianzi University, Hangzhou 310018
-
Received:2020-10-14Online:2022-02-26Published:2022-02-23 -
Contact:Zerong He E-mail:zrhe@hdu.edu.cn -
Supported by:the NSFC(11871185)
CLC Number:
- O211.4
Cite this article
Zerong He,Nan Zhou. Optimal Harvesting in a Competing System of Hierarchical Age-Structured Populations[J].Acta mathematica scientia,Series A, 2022, 42(1): 228-244.
share this article
| 1 | Getz W M , Haight R G . Population Harvesting: Demographic Models of Fish, Forest, and Animal Resources. Princeton: Princeton University Press, 1989 |
| 2 | Aniţa S . Analysis and Control of Age-Dependent Population Dynamics. Dordrecht: Kluwer Academic Publishers, 2000 |
| 3 | Lenhart S , Workman J T . Optimal Control Applied to Biological Models. New York: Taylor & Francis Group, 2007 |
| 4 | Leung A W . Nonlinear System of Partial Differential Equations: Applications to Life and Physical Sciences. Beijing: World Scientific Publishing, 2009 |
| 5 | Boucekkine R , Hritoenko N , Yatsenko Y . Optimal Control of Age-Structured Populations in Economy, Demography, and the Environment. New York: Routledge, 2011 |
| 6 |
Rorres C , Fair W . Optimal harvesting policy for an age-specific population. Mathematical Biosciences, 1975, 24: 31- 47
doi: 10.1016/0025-5564(75)90065-6 |
| 7 |
Brokate M . Pontryagin's principle for control problems in age-dependent population dynamics. J Math Biology, 1985, 23: 75- 101
doi: 10.1007/BF00276559 |
| 8 |
Medhin N G . Optimal harvesting in age-structured populations. J Optim Theor Appl, 1992, 74 (3): 413- 423
doi: 10.1007/BF00940318 |
| 9 |
Cañada A , Gámez J L , Montero J A . Study of an optimal control problem for diffusive nonlinear elliptic equations of Logistic type. SIAM J Control Optim, 1998, 36 (4): 1171- 1189
doi: 10.1137/S0363012995293323 |
| 10 |
Yamauchi A , Matsummiya Y , Iwasa Y . Optimal age-dependent sustainable harvesting of natural resouece populations: Sustainability value. Res Popul Ecol, 1997, 39 (2): 139- 148
doi: 10.1007/BF02765259 |
| 11 |
Gurtin M E , Murphy L F . On the optimal harvesting of persistent age-structured populations. J Math Biology, 1981, 13: 131- 148
doi: 10.1007/BF00275209 |
| 12 | Murphy L F , Smith S J . Optimal harvesting of an age-structured population. J Math Biology, 1990, 29: 77- 90 |
| 13 | Busoni G , Matucci S . A problem of optimal harvesting policy in two-stage age-dependent populations. Mathematical Biosciences, 1997, 43: 1- 33 |
| 14 |
Barbu V , Iannelli M . Optimal control of population dynamics. J Optim Theor Appl, 1999, 102: 1- 14
doi: 10.1023/A:1021865709529 |
| 15 |
Fister K R , Lenhart S . Optimal harvesting in an age-structured predator-prey model. Appl Math Optim, 2006, 54: 1- 15
doi: 10.1007/s00245-005-0847-9 |
| 16 |
Hritoenko N , Yatsenko Y . The structure of optimal time- and age-dependent harvesting in the Lotka-McKendrik population model. Mathematical Biosciences, 2007, 208: 48- 62
doi: 10.1016/j.mbs.2006.09.008 |
| 17 |
Zhao C , Zhao P , Wang M S . Optimal harvesting for nonlinear age-dependent population dynamics. Mathematical and Computer Modelling, 2006, 43: 310- 319
doi: 10.1016/j.mcm.2005.06.008 |
| 18 |
Luo Z . Optimal harvesting problem for an age-dependent n-dimensional food chain diffusion model. Applied Mathematics and Computation, 2007, 186: 1742- 1752
doi: 10.1016/j.amc.2006.08.168 |
| 19 |
Lu D , Gu J , Wang X . Optimal harvesting problems for an age-dependent n-dimensional food chain model with diffusion. Applied Mathematics and Computation, 2007, 184: 659- 668
doi: 10.1016/j.amc.2006.06.065 |
| 20 |
He Z R . Opitmal harvesting of two competing species with age dependence. Nonlinear Analysis: RWA, 2006, 7: 769- 788
doi: 10.1016/j.nonrwa.2005.04.005 |
| 21 |
Braverman E , Braverman L . Optimal harvesting of diffusive models in a nonhomogeneous environment. Nonlinear Analysis, 2009, 71: e2173- e2191
doi: 10.1016/j.na.2009.04.025 |
| 22 |
Dewsbury D A . Dominance rank, copulatory behavior, and differential reproduction. The Quarterly Review of Biology, 1982, 57 (2): 135- 159
doi: 10.1086/412672 |
| 23 | Cushing J M , Li J . Oscillations caused by cannibalism in a size-structured population model. Canadian Applied Mathematics Quarterly, 1995, 3 (2): 155- 172 |
| 24 |
Cañada A , Saldana J . Asymptotic behaviour of a model of hierarchically structured population dynamics. Journal of Mathematical Biology, 1997, 35 (8): 967- 987
doi: 10.1007/s002850050085 |
| 25 |
Kraev E A . Existence and uniqueness for height structured hierarchical population models. Natural Resource Modeling, 2001, 14 (1): 45- 70
doi: 10.1111/j.1939-7445.2001.tb00050.x |
| 26 |
Jang R J , Cushing J M . A discrete hierarchical model of intra-specific competition. Journal of Mathematical Analysis and Applications, 2003, 280 (1): 102- 122
doi: 10.1016/S0022-247X(03)00050-7 |
| 27 | Ackleh A S , Deng K . Monotone approximation for a hierarchical age-structured population model. Dynamics of Continuous, Discrete and Impulsive Systems, 2005, 2 (2): 203- 214 |
| 28 |
Shen J , Shu C W , Zhang M . A high order WENO Scheme for a hierarchical size-structured population model. Journal of Scientific Computing, 2007, 33 (3): 279- 291
doi: 10.1007/s10915-007-9152-x |
| 29 | Liu Y , He Z . On the well-posedness of a nonlinear hierarchical size-structured population model. ANZIAM Journal, 2017, 58 (3/4): 482- 490 |
| 30 |
He Z R , Ni D , Wang S P . Optimal harvesting of a hierarchical age-structured population system. International Journal of Biomathematics, 2019, 12 (8): 1950091
doi: 10.1142/S1793524519500918 |
| 31 | 何泽荣, 周楠, 韩梦杰. 年龄等级结构两种群系统模型解的存在唯一性. 数学进展, 2020, 49 (6): 713- 722 |
| He Z R , Zhou N , Han M J . On the system model of two hierarchical age-structured populations. Advances in Mathematics, 2020, 49 (6): 713- 722 | |
| 32 | Yosida K . Functional Analysis. Beijing: Beijing World Publishing Corporation, 1999 |
| 33 | Barbu V . Mathematical Methods in Optimization of Differential Systems. Dordrecht: Kluwer Academic Publishers, 1994 |
| [1] | Zerong He,Mengjie Han. Optimal Control of Initial Distributions in a Hierarchical Size-Structured Population System with Delay [J]. Acta mathematica scientia,Series A, 2021, 41(4): 1181-1191. |
| [2] |
Qionglin Liu,Yinghui Tang.
Analysis of |
| [3] | Zerong He,Zhiqiang Zhang,Yang Wang. Stability of a Class of Nonlinear Hierarchical Age-Dependent Population Model [J]. Acta mathematica scientia,Series A, 2020, 40(6): 1712-1722. |
| [4] | Ping Shi. Existence and Uniqueness of Fixed Points for a Class of Abstract Binary Nonlinear Operators [J]. Acta mathematica scientia,Series A, 2020, 40(4): 882-890. |
| [5] | Zerong He,Zhiqiang Zhang,Zheyong Qiu. Numerical Method of a Nonlinear Hierarchical Age-Structured Population Model [J]. Acta mathematica scientia,Series A, 2020, 40(2): 515-526. |
| [6] | Le Luo,Yinghui Tang. System Capacity Optimization Design and Optimal Control Policy (N*, D*) for M/G/1 Queue with p-Entering Discipline and Min(N, D, V)-Policy [J]. Acta mathematica scientia,Series A, 2019, 39(5): 1228-1246. |
| [7] | Maoning Tang,Qingxin Meng. Linear-Quadratic Optimal Control Problems for Mean-Field Backward Stochastic Differential Equations with Jumps [J]. Acta mathematica scientia,Series A, 2019, 39(3): 620-637. |
| [8] | Runxin Li,Hui Huang,Zhenhong Shang,Yu Cao,Hongbin Wang,Jing Zhang. Lagrange-Like Multiplier Rules for Weak Approximate Pareto Solutions of Multiobjective Constrained Vector Optimization Problems [J]. Acta mathematica scientia,Series A, 2018, 38(6): 1076-1094. |
| [9] | Quyu Pan,Yinghui Tang. Analysis of M/G/1 Repairable Queueing System and Optimal Control Policy with a Replaceable Repair Facility Under Delay Min(N, D)-Policy [J]. Acta mathematica scientia,Series A, 2018, 38(5): 1014-1031. |
| [10] | Zhang Chunguo, Liu Yubiao, Liu Weiwei. Boundary Optimal Control for the Timoshenko Beam [J]. Acta mathematica scientia,Series A, 2018, 38(3): 454-466. |
| [11] | Gao Lijun, Tang Yinghui. M/G/1 Repairable Queueing System and Optimal Control Policy with Min(N,D)-Policy [J]. Acta mathematica scientia,Series A, 2017, 37(2): 352-365. |
| [12] | He Zerong, Yang Lizhi. A Weighted Population Model with Size-Structure: Stability and Optimal Harvesting [J]. Acta mathematica scientia,Series A, 2016, 36(3): 584-600. |
| [13] | HE Ze-Rong, LIU Rong, LIU Li-Li. Optimal Harvest Rate for a Population System Modeling Periodic Environment and Body Size [J]. Acta mathematica scientia,Series A, 2014, 34(3): 684-690. |
| [14] | LI Xing-Chang, TIAN Shi-Qin. On Fixed Points Theorems of a Class of Decreasing Operator and Applications [J]. Acta mathematica scientia,Series A, 2014, 34(3): 738-743. |
| [15] | ZHOU Shu-Qing, HU Zhen-Hua, PENG Dong-Yun. Global Regularity for Very Weak Solutions to Obstacle Promlems Corresponding to a Class of A-Harmonic Equations [J]. Acta mathematica scientia,Series A, 2014, 34(1): 27-38. |
|
||