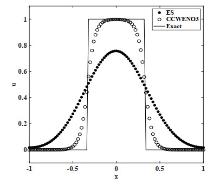
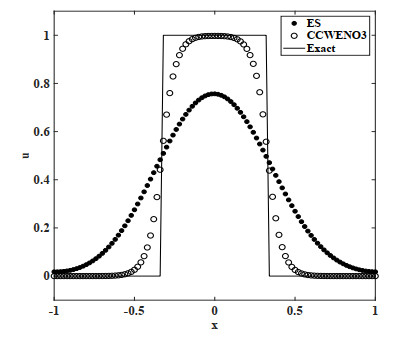
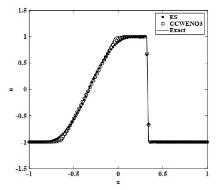
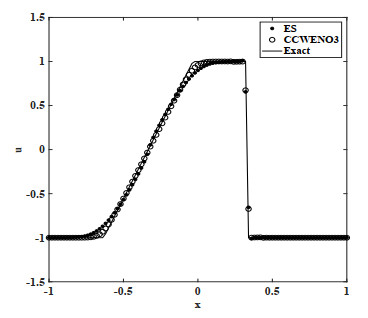
Acta mathematica scientia,Series A ›› 2021, Vol. 41 ›› Issue (5): 1296-1310.
Previous Articles Next Articles
High Order Sign Preserving Entropy Stable Schemes
Supei Zheng( ),Xia Xu*(
),Xia Xu*( ),Jianhu Feng(
),Jianhu Feng( ),Dou Jia(
),Dou Jia( )
)
- College of Science, Chang'an University, Xi'an 710064
-
Received:2020-05-27Online:2021-10-26Published:2021-10-08 -
Contact:Xia Xu E-mail:zsp2008@chd.edu.cn;xuxiachina@163.com;jhfeng@chd.edu.cn;1429594854@qq.com -
Supported by:the NSFC(11971075);the NSF of Shaanxi Province(2020JQ-338);the NSF of Shaanxi Province(2019JM-243)
CLC Number:
- O354
Cite this article
Supei Zheng,Xia Xu,Jianhu Feng,Dou Jia. High Order Sign Preserving Entropy Stable Schemes[J].Acta mathematica scientia,Series A, 2021, 41(5): 1296-1310.
share this article
"
| N | L1 error | Rate | L∞ error | Rate |
| 40 | 0.001979313043305 | 3.629516311932702e-04 | ||
| 80 | 2.726027476503843e-04 | 2.86 | 7.092855086397350e-05 | 2.36 |
| 160 | 3.522356279884232e-05 | 2.95 | 9.975669895746602e-06 | 2.83 |
| 320 | 4.405706993699641e-06 | 3.00 | 1.260137438287312e-06 | 2.99 |
| 640 | 5.373835462491922e-07 | 3.01 | 1.576597490888024e-07 | 3.00 |
"
| N | L1 error | Rate | L∞ error | Rate |
| 40 | 1.827054747602715e-04 | 1.448773504797440e-04 | ||
| 80 | 2.851024314352313e-05 | 2.68 | 1.785661053588239e-05 | 3.02 |
| 160 | 3.845862448196772e-06 | 2.89 | 2.226299234296333e-06 | 3.00 |
| 320 | 4.929538860536765e-07 | 2.96 | 2.782109209450295e-07 | 3.00 |
| 640 | 6.139221843903657e-08 | 3.01 | 3.478015911254420e-08 | 3.00 |
| 1 |
Lax P D . Weak solutions of nonlinear hyperbolic equation and their numerical computation. Commun Pur Appl Math, 1954, 7 (1): 159- 193
doi: 10.1002/cpa.3160070112 |
| 2 | Lax P D . Hyperbolic systems of conservation laws and the mathematical theory of shock waves. Reg Conf Ser Appl Math, 1973, 11, 1- 48 |
| 3 |
Tadmor E . The numerical viscosity of entropy stable schemes for systems of conservation laws. I Math Comp, 1987, 49 (179): 91- 103
doi: 10.1090/S0025-5718-1987-0890255-3 |
| 4 |
Lefloch P G , Mercier J M , Rohde C . Fully discrete, entropy conservative schemes of arbitrary order. SIAM J Numer Anal, 2002, 40 (5): 1968- 1992
doi: 10.1137/S003614290240069X |
| 5 |
Cheng X , Nie Y . A third-order entropy stable scheme for hyperbolic conservation laws. J Hyperbol Differ Eq, 2016, 13 (1): 129- 145
doi: 10.1142/S021989161650003X |
| 6 |
Fjordholm U S , Mishra S , Tadmor E . Arbitrarily high-order accurate entropy stable essentially nonoscillatory schemes for systems of conservation laws. SIAM J Numer Anal, 2012, 50 (2): 544- 573
doi: 10.1137/110836961 |
| 7 | Fjordholm U S , Ray D . A sign preserving WENO reconstruction method. SIAM J Sci Comput, 2015, 68 (1): 42- 63 |
| 8 |
Biswas B , Dubey R K . Low dissipative entropy stable schemes using third order WENO and TVD reconstructions. Adv Comput Math, 2018, 44 (4): 1153- 1181
doi: 10.1007/s10444-017-9576-2 |
| 9 |
Ismail F , Roe P L . Affordable, entropy-consistent Euler flux functions II: entropy production at shocks. J Comput Phys, 2009, 228 (15): 5410- 5436
doi: 10.1016/j.jcp.2009.04.021 |
| 10 |
Levy D , Puppo G , Russo G . Compact central WENO schemes for multidimensional conservation laws. SIAM J Sci Comput, 2000, 22 (2): 656- 672
doi: 10.1137/S1064827599359461 |
| 11 | Jameson A . Analysis and design of numerical schemes for gas dynamics, 1:Artificial diffusion, upwind biasing, limiters and their effect on accuracy and multigrid convergence. Comput Fluids, 1995, 4 (3/4): 171- 218 |
| 12 |
Gottlieb S , Shu C W . Total variation diminishing Runge-Kutta schemes. Math Comput, 1998, 67 (221): 73- 85
doi: 10.1090/S0025-5718-98-00913-2 |
| 13 |
Zakerzadeh H , Fjordholm U S . High-order accurate, fully discrete entropy stable schemes for scalar conservation laws. IMA J Numer Anal, 2016, 36 (2): 633- 654
doi: 10.1093/imanum/drv020 |
| 14 |
Jameson A . The construction of discretely conservative finite volume schemes that also globally conserve energy or entropy. J Sci Comput, 2008, 34 (2): 152- 187
doi: 10.1007/s10915-007-9171-7 |
| 15 |
Dehghan M , Jazlanian R . On the total variation of a third-order semi-discrete central scheme for 1D conservation laws. J Vib Control, 2011, 17 (9): 1348- 1358
doi: 10.1177/1077546310378870 |
| 16 | Puppo G A . Numerical entropy production for central schemes. SIAM J Sci Comput, 2003, 25 (4): 1382- 415 |
| 17 |
Tadmor E . Entropy stability theory for difference approximations of nonlinear conservation laws and related time-dependent problems. Acta Numer, 2003, 12, 451- 512
doi: 10.1017/S0962492902000156 |
| 18 | Shu C W. Essentially non-oscillatory and weighted essentially non-oscillatory schemes for hyperbolic conservation laws//Quarteroni A. Advanced Numer Appr Nonli Hyper Equa. Berlin: Springer-Verlag, 2006: 325-432 |
| 19 | Fjordholm U S , Mishra S , Tadmor E . Energy preserving and energy stable schemes for the shallow water equations. Found Comput Math, 2009, 363 (14): 93- 139 |
| 20 |
陈雨风, 陈停停, 王振. 非等熵Chaplygin气体测度值解存在性. 数学物理学报, 2020, 40A (4): 833- 841
doi: 10.3969/j.issn.1003-3998.2020.04.001 |
|
Chen Y F , Chen T T , Wang Z . The existence of the measure solution for the non-isentropic chaplygin gas. Acta Math Sci, 2020, 40A (4): 833- 841
doi: 10.3969/j.issn.1003-3998.2020.04.001 |
|
| 21 |
陈停停, 屈爱芳, 王振. 等熵Chaplygin气体的二维Riemann问题. 数学物理学报, 2017, 37A (6): 1053- 1061
doi: 10.3969/j.issn.1003-3998.2017.06.005 |
|
Chen T T , Qu A F , Wang Z . The two-dimensional riemann problem for isentropic chaplygin gas. Acta Math Sci, 2017, 37A (6): 1053- 1061
doi: 10.3969/j.issn.1003-3998.2017.06.005 |
|
| 22 | 吴宏伟. 可压缩磁流体动力方程解的正则性. 数学物理学报, 2010, 30A (3): 593- 602 |
| Wu H W . Regularity criteria for the compressible magneto-hydrodynamic equations. Acta Math Sci, 2010, 30A (3): 593- 602 | |
| 23 |
Tadmor E . Perfect derivatives, conservative differences and entropy stable computation of hyperbolic conservation laws. Discret Contin Dyn syst, 2016, 36 (8): 4579- 4598
doi: 10.3934/dcds.2016.36.4579 |
| No related articles found! |
|