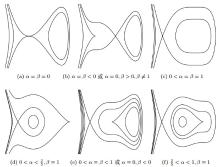
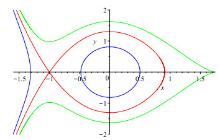
Acta mathematica scientia,Series A ›› 2021, Vol. 41 ›› Issue (4): 936-953.
Previous Articles Next Articles
Bifurcation of Limit Cycles from a Liénard System of Degree 4
Hongying Zhu1( ),Minzhi Wei1(
),Minzhi Wei1( ),Sumin Yang2,*(
),Sumin Yang2,*( ),Caoqing Jiang1(
),Caoqing Jiang1( )
)
- 1 Department of Applied Mathematics, Guangxi University of Finance and Economics, Nanning 530003
2 Department of Public Teaching, Guangxi Technological College of Machinery and Electricity, Nanning 530003
-
Received:2020-05-14Online:2021-08-26Published:2021-08-09 -
Contact:Sumin Yang E-mail:zhy71118@163.com;454742516@qq.com;smyang125@126.com;86072787@qq.com -
Supported by:the NSFC(11861009);the NSFC(11761011);the NSF of Guangxi(2020JJB110007);the Middle-Aged and Young Teachers' Basic Ability Promotion Project in Guangxi and Scientific Research Project(2020KY16020)
CLC Number:
- O175.12
Cite this article
Hongying Zhu,Minzhi Wei,Sumin Yang,Caoqing Jiang. Bifurcation of Limit Cycles from a Liénard System of Degree 4[J].Acta mathematica scientia,Series A, 2021, 41(4): 936-953.
share this article
| 1 | Arnold V I . Arnold's Problems. Berlin: Springer-Verlag, 2004 |
| 2 |
Arnold V I . Loss of stability of self-oscillations close to resonance and versal deformations of equivariant vector fields. Funct Anal Appl, 1977, 11, 85- 92
doi: 10.1007/BF01081886 |
| 3 | Arnold V I . Ten problems. Adv Soviet Math, 1990, 1, 1- 8 |
| 4 |
Chen F D , Li C Z , Llibre J , Zhang Z H . A unified proof on the weak Hilbert 16th problem for    doi: 10.1016/j.jde.2005.01.009 |
| 5 | Chen L , Ma X Z , Zhang G M , Li C Z . Cyclicity of several quadratic reversible systems with center of genus one. J Appl Anal Comput, 2011, 1 (4): 439- 447 |
| 6 | Christopher C , Li C Z . Limit Cycles of Differential Equations. Basel: Birkhäuser, 2007 |
| 7 |
Dumortier F , Li C Z . Perturbations from an elliptic Hamiltonian of degree four: (Ⅰ) Saddle loop and two saddle cycle. J Differ Equations, 2001, 176, 114- 157
doi: 10.1006/jdeq.2000.3977 |
| 8 |
Dumortier F , Li C Z . Perturbations from an elliptic Hamiltonian of degree four: (Ⅱ) Cuspidal loop. J Differ Equations, 2001, 175, 209- 243
doi: 10.1006/jdeq.2000.3978 |
| 9 |
Dumortier F , Li C Z . Perturbation from an elliptic Hamiltonian of degree four: Ⅲ Global center. J Differ Equations, 2003, 188, 473- 511
doi: 10.1016/S0022-0396(02)00110-9 |
| 10 | Dumortier F , Li C Z . Perturbations from an elliptic Hamiltonian of degree four: (Ⅳ) Figure eight-loop. J Differ Equations, 2003, 88, 512- 514 |
| 11 | Écalle J . Introduction Aux Fonctions Analysables et Preuve Constructive De la Conjecture De Dulac. French: Hermann, 1992 |
| 12 |
Gavrilov L . The infinitesimal 16th Hilbert problem in the quadratic case. Invent Math, 2001, 143, 449- 497
doi: 10.1007/PL00005798 |
| 13 |
Grau M , Mañosas F , Villadelprat J . A Chebyshev criterion for Abelian integrals. Trans Amer Math Soc, 2011, 363, 109- 129
doi: 10.1090/S0002-9947-2010-05007-X |
| 14 |
Han M A . Asymptotic expansions of Melnikov functions and limit cycle bifurcations. Int J Bifur Chaos, 2012, 22, 1250296
doi: 10.1142/S0218127412502963 |
| 15 |
Han M A . Existence of at most 1, 2, or 3 zeros of a melnikov function and limit cycles. J Differ Equations, 2001, 170 (2): 325- 343
doi: 10.1006/jdeq.2000.3828 |
| 16 |
Han M A . On Hopf cyclicity of planar systems. J Math Anal Appl, 2000, 245 (2): 404- 422
doi: 10.1006/jmaa.2000.6758 |
| 17 | Han M A , Wang Z , Zang H . Limit cycles by Hopf and homoclinic bifurcations for near-Hamiltonian systems. Chinese Ann Math Series A, 2007, 62 (5): 3214- 3234 |
| 18 |
Han M A , Yang J M , Tarta A A , Gao Y . Limit cycles near homoclinic and heteroclinic loops. J Dynam Differ Equat, 2008, 20, 923- 944
doi: 10.1007/s10884-008-9108-3 |
| 19 |
Han M A , Yang J M , Yu P . Hopf bifurcations for near-Hamiltonian systems. Inter J Bifur Chaos, 2009, 19 (12): 4117- 4130
doi: 10.1142/S0218127409025250 |
| 20 |
Hilbert D . Mathematical problems. Bull Amer Math Soc, 1902, 8, 437- 479
doi: 10.1090/S0002-9904-1902-00923-3 |
| 21 | Horozov E , Iliev I D . On the number of limit cycles in perturbations of quadratic Hamiltonian systems. Proc London Math Soc, 1994, 69, 198- 224 |
| 22 | Ilyashenko Y S . Finiteness Theorems for Limit Cycles. Providence RI: Amer Math Soc, 1991 |
| 23 |
Li C Z . Abelian integrals and limit cycles. Qual Theory Dyn Syst, 2012, 11, 111- 128
doi: 10.1007/s12346-011-0051-z |
| 24 |
李承治, 李伟固. 弱化希尔伯特第16问题及其研究现状. 数学进展, 2010, 39 (5): 513- 526
doi: 10.11845/sxjz.2010.39.05.0513 |
|
Li C Z , Li W G . Weak Hilbert's 16th problem and the relative research. Adv Math(CHINA), 2010, 39 (5): 513- 526
doi: 10.11845/sxjz.2010.39.05.0513 |
|
| 25 |
Li C Z , Zhang Z F . A criterion for determining the monotonicity of the ratio of two abelian integrals. J Differ Equations, 1996, 124, 407- 424
doi: 10.1006/jdeq.1996.0017 |
| 26 |
Li C Z , Zhang Z H . Remarks on 16th weak Hilbert problem for    doi: 10.1088/0951-7715/15/6/310 |
| 27 |
Li J B . Hilberts 16th problem and bifurcations of planar polynomial vector fields. Inter J Bifur Chaos, 2003, 13, 47- 106
doi: 10.1142/S0218127403006352 |
| 28 |
Liu C J . The cyclicity of period annuli of a class of quadratic reversible systems with two centers. J Differ Equations, 2012, 252 (10): 5260- 5273
doi: 10.1016/j.jde.2012.02.005 |
| 29 |
Liu C J , Xiao D M . The monotonicity of the ratio of two Abelian integrals. Tran the Ame Math Soc, 2013, 365 (10): 5525- 5544
doi: 10.1090/S0002-9947-2013-05934-X |
| 30 |
Manosas F , Villadelprat J . Bounding the number of zeros of certain Abelian integrals. J Differ Equations, 2011, 251 (6): 1656- 1669
doi: 10.1016/j.jde.2011.05.026 |
| 31 |
Petrov G S . Number of zeros of complete elliptic integrals. Funct Anal App, 1984, 18 (2): 148- 149
doi: 10.1007/BF01077834 |
| 32 | Pontryagin L S . On dynamical systems close to hamiltonian ones. Zh Exp Theor Phys, 1934, 4, 234- 238 |
| 33 | Smale S . Mathematical problems for the next century. Math Intelligencer, 1998, 2, 7- 15 |
| 34 |
Sun X B , Huang W T . Bounding the number of limit cycles for a polynomial Liénard system by using regular chains. J Symb Comput, 2017, 79, 197- 210
doi: 10.1016/j.jsc.2016.02.004 |
| 35 |
Sun X B , Su J , Han M A . On the number of zeros of Abelian integral for some Liénard system of type (4, 3). Chaos Soliton Fract, 2013, 51, 1- 12
doi: 10.1016/j.chaos.2013.02.003 |
| 36 | 孙宪波, 吴奎霖. 一类扰动超椭圆Hamilton系统的Abel积分零点个数上确界. 中国科学, 2015, 45 (6): 751- 764 |
| Sun X B , Wu K L . The sharp bound on the number of zeros of Abelian integral for a perturbation of hyper-elliptic Hamiltonian system. Science in China, 2015, 45 (6): 751- 764 | |
| 37 |
Sun X B , Xi H J , Zangeneh H R Z , Kazemi R . Bifurcation of limit cycles in small perturbation of a class of Liénard systems. Int J Bifur Chaos, 2014, 24 (01): 1450004
doi: 10.1142/S0218127414500047 |
| 38 |
Tian Y , Han M A . Hopf and homoclinic bifurcations for near-Hamiltonian systems. J Differ Equat, 2017, 262 (4): 3214- 3234
doi: 10.1016/j.jde.2016.11.026 |
| 39 |
Wang J H . Estimate of the number of zeros of Abelian integrals for a perturbation of hyperelliptic Hamiltonian system with nilpotent center. Chaos Soliton Fract, 2012, 45, 1140- 1146
doi: 10.1016/j.chaos.2012.05.011 |
| 40 |
Wang J H , Xiao D M . On the number of limit cycles in small perturbations of a class of hyperelliptic Hamiltonian systems with one nilpotent saddle. J Differ Equations, 2011, 250, 2227- 2243
doi: 10.1016/j.jde.2010.11.004 |
| 41 |
Xiao D M . Bifurcations on a five-parameter family of planar vector field. J Dyn Diff Equat, 2008, 20, 961- 980
doi: 10.1007/s10884-008-9109-2 |
| 42 | Zhang Z F , Li C Z . On the number of limit cycles of a class of quadratic Hamiltonian systems under quadratic perturbations. Adv in Math, 1997, 26, 445- 460 |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 282
|
|
|||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 106
|
|
|||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||
|