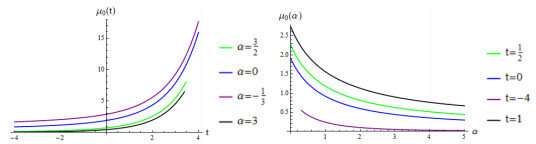
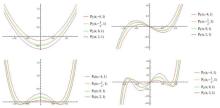
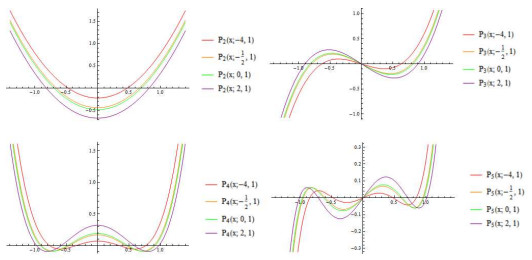
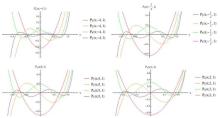
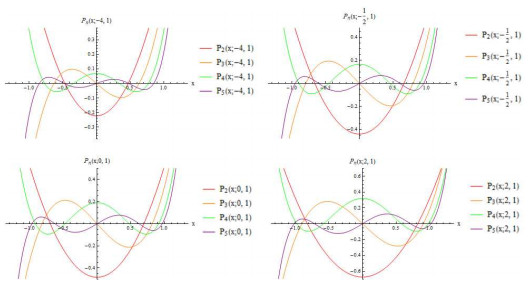
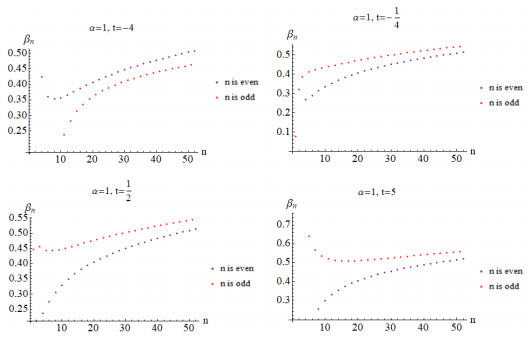
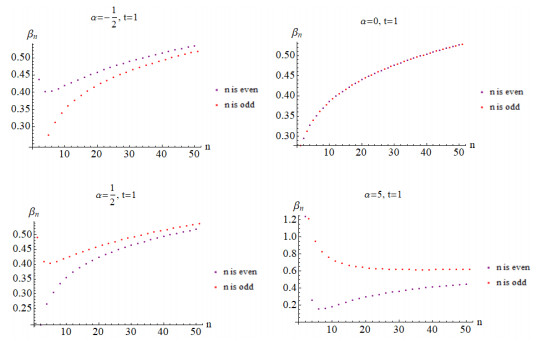
Acta mathematica scientia,Series A ›› 2021, Vol. 41 ›› Issue (4): 921-935.
Previous Articles Next Articles
A Generalised Decic Freud-Type Weight
Dan Wang1( ),Mengkun Zhu2,*(
),Mengkun Zhu2,*( ),Chen Yang1(
),Chen Yang1( ),Xiaoli Wang2(
),Xiaoli Wang2( )
)
- 1 Department of Mathematics, Faculty of Science and Technology, University of Macau, Avenida da Universidade, Macau Taipa 999078
2 School of Mathematics and Statistics, Qilu University of Technology(Shangdong Academy of Sciences), Jinan 250353
-
Received:2020-08-04Online:2021-08-26Published:2021-08-09 -
Contact:Mengkun Zhu E-mail:bohewan@126.com;zmk@qlu.edu.cn;yayangchen@um.edu.mo;wxlspu@qlu.edu.cn -
Supported by:the Macau Science and Technology Development Fund(FDCT023/2017/A1);the Universidade de Macau(MYRG2018-00125FST);the NSFC(11801292);the NSF of Guangdong Province(2021A1515010361)
CLC Number:
- O175.7
Cite this article
Dan Wang,Mengkun Zhu,Chen Yang,Xiaoli Wang. A Generalised Decic Freud-Type Weight[J].Acta mathematica scientia,Series A, 2021, 41(4): 921-935.
share this article
| 1 | Chihara T S . An Introduction to Orthogonal Polynomials. New York: Gordon and Breach, 1978 |
| 2 | Freud G . Othogonal Polynomials. Budapest, Hungary: Pergamon, 1971 |
| 3 | Van Assche W . Asymptotics for Orthogonal Polynomials. Berlin: Springer, 1987 |
| 4 | Freud G . On the coefficients in the recursion formulae of orthogonal polynomials. Proc R Irish Acad, 1976, 76, 1- 6 |
| 5 | Magnus A P. A Proof of Freud's Conjecture About the Orthogonal Polynomials Related to |x|ρexp(−x2m), for Integer m//Brezinski C, Draux A, Magnus A P, et al. Orthogoanl Polynomials and Applications. Berlin: Springer-Verlag, 1985: 362-372 |
| 6 |
Lubinsky D , Mhaskar H , Saff E . A proof of Freud's conjecture for exponential weights. Constr Approx, 1988, 4, 65- 83
doi: 10.1007/BF02075448 |
| 7 |
Magnus A P . On Freud's equations for the exponential weights. J Approx Theory, 1986, 46, 65- 99
doi: 10.1016/0021-9045(86)90088-2 |
| 8 |
Clarkson P A , Jordaan K , Kelil A . A generalized Freud weight. Stud Appl Math, 2016, 136, 288- 320
doi: 10.1111/sapm.12105 |
| 9 | Clarkson P A , Jordaan K . Properties of generalized Freud polynomials. J Approx Theory, 2018, 25, 148- 175 |
| 10 |
Bleher P , Its A R . Semiclassical asymptotics of orthogonal polynomials: Riemann-Hilbert problem, and universality in the matrix model. Ann of Math, 1999, 150, 185- 266
doi: 10.2307/121101 |
| 11 |
Bleher P , Its A R . Double scaling limit in the random matrix model: the Riemann-Hilbert approach. Comm Pure Appl Math, 2003, 56, 433- 516
doi: 10.1002/cpa.10065 |
| 12 |
Clarkson P A , Jordaan K . The relationship between semiclassical Laguerre polynomials and the fourth Painlevé equation. Constr Approx, 2014, 39, 223- 254
doi: 10.1007/s00365-013-9220-4 |
| 13 | Levin E , Lubinsky D . Orthogonal Polynomials with Exponential Weights. New York: Springer-Verlag, 2001 |
| 14 |
Lyu S L , Chen Y . The largest eigenvalue distribution of the Laguerre unitary ensemble. Acta Math Scientia, 2017, 37 (2): 439- 462
doi: 10.1016/S0252-9602(17)30013-9 |
| 15 |
Wong R , Zhang L . Global asymptotics of orthogonal polynomials associated with |x|2αe−Q(x). J Approx Theory, 2010, 162, 723- 765
doi: 10.1016/j.jat.2009.09.007 |
| 16 |
Bonan S , Clark D S . Estimates of the orthogonal polynomials with weight exp(−xm), m an even positive integer. J Approx Theory, 1986, 46, 408- 410
doi: 10.1016/0021-9045(86)90074-2 |
| 17 |
Bonan S , Nevai P . Orthogonal polynomials and their derivatives I. J Approx Theory, 1984, 40, 134- 147
doi: 10.1016/0021-9045(84)90023-6 |
| 18 |
Chen Y , Pruessner G . Orthogonal polynomials with discontinuous weights. J Phys A: Math Gen, 2005, 38, 191- 198
doi: 10.1088/0305-4470/38/12/L01 |
| 19 |
Wang D , Zhu M K , Chen Y . On semi-classical orthogonal polynomials associated with a Freud - type weight. Math Meth Appl Sci, 2020, 43 (8): 5295- 5313
doi: 10.1002/mma.6270 |
| 20 | Vein P R , Dale P . Determinants and Their Applications in Mathematical Physics. New York: Springer-Verlag, 1999 |
| 21 | Van Assche W. Discrete Painlevé Equations for Recurrence Coefficients of Orthogonal Polynomials//Elaydi S, Cushing J, Lasser R, et al. Difference Equations, Special Functions and Orthogonal Polynomials. Hackensack: World Scientific, 2007: 687-725 |
| 22 |
Cresswell C , Joshi N . The discrete first, second and thirty-fourth Painlevé hierarchies. J Phys A: Math Gen, 1999, 32, 655- 669
doi: 10.1088/0305-4470/32/4/009 |
| 23 | Damelin S B . Asymptotics of recurrence coefficients for orthonormal polynomials on the line-Magnus method revisited. Math Comp, 2004, 73, 191- 209 |
| 24 |
Chen Y , Ismail M E H . Thermodynamic relations of the Hermitian matrix ensembles. J Phys A: Math Gen, 1997, 30, 6633- 6654
doi: 10.1088/0305-4470/30/19/006 |
| 25 |
Chen Y , Lawrence N . On the linear statistics of Hermitian random matrices. J Phys A: Math Gen, 1998, 31, 1141- 1152
doi: 10.1088/0305-4470/31/4/005 |
| 26 |
Chen Y , Mckay M R . Coulomb fluid, Painlevé transcendents and the information theory of MIMO systems. IEEE Trans Inform Theory, 2012, 58, 4594- 4634
doi: 10.1109/TIT.2012.2195154 |
| 27 |
Zhu M K , Chen Y . On properties of a deformed Freud weight. Random Matrices Theory Appl, 2019, 8, 1950004
doi: 10.1142/S2010326319500047 |
| 28 |
Berg C , Chen Y , Ismail M E H . Small eigenvalues of large Hankel matrices: The indeterminate case. Math Scand, 2002, 91, 67- 81
doi: 10.7146/math.scand.a-14379 |
| 29 |
Berg C , Szwarc R . The smallest eigenvalue of Hankel matrices. Const Approx, 2011, 34, 107- 133
doi: 10.1007/s00365-010-9109-4 |
| 30 |
Chen Y , Lawrence N D . Small eigenvalues of large Hankel matrices. J Phys A: Math Gen, 1999, 32, 7305- 7315
doi: 10.1088/0305-4470/32/42/306 |
| 31 |
Chen Y , Lubinsky D S . Smallest eigenvalues of Hankel matrices for exponential weights. J Math Anal Appl, 2004, 293, 476- 495
doi: 10.1016/j.jmaa.2004.01.032 |
| 32 |
Emmart N , Chen Y , Weems C . Computing the smallest eigenvalue of large ill-conditioned Hankel matrices. Commun Comput Phys, 2015, 18, 104- 124
doi: 10.4208/cicp.260514.231214a |
| 33 |
Szegö G . On some Hermitian forms associated with two given curves of the complex plane. Trans Amer Math Soc, 1936, 40, 450- 461
doi: 10.1090/S0002-9947-1936-1501884-1 |
| 34 |
Widom H , Wilf H S . Small eigenvalues of large Hankel matrices. Proc Amer Math Soc, 1966, 17, 338- 344
doi: 10.1090/S0002-9939-1966-0189237-7 |
| 35 | Zhu M K , Chen Y , Emmart N , Weems C . The smallest eigenvalue of large Hankel matrices. Appl Math Comput, 2018, 334, 375- 387 |
| 36 |
Zhu M K , Emmart N , Chen Y , Weems C . The smallest eigenvalue of large Hankel matrices generated by a deformed Laguerre weight. Math Meth Appl Sci, 2019, 42, 3272- 3288
doi: 10.1002/mma.5583 |
| 37 | Chen Y , Sikorowski J , Zhu M K . Smallest eigenvalue of large Hankel matrices at critical point: Comparing conjecture with parallelised computation. Appl Math Comput, 2019, 363, 124628- 124646 |
| 38 |
Zhu M K , Chen Y , Li C Z . The smallest eigenvalue of large Hankel matrices generated by a singularly perturbed Laguerre weight. J Math Phys, 2020, 61, 073502
doi: 10.1063/1.5140079 |
| [1] | Jiafa Xu. Positive Solutions for a System of Boundary Value Problems of Fractional Difference Equations Involving Semipositone Nonlinearities [J]. Acta mathematica scientia,Series A, 2020, 40(1): 132-145. |
| [2] | Yang Jiashan, Li Tongxing. Oscillation for a Class of Second-Order Damped Emden-Fowler Dynamic Equations on Time Scales [J]. Acta mathematica scientia,Series A, 2018, 38(1): 134-155. |
| [3] | Xu Jia, Wang Yanxia, Dai Guowei. Fast Heteroclinic Solutions for a Difference Equation Related to Fisher-Kolmogorov's Equation [J]. Acta mathematica scientia,Series A, 2016, 36(6): 1145-1156. |
| [4] | Chen Kai. General Continuous Solutions of a Dhombres Type Functional Equation [J]. Acta mathematica scientia,Series A, 2016, 36(4): 690-702. |
| [5] | Liang Yunyun, Zhu Zeqi, Zhao Min, Zhao Caidi. Finite Fractal Dimension of Kernel Sections for Long-Wave-Short-Wave Resonance Equations on Infinite Lattices [J]. Acta mathematica scientia,Series A, 2015, 35(6): 1146-1157. |
|