具有Holling Ⅲ功能性反应的随机捕食食饵模型的平稳分布和周期解

Abstract

Abstract:

In this paper, we investigate the dynamics of stochastic predator-prey systems with Holling-type Ⅲ functional response. For the autonomous system, we firstly obtain that the system admits unique positive global solution starting from the positive initial value. Then, by comparison theorem for stochastic differential equation, sufficient conditions for extinction and persistence in mean are obtained. Thirdly, by constructing some suitable Lyapunov function, we prove that there are unique stationary distribution and they are ergodic. On the other hand, for the non-autonomous periodic system, we prove that there exists at least one nontrivial positive periodic solution according to the theory of Has'minskii. Finally, some numerical simulations are introduced to illustrate our theoretical result.

Key words: Predator-prey system, Random perturbation, Stationary distribution and ergodicity, Periodic solution

CLC Number:

O211.63

Guijie Lan,Yingjie Fu,Chunjin Wei,Shuwen Zhang. Stationary Distribution and Periodic Solution for Stochastic Predator-Prey Systems with Holling-Type Ⅲ Functional Response[J].Acta mathematica scientia,Series A, 2018, 38(5): 984-1000.

Trendmd

Figures/Tables 7

References 18

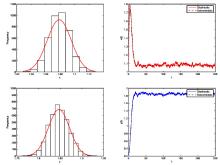
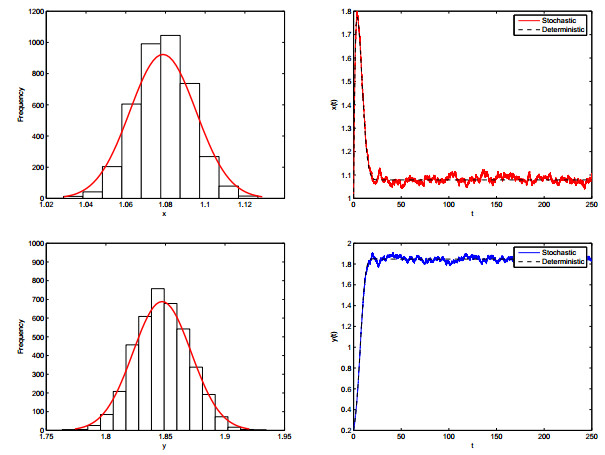
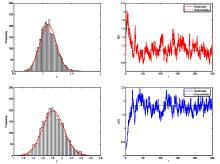
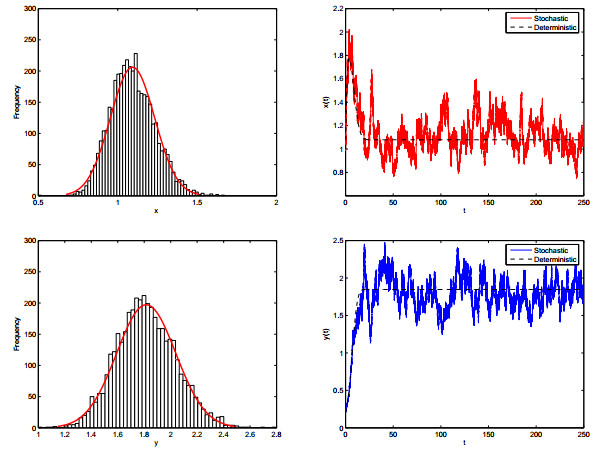
1	Cai Y L , Kang Y , Wang W M . A stochastic SIRS epidemic model with nonlinear incidence rate. Appl Math Comput, 2017, 305: 221- 240
2	Liu Q , Jiang D Q , Shi N Z , et al. Dynamical behavior of a stochastic HBV infection model with logistic hepatocyte growth. Acta Math Sci, 2017, 37B (4): 927- 940
3	魏凤英, 林青腾. 一类具有校正隔离率随机SIQS模型的绝灭性与分布. 数学物理学报, 2017, 37A (6): 1148- 1161
	Wei F Y , Lin Q T . Extinction and distribution for an SIQS epidemi model with quarantined-adjusted incidence. Acta Math Sci, 2017, 37A (6): 1148- 1161
4	Wang W M , Cai Y L , Li J L , Gui Z J . Periodic behavior in a FIV model with seasonality as well as environment fluctuations. J Franklin I, 2017, 354: 7410- 7428 doi: 10.1016/j.jfranklin.2017.08.034
5	Liu M , Mandal P S . Dynamical behavior of a one-prey two-predator model with random perturbations. Commun Nonlinear Sci, 2015, 28 (1/3): 123- 137
6	Ji C Y , Jiang D Q , Shi N Z . A note on a predator-prey model with modified Leslie-Gower and Holling-type Ⅱ schemes with stochastic perturbation. J Math Anal Appl, 2011, 377 (1): 435- 440 doi: 10.1016/j.jmaa.2010.11.008
7	Mandal P S , Banerjee M . Stochastic persistence and stationary distribution in a Holling-Tanner type prey-predator model. Physica A, 2012, 391 (4): 1216- 1233 doi: 10.1016/j.physa.2011.10.019
8	Jiang D Q , Zuo W J , Hayat T , Alsaedi A . Stationary distribution and periodic solutions for stochastic Holling-Leslie predator-prey systems. Physica A, 2016, 460: 16- 28 doi: 10.1016/j.physa.2016.04.037
9	Ji C Y , Jiang D Q , Shi N Z . Analysis of a predator-prey model with modified Leslie-Gower and Holling-type Ⅱ schemes with stochastic perturbation. J Math Anal Appl, 2009, 359 (2): 482- 498 doi: 10.1016/j.jmaa.2009.05.039
10	Zhao D L , Yuan S L . Dynamics of the stochastic Leslie-Gower predator-prey system with randomized intrinsic growth rate. Physica A, 2016, 461: 419- 428 doi: 10.1016/j.physa.2016.06.010
11	Xu Y , Liu M , Yang Y . Analysis of a stochastic two-predators one-prey system with modified Leslie-Gower and Holling-type Ⅱ schemes. J Appl Anal Comput, 2017, 7 (2): 713- 727
12	Liu M , Wang K . Dynamics of a two-prey one-predator system in random environments. J Nonlinear Sci, 2013, 23 (5): 751- 775 doi: 10.1007/s00332-013-9167-4
13	Qiu H , Liu M , Wang K , Wang Y . Dynamics of a stochastic predator-prey system with BeddingtonDeAngelis functional response. Appl Math Comput, 2012, 219 (4): 2303- 2312
14	Liu M , Wang K . Persistence, extinction and global asymptotical stability of a non-autonomous predatorprey model with random perturbation. Appl Math Model, 2012, 36 (11): 5344- 5353 doi: 10.1016/j.apm.2011.12.057
15	王克. 随机生物数学模型. 北京: 科学出版社, 2010
	Wang K . Random Biological Mathematical Model. Beijing: Science Press, 2010
16	Khasminskii R . Stochastic Stability of Differential Equations. Alphen aan den Rijn: Sijthoff & Noordhoff, 1980
17	Mao X R , Marion G , Renshaw E . Environmental Brownian noise suppresses explosions in population dynamics. Stoch Proc Appl, 2002, 97 (1): 95- 110 doi: 10.1016/S0304-4149(01)00126-0
18	Kloeden P , Platen E . Numerical Solution of Stochastic Differential Equations. Berlin: Springer, 1999

[1]	Yao Shaowen, Cheng Zhibo. Positive Periodic Solution for Damped Duffing Equation with Singularity [J]. Acta mathematica scientia,Series A, 2018, 38(3): 543-548.
[2]	Liang Zhiqing, Zeng Xiaping, Zhou Zewen, Pang Guoping, Huang Junhua. Existence of Periodic Solution of Holling-Tanner System with State Feedback Impulsive Control [J]. Acta mathematica scientia,Series A, 2018, 38(1): 174-189.
[3]	Li Zhouhong, Zhang Fengshuo, Cao Jinde, Alsaedi Ahmed, Alsaadi Fuad E. Almost Periodic Solution for a Non-Autonomous Two Species Competitive System with Feedback Controls on Time Scales [J]. Acta mathematica scientia,Series A, 2017, 37(4): 730-750.
[4]	Fu Jinbo, Chen Lansun. Positive Periodic Solution of Multiple Species Comptition System with Ecological Environment and Feedback Controls [J]. Acta mathematica scientia,Series A, 2017, 37(3): 553-561.
[5]	Duan Shuangshuang, Huang Shoujun. Blow up of Periodic Solutions for a Class of Keller-Segel Equations Arising in Biology [J]. Acta mathematica scientia,Series A, 2017, 37(2): 326-341.
[6]	Huang Zuda. Positive Pseudo Almost Periodic Solutions for a Delayed Nicholson's Blowflies Model with a Feedback Control [J]. Acta mathematica scientia,Series A, 2016, 36(3): 558-568.
[7]	Zhang Shuwen. Dynamics of a Predator-Prey System with Impulsive Perturbations and Markovian Switching [J]. Acta mathematica scientia,Series A, 2016, 36(3): 569-583.
[8]	Wei Hanyu, Xia Tiecheng. Quasi-Periodic Solution of the Generalized Broer-Kaup-Kupershmidt Soliton Equation [J]. Acta mathematica scientia,Series A, 2016, 36(2): 317-327.
[9]	Jiang Ani. Pseudo Almost Periodic Solutions for a Model of Hematopoiesis with an Oscillating Circulation Loss Rate [J]. Acta mathematica scientia,Series A, 2016, 36(1): 80-89.
[10]	Zhang Shuwen. A Stochastic Predator-Prey System with Time Delays and Prey Dispersal [J]. Acta mathematica scientia,Series A, 2015, 35(3): 592-603.
[11]	WANG Xue-Chen, WEI Jun-Jie. The Effect of Delay on a Diffusive Predator-Prey System with Ivlev-Type Functional Response [J]. Acta mathematica scientia,Series A, 2014, 34(2): 234-250.
[12]	LI Xiao-Pei. Generalized Homoclinic Solutions for the Stationary Extended Fisher-Kolmogorov Equation [J]. Acta mathematica scientia,Series A, 2014, 34(1): 126-138.
[13]	YE Hui, CAI Dong-Han. Global Attractivity in a Periodic Nicholson's Blowflies Model [J]. Acta mathematica scientia,Series A, 2013, 33(6): 1013-1021.
[14]	ZHANG Xing-Yong. Periodic Solutions for the First Order Hamiltonian System with Linear Part [J]. Acta mathematica scientia,Series A, 2013, 33(5): 894-905.
[15]	HU Guang-Ping, LI Xiao-Ling. Stationary Patterns of a Leslie-Gower Type Three Species Model with Cross-Diffusions [J]. Acta mathematica scientia,Series A, 2013, 33(1): 16-27.

Stationary Distribution and Periodic Solution for Stochastic Predator-Prey Systems with Holling-Type Ⅲ Functional Response

RichHTML

PDF (PC)

Abstract

Cite this article

share this article

Figures/Tables 7

References 18

Related Articles 15

Metrics

Comments

Recommended 0