Acta mathematica scientia,Series A ›› 2024, Vol. 44 ›› Issue (5): 1352-1367.
Previous Articles Next Articles
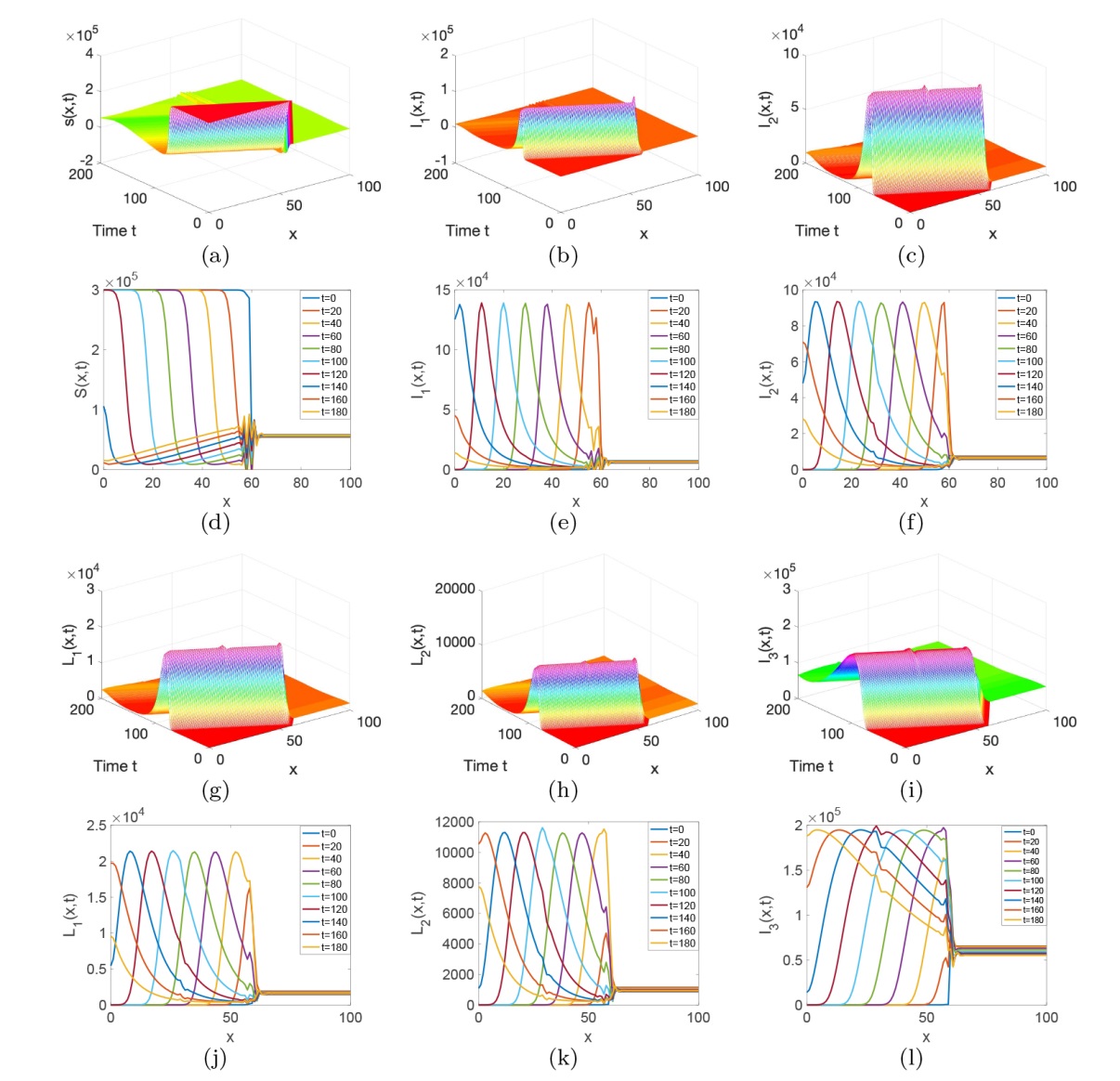
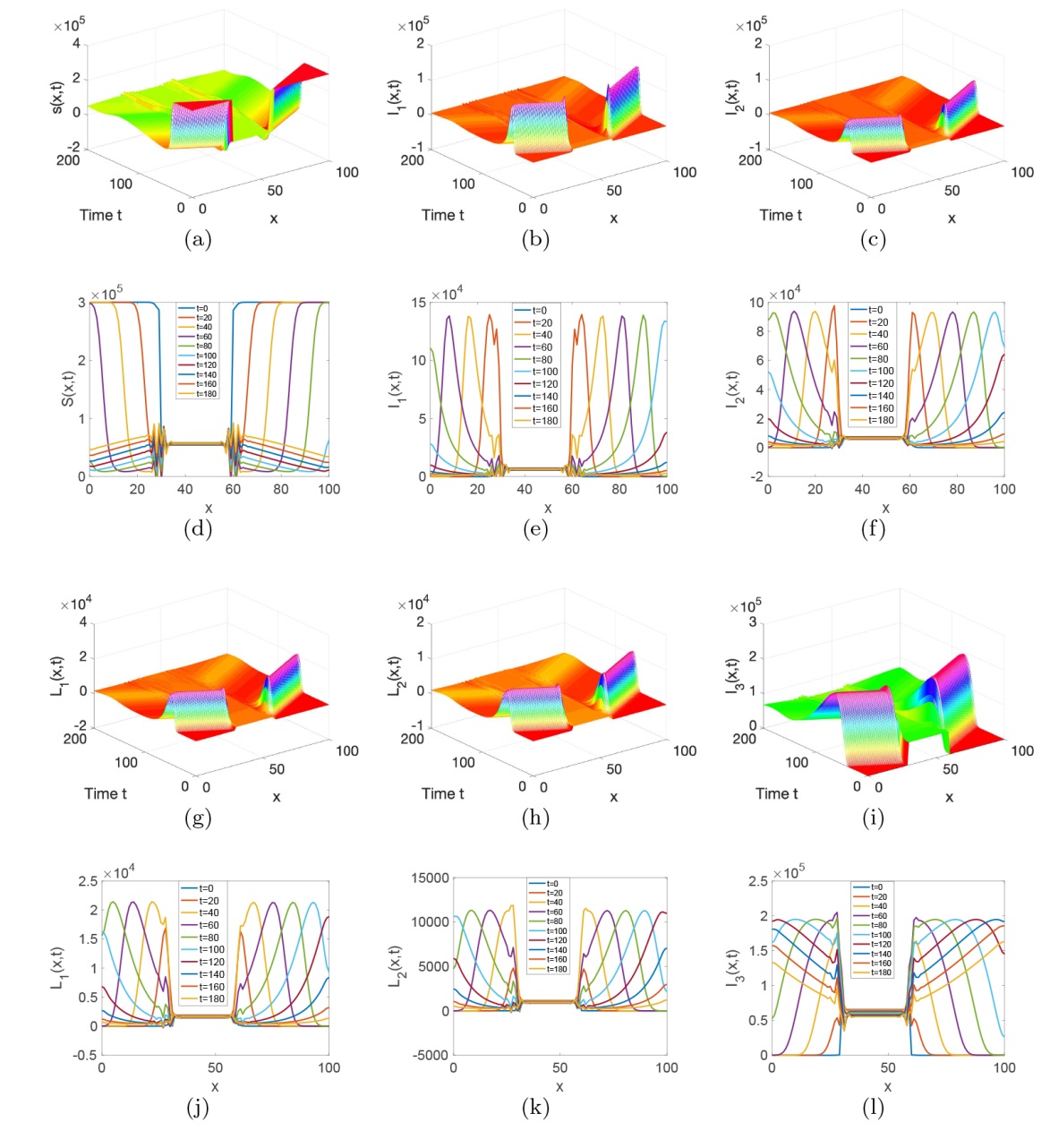
Dynamical Analysis and Numerical Simulation of a Syphilis Epidemic Model with Heterogeneous Spatial Diffusion
- 1School of Sciences, Hangzhou Dianzi University, Hangzhou 310018
2School of Data Sciences, Zhejiang University of Finance and Economics, Hangzhou 310018
-
Received:2023-07-11Revised:2024-04-16Online:2024-10-26Published:2024-10-16 -
Supported by:NSFC(12201557);NSFC(12001483);Foundation of Zhejiang Provincial Education Department(Y202249921);Anhui Provincial Natural Science Foundation(2108085MA10)
CLC Number:
- O175.23
Cite this article
Wu Peng, Fang Cheng. Dynamical Analysis and Numerical Simulation of a Syphilis Epidemic Model with Heterogeneous Spatial Diffusion[J].Acta mathematica scientia,Series A, 2024, 44(5): 1352-1367.
share this article
| [1] | Sparling T F. Natural history of syphilis// Helmes KK, et al. Sexually Transmitted Diseases, New York: McGrans-Hill Book co. 1981: 298-299 |
| [2] |
Mathew A B, Michael M, et al. Global phylogeny of Treponema pallidum lineages reveals recent expansion and spread of contemporary syphilis. Nature Microbiology, 2021, 6(12): 1549-1560
doi: 10.1038/s41564-021-01000-z pmid: 34819643 |
| [3] | National Institute of Infectious Diseases, Infectious agents surveillance report, 2020, https://www.niid.go.jp/niid/en/iasr-e.html [2023-6-11] |
| [4] | Garnett G P, Aral S O, Hoyle D V, et al. The natural history of syphilis: Implications for the transmission dynamics and control of infection. Sexually Transmitted Dis, 1997, 24(4): 185-200 |
| [5] |
Fenton K A, Breban R, Vardavas R, et al. Infectious syphilis in high-income settings in the 21st century. Lancet Infect Dis, 2008, 8(4): 244-253
doi: 10.1016/S1473-3099(08)70065-3 pmid: 18353265 |
| [6] | Grassly C, Fraser C, Garnett G P. Host immunity and synchronized epidemics of syphilis across the United States. Nature, 2005, 433(7024): 417-421 |
| [7] | Iboi E, Okuonghaea D. Population dynamics of a mathematical model for syphilis. Appl Math Model, 2016, 40: 3573-3590 |
| [8] |
Saad-Roy C M, Shuai Z, van den Driessche P. A mathematical model of syphilis transmission in an MSM population. Math Biosci, 2016, 277: 59-70
doi: 10.1016/j.mbs.2016.03.017 pmid: 27071977 |
| [9] | Gumel A B, Lubuma J M-S, Sharomi O, Terefe Y A. Mathematics of a sex-structured model for syphilis transmission dynamics. Math Meth Appl Sci, 2017, 23: 26-47 |
| [10] | Nwankwo A, Okuonghae D. Mathematical analysis of the transmission dynamics of HIV syphilis co-infection in the presence of treatment for syphilis. Bull Math Biol, 2018, 80: 437-492 |
| [11] | Omame A, Okuonghae D, Nwafor U E, et al. A co-infection model for HPV and syphilis with optimal control and cost-effectiveness analysis. Int J Biomath, 2021, 14: 2150050 |
| [12] | 吴鹏, 赵洪涌. 基于空间异质反应扩散 HIV 感染模型的最优治疗策略. 应用数学学报, 2022, 45(5): 752-766 |
| Wu P, Zhao H. Optimal treatment strategies for a reaction-dffusion HIV infection model with spatial heterogeneity. Acta Math Appl Sinica, 2022, 45(5): 752-766 | |
| [13] |
Wang X, Wang H, Michael Y L. Modeling rabies transmission in spatially heterogeneous environments via  |
| [14] | Gao Y, Wang J. Threshold dynamics of a delayed nonlocal reaction-diffusion HIV infection model with both cell-free and cell-to-cell transmissions. J Math Anal Appl, 2020, 488: 1-21 |
| [15] | Smith H L. Monotone Dynamical Systems: An Introduction to the Theory of Competitive and Cooperative Systems. Providence, RI: American Mathematical Society, 1995 |
| [16] | 叶其孝, 李元正, 王明新, 吴雅萍. 反应扩散方程引论. 北京: 科学出版社, 2016 |
| Ye Q X, Li Z Y, Wang M X, Wu Y P. Introduction to Reaction Diffusion Equations. Beijing: Science Press, 2016 | |
| [17] | Martin R H, Smith H L. Abstract functional differential equations and reaction-diffusion systems. Trans Am Math Soc, 1990, 321: 1-44 |
| [18] | Wu J. Theory and Applications of Partial Functional-Differential Equations. Vol 119. New York: Springer, 1996 |
| [19] | Hale J. Asymptotic Behavior of Dissipative Systems. Province, RI: American Mathematical Society, 1988 |
| [20] | Pazy A. Semigroups of Linear Operators and Applications to Partial Differential Equations. Applied Mathematical Science. New York: Springer-Verlag, 1983 |
| [21] |
Ren X, Tian Y, Liu L, Liu X. A reaction-diffusion within-host HIV model with cell-to-cell transmission. J Math Biol, 2018, 76(7): 1831-1872
doi: 10.1007/s00285-017-1202-x pmid: 29305736 |
| [1] | Zhang Xiao, Zhang Hongwu. Fractional Tikhonov Regularization Method for an Inverse Boundary Value Problem of the Fractional Elliptic Equation [J]. Acta mathematica scientia,Series A, 2024, 44(4): 978-993. |
| [2] | Wu Peng, He Zerong. Spatio-temporal Dynamics of HIV Infection Model with Periodic Antiviral Therapy and Nonlocal Infection [J]. Acta mathematica scientia,Series A, 2024, 44(1): 209-226. |
| [3] | Zhong Yi, Wang Yi, Jiang Tianhe. Dynamic Analysis and Optimal Control of an SIAQR Transmission Model with Asymptomatic Infection and Isolation [J]. Acta mathematica scientia,Series A, 2023, 43(6): 1914-1928. |
| [4] | Wu Peng,Wang Xiunan,He Zerong. Dynamical Analysis of an Age-Space Structured HIV/AIDS Model with Homogeneous Dirichlet Boundary Condition [J]. Acta mathematica scientia,Series A, 2023, 43(3): 970-984. |
| [5] | Zhenxiang Hu,Linfei Nie. Analysis of a Reaction-Diffusion Epidemic Model with Horizontal Transmission and Environmental Transmission [J]. Acta mathematica scientia,Series A, 2022, 42(6): 1849-1860. |
| [6] | Kai Wang,Hongyong Zhao. Traveling Wave of a Reaction-Diffusion Dengue Epidemic Model with Time Delays [J]. Acta mathematica scientia,Series A, 2022, 42(4): 1209-1226. |
| [7] | Tailei Zhang,Junli Liu,Mengjie Han. Dynamics of an Anthrax Epidemiological Model with Time Delay and Seasonality [J]. Acta mathematica scientia,Series A, 2022, 42(3): 851-866. |
| [8] | Hongwu Zhang. One Regularization Method for a Cauchy Problem of Semilinear Elliptic Equation [J]. Acta mathematica scientia,Series A, 2022, 42(1): 45-57. |
| [9] | Yu Yang. Global Attractivity of a Nonlocal Delayed and Diffusive SVIR Model [J]. Acta mathematica scientia,Series A, 2021, 41(6): 1864-1870. |
| [10] | Dandan Sun,Yingke Li,Zhidong Teng,Tailei Zhang. Analysis of the Stability for Measles Epidemic Model with Age-Structured [J]. Acta mathematica scientia,Series A, 2021, 41(6): 1950-1968. |
| [11] | Lixiang Feng,Defen Wang. Global Stability of an Epidemic Model with Quarantine and Incomplete Treatment [J]. Acta mathematica scientia,Series A, 2021, 41(4): 1235-1248. |
| [12] | Xiaojie Jing, Aimin Zhao, Guirong Liu. Global Stability of a Measles Epidemic Model with Partial Immunity and Environmental Transmission [J]. Acta mathematica scientia,Series A, 2019, 39(4): 909-917. |
| [13] | Xiaomin Yang,Zhipeng Qiu,Ling Ding. Complex Dynamics of an Intraguild Predation Model [J]. Acta mathematica scientia,Series A, 2019, 39(4): 963-970. |
| [14] | Zhang Liping, Zhao Yu, Yuan Sanling. Threshold Dynamical Behaviors of a Stochastic SIS Epidemic Model with Nonlinear Incidence Rate [J]. Acta mathematica scientia,Series A, 2018, 38(1): 197-208. |
| [15] | Wei Aiju, Zhang Xinjian, Wang Junyi, Li Kezan. Dynamics Analysis of an Ebola Epidemic Model [J]. Acta mathematica scientia,Series A, 2017, 37(3): 577-592. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 239
|
|
|||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 61
|
|
|||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||
|